Sound & Vibration

DOI: 10.32604/sv.2022.012085

ARTICLE

A Complete Analysis of Clarity (C50) Using I-SIMPA to Maintain Ideal Conditions in an Acoustic Chamber

R. Adithya Pillai1, S. Sakthivel Murugan2,* and Guruprasad Gupta1

1Department of Electrical and Electronics Engineering, Sri Sivasubramaniya Nadar College of Engineering, Chennai, 603110, India
2Department of Electronics and Communication Engineering, Sri Sivasubramaniya Nadar College of Engineering, Chennai, 603110, India
*Corresponding Author: S. Sakthivel Murugan. Email: sakthivels@ssn.edu.in
Received: 14 June 2020; Accepted: 20 October 2020

1  Introduction

Sound in a medium propagates in the form of a wave. A fraction of these sound waves are reflected off the surfaces in a closed environment while the rest of the sound waves are absorbed by the surface medium. Due to this, the intensity of the sound wave initially propagated from the source differs with the position of the audience in the room. Several parameters affect the intensity of sound waves in a room. Acoustic parameters like Clarity (C50) [1] that are key to the auditory comfort of the audience need to be studied in detail to determine a strategy to maintain conditions of acoustic ideality in a room. The audience, being the most important part of the room, need to be in a state of auditory satisfaction, irrespective of their position in the closed room. The surface absorption coefficients, commercially known as the Sound Transmission Class (STC), also affect the clarity and intensity of the sound in a walled room. Various simulations using I-SIMPA, an open source software are performed to determine the conditions of acoustic ideality in the closed environment. These simulations have been performed by varying the surface absorption percentages of the floor, ceiling and walls of the acoustic model, keeping in mind the effect of the external environment.

2  System Design

The simple chamber for testing is designed with a single source and two receivers as shown in Fig. 1. The dimension and positions of the source and receivers are as follows:

•   Chamber Dimensions: 9.3 m × 7.7 m × 2.8 m (width × length × height) [2].

•   Source position: (4.5, 0.5, 1.2).

•   Receiver 1 position: (3.5, 1, 1.2), Receiver 2 position: (5.5, 1, 1.2).

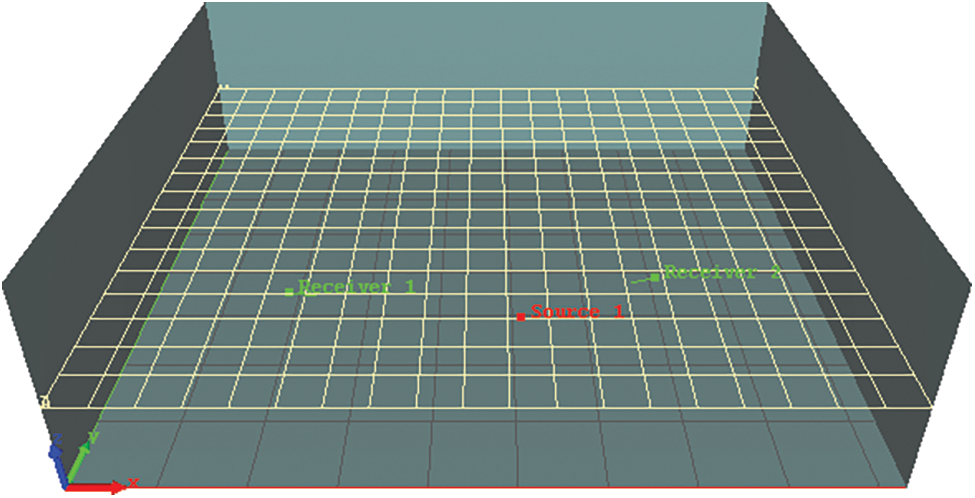
Figure 1: Original dimensions

The various parameters considered prior to performing the simulation are listed in Table 1. The calculation method preferred is Simulation de la Propagation de Particules Sonores (abbreviated as SPPS) method owing to the advantages offered by the sound particle concept over the sound ray concept in calculation and modelling [3]. Energetic type of calculation is preferred for modelling over the ‘random modelling’ calculation type due to the fact that the energy of the particle depends on the atmospheric absorption and the absorption coefficients of the material [4].

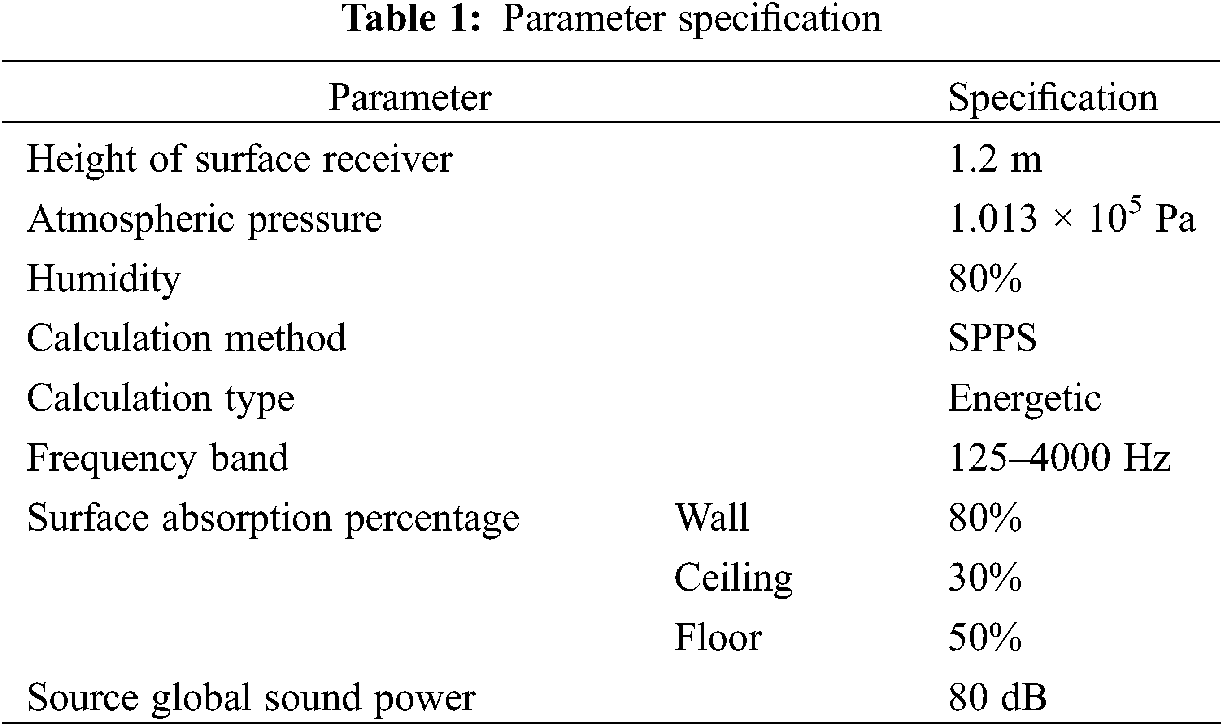
The environmental parameters that need to be considered can be defined in the properties and the various environmental properties considered for this analysis are shown in Fig. 2. Atmospheric absorption is negligible at standard temperature and pressure conditions [5].

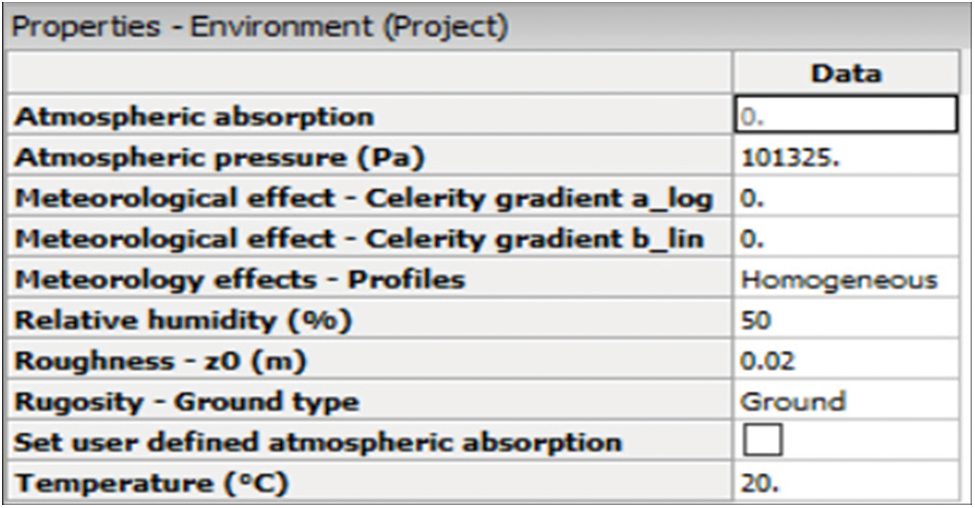
Figure 2: Environmental properties

The work carried in this paper is represented as two parts. In the first part, the theoretical analysis of the chamber with its performance analysis is presented and the second part concentrates on the simulation analysis of the same and variation with wall absorption percentages and chamber dimensions. The schematic of workflow is shown in Fig. 3.

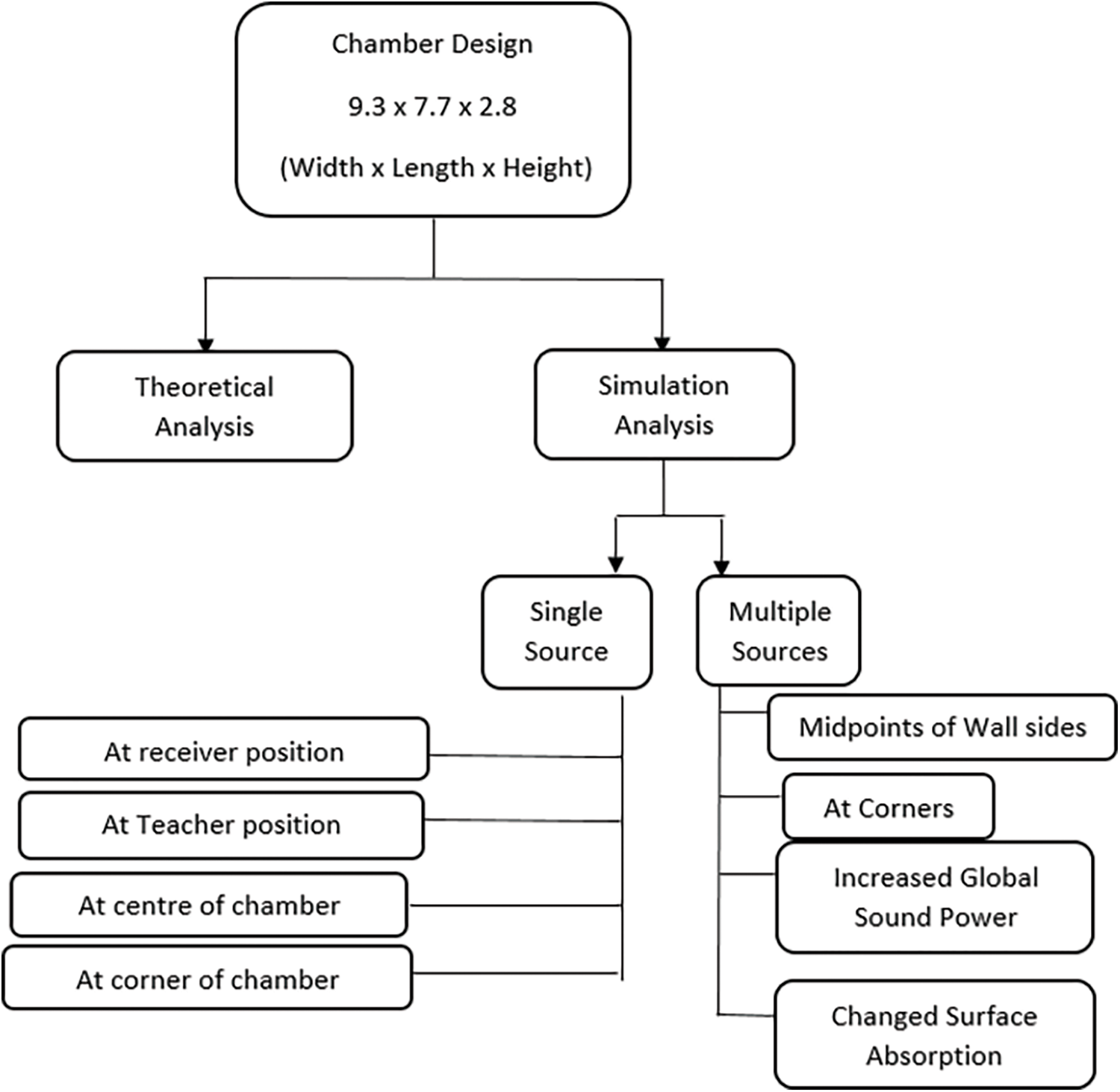
Figure 3: Schematic of the workflow in the paper

3  Theoretical Analysis

The theoretical analysis Clarity C50 was carried out and verified by using the standard equation pertaining to its mathematical calculations.

In definitive terms, C50 is the ratio between the early sound energy (between 0 and 50 ms) and late sound energy (50 ms onwards) [6]. The C-50 values in dB, generally decreases as the distance from the source increases. Being a measure of intelligibility of speech in an environment, Clarity (C50) is defined in terms of the time limit after which the intelligibility becomes detrimental. The critical time limit is 50 ms. The anticipated value C50,E for the definition measure C50 was theoretically calculated by means of the formula used in the EASERA Appendix [7] as given below:

C50,E=10log10γs(rHrx)2+1−e−13.8.0.05Te−13.8.0.05TdB (1)

where,

rx-distance from sound source to listener in m

rH-Half room diffuse-field distance

rH=0.057VTm (2)

V-Volume in m3

T-reverberation time in s

γ s–Front-to-random factor of a normal speaker characteristic

Reverberation Time was calculated using the standard Sabine’s formula [8]. According to Sabine

RT=0.161×VSas (3)

where

•   V is the volume of the room/system under consideration (in m3).

•   Sa is in Sabins, which is the total surface absorption of the room/system under consideration.

•   Sa = S x a where a is the absorption coefficient of the surface under consideration.

•   S is the surface absorption of a particular surface, for instance, the ceiling.

•   The factor 0.161 is obtained during derivation of Sabine’s formula and has a unit of seconds/meter. It can be expanded as (24×ln⁡10)/c20 .

•    c20=343m/s , is the speed of sound at 20°C.

For a source transmitting frequency of 500 Hz, the reverberation time (RT60) is calculated for different values of absorption coefficients of walls, ceiling and floor. The total Sabine absorption [6] and Reverberation time (RT60) obtained using (3) for various coefficients are shown in Table 2.

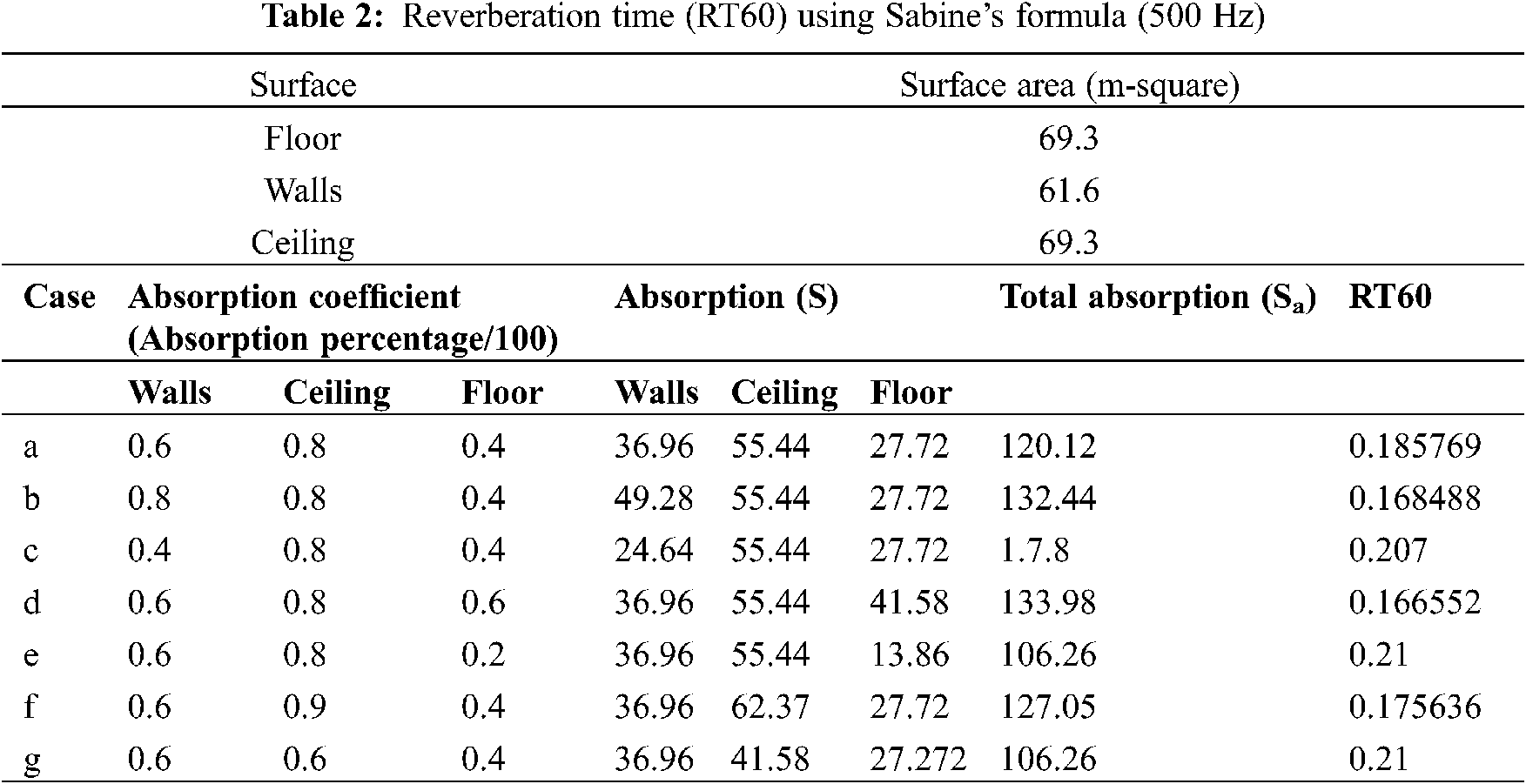
The theoretically calculated RT60 for various coefficients in Table 2 are modelled below. The verification of the above table for RT60 using I-SIMPA is shown in Fig. 4.

Figure 4: Simulation output for RT60 verification (a): Walls-60%, Ceiling-80%, Floor-40% -0.18 s (b): Walls-80%, Ceiling-80%, Floor-40%-0.17 s (c): Walls-40%, Ceiling-80%, Floor-40%-0.22 s (d): Walls-60%, Ceiling-80%, Floor-60%-0.16 s (e): Walls-60%, Ceiling-80%, Floor-20%-0.21 s (f): Walls-60%, Ceiling-90%, Floor-40%-0.17 s (g): Walls-60%, Ceiling-60%, Floor-40%-0.21 s

Figs. 4a–4g depict the simulation output for RT60 with different values of surface absorption coefficients according to Table 2. A comparison of the values obtained by simulation and theoretical analysis is shown in Table 3.

It is clear from Table 3 that the values of RT60 from the simulation and using the theoretical calculations carried out for the same simulation settings are nearly equal. This implies that I-SIMPA is an efficient tool that can be used for analysis of reverberation times. Although the I-Simpa simulations indicate the values of RT15, it can be assumed that the values of RT60 and RT15 are approximately equal due to the “Sabine-like” nature of the room [9]. The varying sound intensities at different points in a chamber can cause great discomfort to the audience present in the area of sound reception [10].

Clarity (C50) is calculated by using the formula (1) obtained from the EASARA appendix, for different values of absorption coefficients of walls, ceiling, and floor as per Table 2. The theoretical values calculated for Clarity (C50) are shown in Table 4.

Figs. 5a–5g depicts the simulation output for C50 with different values of surface absorption coefficients according to Table 2. A comparison of the values obtained by simulation and theoretical analysis is shown in Table 5.

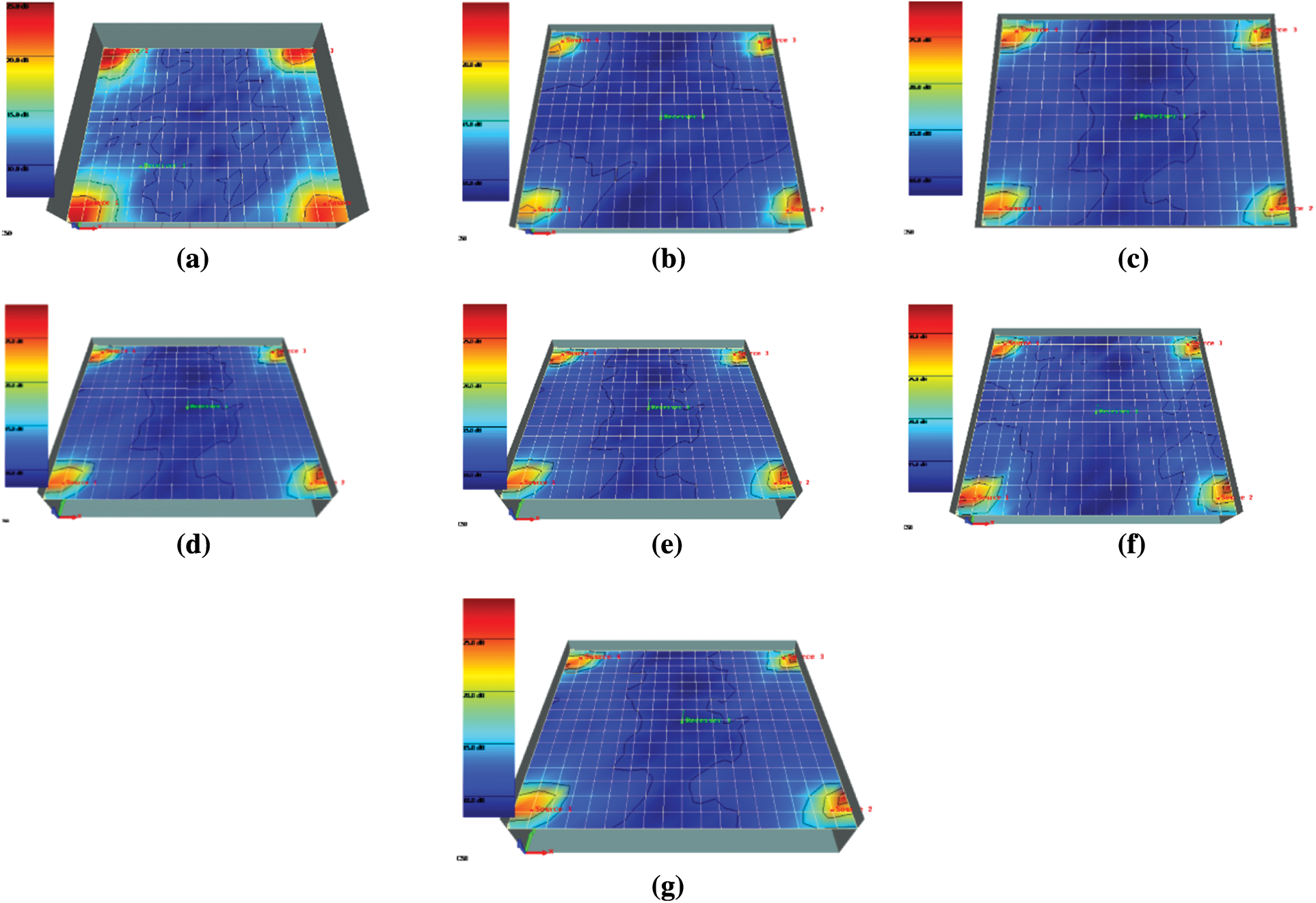
Figure 5: Simulation output for C50 verification (a): Walls-60%, Ceiling-80%, Floor-40%-8.77 dB (b): Walls-80%, Ceiling-80%, Floor-40%-9.62 dB (c): Walls-40%, Ceiling-80%, Floor-40%-7.92 dB (d): Walls-60%, Ceiling-80%, Floor-60%-9.78 dB (e): Walls-60%, Ceiling-80%, Floor-20%-7.92 dB (f): Walls-60%, Ceiling-90%, Floor-40%-9.59 dB (g): Walls-60%, Ceiling-60%, Floor-40%-7.92 dB

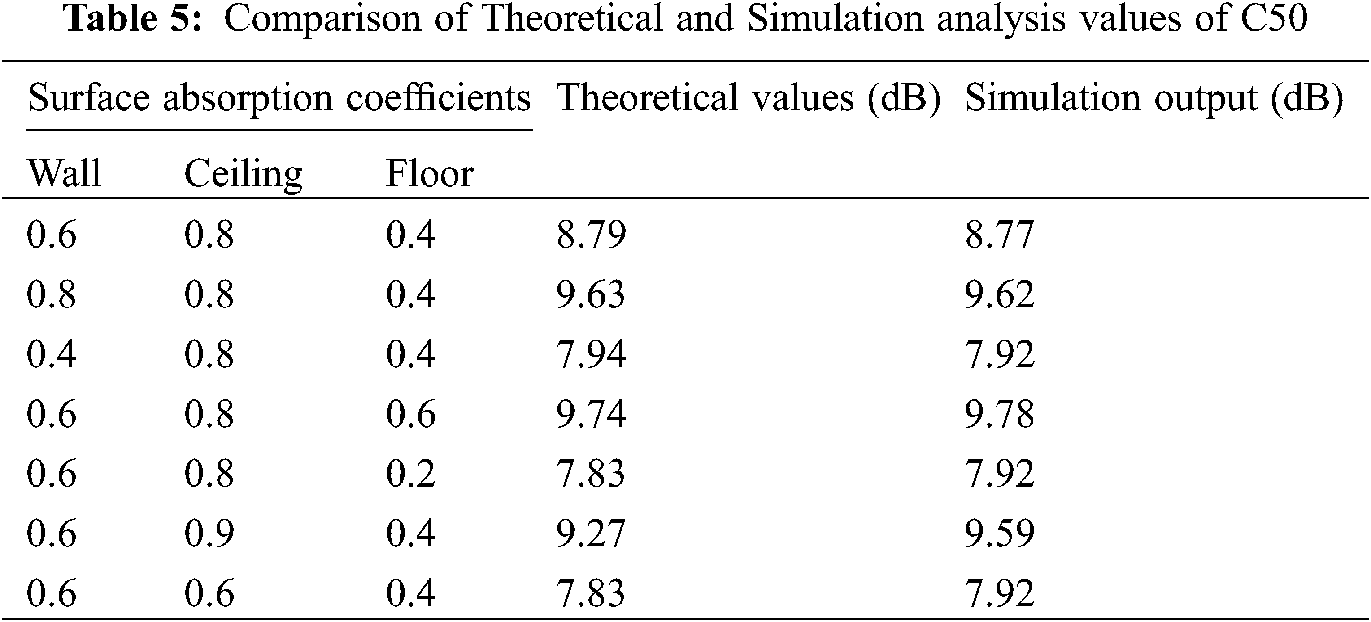
It is clear from Table 5 that the values of C50 in the simulation and the theoretical calculations carried out for the same simulation settings are very close to each other in value. This implies that I-SIMPA simulations produce results corresponding to the actual theoretical values obtained using standard mathematical equations.

4  Simulation Analysis

The simulations analysis C50 were carried for the following two major circumstances:

1.    Single Source

2.    Multiple Sources

By primarily varying the distribution and number of sources in the chamber, the conditions required to maintain a constant value of C50 in the majority of the chamber were obtained by altering the wall absorption coefficients and chamber dimensions.

4.1 Single Source

4.1.1 Simulation

The analysis of Clarity (C50) by means of single source placement and distribution in the chamber was done by considering the following four cases:

1.    Source at Receiver position-The source is placed at the position of the receiver, that is at (4.65, 6.5, 1.2).

2.    Source at Teacher position-The general position of a teacher is in the front of a classroom, towards the centre. Keeping this in mind, the source is placed at (4.65, 7.2, 1.2).

3.    Source placed at the centre of the chamber-The source is placed at the centre of the chamber, at (4.65, 3.75, 1.2).

4.    Source at corner-The source is placed at one corner at (8.8, 7.2, 1.2).

4.1.2 Analysis and Discussion

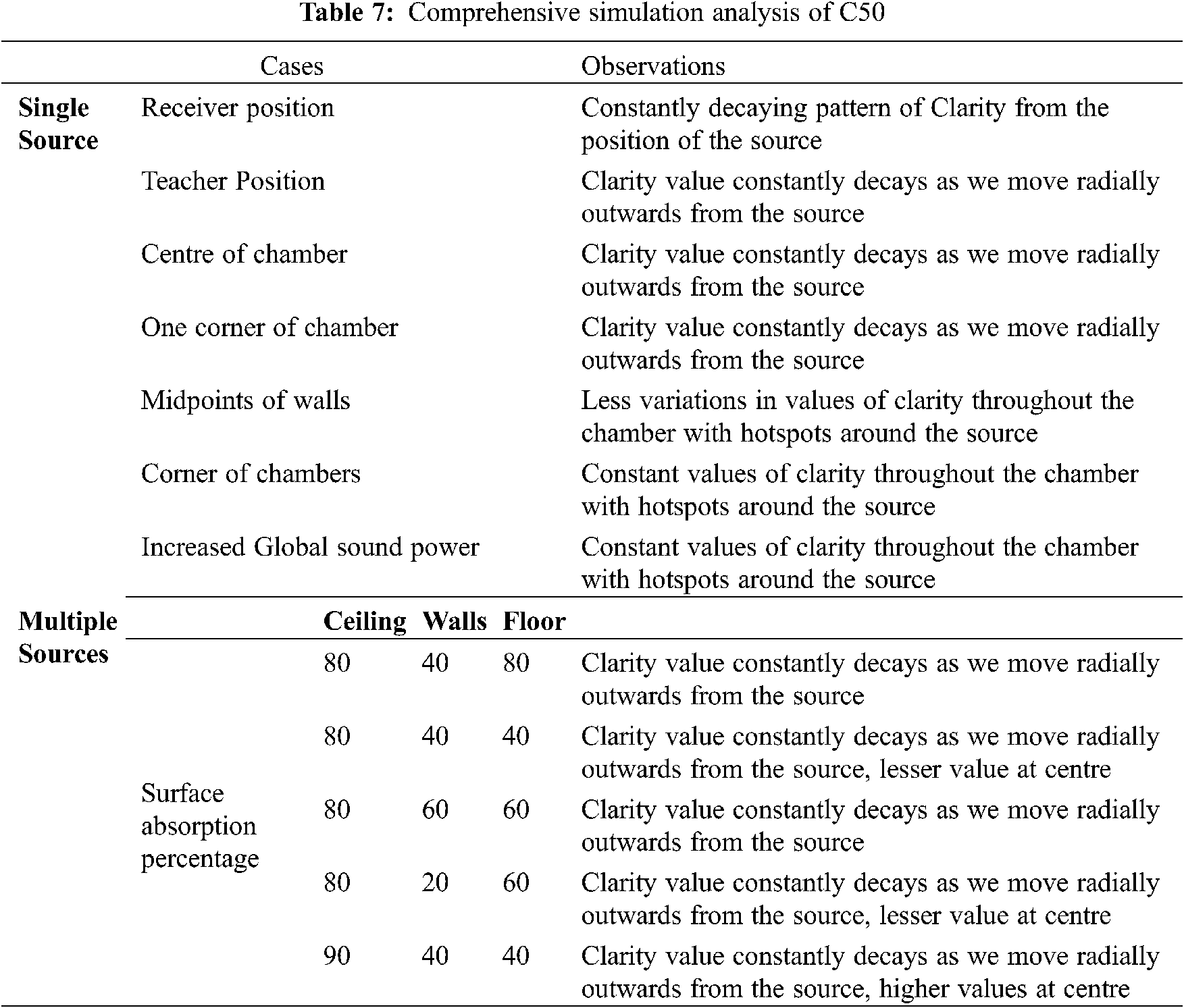
Case (1) simulation output in Fig. 6a resulted in a constantly decaying pattern of Clarity when moving radially outwards from the position of the source. Here positions of the source and receiver are the same. This depicts the well-known fact that the clarity of auditory perception decreases as we move away from the source. Case (2) Fig. 6b, the pattern obtained from simulation is the same as the one obtained in Fig. 6a, with the Clarity value constantly decaying as we move radially outwards from the source. Case (3) output is shown in Fig. 6c which also indicates the same pattern of decaying clarity value for radially outward movement. A similar pattern was obtained for Case (4) in Fig. 6d. The same pattern of clarity is observed when the source is kept at any of the corners in the chamber.

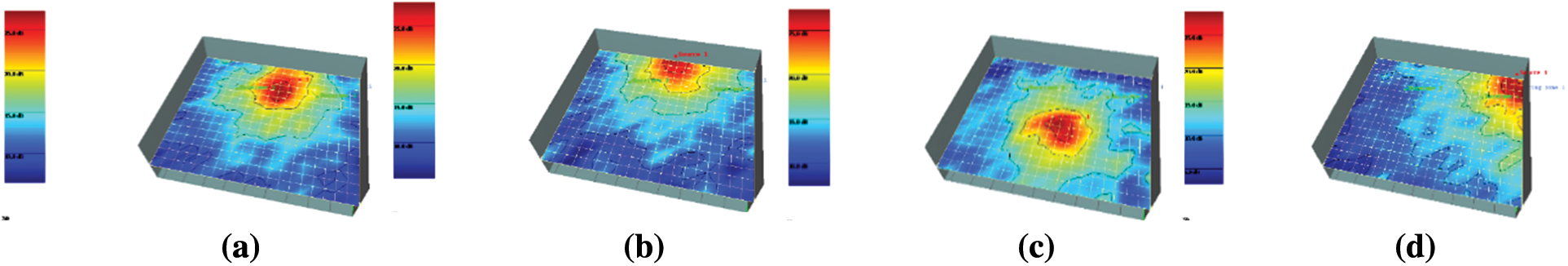
Figure 6: C50 for Single Source. (a): Auditory perception decreases, (b): Move radially outwards from the source, (c): Decaying clarity value, (d): Source is kept at the corners in the chamber

4.1.3 Inferences

From the above simulation outputs for a single sound source system, it can be inferred that

•   Clarity (C50) follows a constantly decaying pattern as the observer/listener moves radially outward from the position of the source.

•   Position of the source in the chamber does not affect the pattern of C50.

•   The condition for acoustically ideal system using Clarity (C50) for increased auditory comfort of the observer needs to be effected by the use of multiple sources in the same, arranged in pre-specified manner, symmetrically.

•   For instance, the simulation pattern for the Clarity parameter is observed to be inconsistent when the properties of the source, such as the Global Sound Power is increased separately. From Fig. 6c, it can be seen that when the Global Sound Power is altered to 120 dB for every source simultaneously, the clarity pattern remains consistent, but when only of the source’s Global Sound Power is increased from 80 dB to 120 dB, the inconsistent pattern as in Fig. 7 is observed. This strengthens the implication of the C50 parameter’s dependency on multiple properties of the source and environment that need to be further studied at parallel with the effect of other acoustic parameters’ effect on C50.

•   It can thus be comprehended that the pattern of C50 depends on the source properties such as its position as seen in Fig. 6a and its Global Sound Power.

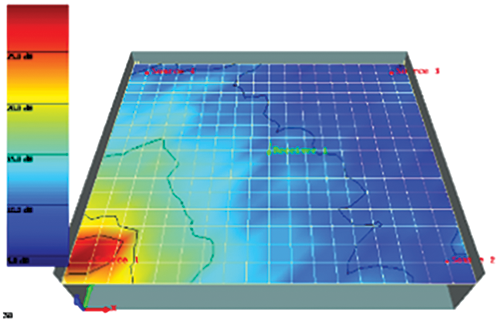
Figure 7: C50 when Source 1 at (0.5, 0.5, 1.5) Global Sound Power is increased from 80 dB to 120 dB

4.2 Multiple Sources

The pattern of steady decay of clarity can be observed when sources are placed unsymmetrically in the chamber designed. Due to imbalance in various acoustic parameters owing odd number of sources and unsymmetrical arrangement in the area considered, a constant value of clarity throughout the entire chamber cannot be obtained.

Therefore, the minimum number of sources required for the four-walled chamber designed [2] to maintain constant conditions is 4, one for each face. By balancing the source distribution with respect to the size and shape of the chamber, constancy in parameters can be obtained.

4.2.1 Simulation

The analysis of Clarity C50 by means of multiple (4) source placement and distribution in the chamber was done by considering the following cases:

1. Sources at the midpoints of the wall sides-Four sources were placed at (4.5, 0.5,1.2), (0.5,3.75,1.2), (4.5,7.2,1.2) and (8.5,3.75,1.2) (in metres).

2. Sources at the corners-Four sources were placed at (0.5, 0.5,1.2), (8.8,0.5,1.2), (8.8,7.2,1.2) and (0.5,7.2,1.2) (in metres).

3. Sources at corner with increased global sound power-Four sources were placed at (0.5,0.5,1.2), (8.8,0.5,1.2), (8.8,7.2,1.2) and (0.5,7.2,1.2) (in metres). The Global sound power for every source was increased from 80 dB to 120 dB.

4. Changes in in Wall Absorption percentages-The variation in Clarity (C50) is studied using the same acoustic model by varying the surface absorption percentages of the walls, ceiling and floor.

4.2.2 Analysis and Discussion

The source positions corresponded to the midpoints of the wall faces in Case (1) as shown in Fig. 8a. The Clarity (C50) simulation output resulted in the appearance of nearly constant pattern of clarity throughout the chamber with variations of the order of 2 to 3 dB. The regions surrounding the sources acted as hotspots of clarity with values of Clarity above 20 dB in these regions. Case (2) is indicated by Fig. 8b which depicts the output when the source positions correspond to the corners of the designed chamber. The simulation of Clarity resulted in a nearly constant value environment with variations of the order of 0.1 dB. The regions immediately surrounding the sources have high values of clarity of the range of 25 dB. However, the major portion of the chamber experience a clarity value in the range 10–15 dB. A mere visual comparison of the above simulations comprising of multiple sources shows that the arrangement of sources in the corner of the chamber as the better source distribution model. Case (3) simulation output shown in Fig. 8c displays the same pattern of clarity as indicated in Fig. 8b. Thus it can be said that the simulation patterns do not vary due to the change in the global sound power values of the sources.

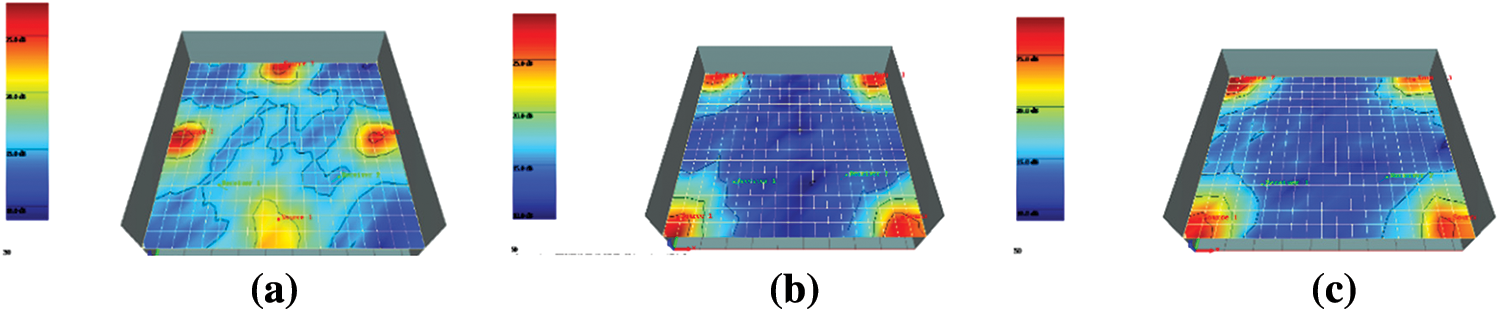
Figure 8: C50 for Multiple Sources Cases 1–3. (a): Source positions corresponded to the midpoints (b): Source positions correspond to the corners (c): Source positions correspond to the corners of the designed chamber

The above acoustic models were simulated with the absorption percentages of the ceiling, floor and walls at 80%, 40% and 60%, respectively. Absorption here is depicted in percentage, that is, a wall with 40% absorption will allow 60% of the sound waves to pass through or be reflected back into the system.

Table 6 shows the simulation output for Case 4 of Multiple Source Simulations. Case 4(a) simulation output is seen in Fig. 9. Here, C50 follows the pattern as seen in Figs. 8b and 8c with the value decreasing as the distance from the source increases. Previously the clarity at the centre (4.5, 3.75,1.5) was about 15 dB (Fig. 8b). Fig. 9 shows that C50 has increased to about 22 dB. This implies that this surface absorption percentage combination increases clarity for major part of the chamber. Cases 4(b), 4(c) and 4(d) shown in Figs. 10–12 respectively follow the same previous pattern as seen in Fig. 9. The clarity C50 value at the centre of the model is lesser than 10 dB which is significantly lesser than what was obtained in Case 4(a) Fig. 9. This implies that this combination of surface absorption percentages decreases the clarity value in the majority of the chamber is not ideal to be used. Case 4(e) Fig. 13 shows that the clarity C50 at the centre varies from 15 dB to 18 dB, following the same pattern as Case 4(a) Fig. 9. There are, however increased concentration of low clarity regions at the centre of the chamber. The variation is of the order of 1 dB. Commercially the ‘Sound Transmission Class’ (STC) gives an objective measure of the absorption percentages of the surfaces constituting the chamber [11]. Acoustic signals [12–16] and noises against various situations were determined.

4.2.3 Inferences

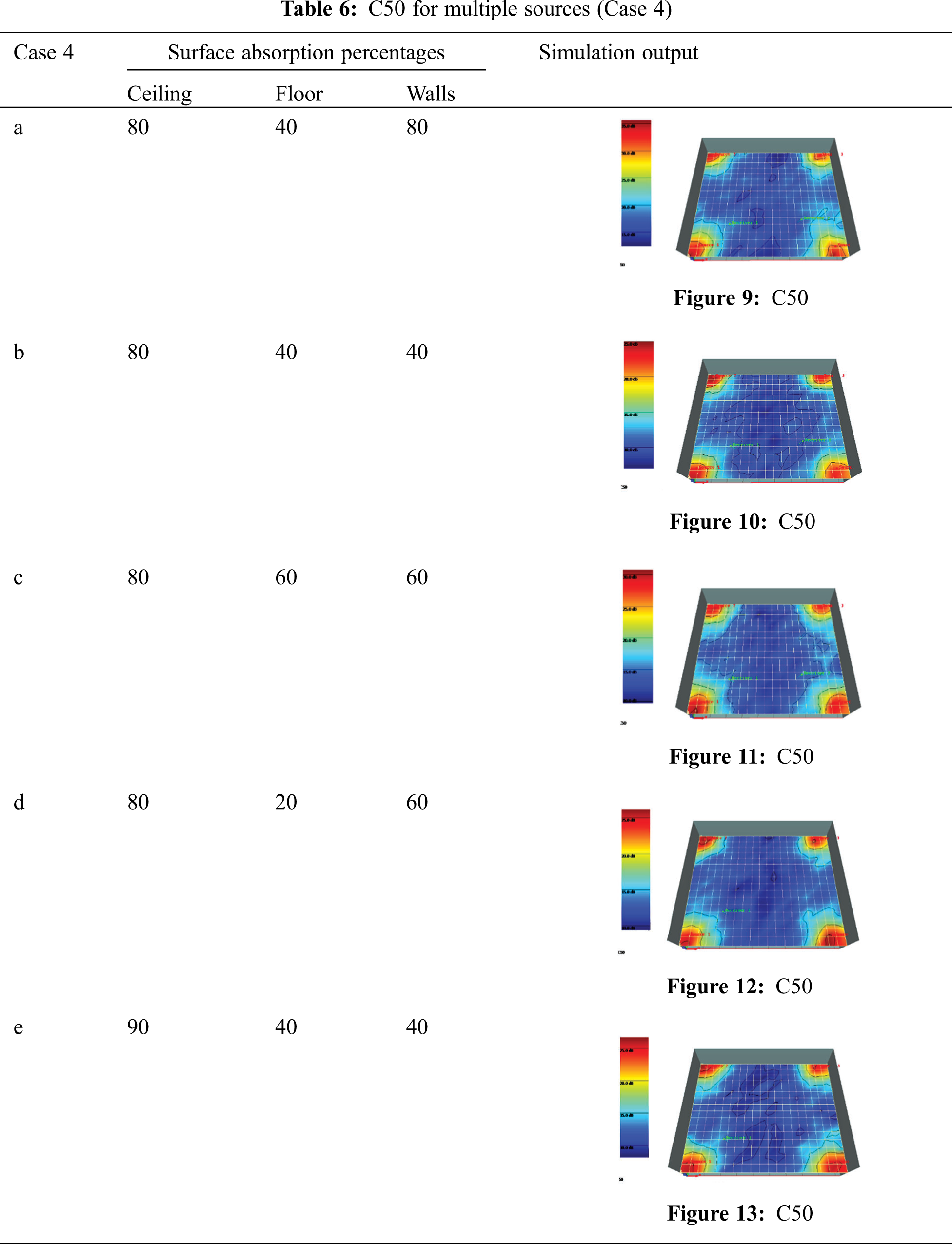
•   The symmetric arrangement of the sources in chamber gives rise to constant Clarity (C50) patterns in the chamber which can attribute to better auditory comfort.

•   The corner placement of the sources results in enhanced required patterns of C50 than when the sources were placed at the midpoint of the wall surfaces. This might be attributed to the confinement of the transmitted waves to an angle range of 0 to 90 degrees rather than 0 to 180 degrees which is the case during placement of sources at the midpoint of wall surfaces.

•   As the number of faces in the chamber structure change, the number of sources need to be increased to match this number for better Clarity (C50) pattern.

•   Table 7 gives an overall perception in the behaviour patterns of the C50 parameter for Single sound Source and Multiple sound Source systems.

5  Conclusion

The following conclusions can be drawn from the various simulations conducted by means of changing the absorption percentages of the different surfaces in the chamber:

•   Clarity (C50) pattern is the same for all parameter changes but the value differs with each change of parameter.

•   The Clarity (C50) value is dependent on one or more parameters.

•   The values of Clarity depend on the Surface absorption percentages and with that of Source Power.

The varying sound intensities at different points in a chamber can cause great discomfort to the audience present in the area of sound reception. This paper provides an insight into how the Clarity (C50) can be made constant in the greater part of the chamber by merely varying the surface absorption coefficients of the surfaces and by varying the number and arrangement of the sources in the designed chamber. A combined study of other important acoustic parameters along with C50 can provide a deeper understanding into how the condition of acoustic ideality can be effected in any closed chamber. It has been seen that these ideal conditions can be maintained by varying the values of absorption percentages of the surfaces involved. Commercially, ‘Sound Transmission Class’ (STC) gives an objective measure of the absorption percentages of the surfaces constituting the chamber. The STC rating is a measure of the reduction in decibel noise that a material can provide.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Bistafa, S. R., Bradley, J. S. (2000). Predicting reverberation times in a simulated classroom. The Journal of the Acoustical Society of America, 108, 1721. DOI 10.1121/1.1310191. [Google Scholar] [CrossRef]

 2.  Sato, H., Bradley, J. S. (2008). Evaluation of acoustical conditions for speech communication in working elementary school classrooms. The Journal of the Acoustical Society of America, 123(4), 2064–2077. DOI 10.1121/1.2839283. [Google Scholar] [CrossRef]

 3.  PicautJ.(2019). Ifsttar Revision 4d581f76 “Using SPPS with I-Simpa”, I-Simpa Documentation. [Google Scholar]

 4.  PicautJ.(2019). Ifsttar Revision 4d581f76 ‘Energetic modelling with I-Simpa’, I-Simpa Documentation. [Google Scholar]

 5.  Jackett R., National Physics Laboratory (2018). http://resource.npl.co.uk/acoustics/techguides/absorption/. [Google Scholar]

 6.  Acoustic Bulletin (2018). Room acoustic descriptors–RT, C50 and Strength/Gain. https://www.acousticbulletin.com. [Google Scholar]

 7.  Ahnert, W. S. M. (2019). Fundamentals to perform acoustical measurements. Appendix to EASERA. https://www.semanticscholar.org/paper/Fundamentals-to-perform-acoustical-measurements-Ahnert/3e00dfaa0b8e3eda94da4469d9b5c71e5b0b8cd6. [Google Scholar]

 8.  Joyce (1974). Sabine’s formula. The Journal of the Acoustical Society of America, 55, S12. [Google Scholar]

 9.  Honeycutt, R. (2011). Reverberation Time: RT10, RT15, RT20, RT30, RT60. https://www.prosoundtraining.com/2011/12/22/reverberation-time/. [Google Scholar]

10. Valente, D. L., Plevinsky, H. M., Franco, J. M., Elizabeth, C., Heinrichs–Graham et al. (2012). Experimental investigation of the effects of the acoustical conditions in a simulated classroom on speech recognition and learning in children. The Journal of the Acoustical Society of America, 131, 232. DOI 10.1121/1.3662059. [Google Scholar]

11. Clark, D. M. (1970). Subjective study of the sound-transmission class system for rating building partitions. The Journal of the Acoustical Society of America, 47(3A), 676–682. DOI 10.1121/1.1911950. [Google Scholar] [CrossRef]

12. Kalpana, G., Rajendran, V., Murugan, S. S. (2014). Study of de-noising techniques for SNR improvement for underwater acoustic communication. Journal of Marine Engineering & Technology, 13(3), 29–35. DOI 10.1080/20464177.2014.11658119. [Google Scholar] [CrossRef]

13. Murugan, S. S., Natarajan, V., Kumar, R. R. (2012). Estimation of noise model and denoising of wind driven ambient noise in shallow water using the LMS algorithm. Acoustics Australia, 40(2), 111. [Google Scholar]

14. Raj, K. M., Murugan, S. S., Natarajan, V., Radha, S. (2011). Denoising algorithm using wavelet for underwater signal affected by wind driven ambient noise. International Conference on Recent Trends in Information Technology, pp. 943–946. Chennai:IEEE. [Google Scholar]

15. Veni, S. K., Murugan, S. S., Natarajan, V. (2011). Modified LMS adaptive algorithm for detection of underwater acoustic signals against ambient noise in shallow water of Indian Sea. International Conference on Recent Trends in Information Technology, pp. 901–905. Chennai: IEEE. [Google Scholar]

16. Veni, S. K., Murugan, S. S., Radha, S. (2011). Adaptive algorithm for detection of underwater acoustic signals against ambient noise in shallow water at Indian seas. International Conference on Emerging Trends in Electrical and Computer Technology, pp. 758–761. St. Xaviers Catholic College of Engineering, Nagerkoil: IEEE. [Google Scholar]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.