Structural Durability & Health Monitoring

---

DOI: 10.32604/sdhm.2022.018293

ARTICLE

Seismic Analysis of Reinforced Concrete Silos under Far-Field and Near-Fault Earthquakes

Anwer H. Hussein* and Hussam K. Risan

Department of Civil Engineering, Al-Nahrain University, Baghdad, 10072, Iraq
*Corresponding Author: Anwer H. Hussein. Email: anwer.h.hussein@gmail.com
Received: 14 July 2021; Accepted: 29 December 2021

Nomenclature

γ	The stored material unit weight
R	Hydraulic radius
μ	Wall friction coefficient
k	Coefficient of at-rest lateral pressure
v	Poisson’s ratio of the stored material
y	Height of the stored material measured from the top
A	Cross-section area of the silo

1  Introduction

Silos are upright bins that are used to store various types of materials such as wheat, cement, sand, and coal. They are commonly built with circular cross-sections with either reinforced concrete or steel walls. International codes and guidelines generally adopt the term squat to describe silos with shallow depths and slender silos for containers with high depth to diameter ratios. However, the complex behavior of the ensiled material produces unconventional static and dynamic loads. Accordingly, the silo walls are subjected to excessive stresses as a result of asymmetrical pressure distributions in the silo during earthquake loading [1]. The unique combination of loads has raised the rate of silo failures over any other industrial structure [2]. Thus, identifying the type and amount of loads acting on any structure is a crucial step in the assessment process. Additionally, successful numerical simulation of a silo is achieved by choosing suitable parameters to represent the silo wall, the ensiled granular material, and the contact element between the silo wall and the stored material.

The well-known classical slice theory of Janssen 1895 is widely adopted by the design standards to determine the stored material’s lateral pressure on silo walls [3]. Over the years many studies have confirmed its reliability to describe granular material filling pressure [4]. However, when the silo undergoes seismic excitation the wall stresses will be dramatically changed and additional non-uniformly distributed pressures will be produced in the circumferential and vertical directions. These additional pressures are not accounted for in the seismic codes, and the seismic effect on the silo system is usually transformed to lateral static force on a cantilever beam with lumped effective masses of 80% of the total mass of the silo and its content [5,6].

However, many experimental, mathematical, and numerical studies have discussed the reliability and the cost efficiency of using the lateral static force method adopted by the silo-related international specifications. Additionally, the distinction in the seismic behavior of the squat silos and the slender silos has been evaluated by comparing the produced stresses utilizing the static lateral force method. Consequently, Durmuş et al. [7] deeply discussed the critical slenderness ratio that acts as a changing value in the seismic stresses of silos. The proposed finite element model assumed a simple elastic representation of the stored material. Moreover, Butenweg et al. [8] discussed the advantages and the disadvantages of two approaches that were used to define the ground motion over a steel cylindrical silo. The first method utilizes a finite element simulation with time history analysis (time-domain) while the second procedure was done using the frequency domain with a lumped-mass method.

Saleem et al. [9] assessed by finite element approach the effectiveness of the seismic codes in designing reinforced concrete silos. An oversimplification was noticed in this study by not considering the wall-material interaction and the assumption of an empty silo condition during earthquakes. A comparison between a numerical model of a full-interaction slender steel silo and the Eurocode-8 seismic design provisions is presented [1]. The nonlinear analysis discussed the effect of wall flexibility and sub-soil contact; meanwhile, the earthquake loading was introduced to the finite element model by the acceleration time history. Moreover, a mathematical expression was implemented to predict the lateral and hoop pressures of a flat-bottomed silo exposed to sustained horizontal and vertical accelerations [10]. The seismic behavior of elevated type reinforced concrete silos was evaluated in the initial conditions [11,12] and while silo discharging [13]. Nevertheless, the silo wall rigidity is inspected for silos subjected to ground motions [14] and the response of the ensiled material under earthquakes [15]. Furthermore, a study was implemented to discuss the causes of the major failures in singular silos [16] and a group of silos under seismic excitation [17].

This study focuses on the evaluation of the nonlinear seismic behavior of flat-bottomed reinforced concrete cylindrical silos under near-fault ground motions (NFGM) and far-field ground motions (FFGM). Though there is no fixed distance for the designation of the near-fault earthquakes; a distance range of (15–50) km is widely adopted by researchers and international codes as a reasonable value [18]. Zhou et al. [19] studied the impact of NFGM characteristics on the structural demand of structures through attention relationships for 34 near-fault records. A single degree of freedom (SDOF) system was adopted and the effect of earthquake stochastic pulse was investigated. However, ground motions that happen within a short distance from the earthquake source often have a distinguishable characteristic when compared to those that occur at a relatively far distance. Mainly, large pulses with relatively high amplitude appear at the beginning of the earthquake records. Normally, these pulses can be recognized within the acceleration, velocity, and displacement time histories. Though, some records of the acceleration time histories may not be quite sharp catching up with such pulses [20–22], Hence, distance and Magnitude are not the only contributors to the severity of an earthquake; the direction of the fault rupture propagation concerning site location plays a critical role in deciding the earthquake intensity. This phenomenon is commonly referred to as the directivity effect of the near-fault ground motions. As a result, three types of direction effects are recognized; the forward directivity effect and the backward directivity effect [23].

Nevertheless, researchers observed numerous other factors that characterize the NFGM and contribute to the overall seismic demand of structures, e.g., the effect of the fling step. The fling step is a permanent deformation that happened as a consequence of the movement of tectonic plates. This type of deformation takes relatively little time to be formed and comes up as a pulse which is mostly recognized at the end duration of the NFGMs displacement time histories. Typically appears as a monotonic and unidirectional pulse [24–27].

2  The Finite Element Model

The international codes and standards deal diversely with the classification of silos. For instance, the Eurocode-1 [28] introduces two approaches to differentiate silo types. The first method utilizes the silo storage capacity and the symmetric discharge impact, while the second guideline employs the height to diameter ratio. However, as far as the dynamic seismic analysis is concerned, the slenderness ratio performs a vital role in the determination of the silo seismic demand. The previously covered literature survey has confirmed that claim. Consequently, the proposed system consists of four silo models with slenderness ratios of 1, 2, 3, and 4 as shown in Fig. 1. The silo base was assumed to be fixed and each model is composed of three major components; the silo wall, the ensiled bulk-type material, and the interaction between the silo wall and the stored material.

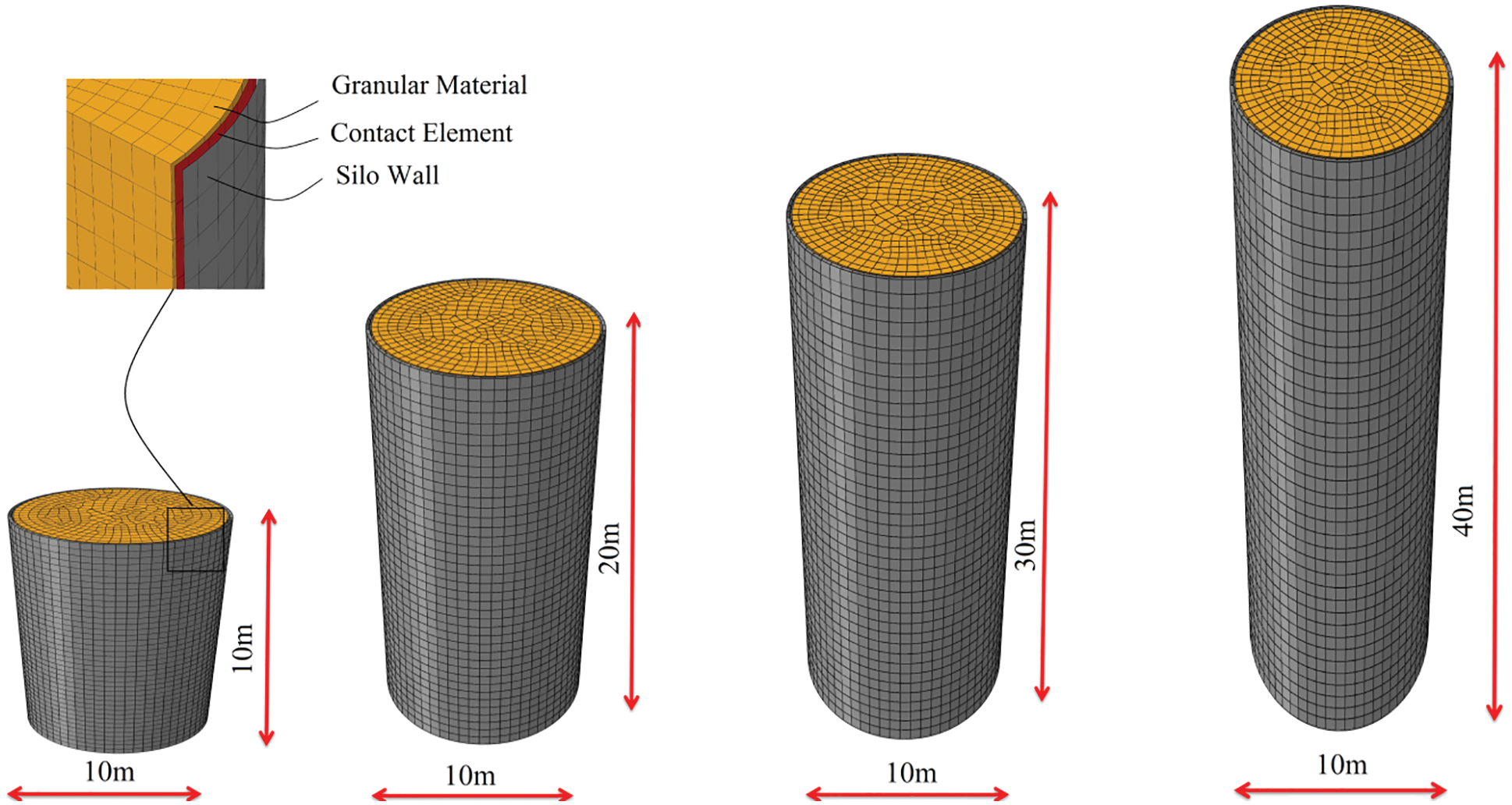
Figure 1: Silo adopted in the analysis shows different slenderness ratios

2.1 Materials Definition

A successful finite element simulation depends entirely on the proper definition of the used materials and the selection of element type. The below section characterizes the linear and nonlinear behavior of reinforced concrete wall and the ensiled granular material in addition to the criterion adopted to describe the interaction between the wall and the solids.

2.1.1 Silo Wall

4-noded doubly curved shell elements with reduced integration points (S4R) were chosen to represent the silo wall. In which, the reinforced concrete damage plasticity (CDP) model was selected to describe the nonlinear behavior of reinforced concrete walls to evaluate the tension and compression damages in the plastic regions. The mass density of concrete was set to be 2300 kg/m3 while the Poisson’s ratio (ν) was specified as 0.18. However, to obtain realistic results the parameters used to define the CDP should be properly selected and validated. Table 1 shows the values of these parameters. Concrete normally has a dilatation angle between 31° and 42° while the value of concrete viscosity is usually obtained through experiments. A detailed discussion about the selection of CDP parameters is presented by Nguyen et al. [29]. The ratio fbo/fco shown in the table refers to the biaxial over the uniaxial compressive strengths. The eccentricity constant and K coefficient was taken as default values suggested by the ABAQUS built-in CDM model. Further components are required to describe the compression hardening behavior as in Fig. 2a and compression damage parameter presented in Fig. 2b in addition to the tension stiffening behavior as in Fig. 2c and tension damage parameter shown in Fig. 2d.

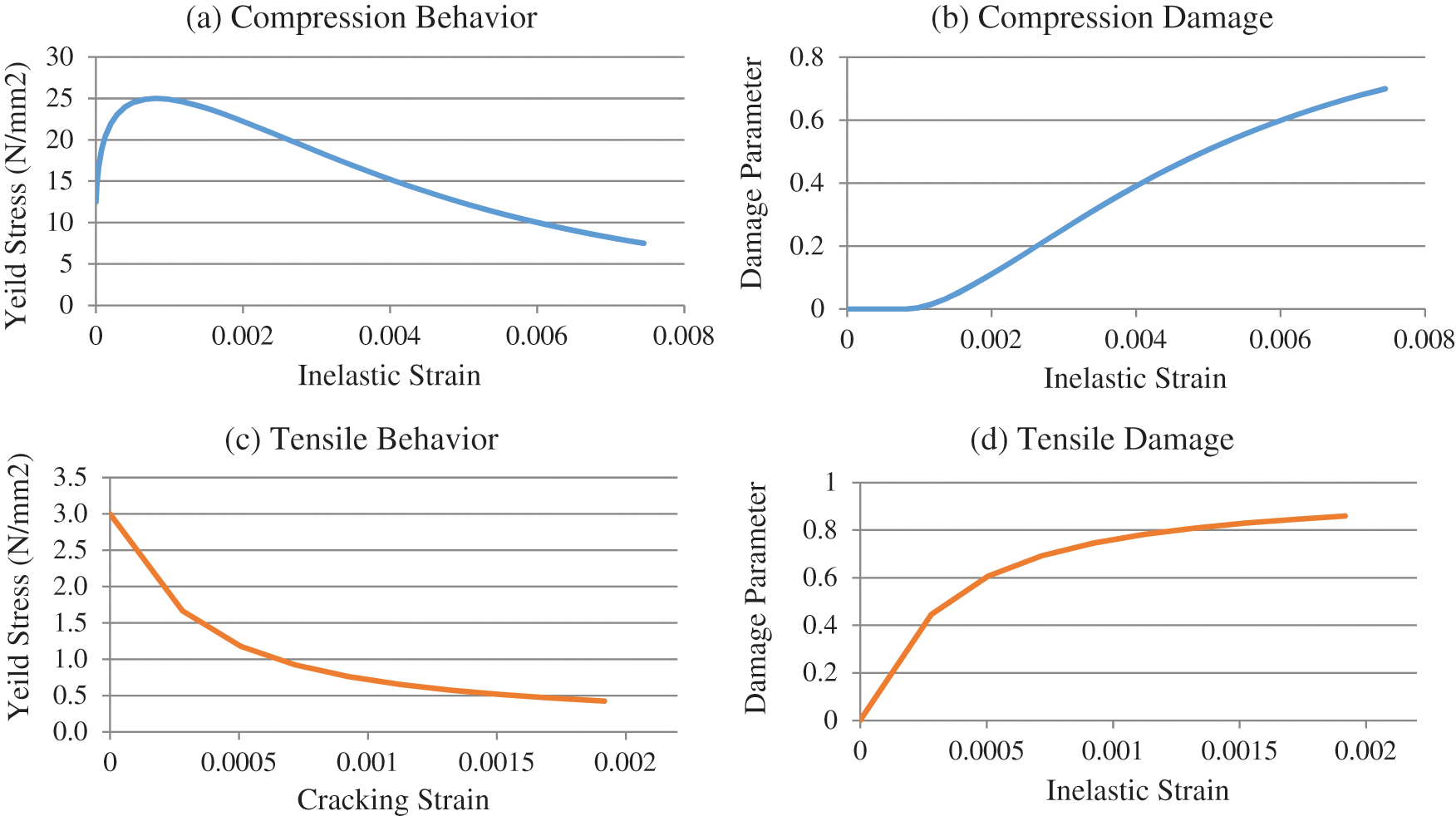
Figure 2: Concrete damage plasticity tension and compression parameters

The steel was assigned in full bond to the shell elements as double layers in the vertical and circumferential directions. The yield stress of the reinforcement bars was assumed to be 400 MPa and the ultimate stress is set equal to 600 MPa at 0.14 plastic strain. The Poisson’s ratio of steel was assumed to be 0.3 and 210 GPa Modulus of Elasticity. The dimensions of silo models and their steel reinforcement are illustrated in Table 2.

2.1.2 The Stored Material

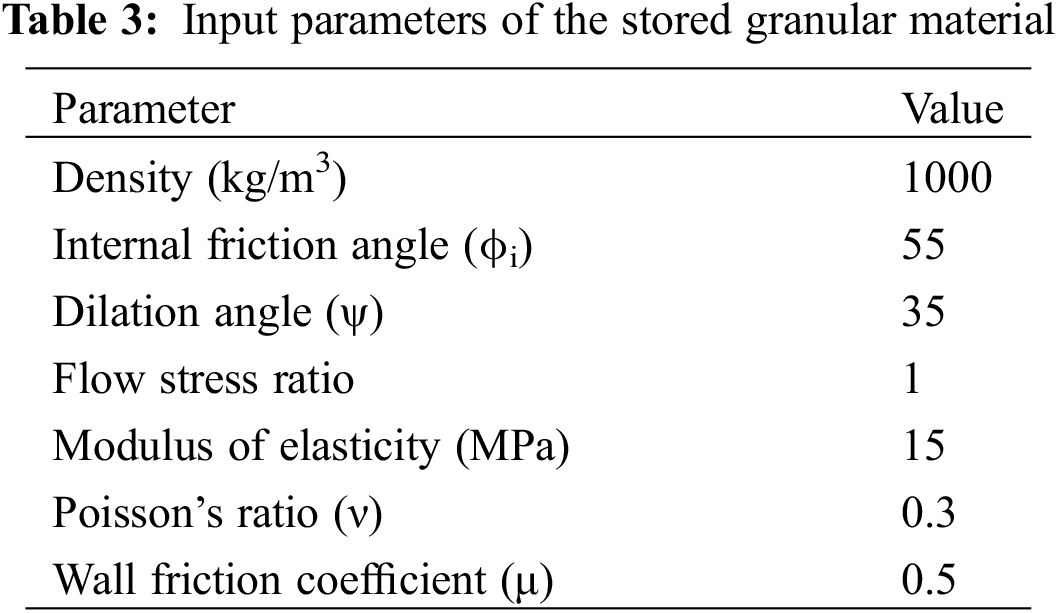
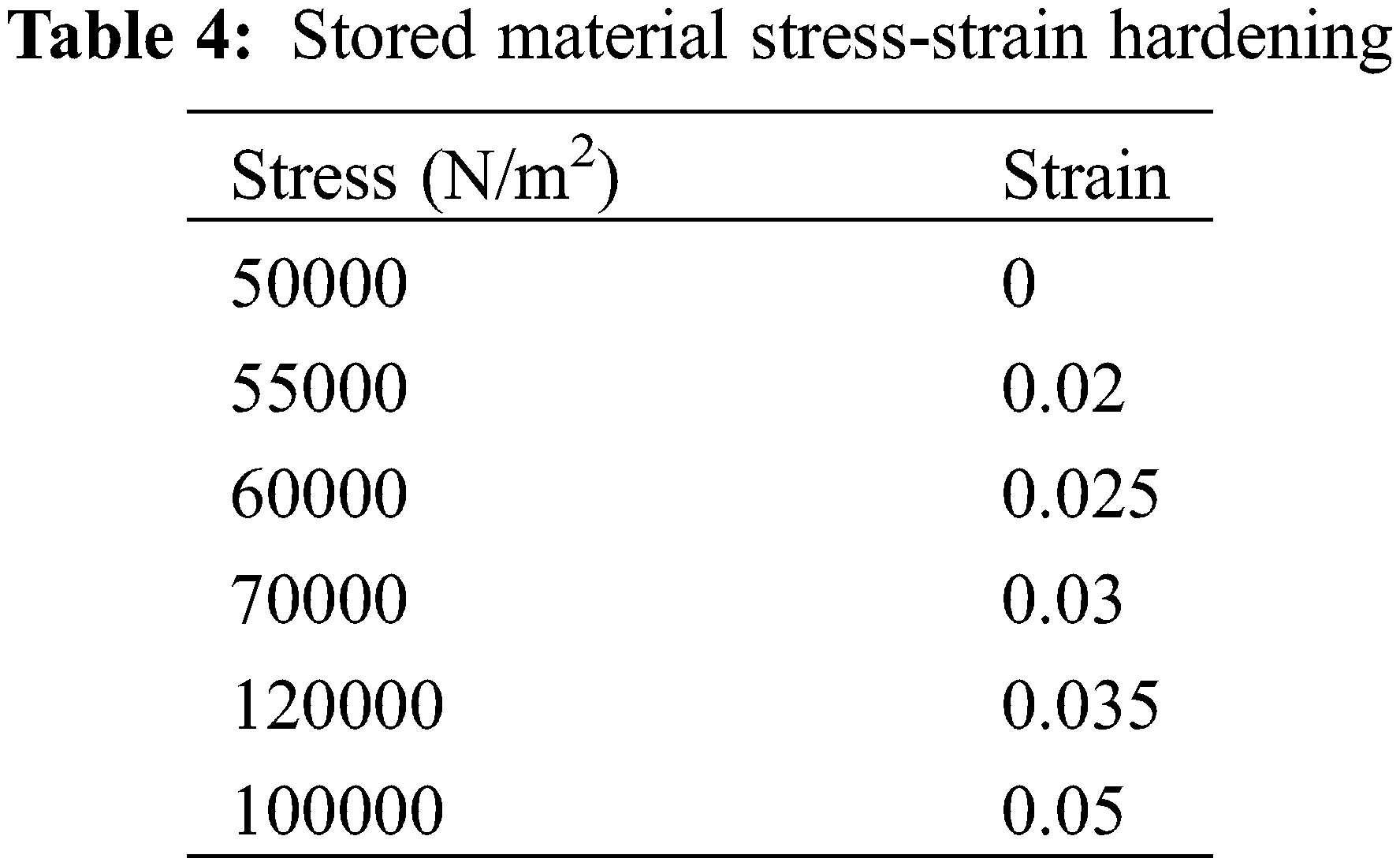
The stored granular material was constructed by 8-nodes linear brick elements with reduced integration points (C3D8R) to improve numerical accuracy. The behavior of the stored material was defined both elastically and plastically. The elastic part was defined by the elastic modulus (E) of 15 MPa and Poisson’s ratio (ν) of 0.3, whereas the plastic behavior was assumed to follow the Drucker-Prager constitutive plastic model. The Drucker-Prager is a perfectly plastic model that is characterized by three components; the angle of internal friction (ϕi), the dilation angle (ψ), and the flow stress ratio. The bulk-type material parameters are summarized in Table 3, in addition to the granular material hardening explained in Table 4. The Drucker-Prager parameters were adopted from previous experiments [30].

2.1.3 Wall-Granular Material Interaction

The interaction between the silo wall and the ensiled material was defined with The Coulomb friction theory. This theory is used to evaluate the friction force between the silo wall and the surface of the granular material. The friction force is the product of the force applied in the normal direction and a constant friction coefficient (μ). Two surfaces are assumed to be entirely bonded to each other. Accordingly, if the maximum friction force is achieved then the relative slipping begins. Based on the isotropic concept of the Coulomb friction criterion, the sliding between the two surfaces will occur when the resultant shear stress τR reaches the critical shear stress τcr. The resultant shear stress τR is computed from two transverse shear stress components τ1, and τ2 using Eq. (1). Meanwhile, the critical shear stress τcr is the result of normal stress ( σ ) and constant friction coefficient (μ) as demonstrated in Eq. (2).

τR= τ12+τ22 (1)

τcr= μσ (2)

However, a constant friction coefficient of 0.5 along the silo inner surface was assigned permitting material separation and loss of contact. ABAQUS finite element package provides a Master-Slave contact pair algorithm. In which, the silo wall was considered as a master surface since it has the larger rigidity whereas the granular material surface was set as a slave surface.

2.2 Validation of the Numerical Model

The verification process is a crucial step to maintain the accuracy and reliability of the FE computational model. Hence, the proposed three-dimensional (3D) finite element model is verified both experimentally and analytically. In the first place, the experimental work was accomplished by Wang et al. [31] employing the University of Edinburgh flat-bottomed cylindrical silo. The geometry of the validation silo was 4 m in height and 0.7 m in diameter with a 0.04 m single concentric outlet. Additionally, a total of 56 lateral pressure cells were distributed in seven levels vertically and eight layers circumferentially at 45 degrees. Fig. 3 shows the lateral pressure distribution of the validation silo.

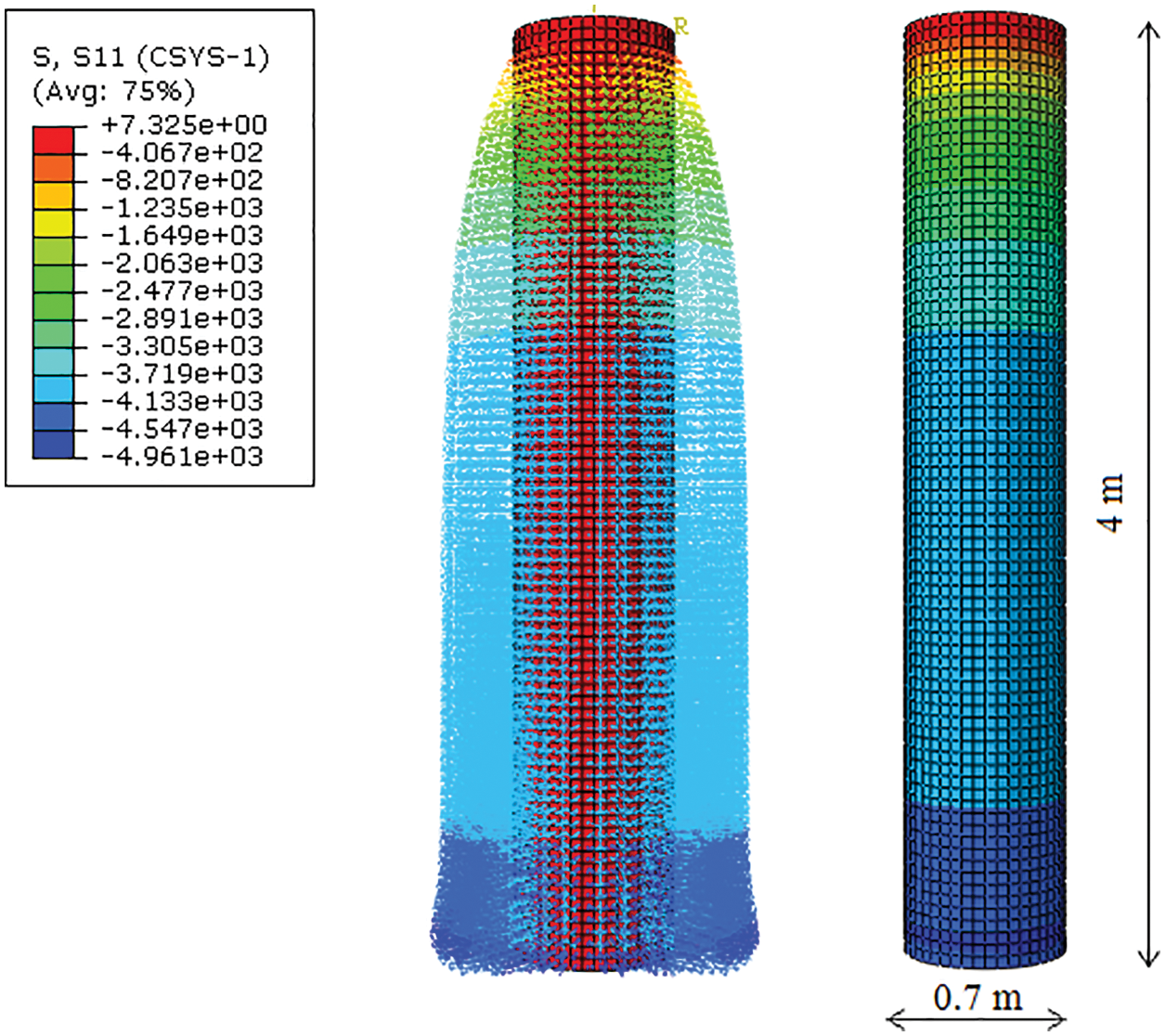
Figure 3: Lateral pressure produced by the numerical model

On the other hand, the analytical verification was satisfied through Janssen’s classical theory. This theory is the typical expression for the determination of the granular material pressures on silo walls and it is widely adopted by the international codes and standards. Janssen’s expression is produced by solving the differential equation excreted by the vertical static equilibrium of a horizontal section of silo height. This equation can be written as follows:

Lateral Pressure (P)= γ Rμ (1−e−μkyR) (3)

where:

R=Cross−sectionalAreaSiloperimeter (4)

k=ν1+ν (5)

The results of the normal pressure obtained from the numerical model were compared to the predictions of Janssen’s expression and the experimental data and illustrated in Fig. 4. As can be seen, the results of the finite element model produce slightly higher lateral pressure than the experimental data which is acceptable, since the finite element analysis is considered a lower bound theorem. This reduces the magnitude of the calculated deformation and as result yields higher stress values. Meanwhile, the pressure of Janssen theory and the finite model pressure are almost identical except for the extreme lower part. This difference can be attributed to Janssen’s assumptions that were assumed in the establishment of his equation. In which the friction between the stored material and the bottom of the silo is discounted which leads to the elimination of the bottom support reaction and producing uniform pressure at their intersection; in contrast to the actual case. So, the results are in a good match and the suggested finite elements model is accepted for the succeeding analysis.

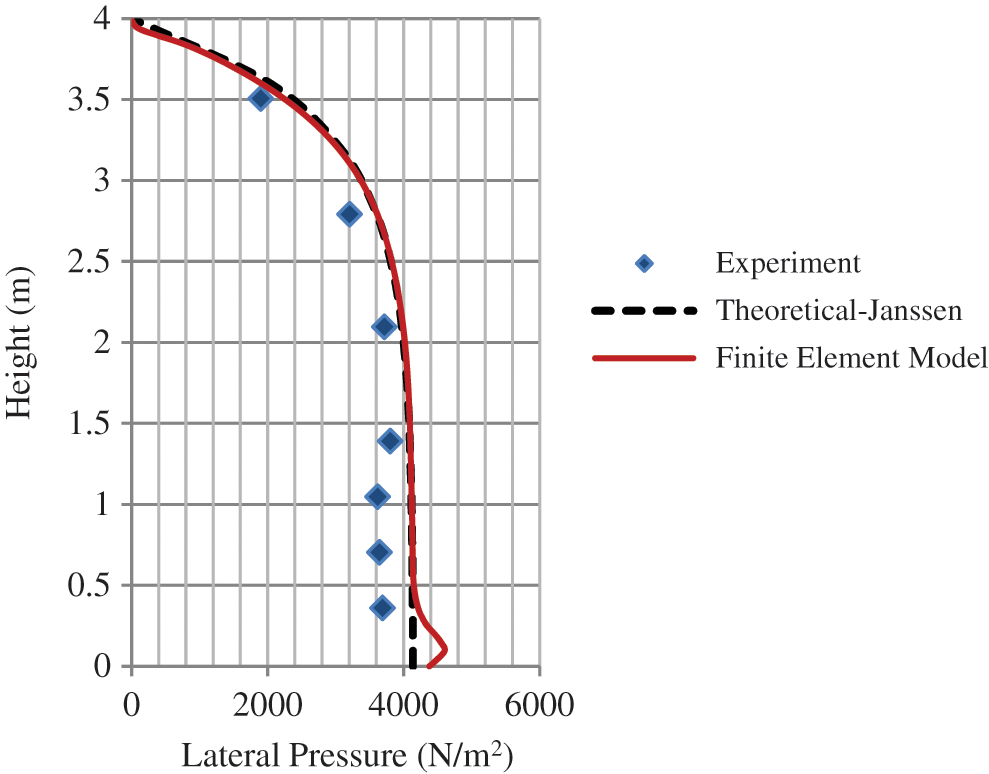
Figure 4: Validation of the Numerical model

3  Numerical Procedure

ABAQUS Finite element package with a nonlinear implicit solver was used to perform the numerical simulation. The numerical methodology was incorporated into three steps. Firstly, a free vibration eigenvalue analysis was done to extract the silo’s natural frequencies. These frequencies were integrated with a damping ratio of 5% to illustrate Rayleigh factors (α, β). These factors were used to define the material inelastic damping. Secondly, the stored material was filled into the silo by introducing the gravitational forces to the system and allowing the material-wall interaction to activate the pair contact friction algorithm. The filling process is a critical step to ensure the success of the next loading stages and a total of five seconds were allocated as a time for filling and solids stabilization.

Hence, the lateral pressure at the end of the filling phase for each model was checked with the Janssen filling pressure. Lastly, the seismic excitation was implemented into the silo base. Two different time histories of the Northridge 1994 earthquake with (6.69) moment magnitude were selected. The strong motions were adopted from the Center of Pacific Earthquake Engineering Research (PEER). This center is a project established by the University of California that provides a large-scale database of crustal strong motions. The first station of (Pacoima Dam-downstr) represents the near-fault ground motion since it is located at a 7.01 km distance from the rupture. While the second station of (Riverside Airport) acts as a conventional far-field ground motion and is situated at a 98.95 km distance from the earthquake source. Additionally, to make the comparison between these two records, their peak ground accelerations (PGA) were scaled to 0.5 g. The scaled acceleration time histories of the near-fault and far-field earthquakes are illustrated in Fig. 5.

Figure 5: Scaled acceleration time histories of Northridge earthquake; (a) Near-fault St. (b) Far-field

4  Analysis Results and Discussion

The near-fault and far-field outcomes will be presented and evaluated utilizing two main categories; the first category is the overall time-based response (or massive behavior) parameters such as the base shear and the overturning moment. Meanwhile, the second category shows the precise behavior parameters along with silo depth, for instance, the hoop stress and vertical stress of the silo wall. Moreover, the effect of compression and the tension damages will be assessed for each category.

4.1 Base Shear and Overturning Moment

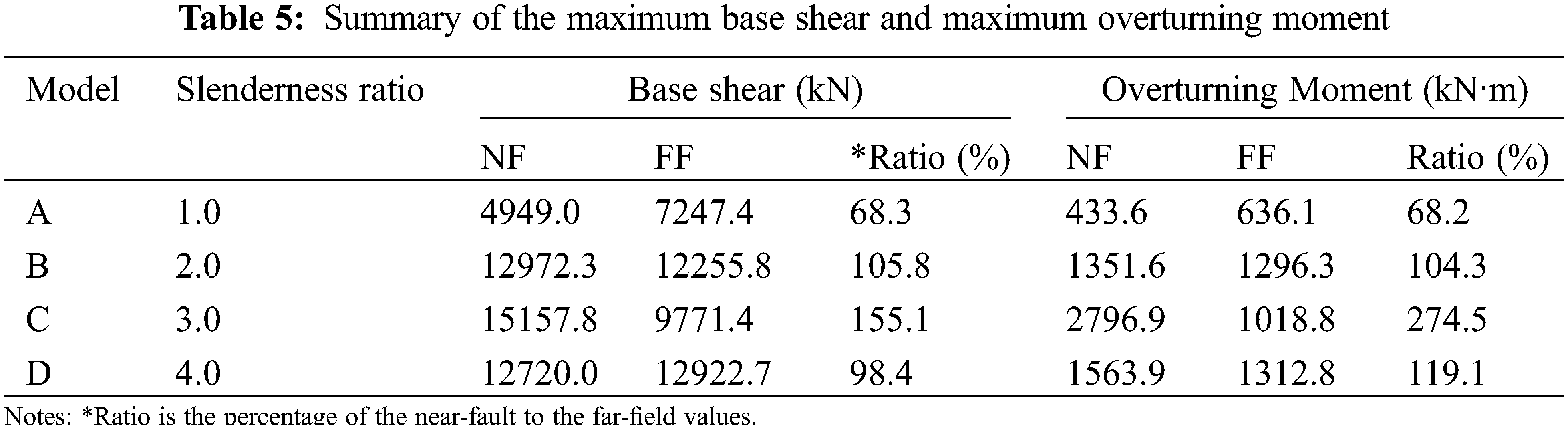
Silos’ response to the acceleration time histories of the near-fault and far-field ground motions of the Northridge 1994 earthquake was assessed in regards to base shear and base moment time histories; in addition to the concrete tension and compression damage propagations. For each factor, the influence of four different slenderness ratios was evaluated to estimate the structural safety and to specify the critical loading case. However, a total of eight runs were demonstrated and its corresponding base shear and base moment time profiles are presented in Figs. 6–9.

Figure 6: Model-A seismic response profiles: (a) Base shear, (b) Overturning moment

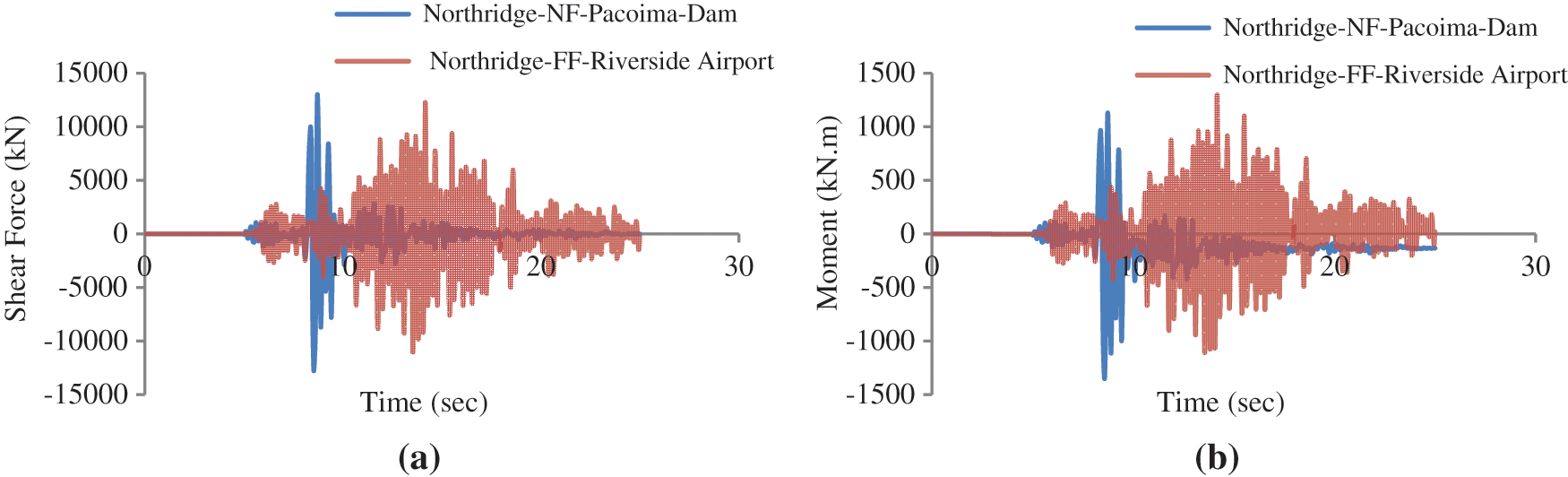
Figure 7: Model-B seismic response profiles: (a) Base shear, (b) Overturning moment

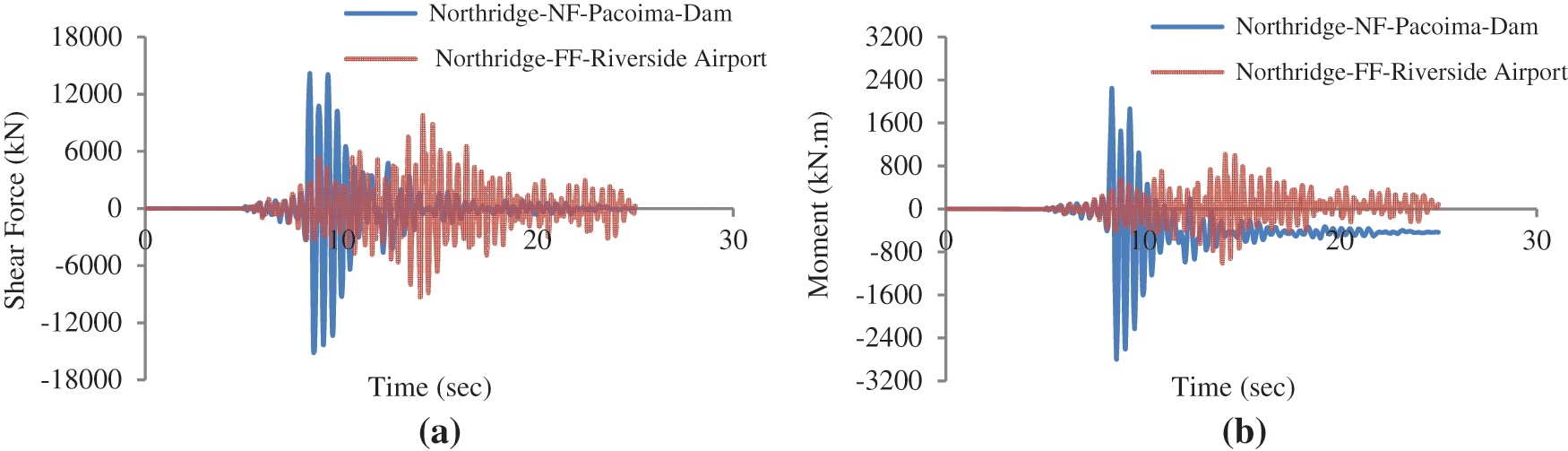
Figure 8: Model-C seismic response profiles: (a) Base shear, (b) Overturning moment

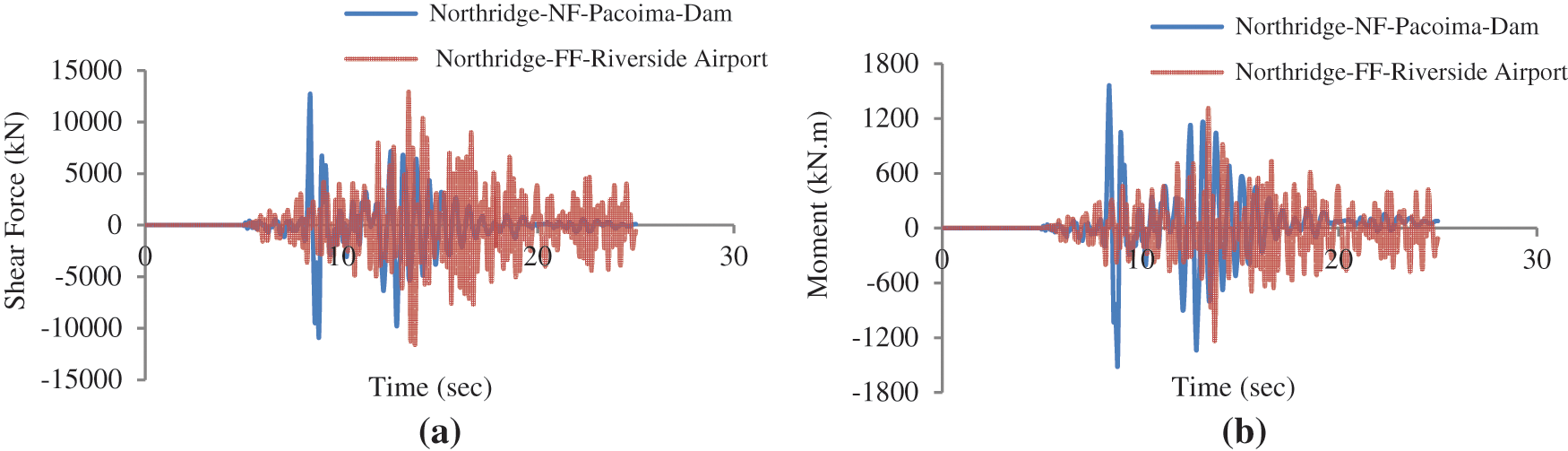
Figure 9: Model-D seismic response profiles: (a) Base shear, (b) Overturning moment

For Model-A of a squat silo with a height to diameter ratio = 1.0, the structural demand of the Riverside far-field ground motion station was higher than the Pacoima near-fault ground motion station. The percentage near-fault to far-field results of the base shear and overturning moment were 68.3% and 68.2%, respectively. While for Model-B of 2.0 slenderness ratio, the response of the near-fault station was slightly higher than the far-field station with no more than 5.8% increment in terms of maximum base shear and moment. However, a dramatic increase was recorded for Model-C with a slenderness ratio = 3.0, which has yielded the greatest demand for the NF station, with an NF/FF ratio of 155.1% maximum base shear and 274.5% in the max base moment. Finally, for model-D with an aspect ratio = 4.0 the max base shear in both NFGM and FFGM stations were almost identical, whereas the max overturning moment was 19.1% higher in the NFGM station. Table 5 summarizes the results of max base shear and base moments for all models.

On the other hand, the impact of seismic loading on the tension and compression behavior of the reinforced concrete walls has also fluctuated. At first, the squat-type silo has not experienced any concrete damage neither in the near-fault record (Pacoima Dam St.) nor in the far-field station (Riverside Airport St.). For Model-B, tension cracks occurred and were mostly concentered at the base part of the silo. In which the silo wall with NFGM record was damaged slightly more than the near-fault station. Unsurprisingly, Model-C endures aggressive concrete tension damage for the Pacoima near-fault station and it was spread along the lower half of the silo wall as shown in Fig. 10. Additionally, Fig. 11 reveals a presence of compressive crash in the lower part of Model C, whereas no other model has suffered from it. Finally, only the near-fault records caused concrete tension damage in the silo base region for Model D while the FFGM station showed no loss. Hence, for minimizing the graph’s area, only Model C tension and compression results were shown.

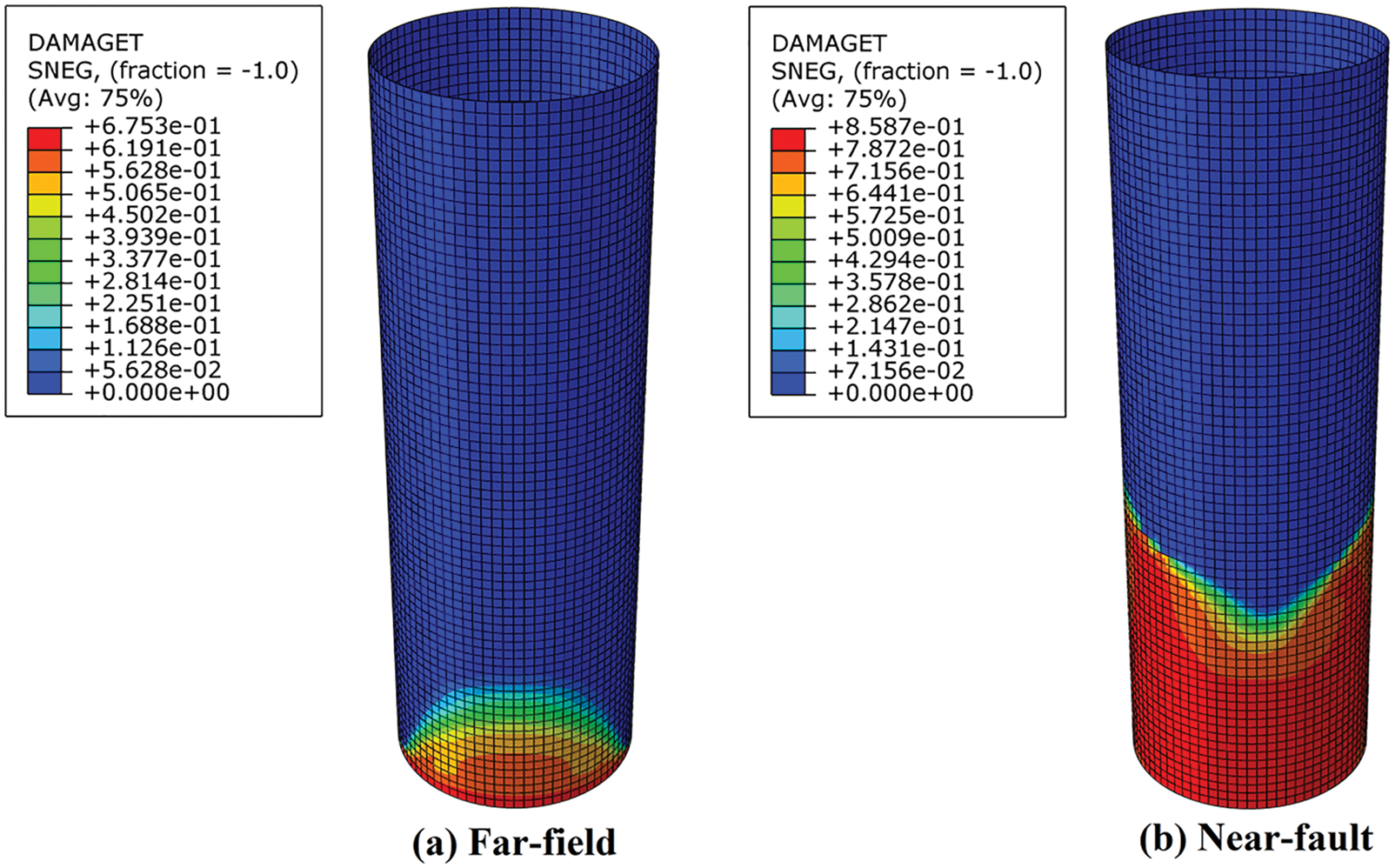
Figure 10: Model-C tension damage propagation

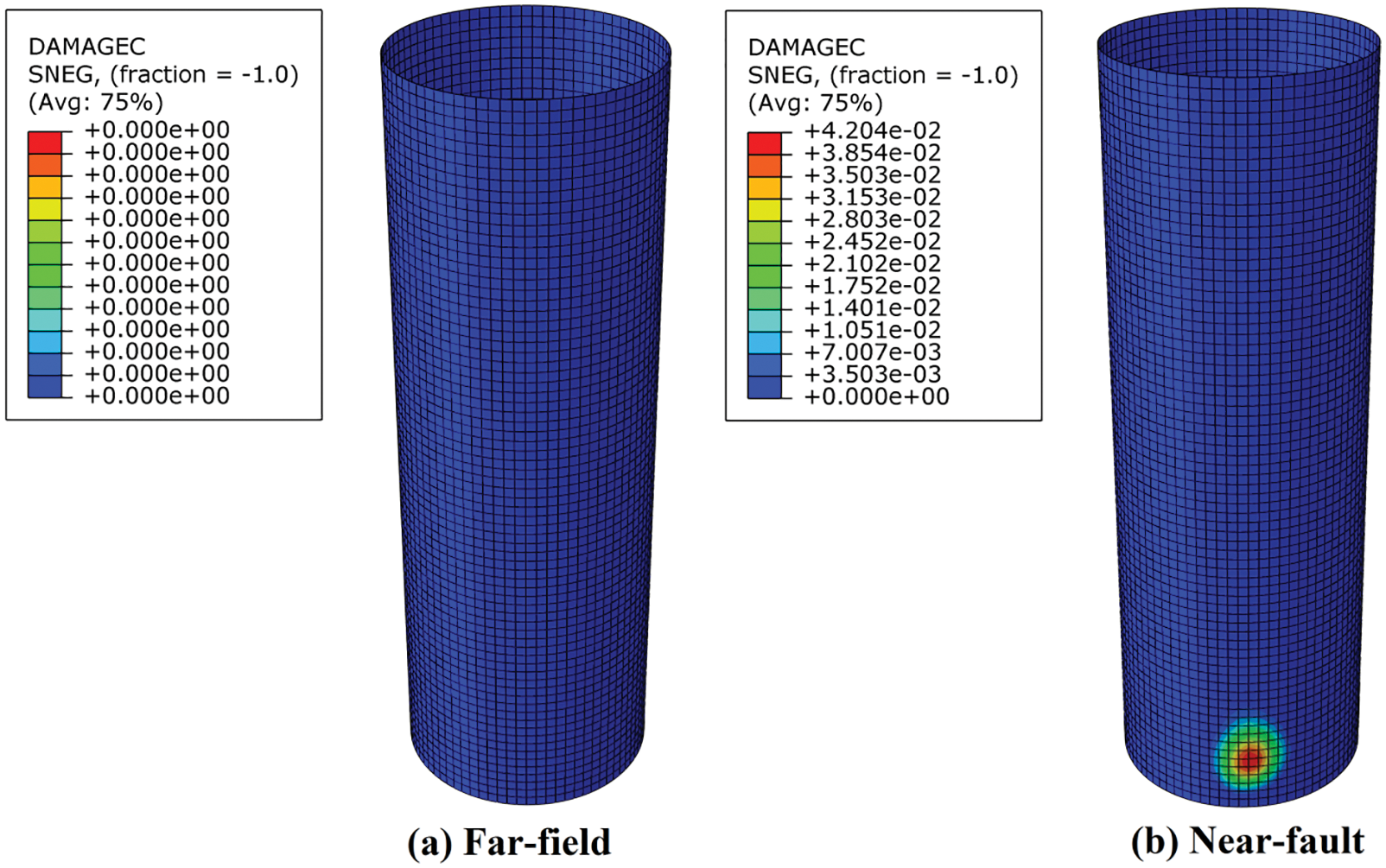
Figure 11: Model-C compression damage propagation

4.2 Silo Wall Stresses

The determination of the amount and distribution of silo stresses is essential to understanding the seismic behavior of silos. Additionally, the hoop stress ( fh ) and the vertical stress ( fv ) are the key parameters to design the grid steel reinforcement of the silo wall. Hence, these stresses will be evaluated for the same above-mentioned four slenderness ratios (Sr). Figs. 12–15 show the distribution of maximum hoop and vertical stresses for Model-A through Model-D, respectively.

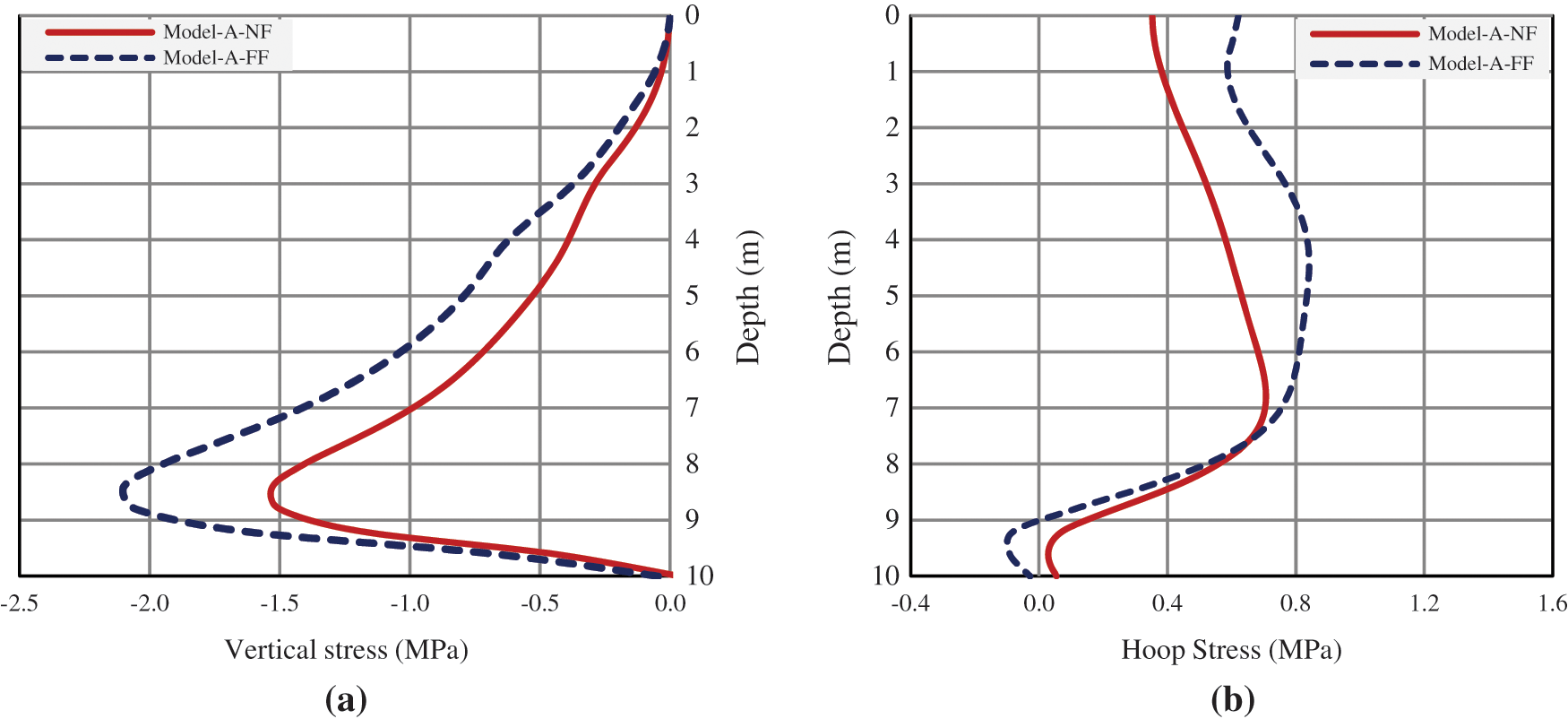
Figure 12: Model-A maximum wall stresses distribution; (a) Vertical stress, (b) Hoop stress

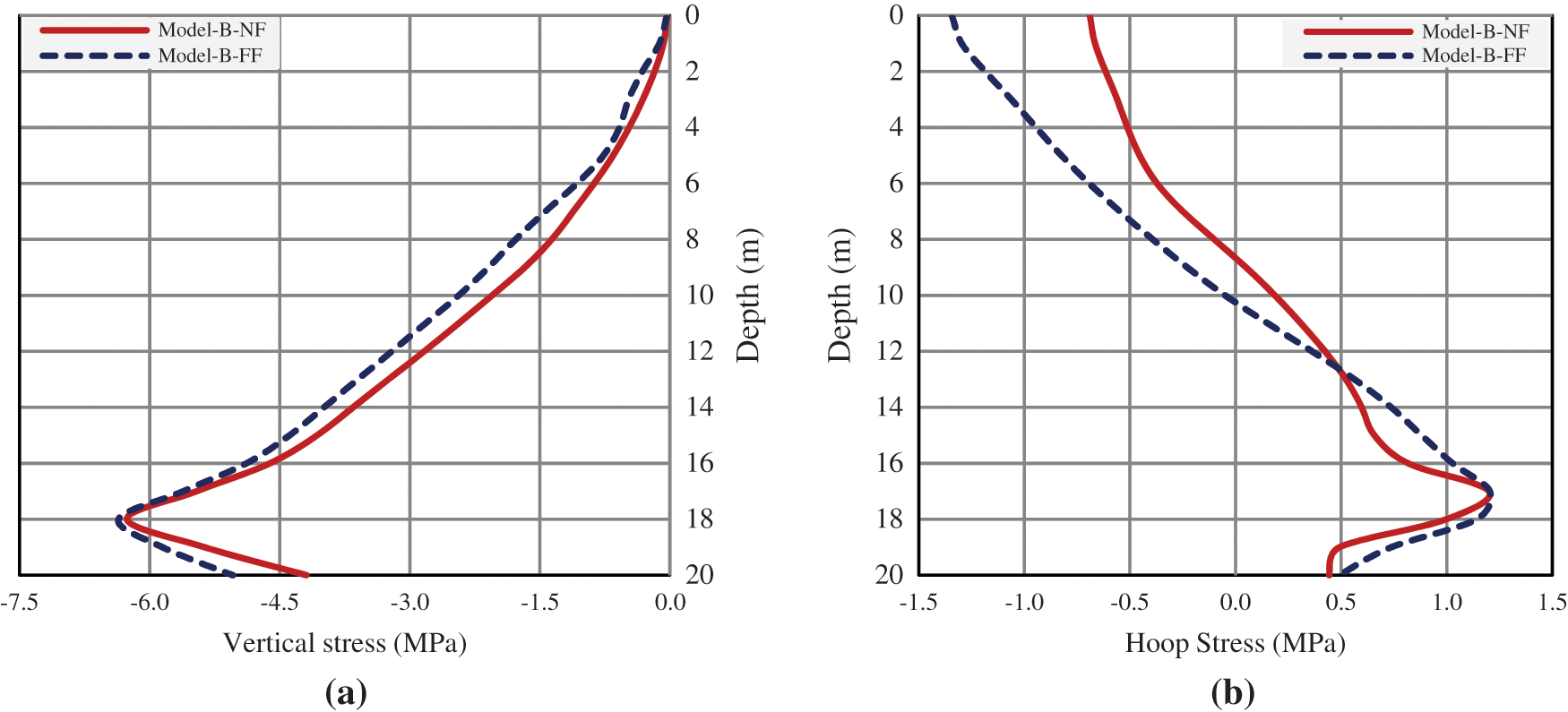
Figure 13: Model-B maximum wall stresses distribution; (a) Vertical stress, (b) Hoop stress

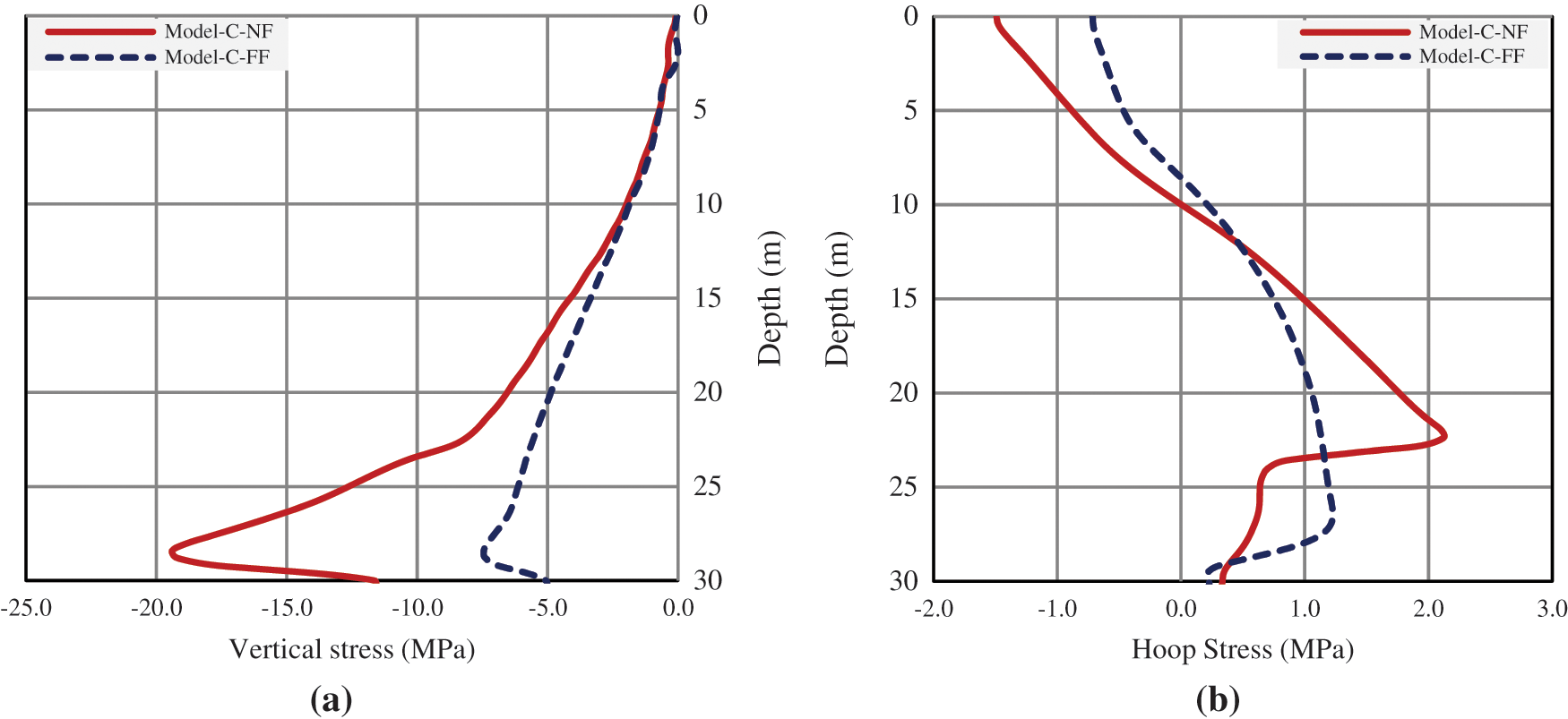
Figure 14: Model-C maximum wall stresses distribution; (a) Vertical stress, (b) Hoop stress

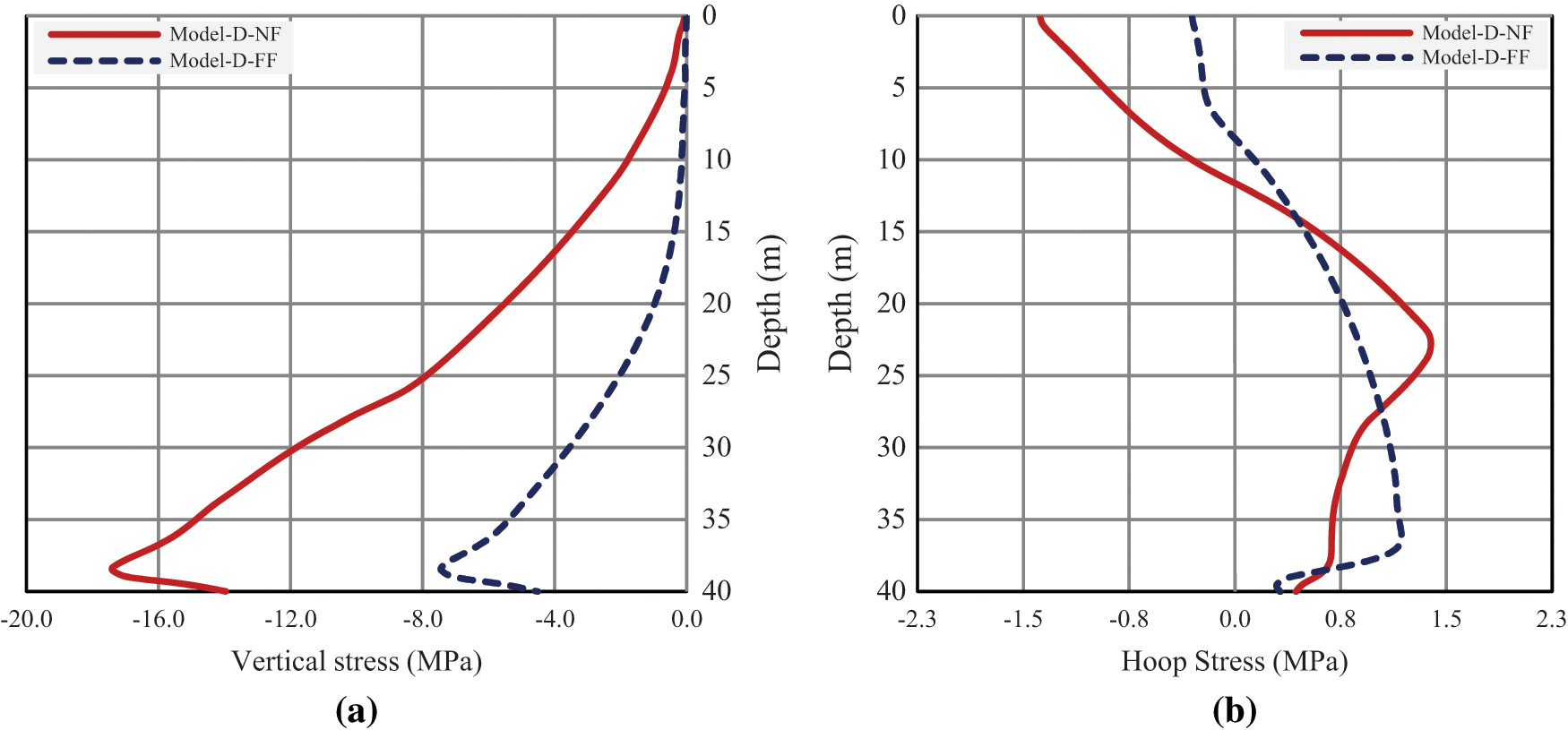
Figure 15: Model-D maximum wall stresses distribution; (a) Vertical stress, (b) Hoop stress

As can be observed, for the squat silo of Sr = 1.0, the far-field station response was greater than the response of the near-fault record for both stresses. This is the only case to get higher demand under FFGM than NFGM. On the other hand, the results of Model-B (Sr = 2.0) reveal equivalent stresses for the near-fault and far-field records, and the location of the peak stresses was at the bottom quarter of the silo for all cases. Nevertheless, Model-C (Sr = 3.0) has a remarkable performance in the near-fault record for both stresses. However, the behavior of Model-D (Sr = 4.0) has fluctuated over the type of ground motion. In which the hoop stresses for the NFGM and FFGM have yielded comparable values, meanwhile the NFGM has produced exceptional vertical stress compared to the vertical stress of the FFGM. This non-uniformity has not been recognized in the other models and the variations in their results were almost proportional to the type of stress. The reason behind the difference in Model-D results can be attributed to the occurrence of concrete tension damage in the near-fault record only; whereas the far-field scenario was free of any concrete damage as can be seen in Fig. 16. Consequently, this tension damage in the NFGM will force the structure to redistribute the tensile stress in the concrete as vertical stress. This conclusion promotes a critical fact about the importance of the near-fault ground motions in the seismic behavior of RC silos.

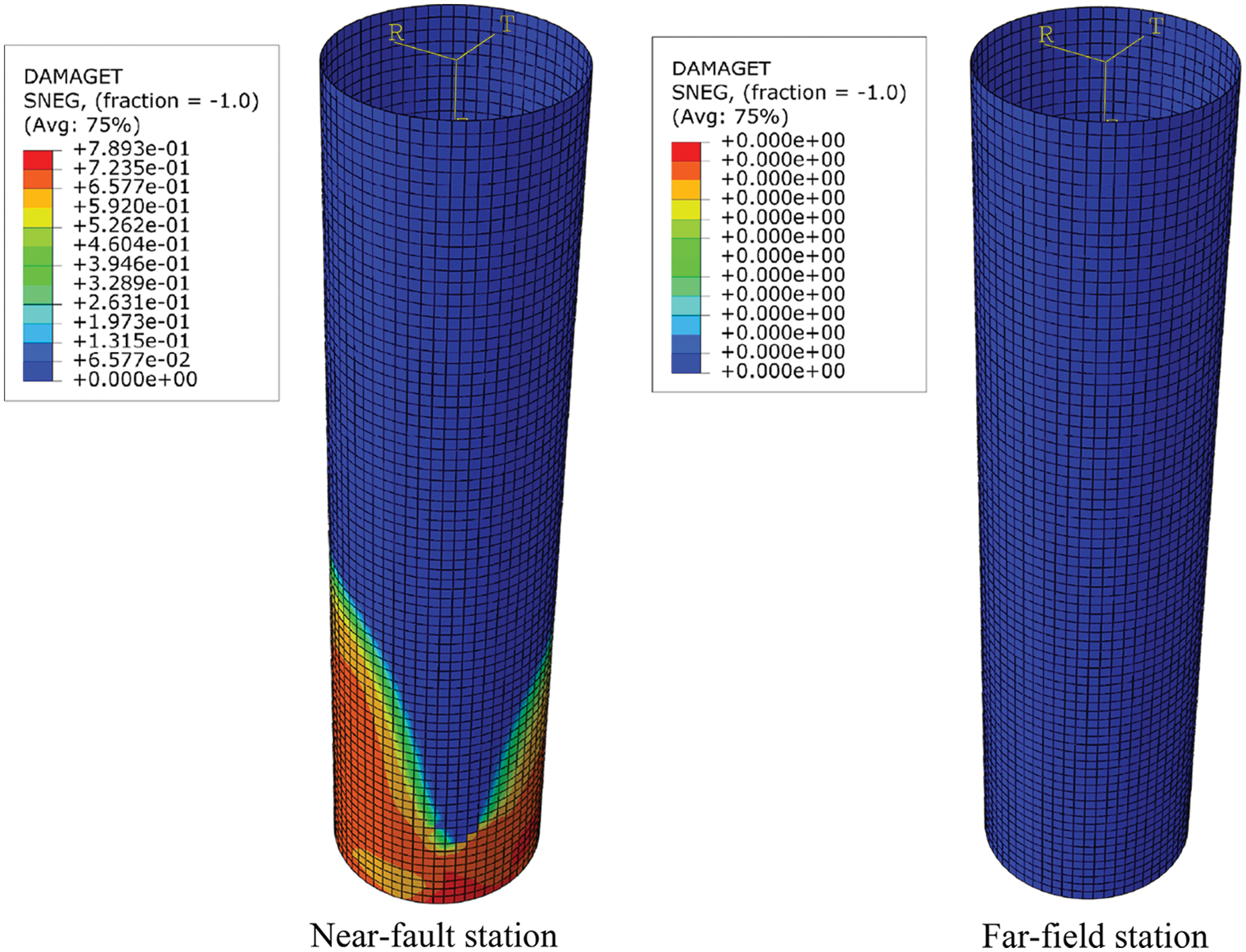
Figure 16: Model-D tension damage propagation due to Northridge earthquake

This distinction in the behavior of the squat-type silos and slender silos has been also noticed by previous studies [7,8]. Therefore, this judgment can be generalized for squat silos under any type of seismic excitations. On the other hand, Sr = 3.0 is proposed by this study as a critical aspect ratio due to its outstanding seismic demand under NFGM; which amend the ratio of Sr = 2.0 that was previously suggested by Durmuş et al. [7] for RC silos under conventional seismic excitations.

On the other hand, Table 6 summarizes the results of the maximum tensile hoop stress ( fh ) and the maximum compressive vertical stress ( fv ) of the four models. As can be demonstrated throughout all models, the ratios of the near-fault to the far-field results show overall compliance with the previously discussed ratios of the maximum base shear and overturning moment presented in Table 5. However, the only exception was for the near-fault results of Model-D, in which the response in terms of wall stresses was higher than the base shear and moment. Consequently, the ratio of NFGM to the FFGM was 117.7% for the maximum fv and 233.8% for maximum fh ; meantime the maximum base shear and maximum overturning moment have NFGM/FFGM values of 98.4% and 119.1%, respectively.

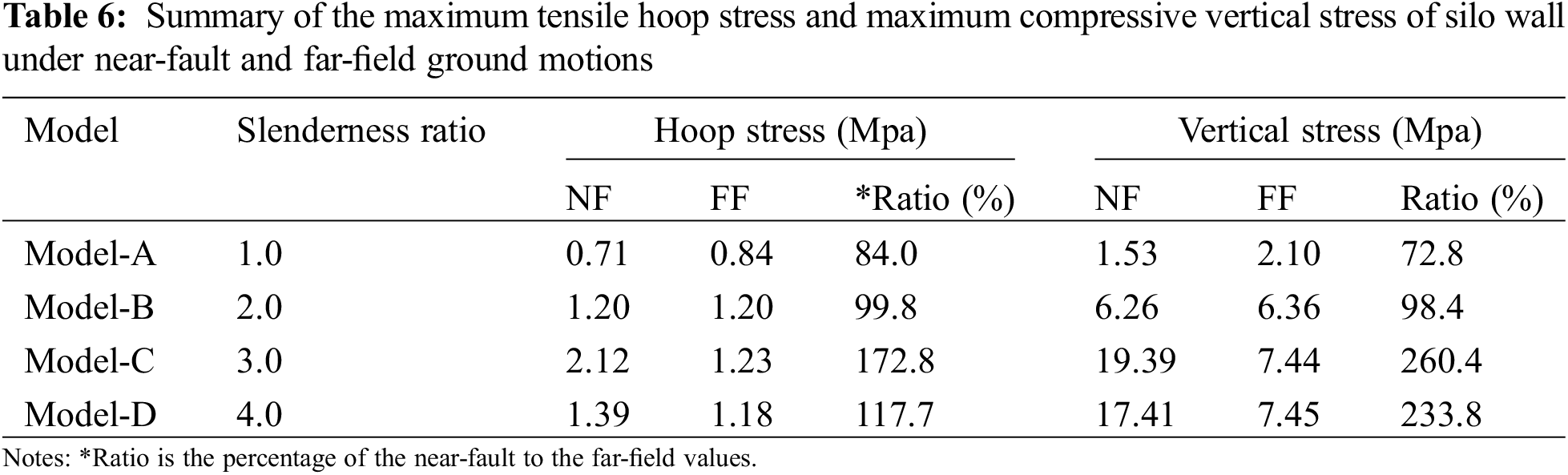
Finally, the tendency of the silo wall stresses in the near-fault record was climbing upward as the slenderness ratio (Sr) increased except for Sr = 3.0 which produced higher stresses than Sr = 4.0 as shown in Fig. 17. Nevertheless, the demand for far-field ground motions was moving up stably over the slenderness ratios, as illustrated in Fig. 18. It’s noticed for both NFGM and FFGM that the variations of the maximum hoop stresses were less than the changes in the peak vertical stresses. This difference is mainly due to the column packing effect of solid materials which transfers a portion of the lateral pressure of solids into a friction force on the surface of the silo walls. That portion increases as the column height of solid material increases resulting in additional axial stress and impacting the hoop stress distribution. Also, it can be illustrated that the positions of the maximum hoop stresses are mostly located in the middle region of silo depth for NFGM and close to the silo base for FFGM. Consequently, the observed distribution of the maximum hoop stresses of silos under FFGM is fully consistent with the results of the preceding works [1,7,8]; which are, apparently, not valid when silos are subjected to NFGM.

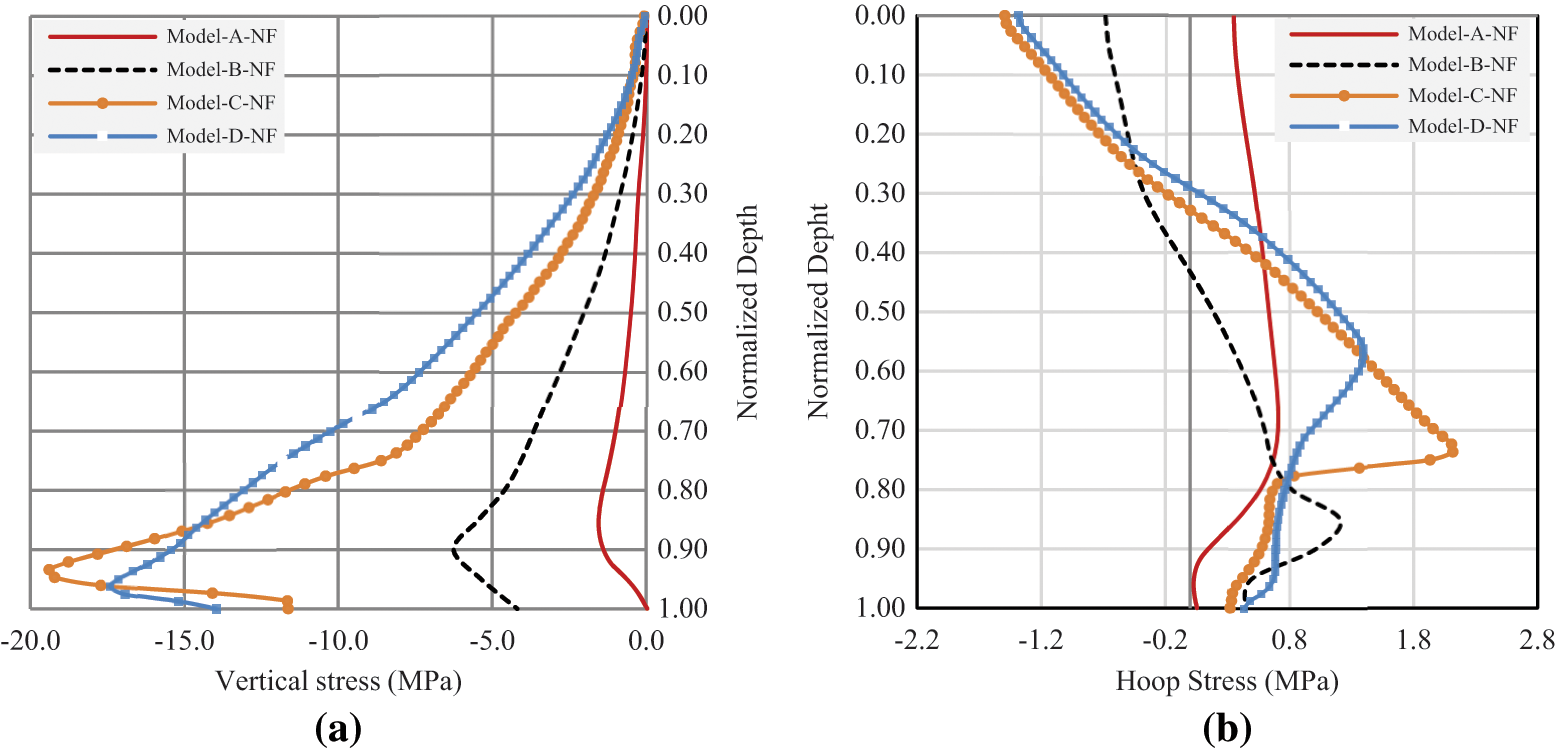
Figure 17: Maximum wall stresses distribution of the FE models under Near-fault ground motion; (a) Vertical stresses, (b) Hoop stresses

Figure 18: Maximum wall stresses distribution of the FE models under far-field record; (a) Vertical stresses, (b) Hoop stresses

5  Evaluation of the Seismic Codes under NFGM and FFGM

To compare the stress results of the finite element models under NFGM and FFGM, the Eurocode-8 procedure is selected as a reference for the seismic evaluation of silos. However, the response spectral acceleration will be extracted utilizing the Eurocode-1, AISC SEI 7/16, IBC-2012, UBC-97, and IS-1893/2016. Hence, these codes have been audited to obtain the code that predicts the highest stress demand. Consequently, the Eurocode-1 and AISC SEI 7/16 has yielded the highest values of the response spectral acceleration over all of the models for the selected near-fault and far-field ground motions that as shown in Fig. 5. As a result, the comparison in hoop stresses between the Eurocode-8 with Model-A (Sr = 1.0) and Model-B (Sr = 2.0) are presented in Figs. 19a and 19b, respectively. As can be seen, the Eurocode-8 procedure was able to control the demands of both ground motions. However, the Eurocode-8 was less effective in predicting the hoop stresses of Model-C (Sr = 3.0) and Model-D (Sr = 4.0) under NFGM as cleared in Fig. 20a. Meanwhile, the hoop stresses of the FFGM were successfully estimated by the Eurocode-8 as shown in Fig. 20b. These results confirm that the seismic codes are derived from far-field earthquakes. However, the effectiveness of the Eurocode-8 in the seismic analysis of silos experiencing FFGM complies with the conclusions of [8]; whereas, the outcomes of the NFGM disagree with their suggestions.

Figure 19: Comparing the hoop stresses of near-fault and far-field earthquakes with the Eurocode-8; (a) Model-A, (b) Model-B

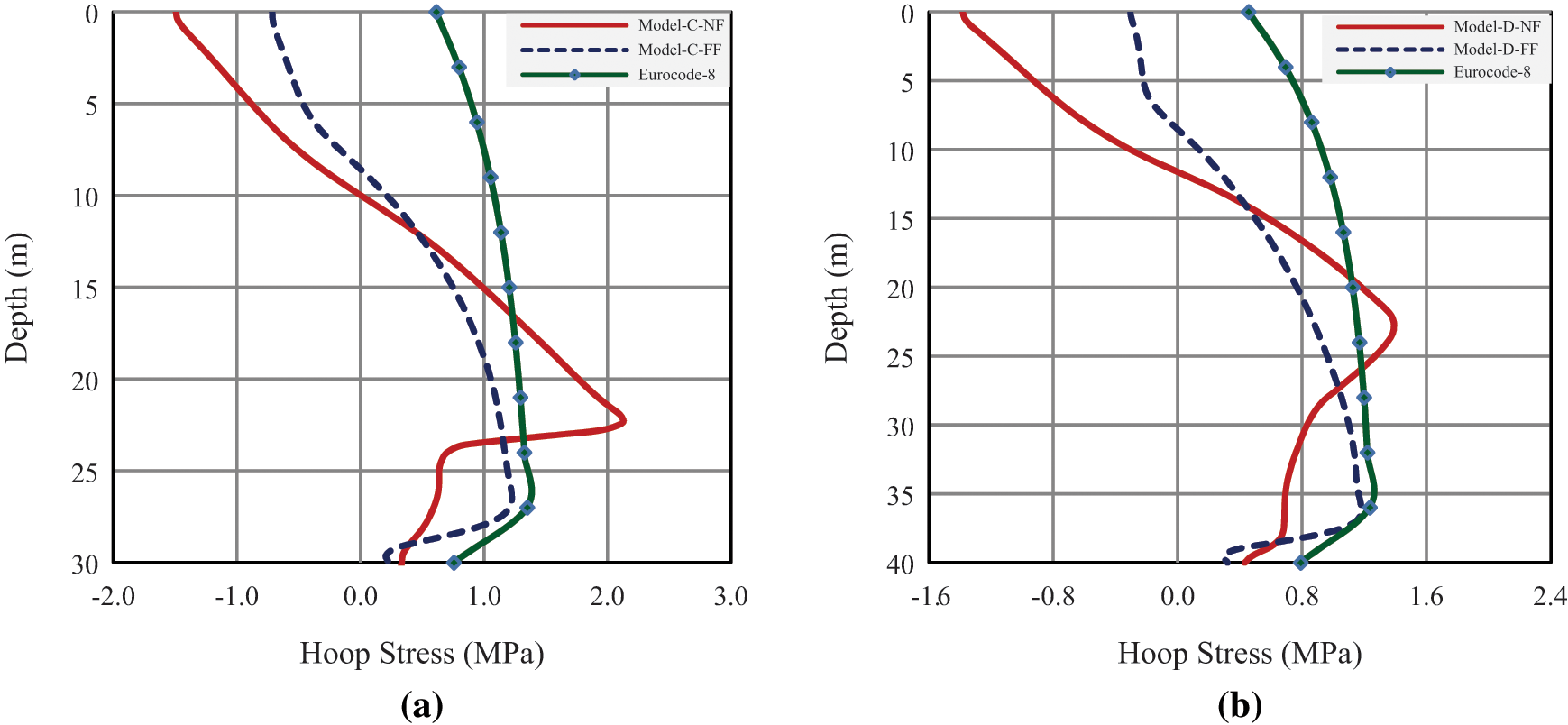
Figure 20: Comparing the hoop stresses of near-fault and far-field earthquakes with the Eurocode-8; (a) Model-C, (b) Model-D

6  Major Parameters Effect on the FE Results

The evaluation of the main input parameters will be executed utilizing a 20 m height, 10 m diameter, and 0.3 m thickness flat-bottomed reinforced concrete silo. The stored material is defined by the Drucker-Prager criterion and the interaction between the silo wall and the stored material was introduced utilizing Coulomb friction theory with constant friction of μ = 0.5, allowing material separation and loss of contact. The seismic loading is defined by the acceleration time history of the Chi-Chi_TCU102 earthquake (Mw = 7.62, Rrup = 1.49 km, and PGA = 0.212 g) as shown in Fig. 21. The material damping is added using Rayleigh factors with a damping ratio ξ = 5%. Hence, examining the impact of each parameter will be executed by varying its input values while other factors remain stationary. However, it is crucial to comprehend that the impact will not be the same using a different combination of the input parameters, but it does provide a general impression of the effectiveness of the parameters.

Figure 21: Acceleration time histories of Chi-Chi-Taiwan (1999) earthquake

6.1 Wall-Material Friction

The roughness between the silo wall and the stored material is a key parameter in determining the stored material pressures as has been discussed in the previous sections. The roughness herein is evaluated by the coefficient of the wall friction (μ). Therefore, three values of wall friction coefficient (μ = 0.2, μ = 0.3 and μ = 0.4) have been investigated in terms of maximum hoop stress. As can be demonstrated from Fig. 22, the maximum hoop stress has been raised by 32.6% when the wall friction coefficient decreases from 0.6 to 0.2. This growth can be assigned to the role of the wall friction in transferring a fraction of the lateral pressure of the stored material as a vertical compressive pressure in the silo wall. Accordingly, low values of μ will transform less lateral pressure as vertical stress and higher hoop stress in the wall of the RC silo.

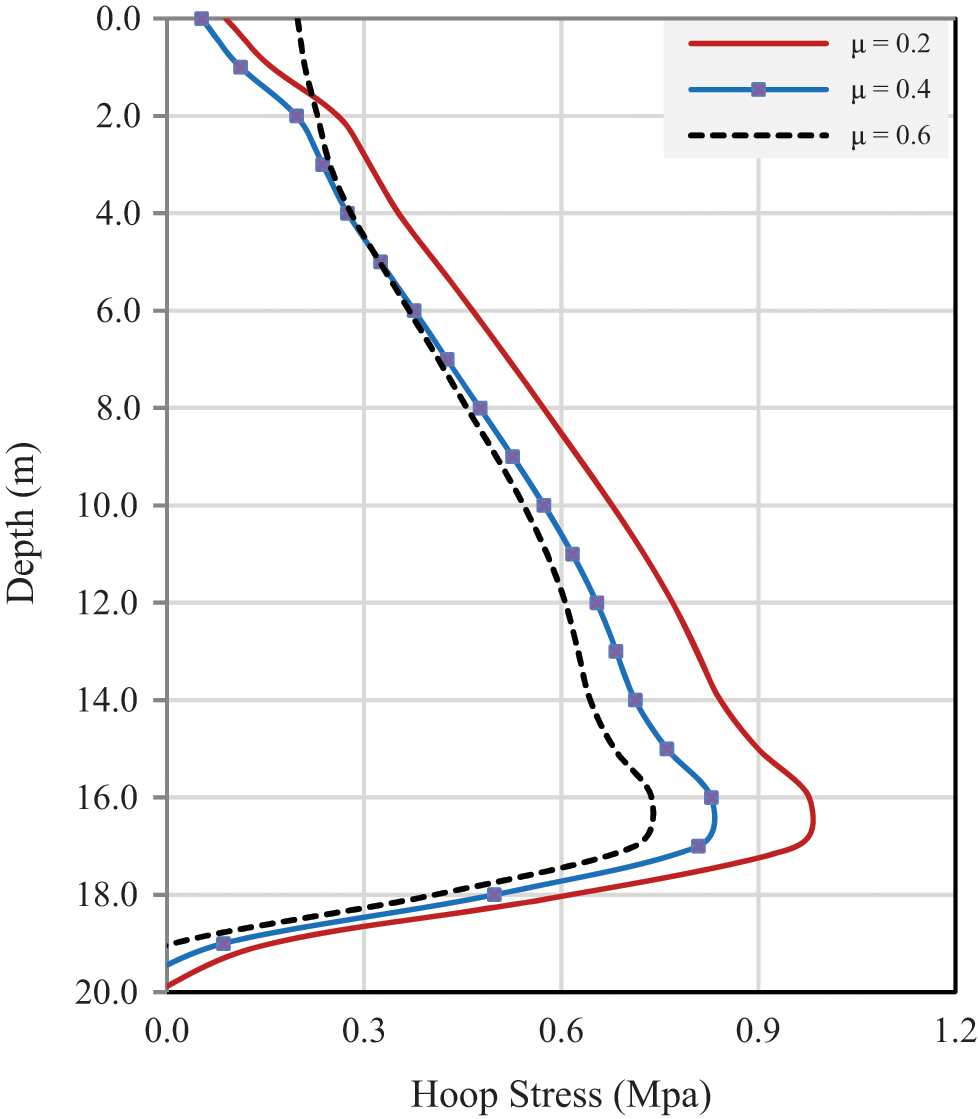
Figure 22: The effect of wall friction coefficient (μ) on the seismic hoop stress of the FE model

6.2 Poisson’s Ratio of the Stored Material

Poisson’s ratio (ν) or the ratio of the lateral strain to the longitudinal strain of the stored granular material is a major contributor to the computation of the granular pressure. There is a strong relationship between the Poisson’s ratio (ν) and the lateral pressure coefficient as has been previously shown in Eq. (5). However, this effect under seismic excitation is assessed by employing Poisson’s ratio (ν) of ν = 0.2, ν = 0.3, and ν = 0.4 as can be seen in Fig. 23. The result indicates an increment of 53.5% in the silo wall hoop stress when moving the Poisson’s ratio (ν) from 0.2 to 0.4.

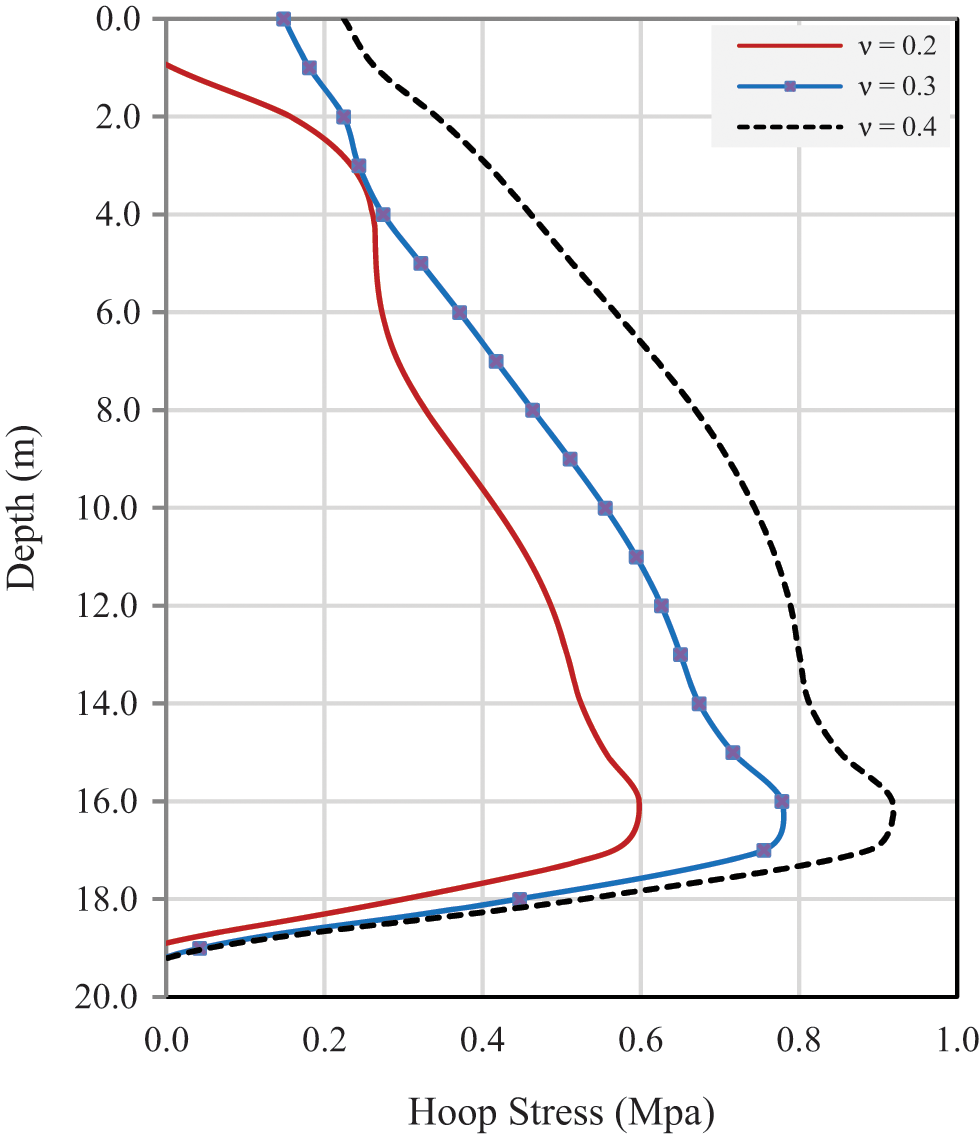
Figure 23: The effect of stored material Poisson’s ratio (ν) on the seismic hoop stress of the FE model

6.3 The Angle of Internal Friction

The internal friction angle (ϕ) of the stored material is one of the parameters that have been adopted in the Drucker-Prager model and the classic theories of describing the behavior of the material in the static and dynamic phases. The significance of that parameter can be observed in the plastification pheromone of the stored material. Accordingly, lower values of the internal friction angle are expected to ease the plastification process in the stored material; which leads to producing higher lateral pressure. Hence, this claim has been proven by examining various values of ϕ to determine its influence on the dynamic hoop stress of silo walls. The results have recorded a 36.2% increase in the maximum dynamic hoop stress when the internal friction angle is reduced from 60° to 20°.

6.4 The Dilation Angle

The dilation angle (ψ) is one of the components that characterize the behavior of granular material of an elastic-plastic type. So, it defines the volumetric change of a particulate material when it suffers shearing. The value of the dilation angle may vary from zero up to the angle of internal friction (ϕ). However, testing different values of ψ has deduced that the dilation angle has a modest influence on the yielded dynamic hoop stress of about 2% growth when ψ was changed from 10° to 50°.

7  Summary and Conclusions

This study aims to numerically examine the dynamic seismic behavior of cylindrical flat-bottomed reinforced concrete silos, taking into account the influence of near-fault and far-field ground motions. The concrete damage plasticity was utilized for the silo wall and the granular material was represented by the Drucker-Prager plastic model. In addition, the interaction between the silo wall and ensiled material was introduced by Coulomb’s constant friction theory; permitting material separation and local loss of contact. The proposed model was verified with experimental data and theoretical expressions. However, the impact of slenderness ratio (Sr) was incorporated by examining four slenderness ratios of Sr = 1.0, Sr = 2.0, Sr = 3.0, and Sr = 4.0. The main conclusions of this study are as follows:

1.    There is a remarkable difference in the seismic response of the RC silos under NFGM and FFGM in terms of stresses, base shear, overturning moments, and concrete damage propagation. This conclusion is valid for all the four studied models under earthquakes with an identical PGA. Hence, the time profile of ground motions plays a significant role in the seismic behavior of silos.

2.    The slenderness ratio (Sr) of RC silos has a direct impact on the behavior of silos under seismic loading. However, the seismic responses to NFGM and FFGM have fluctuated over various slenderness ratios.

3.    Squat silos (Sr ≤1.0 ) have unique seismic performance compared to slender silos. For instance, the FFGM dominates the seismic behavior of squat silos, and their distribution of wall stresses produces perfectly compressive vertical stresses and plain tensile hoop stresses for both NFGM and FFGM.

4.    Slender silos tend to endure larger seismic structural demand undergoing NFGM. However, the growth in the demand is not consistent with the increment of the slenderness ratio. Thus, the NFGM is found to impose spectacular seismic demand for the slender silo of Sr = 3.0 in respect of base shear, overturning moments, and wall stresses. Moreover, the distribution of the hoop stresses in slender silos has recorded an inflection depth in which the hoop stress inverted from tensile to compressive at the upper part of the silo. The difference in the distribution of stresses between the squat and slender silos is attributed to the effect of granular material packing pheromone which is a characteristic of wall-granular material interaction. Despite the influence of the material interaction in the seismic behavior of silos, the Eurocode-8 does not account for it in the calculations.

5.    The position of the maximum hoop and vertical stresses is found to be near the base of the silo for the FFGM; whereas, the maximum hoop stresses of the NFGM are located closer to the middle part of the silo depth. Hence it is suggested to assign the max hoop steel reinforcement for the entire depth in the seismic design of RC silos.

6.    The overall concrete damage propagation is more aggressive in the RC silo walls exposed to NFGM than those subjected to FFGM. Silos subjected to NFGM suffer concrete tension damage in high values of slenderness ratios (Sr = 3 and Sr = 4); meanwhile, there is no damage has occurred at low slenderness ratios (Sr = 2 and Sr = 2). However, the FFGM cause tension damage for a single case of Sr = 3, while all other models were free of damage. In terms of concrete compression crush, the slenderness ratio of Sr = 3.0 under NFGM is the only case that was found to have such failure. These conclusions illustrate two facts about the seismic behavior of RC silos; the first one suggests the tensile stress as the critical stress since it is the primary cause of concrete wall failures. Secondly, high-grade concrete is recommended in the seismic design of silos under NFGM to avoid immediate structural collapse and to ensure the satisfaction of the secondary compression failure concept.

7.    The Eurocode-8 part-4 provisions are adequate to control the seismic response of RC silos under FFGM and less effective in the NFGM. This behaviour under NFGM is particularly valid for slender silos of Sr ≥3.0 . The explanation of the Eurocode-8 being less effective with the NFGM can be assigned to two main reasons; firstly, the Eurocode-8 provides an alike procedure for silos of different slenderness ratios. Secondly, the interaction between the silo wall and the stored material is not considered in the Eurocode.

8.    The selection of the granular material properties has an enormous impact on the dynamic stresses of silos. Poisson’s ratio (ν), the wall-granular material friction coefficient (μ), and the angle of internal friction (ϕ) are the major parameters in the seismic response of reinforced concrete silos.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Djelloul, Z., Mohammed, D. (2018). Contribution to the seismic behavior of steel silos: Full finite-element analysis versus the eurocode approach. Asian Journal of Civil Engineering, 19(7), 757–773. DOI 10.1007/s42107-018-0062-z. [Google Scholar] [CrossRef]

 2.  Chavez Sagarnaga,J.,Kulkarni, A. D. (2020). The need for a comprehensive standard/code for the design of bins and silos in the USA. Structures Congress, pp. 349–360. DOI 10.1061/9780784482230.034. [Google Scholar] [CrossRef]

 3.  Matthias, M. C. (2020). Concrete silos: Failures, design issues and repair/strengthening methods. Applied Sciences, 10, 663–695 DOI 10.3390/app10113938. [Google Scholar] [CrossRef]

 4.  Mahamid, M., Gaylord, H., Gaylord, C. (2020). Structural engineering handbook, Fifth Edition. NY, USA: McGraw Hill. [Google Scholar]

 5.  Eurocode-8 (2006). Design of structures for earthquake resistance–Part 4: Silos, tanks, and pipelines. European Committee for Standardization, rue de Stassart-36, B-1050 Brussels. [Google Scholar]

 6.  ACI 313-16 (2016). Design Specification for Concrete Silos and Stacking Tubes for Storing Granular Materials (ACI 313-16) and Commentary. American Concrete Institute, Farmington Hills, MI, USA. [Google Scholar]

 7.  Durmuş Demir, A., Livaoğlu, R. (2019). The role of slenderness on the seismic behavior of ground-supported cylindrical silos. Advances in Concrete Construction, 7, 65–74. DOI 10.12989/acc.2019.7.2.065. [Google Scholar] [CrossRef]

 8.  Butenweg, C., Rosin, J., Holler, S. (2017). Analysis of cylindrical granular material silos under seismic excitation. Buildings, 7, 61. DOI 10.3390/buildings7030061. [Google Scholar] [CrossRef]

 9.  Saleem, M., Khurshid, H., Qureshi, H., Siddiqi, Z. (2018). A simplified approach for analysis and design of reinforced concrete circular silos and bunkers. The Open Construction and Building Technology Journal, 12, 234–250. DOI 10.2174/1874836801812010234. [Google Scholar] [CrossRef]

10. Silvestri, S., Ivorra, S., Chiacchio, L. D., Trombetti, T., Foti, D. et al. (2016). Shaking-table tests of flat-bottom circular silos containing grain-like material. Earthquake Engineering & Structural Dynamics, 45(1), 69–89. DOI 10.1002/eqe.2617. [Google Scholar] [CrossRef]

11. Abd el-Rahim, H. H. A. (2013). Response of cylindrical elevated wheat storage silos to seismic loading. JES. Journal of Engineering Sciences, 41(6), 2079–2102. DOI 10.21608/jesaun.2013.114948. [Google Scholar] [CrossRef]

12. Benkhellat, S., Kada, O., Seghir, A., Kadri, M. (2021). Seismic damage assessment of reinforced concrete grain silos. International Journal of Structural Stability and Dynamics, 22(1), 2250005. DOI 10.1142/S0219455422500055. [Google Scholar] [CrossRef]

13. El-Arab, I. E. (2014). Seismic analysis of RC silos dynamic discharge phenomena. International Journal of Engineering and Advanced Technology, 4, 91–99. [Google Scholar]

14. Livaoğlu, R., Durmuş, A. (2015). Investigation of wall flexibility effects on seismic behavior of cylindrical silos. Structural Engineering and Mechanics, 53, 159–172. DOI 10.12989/sem.2015.53.1.159. [Google Scholar] [CrossRef]

15. Mansour, S., Pieraccini, L., Palermo, M., Foti, D., Gasparini, G. et al. (2022). Comprehensive review on the dynamic and seismic behavior of flat-bottom cylindrical silos filled with granular material. Frontiers in Built Environment, 7, 805014. DOI 10.3389/fbuil.2021.805014. [Google Scholar] [CrossRef]

16. Maraveas, C. (2020). Concrete silos: Failures, design issues and repair/strengthening methods. Applied Sciences, 10(11), 3938. DOI 10.3390/app10113938. [Google Scholar] [CrossRef]

17. Xu, Q., Zhang, H., Liu, Q., Wang, L. (2021). Seismic analysis on reinforced concrete group silos through shaking table tests. Structural Concrete, 22(3), 1285–1296. DOI 10.1002/suco.202000207. [Google Scholar] [CrossRef]

18. Jouneghani, K., Hosseini, M., Rohanimanesh, M., Raissi Dehkordi, M. (2017). Evaluating main parameters effects of near-field earthquakes on the behavior of concrete structures with moment frame system. Advances in Science and Technology Research Journal, 11, 10–23. DOI 10.12913/22998624/74135. [Google Scholar] [CrossRef]

19. Zhou, J., Sun, C., Dai, X., Chen, G. (2019). Seismic reliability assessment of inelastic SDOF systems subjected to near-fault ground motions considering pulse occurrence. Structural Durability & Health Monitoring, 13(4), 361–378. DOI 10.32604/sdhm.2019.05171. [Google Scholar] [CrossRef]

20. Sgobba, S., Lanzano, G., Pacor, F., Felicetta, C. (2021). An empirical model to account for spectral amplification of pulse-like ground motion records. Geosciences, 11(1), 15. DOI 10.3390/geosciences11010015. [Google Scholar] [CrossRef]

21. Bray, J. D., Rodriguez-Marek, A. (2004). Characterization of forward-directivity ground motions in the near-fault region. Soil Dynamics and Earthquake Engineering, 24(11), 815–828. DOI 10.1016/j.soildyn.2004.05.001. [Google Scholar] [CrossRef]

22. Zhao, D., Wang, H., Wang, D., Zhu, R., Zhang, J. (2022). Quantitative classification of near-fault ground motions selected by energy indicators. Structures, 35, 780–791. DOI 10.1016/j.istruc.2021.11.032. [Google Scholar] [CrossRef]

23. Kohrangi, M., Vamvatsikos, D., Bazzurro, P. (2018). Pulse-like versus non-pulse-like ground motion records: Spectral shape comparisons and record selection strategies. Earthquake Engineering & Structural Dynamics, 48(1), 48–64. DOI 10.1002/eqe.3122. [Google Scholar] [CrossRef]

24. Yang, J., Li, P., Jing, H., Gao, M. (2021). Near-fault ground motion influence on the seismic responses of a structure with viscous dampers considering SSI effect. Advances in Civil Engineering, 2021, 6649124. DOI 10.1155/2021/6649124. [Google Scholar] [CrossRef]

25. Chang, T. Y., Cotton, F., Tsai, Y. B., Angelier, J. (2004). Quantification of hanging-wall effects on ground motion: Some insights from the 1999 Chi-chi earthquake. Bulletin of the Seismological Society of America, 94(6), 2186–2197. DOI 10.1785/0120030233. [Google Scholar] [CrossRef]

26. Kalkan, E., Kunnath, S. K. (2006). Effects of fling step and forward directivity on seismic response of buildings. Earthquake Spectra, 22(2), 367–390. DOI 10.1193/1.2192560. [Google Scholar] [CrossRef]

27. Dang, P., Cui, J., Liu, Q. (2022). Parameter estimation for predicting near-fault strong ground motion and its application to Lushan earthquake in China. Soil Dynamics and Earthquake Engineering, 156, 107223. DOI 10.1016/j.soildyn.2022.107223. [Google Scholar] [CrossRef]

28. Eurocode-1 (2006). Actions on structures–Part 4: Silos and tanks. European Committee for Standardization, rue de Stassart-36, B-1050 Brussels. [Google Scholar]

29. Nguyen, Q. T., Livaoğlu, R. (2020). The effect of the ratio of Λ-shaped shear connectors on the flexural behavior of a reinforced concrete frame. Advances in Structural Engineering, 23(12), 2724–2740. DOI 10.1177/1369433220920442. [Google Scholar] [CrossRef]

30. Mehretehran, A., Maleki, S. (2020). Evaluation of normal pressures during filling in steel hoppers with eccentric outlet. Journal of Civil Engineering and Construction, 9, 138–149. DOI 10.32732/jcec.2020.9.3.138. [Google Scholar] [CrossRef]

31. Wang, Y., Lu, Y., Ooi, J. (2015). A numerical study of wall pressure and granular flow in a flat-bottomed silo. Powder Technology, 282, 43–54. DOI 10.1016/j.powtec.2015.01.078. [Google Scholar] [CrossRef]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.