Journal of Renewable Materials

DOI: 10.32604/jrm.2021.015166

ARTICLE

Characterization of the Flexural Behavior of Bamboo Beams

Limin Tian1,2, Jianpeng Wei3, Jiping Hao1,*and Qiushuo Wang1

1School of Civil Engineering, Shaanxi Key Laboratory of Structure and Earthquake Resistance, Xi’an University of Architecture and Technology, Xi’an, 710055, China
2The Key Laboratory of Plateau Building and Eco-Community in Qinghai, Qinghai Building and Materials Research Co., Ltd., Xining, 810008, China
3Anhui Province Key Laboratory of Green Building and Assembly Construction, Anhui Institute of Building Research & Design, Hefei, 230031, China
*Corresponding Author: Jiping Hao. Email: haojiping@xauat.edu.cn
Received: 27 November 2020; Accepted: 19 February 2021

1  Introduction

Bamboo is a sustainable material used in the construction of buildings [1,2]. It is renewable and environmentally friendly. Its growth speed and strength are greater than those of timber. Bamboo is a typical anisotropic material. García et al. [3] and Akinbade et al. [4] studied the transverse properties of bamboo, testing the elastic constants and tensile capacity in the transverse direction. The longitudinal properties of bamboo were also investigated. Liu et al. [5] measured the longitudinal tensile strength and elastic modulus. Qiu et al. [6] constructed a longitudinal constitutive model using the Ramberg–Osgood relation. These studies indicated that the tensile strength of bamboo in the longitudinal direction was close to the strength of steel while the elastic modulus of bamboo was approximately one-twentieth of that of steel. Hence, bamboo is considered suitable for use as a centrally stressed member [7–12]. As one of the most important members of a structure, understanding the flexural behavior of bamboo beams is extremely important to increase their application in construction [2,13]. Currently, two types of bamboo beams have been widely studied: Original and engineered bamboo beams.

For original bamboo beams, the natural shape of the bamboo is maintained; this advantage can be exploited in structures. García-Aladín et al. [14,15] conducted bending tests on single and double bamboo poles to calculate their shear moduli and stiffnesses. Trujillo et al. [16] implemented more than 200 bending tests on bamboo poles, which were used for bamboo strength grading. Nurmadina et al. [17] adopted three-point bending tests to obtain the bending stiffness of a single bamboo pole. Lorenzo et al. [18] proposed an analytical bimodulus model to determine the strain and stress distribution of a single bamboo beam. These studies indicated that owing to the deflection limit, it was difficult to achieve large spans using a single bamboo pole as a beam [19,20]. Considering the superiority of original bamboo, a bionic bamboo beam, including tubes and diaphragms, was invented [21,22]. Its bending model was deduced using Euler-Bernoulli beam theory.

To increase the applicability of original bamboo beams, Tian et al. [23] presented a composite mortar-sprayed bamboo beam. The results demonstrated that interfacial slippage between two bamboo poles was difficult to avoid and the composite effect could not be fully exploited. Therefore, bolted connections are commonly applied to avoid interfacial slip [24–28]. Conversely, the engineered bamboo beam is a new bamboo product, the section of which can be varied and the bending stiffness can be determined according to the design requirement [29,30]. Huang et al. [31] and Li et al. [32] analyzed the flexural behavior of engineered bamboo beams and their ultimate bearing capacity and deformation were deduced. Furthermore, engineered bamboo can be effectively combined with other materials (i.e., steel, concrete, and timber) to form new composite bamboo beams. Wei et al. [33] and Wang et al. [34] proposed a fiber-reinforced engineered bamboo beam and a bamboo-concrete composite beam, respectively. Zhong et al. [35] and Wei et al. [36] adopted steel bars to enhance the engineered bamboo beam. Li et al. [37] presented an I-section bamboo-steel composite beam. However, producing engineered bamboo is energy consuming and the process is not environmentally friendly.

To completely exploit the excellent performance of original bamboo beams, this study investigated the flexural behavior of single-pole and double-pole bamboo beams via theoretical analysis. Simultaneously, the influence of interfacial slippage was analyzed. Then, an effective configuration to avoid slippage without destroying the original bamboo was proposed and validated.

2  Flexural Behavior Analysis

2.1 Constitutive Model Along the Grain

The flexural behavior of bamboo beams is directly related to the constitutive model of bamboo along the grain, as shown in Fig. 1 and Eq. (1). Et and Ec represent the elastic moduli in the tensile and compressive states, respectively; σtu and σcu denote the ultimate stresses; εtu and εcu define the ultimate strains. εcp denotes the strain at the compressive yield point. The constitutive model includes two aspects: Tension and compression [6,31,32]. Based on previous tests [3–6], bamboo exhibits a linear elastic strain-stress relationship in the tensile state and fractures occur corresponding to the ultimate stress. There is almost no plastic deformation stage. Bamboo approximates an ideal elastoplastic material under a compressive load. Moreover, the elastic modulus in the tensile state is always greater than that under compression.

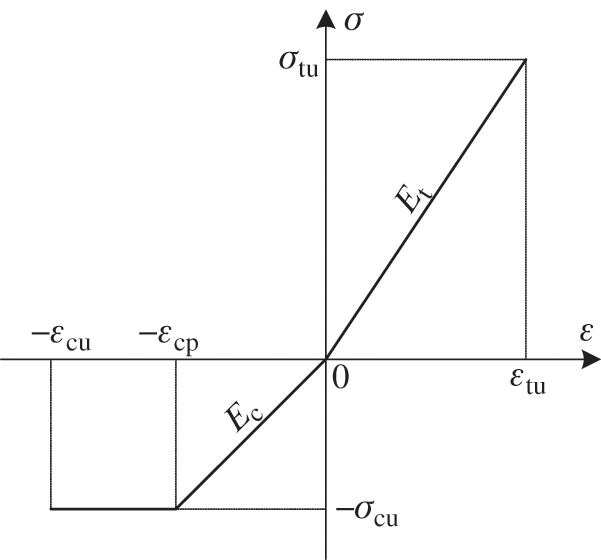
Figure 1: The constitutive model of bamboo along the grain

σ(ε)={−σcu−εcu≤ε≤−εcpEcε−εcp≤ε≤0Etε0≤ε≤εtu (1)

2.2 Curvature of a Single-Pole Bamboo Beam

2.2.1 Elastic Stage

Fig. 2 presents the stress and strain distributions in the elastic stage for a single-pole bamboo beam, conforming to the plane-section assumption. Owing to the greater elastic modulus in the tensile zone, the neutral axis is below the midline of the beam. The cross-section of bamboo is assumed to be an ideal annulus with invariable thickness. A polar coordinate system is established such that the origin of the coordinate system is located at the center of the section and the lower part of the vertical symmetry axis is used as the polar axis.

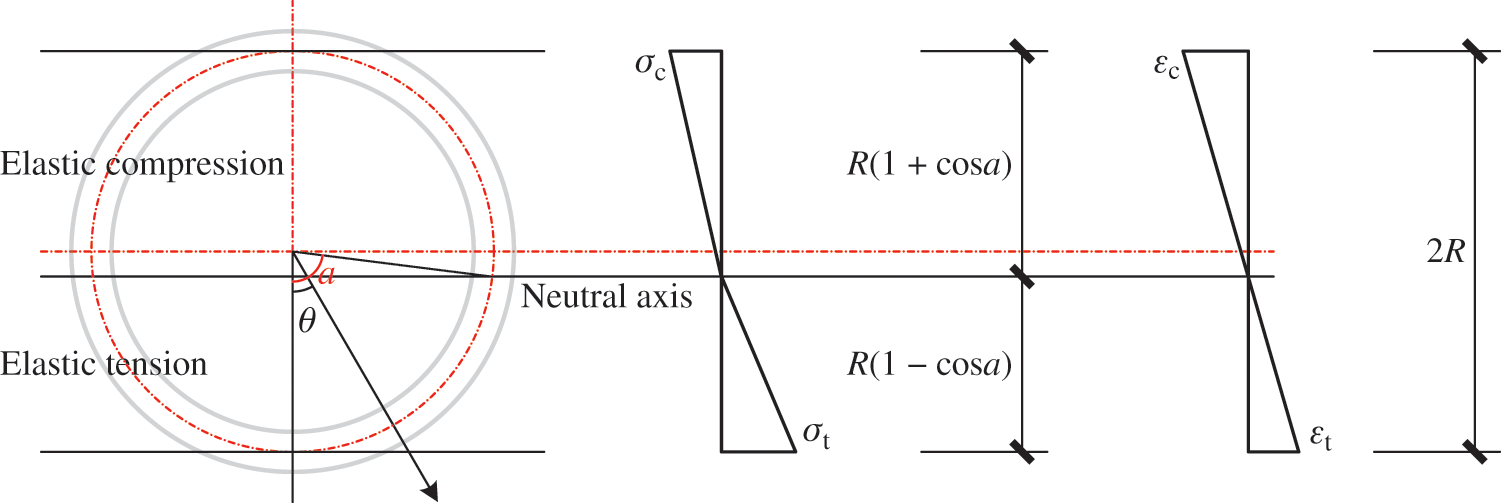
Figure 2: Stress and strain distributions during the elastic stage

The strain and stress on the central line of the annulus are adopted to represent the strain and stress at the same polar angle. Thus, the area integral can be simplified as the arc integral in the following deduction. Eq. (2) expresses the stress distribution along the central line of the annulus, where the stress is a function of the polar angle.

σ(θ)={cos⁡θ−cos⁡a1−cos⁡aσt0≤θ≤acos⁡θ−cos⁡aβ(1−cos⁡a)σta≤θ≤π(β=Et/Ecβ≥1) (2)

where σt is the tensile stress at the bottom surface and θ is the polar angle. The polar angle a corresponds to the location of the neutral axis. β is the ratio of Et to Ec . The polar angle a can be calculated by the longitudinal force equilibrium, as follows:

∫0πσ(θ)Rtdθ=0 (3)

∫0acos⁡θ−cos⁡a1−cos⁡aσtRtdθ+∫aπcos⁡θ−cos⁡aβ(1−cos⁡a)σtRtdθ=0 (4)

sin⁡a−acos⁡a1−cos⁡a+−πcos⁡a−sin⁡a+acos⁡aβ(1−cos⁡a)=0 (5)

tan⁡a−a=πβ−1 (6)

where R is the radius of the central line of the annulus and t is the thickness of the annulus. In Eq. (6), the polar angle a is only relevant to β , illustrating that the neutral axis does not change during the elastic stage. The bending moment in this section is expressed as follows:

∫0πσ(θ)(cos⁡θ−cos⁡a)R2tdθ=M/2 (7)

∫0a(cos⁡θ−cos⁡a)21−cos⁡aσtR2tdθ+∫aπ(cos⁡θ−cos⁡a)2β(1−cos⁡a)σtR2tdθ=M/2 (8)

−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.5π+πcos2a+0.75sin⁡2a−0.5a−acos2aβ(1−cos⁡a)=M2σtR2t (9)

λ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.5π+πcos2a+0.75sin⁡2a−0.5a−acos2aβ(1−cos⁡a) (10)

M=2λσtR2t (11)

When the polar angle a is solved using Eq. (6), the constant coefficient λ can be obtained using Eq. (10). Then, the bending moment can be obtained using Eq. (11). The bending moment is proportional to σt . The relationship between strain and the neutral axis displayed in Fig. 2 leads to:

εtεc=σtEcσcEt=αeβ=1−cos⁡a1+cos⁡a(αe=σt/σcσc≤σcu) (12)

where εt represents the tensile strain at the bottom surface and εc signifies the compressive strain at the top surface. Substituting σt=αeσcu into Eq. (11) yields:

Mp=2λαeσcuR2t=2λβ1−cos⁡a1+cos⁡aσcuR2t (13)

where Mp is the maximum bending moment of the elastic stage. When the bending moment is smaller than Mp , the curvature can be calculated as follows:

ke=σtEt(1−cos⁡a)R=M2λ(1−cos⁡a)EtR3tM≤Mp (14)

2.2.2 Elastic-Plastic Stage

Fig. 3 shows the stress and strain distributions during the elastic-plastic stage. The cross-section of bamboo comprises three areas: Elastic tension, elastic compression, and plastic compression. Two dividing lines, corresponding to angles a and b , divide the three areas.

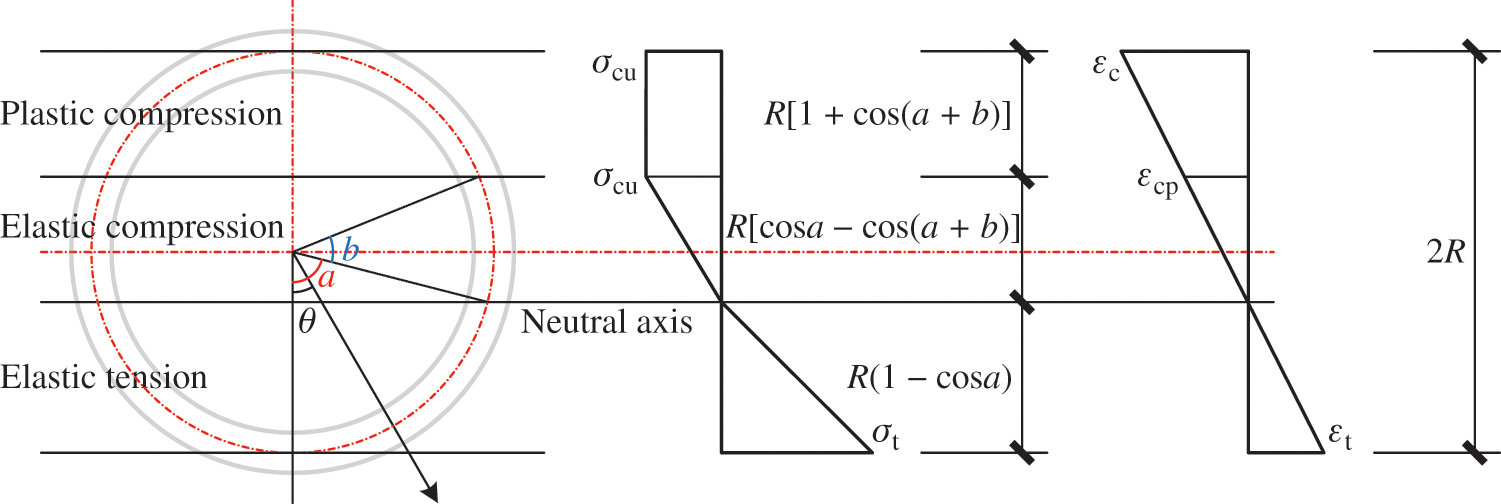
Figure 3: Stress and strain distributions during the elastic-plastic stage

Eq. (15) expresses the stress distribution during the elastic-plastic stage, which comprises three parts.

σ(θ)={cos⁡θ−cos⁡a1−cos⁡aσt0≤θ≤acos⁡θ−cos⁡aβ(1−cos⁡a)σta≤θ≤a+b−σcu(a+b)≤θ≤π (15)

Considering the deformation relationship over the section yields:

εtεcp=σtEcσcuEt=αpβ=1−cos⁡acos⁡a−cos⁡(a+b)(αp=σt/σcuαeσcu≤σt≤σtm) (16)

b=arccos⁡[cos⁡a−βαp(1−cos⁡a)]−a (17)

where σtm is the maximum value of σt . The force equilibrium along the longitudinal direction yields.

∫0πσ(θ)Rtdθ=0 (18)

∫0acos⁡θ−cos⁡a1−cos⁡aσtRtdθ+∫aa+bcos⁡θ−cos⁡aβ(1−cos⁡a)σtRtdθ+∫a+bπ−σcuRtdθ=0 (19)

sin⁡a−acos⁡a1−cos⁡a+sin⁡(a+b)−sin⁡a−bcos⁡aβ(1−cos⁡a)−π−a−bαp=0 (20)

The bending moment of the section is expressed as

∫0πσ(θ)(cos⁡θ−cos⁡a)R2tdθ=M/2 (21)

∫0a(cos⁡θ−cos⁡a)21−cos⁡aσtR2tdθ+∫aa+b(cos⁡θ−cos⁡a)2β(1−cos⁡a)σtR2tdθ+∫a+bπ−σcu(cos⁡θ−cos⁡a)R2tdθ=M/2 (22)

−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.25sin⁡2(a+b)−2sin⁡(a+b)cos⁡a+0.75sin⁡2a+0.5b+bcos2aβ(1−cos⁡a)+πcos⁡a+sin⁡(a+b)−(a+b)cos⁡aαp=M2σtR2t (23)

γ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.25sin⁡2(a+b)−2sin⁡(a+b)cos⁡a+0.75sin⁡2a+0.5b+bcos2aβ(1−cos⁡a)+πcos⁡a+sin⁡(a+b)−(a+b)cos⁡aαp (24)

M=2γσtR2t (25)

where the constant coefficient γ in Eq. (24) is related to a , b , and αp . When the bending moment M is given, the unknown variables a , b, and αp can be solved using Eqs. (17), (20), and (23). Two types of failures are considered in the ultimate state: Tensile and compressive failures. If the compressive failure occurs at the top surface of the section, εt=1−cos⁡a1+cos⁡aεcu will not exceed εtu . Substituting αp=σtm/σcu=Etmin(1−cos⁡a1+cos⁡aεcu,εtu)/σcu into Eqs. (17), (20), and (23) yields:

Mu=2γσtmR2t=min(1−cos⁡a1+cos⁡aεcu,εtu)2γEtR2t (26)

where Mu is the ultimate bending moment. When the bending moment is greater than Mp and smaller than Mu , the curvature can be calculated as follows:

kp=σtEt(1−cos⁡a)R=M2γ(1−cos⁡a)EtR3tMp≤M≤Mu (27)

2.3 Curvature of a Double-Pole Bamboo Beam

There are also two curvature stages for a double-pole bamboo beam. Owing to the cross-section containing two annuli, the elastic stage can be considered for two cases and the elastic-plastic stage for four. Two polar coordinate systems are separately established for the top and bottom bamboo poles. a and b determine the location of the neutral axis and the boundary of the elastic and plastic areas, as displayed below in six different diagrams (Figs. 4–9) illustrating the stress and strain distributions. The curvature analysis of a single-pole bamboo beam can be referenced for a double-pole bamboo beam. Therefore, the description of the curvature analysis process is not repeated but the main equations and results are presented as follows.

2.3.1 Elastic Stage

The location of the neutral axis differentiates the two cases. The neutral axis is located between the centerlines of the two annuli in Case 1; the neutral axis in Case 2 intersects the centerline of the bottom bamboo pole.

(1) Case 1:

Fig. 4 shows the stress and strain distributions in Case 1.

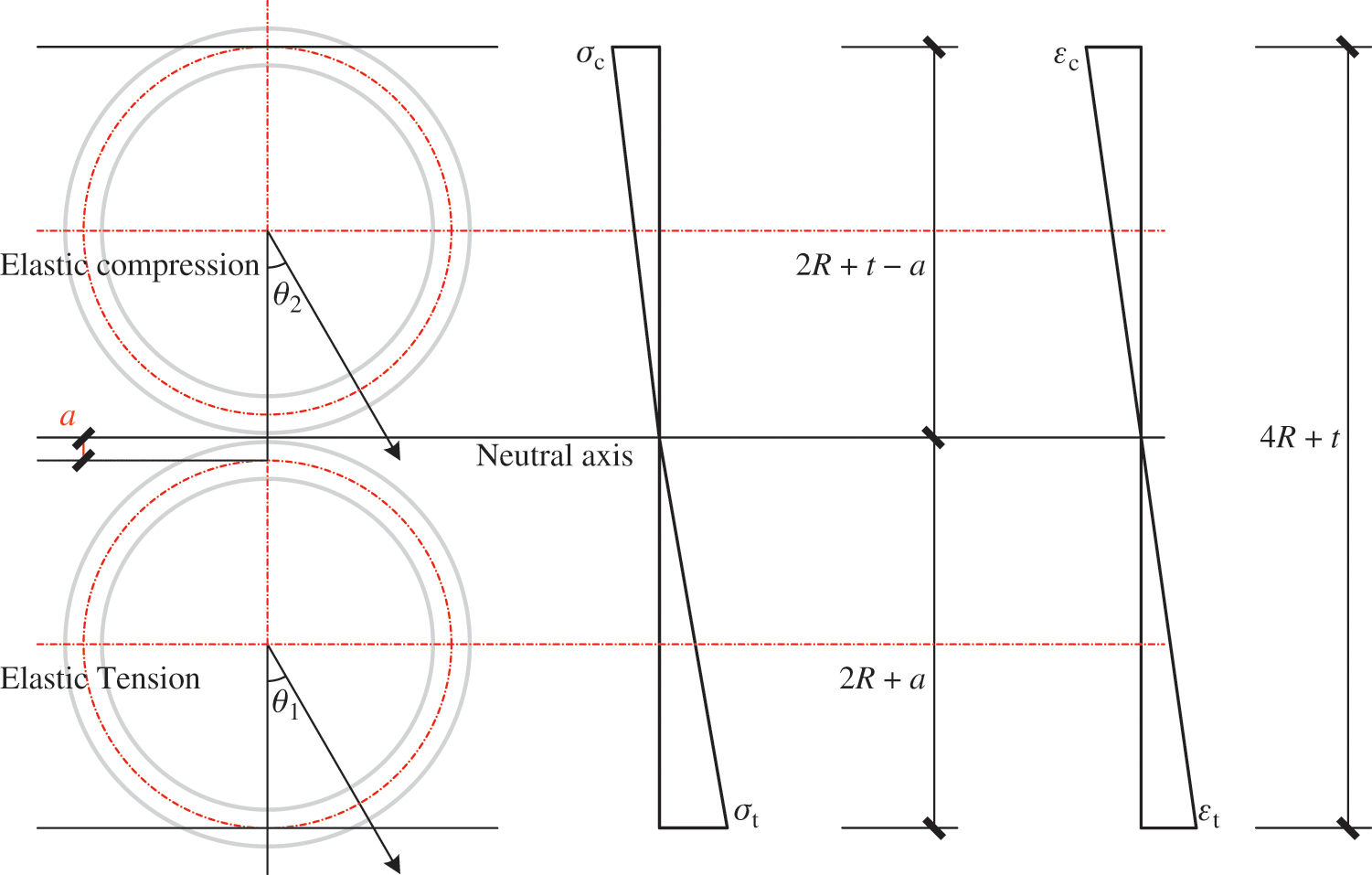
Figure 4: Stress and strain distributions in Case 1

Eqs. (28)–(32) express the curvature analysis of a double-pole bamboo beam in Case 1.

a=R(1−β)+t1+β0≤a≤0.5t (28)

λ=0.5πR2+π(R+a)22R2+aR+0.5πR2+π(R+t−a)2β(2R2+aR) (29)

εtεc=σtEcσcEt=αeβ=2R+a2R+t−a (30)

Mp=2λαeσcuR2t=2λβ2R+a2R+t−aσcuR2t (31)

ke=σtEt(2R+a)=M2λ(2R+a)EtR2tM≤Mp (32)

(2) Case 2

Fig. 5 shows the stress and strain distributions in Case 2.

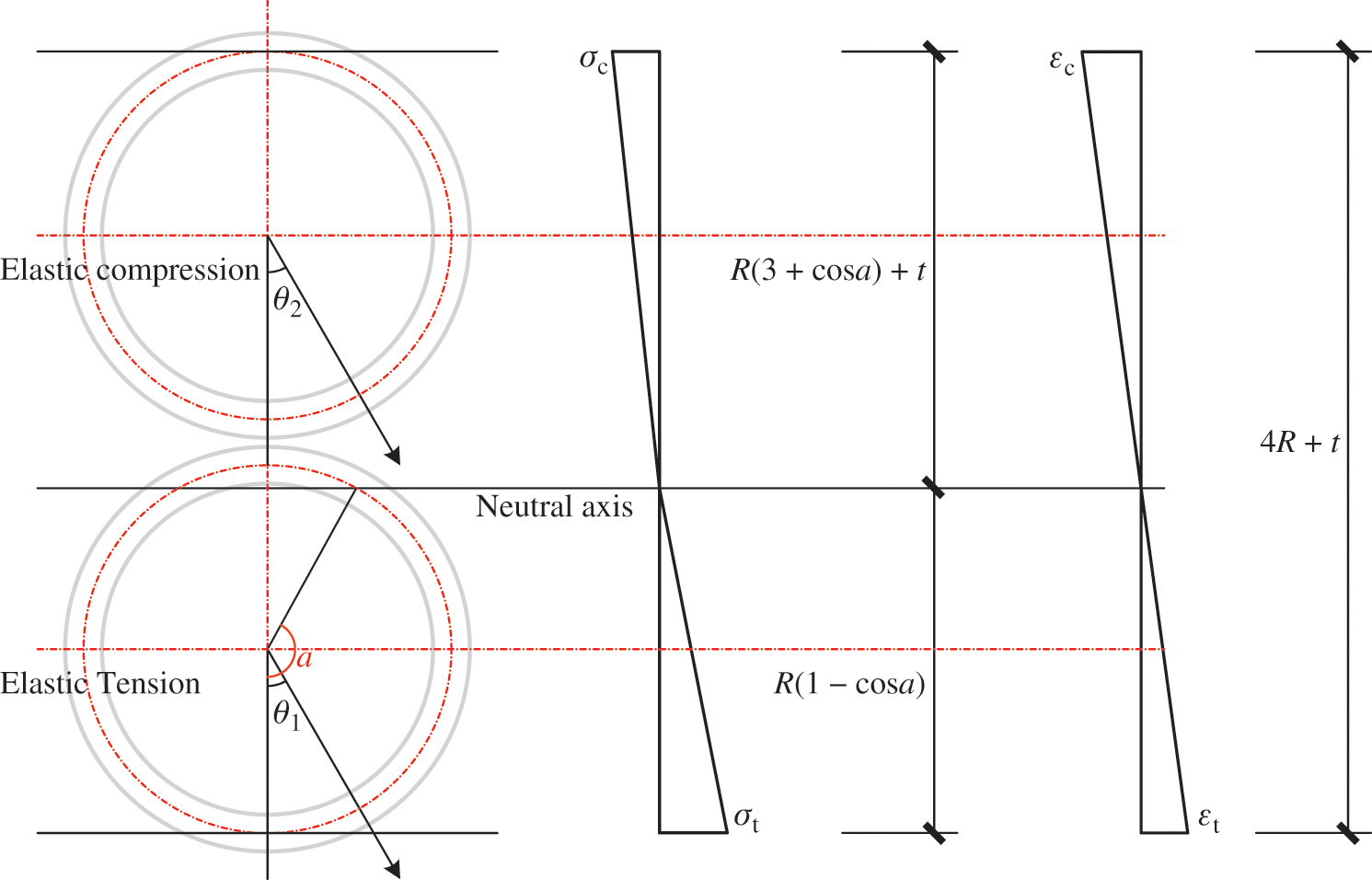
Figure 5: Stress and strain distributions in Case 2

Eqs. (33)–(37) express the curvature analysis of a double-pole bamboo beam in Case 2.

2πR(1+cos⁡a)+πtR(sin⁡a−acos⁡a)=β−1 (33)

λ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.5π+πcos2a+0.75sin⁡2a−0.5a−acos2aβ(1−cos⁡a)+0.5πR2+π(Rcos⁡a+2R+t)2βR2(1−cos⁡a) (34)

εtεc=σtEcσcEt=αeβ=R(1−cos⁡a)R(3+cos⁡a)+t (35)

Mp=2λαeσcuR2t=2λβR(1−cos⁡a)R(3+cos⁡a)+tσcuR2t (36)

ke=σtEt(1−cos⁡a)R=M2λ(1−cos⁡a)EtR3tM≤Mp (37)

2.3.2 Elastic-Plastic Stage

The neutral axis and boundary of the elastic and plastic areas differentiate the four cases.

(1) Case 3

Fig. 6 shows the stress and strain distributions in Case 3.

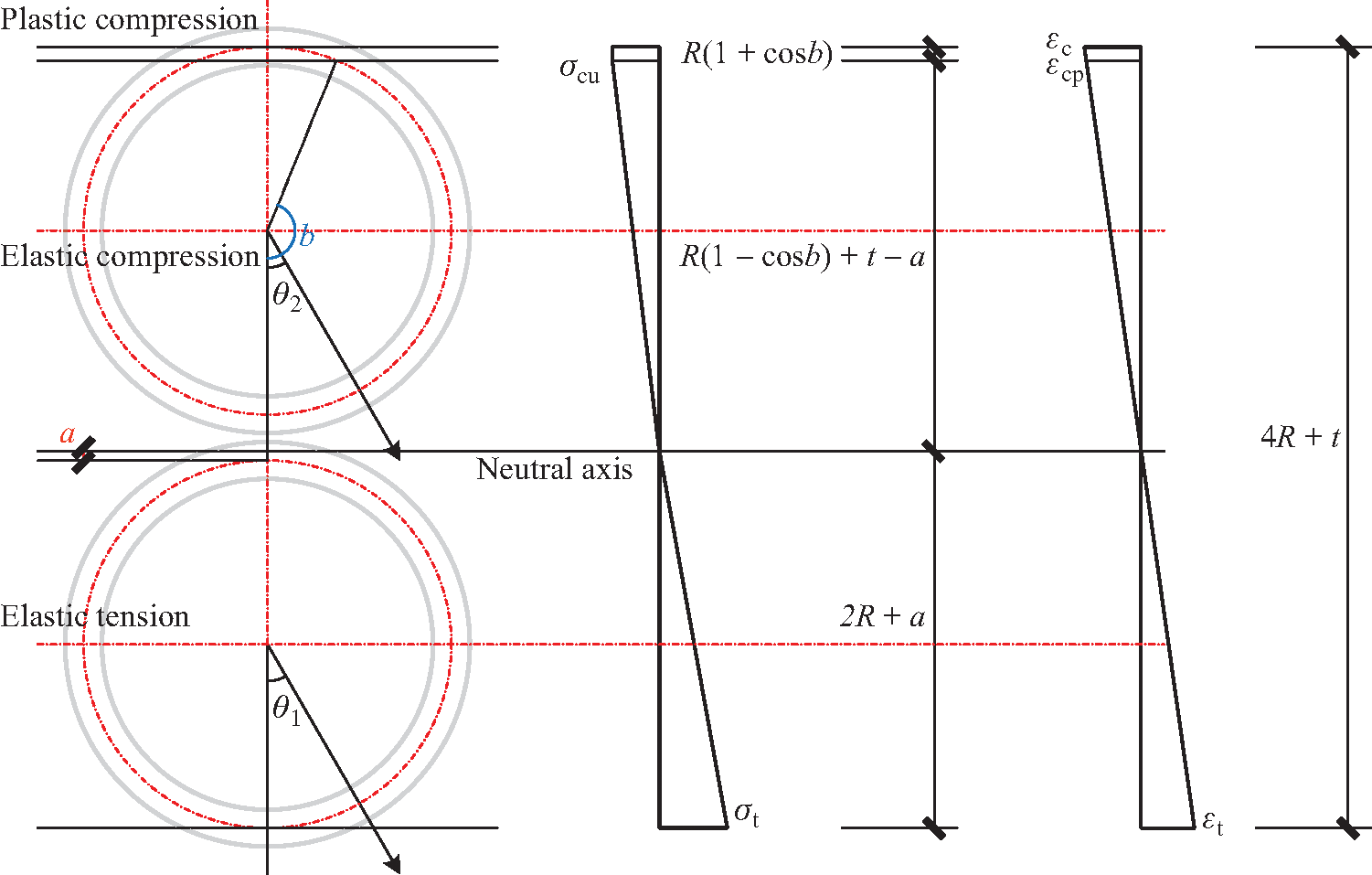
Figure 6: Stress and strain distributions in Case 3

Eqs. (38)–(43) express the curvature analysis of a double-pole bamboo beam in Case 3.

εtεcp=σtEcσcuEt=αpβ=2R+aR(1−cos⁡b)+t−a (38)

b=arccos⁡[1+t−aR−βαp(2+aR)] (39)

π(R+a)2R+a+Rsin⁡b−b(R+t−a)β(2R+a)−π−bαp=0 (40)

γ=0.5πR2+π(R+a)22R2+aR+0.25R2sin⁡2b+0.5bR2−2(R+t−a)Rsin⁡b+b(R+t−a)2β(2R2+aR)+(R+t−a)(π−b)+Rsin⁡bRαp (41)

Mu=2γσtmR2t=min(2R+t−a2R+aεcu,εtu)2γEtR2t (42)

kp=σtEt(2R+a)=M2γ(2R+a)EtR2t (43)

(2) Case 4

Fig. 7 displays the stress and strain distributions in Case 4.

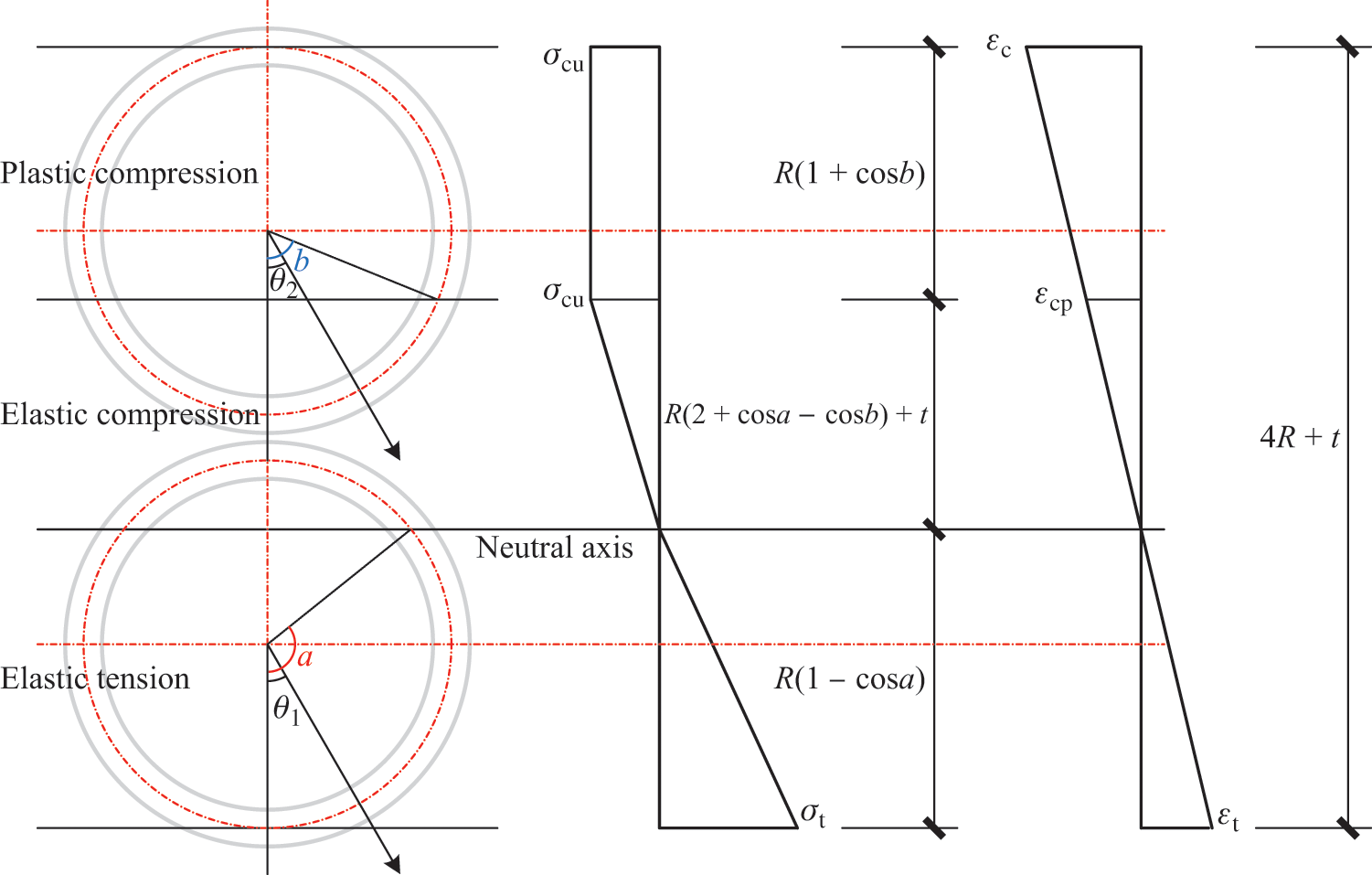
Figure 7: Stress and strain distributions in Case 4

Eqs. (44)–(49) express the curvature analysis of a double-pole bamboo beam in Case 4.

εtεcp=σtEcσcuEt=αpβ=R(1−cos⁡a)R(2+cos⁡a−cos⁡b)+t (44)

b=arccos⁡[2+cos⁡a+tR−βαp(1−cos⁡a)] (45)

sin⁡a−acos⁡a1−cos⁡a+−πcos⁡a−sin⁡a+acos⁡aβ(1−cos⁡a)+Rsin⁡b−b(Rcos⁡a+2R+t)βR(1−cos⁡a)−π−bαp=0 (46)

γ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.5π+πcos2a+0.75sin⁡2a−0.5a−acos2aβ(1−cos⁡a)+R2(0.25sin⁡2b+0.5b)−2R(Rcos⁡a+2R+t)sin⁡b+b(Rcos⁡a+2R+t)2βR2(1−cos⁡a)+(Rcos⁡a+2R+t)(π−b)+Rsin⁡bRαp (47)

Mu=2γσtmR2t=min(R(1−cos⁡a)R(3+cos⁡a)+tεcu,εtu)2γEtR2t (48)

kp=σtEt(1−cos⁡a)R=M2γ(1−cos⁡a)EtR3t (49)

(3) Case 5

Fig. 8 illustrates the stress and strain distributions in Case 5.

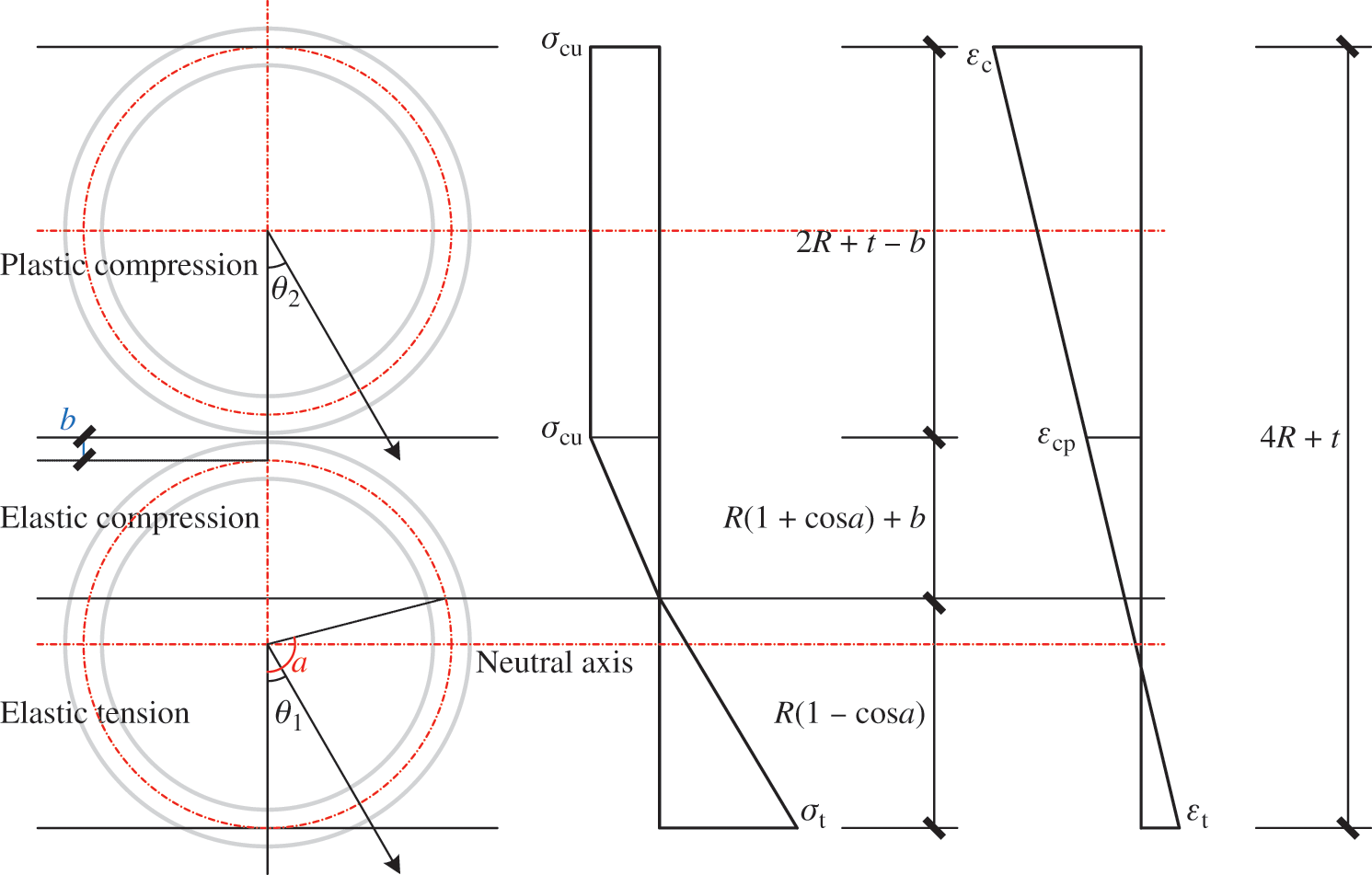
Figure 8: Stress and strain distributions in Case 5

Eqs. (50)–(55) express the curvature analysis of a double-pole bamboo beam in Case 5.

εtεcp=σtEcσcuEt=αpβ=R(1−cos⁡a)R(1+cos⁡a)+b (50)

b=βαpR(1−cos⁡a)−R(1+cos⁡a) (51)

sin⁡a−acos⁡a1−cos⁡a+−πcos⁡a−sin⁡a+acos⁡aβ(1−cos⁡a)−παp=0 (52)

γ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.5π+πcos2a+0.75sin⁡2a−0.5a−acos2aβ(1−cos⁡a)+π(Rcos⁡a+2R+t)Rαp (53)

Mu=2γσtmR2t=min(R(1−cos⁡a)R(3+cos⁡a)+tεcu,εtu)2γEtR2t (54)

kp=σtEt(1−cos⁡a)R=M2γ(1−cos⁡a)EtR3t (55)

(4) Case 6

Fig. 9 shows the stress and strain distributions in Case 6.

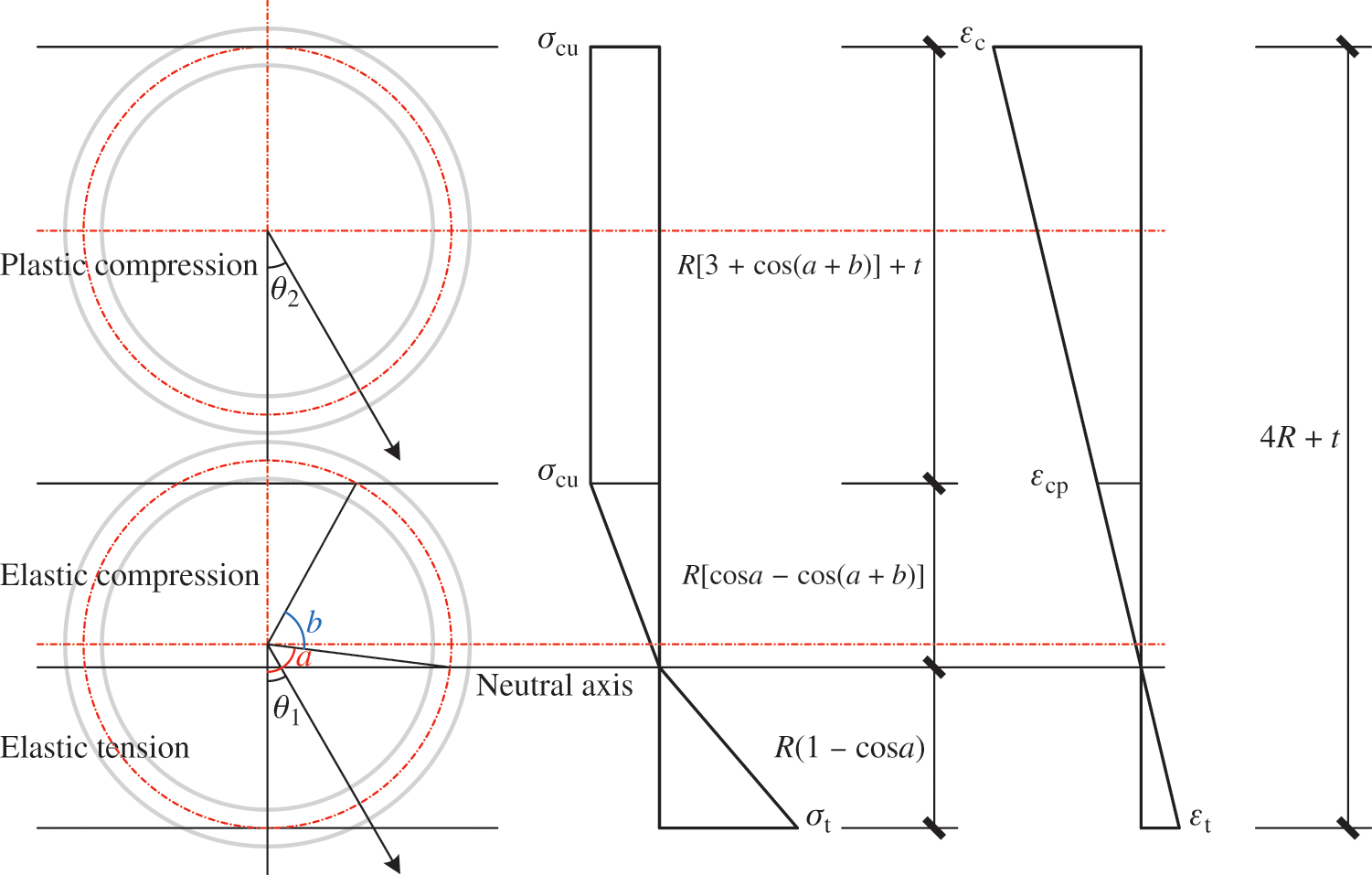
Figure 9: Stress and strain distributions in Case 6

Eqs. (56)–(61) express the curvature analysis of a double-pole bamboo beam in Case 6.

εtεcp=σtEcσcuEt=αpβ=1−cos⁡acos⁡a−cos⁡(a+b) (56)

b=arccos⁡[cos⁡a−βαp(1−cos⁡a)]−a (57)

sin⁡a−acos⁡a1−cos⁡a+sin⁡(a+b)−sin⁡a−bcos⁡aβ(1−cos⁡a)−2π−a−bαp=0 (58)

γ=−0.75sin⁡2a+0.5a+acos2a1−cos⁡a+0.25sin⁡2(a+b)−2sin⁡(a+b)cos⁡a+0.75sin⁡2a+0.5b+bcos2aβ(1−cos⁡a)+πcos⁡a+sin⁡(a+b)−(a+b)cos⁡aαp+π(Rcos⁡a+2R+t)Rαp (59)

Mu=2γσtmR2t=min(R(1−cos⁡a)R(3+cos⁡a)+tεcu,εtu)2γEtR2t (60)

kp=σtEt(1−cos⁡a)R=M2γ(1−cos⁡a)EtR3t (61)

2.4 Load-Deflection Curves

For a simply supported beam, the bending moment is easily plotted. Fig. 10a shows the bending moment diagram under an external load M(P,x) and Fig. 10b shows the bending moment diagram under the unit load M¯(x) . According to the relationship between the curvature and bending moment, the load-deflection curve Δ(P) at the unit load point can be calculated using the virtual work principle, as defined in Eq. (62).

Δ(P)=∫0LM¯(x)k[M(P,x)]dx (62)

where k[M(P,x)] refers to the relationship between the curvature and bending moment. The curvature k is a function of bending moment M .

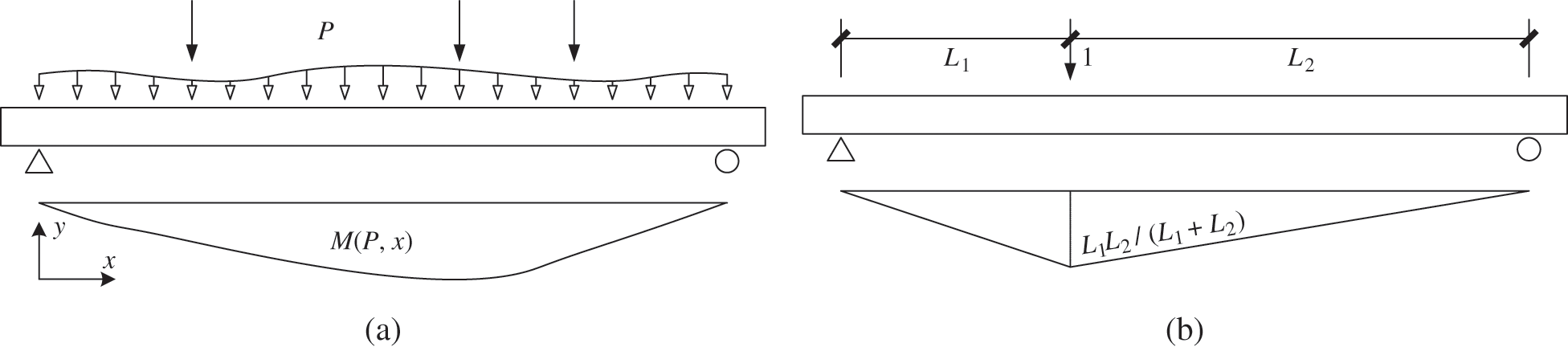
Figure 10: Bending moment diagram of external loads and unit load. (a) External load and (b) Unit load

Fig. 11 shows the calculation process of the load-deflection curves. There are three steps in the calculation. For a single-pole bamboo beam, 1) The maximum bending moment of the elastic stage Mp and the ultimate bending moment Mu should be solved. Two bending moments are adopted to differentiate the elastic stage, elastic-plastic stage, and failure stage. 2) When a bending moment M is given, the stage can be determined. Then, the curvature k is calculated for the corresponding stage. Based on the previous analysis, a function for calculating curvature k has been established, where the bending moment M is an independent variable. 3) Finally, the moment diagram M(P,x) , induced by external load P , is plotted. The load-deflection curve Δ(P) can be calculated using Eq. (62).

The elastic stage of a double-pole bamboo beam can be considered for two cases and the elastic-plastic stage for four. For this reason, the first two steps for a double-pole bamboo beam are more complicated. However, the load-deflection curve calculations are the same as for a single-pole bamboo beam. The critical bending moment Mi is the maximum bending moment of case i in the elastic-plastic stage. It is utilized to differentiate four cases in the elastic-plastic stage. The solution for Mp contains two types of conditions that correspond to two cases of the elastic stage. Failure in the elastic-plastic stage may occur in one of four cases; thus, the solution of Mu includes four types of conditions.

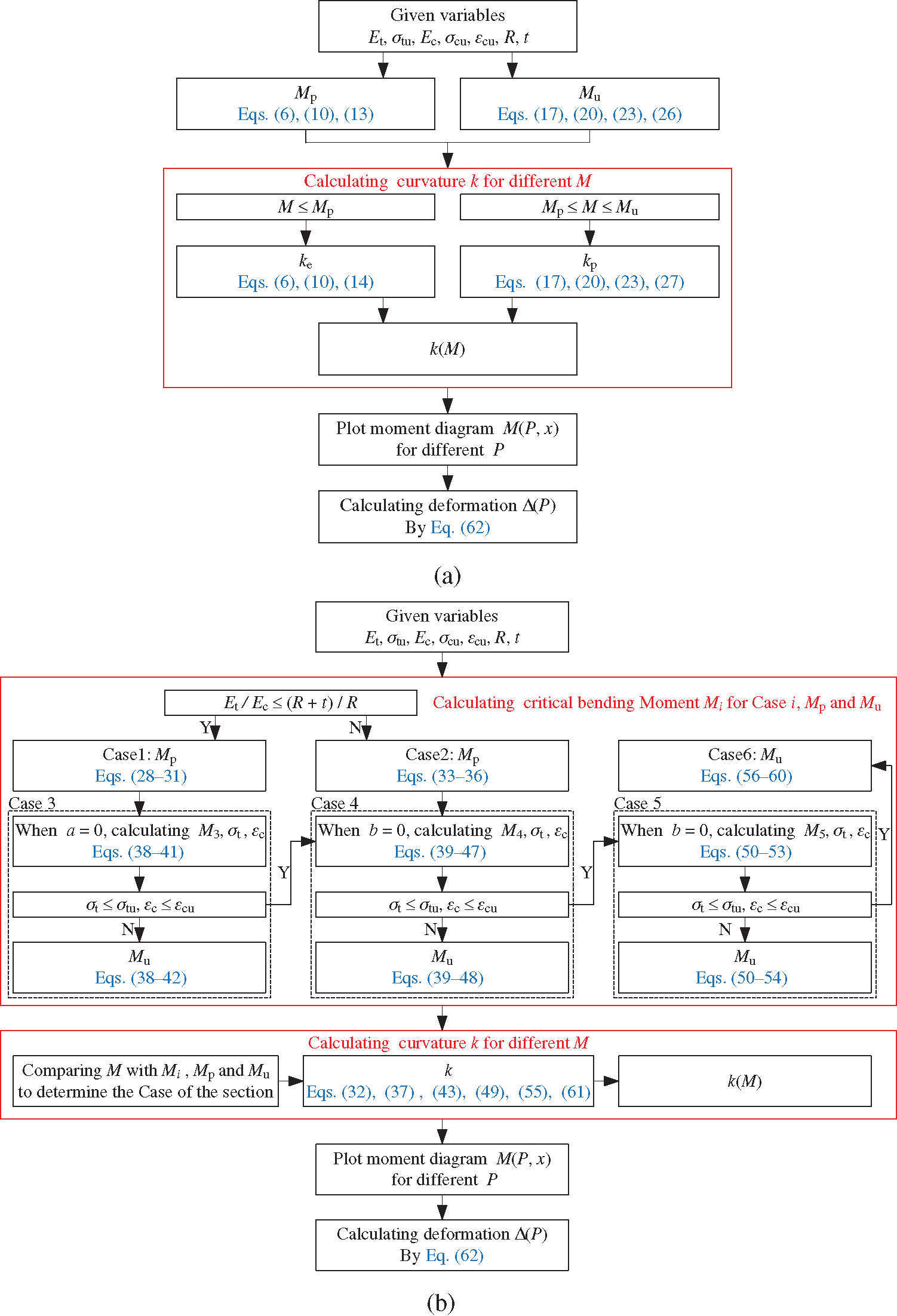
Figure 11: Calculation process of load-deflection curves. (a) Single-pole bamboo beam and (b) Double-pole bamboo beam

2.5 Influence of Interfacial Slippage

The interfacial slippage between two bamboo poles is difficult to avoid; this has not been previously considered in double-pole bamboo beam analyses. The influence of interfacial slippage is analyzed based on the following assumptions: 1) The bamboo materials are linearly elastic. 2) The shear force at the interface is proportional to the slippage. 3) The curvatures and deflections of the two bamboo poles are consistent. 4) The shear deformation is not considered when the plane-section assumption is satisfied.

Fig. 12 shows an interfacial slippage diagram. The equilibrium differential equation, based on a tiny segment, is presented in Eq. (63). When the boundary conditions are imported, the interfacial slippage u can be solved. For simply supported and free ends, u′=0 ; for a fixed end and symmetric section, u=0 . Then, the extra curvature ks caused by the interfacial slippage can be calculated using Eq. (66). The extra curvature ks is a function of the vertical shear force V . Ultimately, Eq. (67) provides the method for solving the extra deflection Δs(P) caused by interfacial slippage.

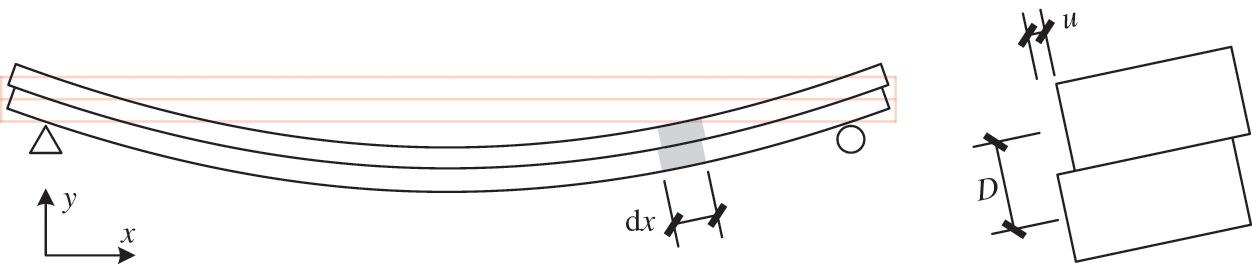
Figure 12: Interfacial slippage diagram

u′′−α2u=VD/EI0 (63)

α2=KD2EI0(1−EI0/EI∞)D=2R+t (64)

EI∞=EI0+EAD2EI0=E1I1+E2I21/EA=1/E1A1+1/E2A2 (65)

ks=(1−EI0/EI∞)u′/D (66)

Δs(P)=∫0LM¯ks[V(P,x)]dx (67)

where D represents the distance of the horizontal symmetry axes in the two bamboo poles. K is the shear stiffness of the interface per unit length. For simplification, the difference in the elastic modulus is not considered in the tensile and compressive states. The influence of not considering the difference can be ignored, which will be demonstrated in the following analysis. E1 and E2 are the elastic moduli of the top and bottom bamboo poles, respectively, i.e., the mean value of Et and Ec . I1 and I2 represent the moments of inertia and A1 and A2 represent the sectional areas.

3  FE Analysis of Bamboo Beams

3.1 Single-Pole Bamboo Beam

FE analysis was adopted to validate the above analysis. Fig. 13a manifests the two-point loading pattern that is recommended for bending tests on bamboo [1]. This two-point loading pattern was adopted to conduct a case study of bamboo beams. The bending moment diagram of two-point loading is similar to that of a uniform load. To calculate the midspan deflection, Fig. 13(b) displays the bending moment diagram of the midspan unit load.

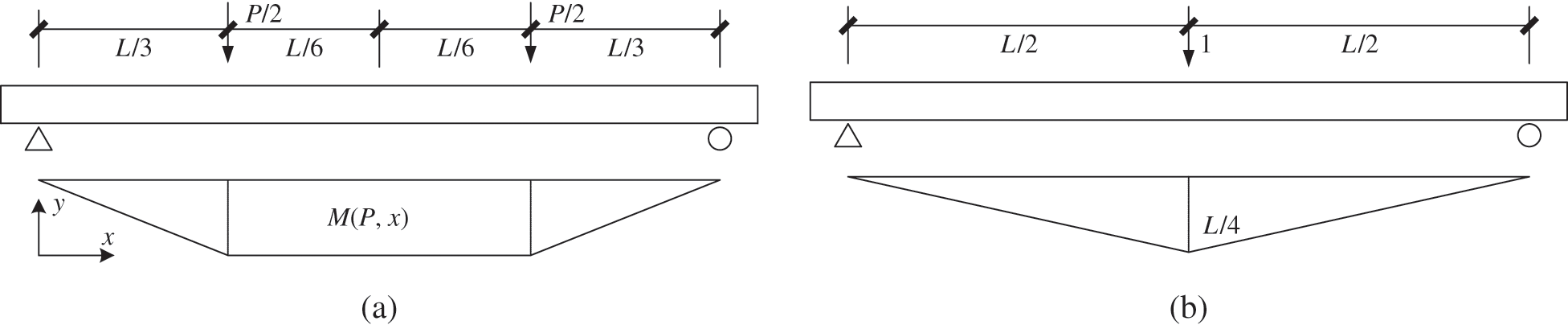
Figure 13: Bending moment diagram of two-point loading and midspan unit load. (a) Two-point loading and (b) Midspan unit load

Fig. 14 presents the FE model of a single-pole bamboo beam, using the method presented in the literature [38]. The span was 3 m and the section size was Φ100 × 8 mm. Coupling was used at two loading points and two support points. The solid element C3D8R was employed. The overall mesh size was 16 mm while the mesh size in the thickness direction was 4 mm. The properties of the bamboo are listed in Tab. 1. The different elastic moduli in the tensile and compressive states could not be simulated in the FE model. Therefore, the mean values of Et and Ec were applied. Based on the original model, two additional FE models that consider the taper and bamboo joints were developed. The taper was 0.6% and the thickness of the bamboo joints was 8 mm. The properties of the joints are consistent with those of bamboo. The joints were tied to the internal surface at intervals of 300 mm. In addition, single-pole bamboo beams with spans of 4.5 m and 6 m were simultaneously analyzed.

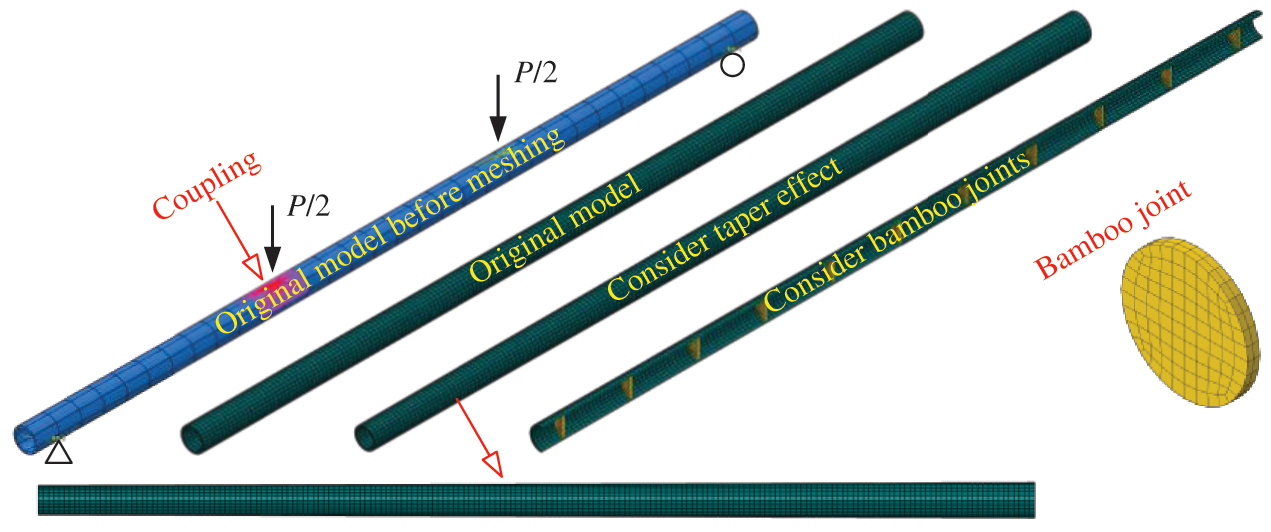
Figure 14: FE model of a single-pole bamboo beam

Table 1: Properties of the bamboo [23]

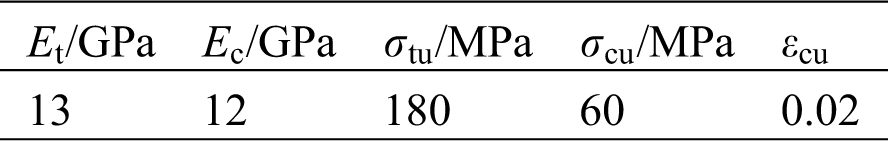
Fig. 15 compares the theoretical and FE results for a single-pole bamboo beam. When the difference in the elastic modulus is not considered, the load-deflection curves can be calculated from theoretical analysis (the ‘Theory-Simplify’ curves in Fig. 15). These match well with the theoretical load-deflection curves when the difference is considered. Consequently, the mean values of Et and Ec are utilized in the FE analysis.

During the initial stage, the FE results are consistent with the theoretical results. However, there are some discrepancies in the large deformation stage. The load-deflection curves (referred to as FE-Linear because the FE model is analyzed without considering geometric nonlinearities) almost coincide with the theoretical results, indicating that the discrepancy is caused by geometric nonlinearity. The effect of tapering and joints along the length of the bamboo beam is limited. Considering these two factors improves the similarity between the load-deflection curves and original model.

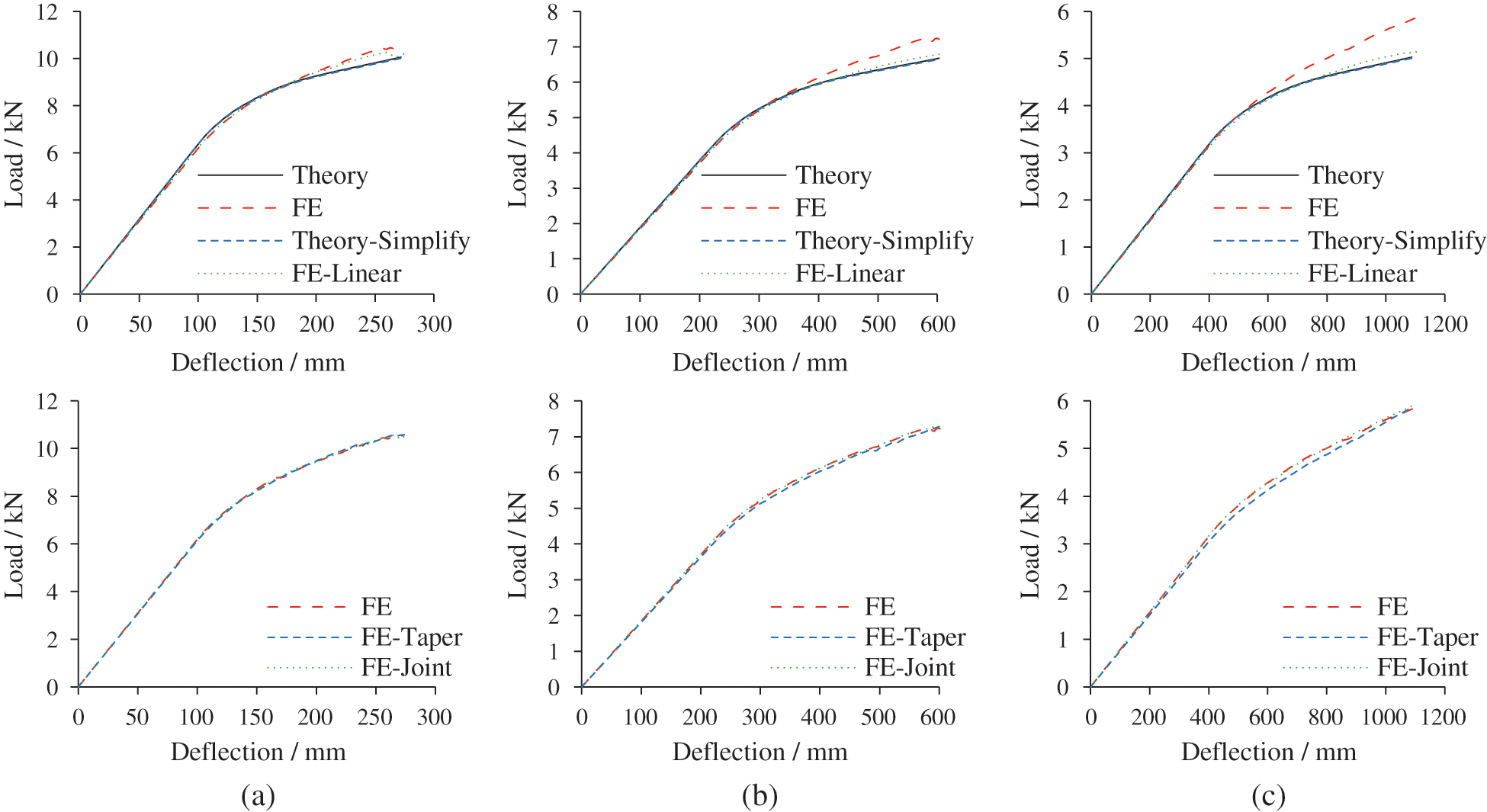
Figure 15: Comparison of theoretical and FE results for single-pole bamboo beams. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

Fig. 16 shows the ultimate state of single-pole bamboo beams with a span of 3 m. The neutral axis moves downward due to the greater tensile strength. When the geometric nonlinearity is not considered, the horizontal displacement of the support points is negligible. After considering the taper, the deformation is asymmetric and the maximum stress occurs on the left side with a smaller section size. The stress and deformation distributions when considering the bamboo joint are the same as those of the original model.

Figure 16: Ultimate state of single-pole bamboo beams (L = 3 m). (a) Stress distribution (Unit: MPa) and (b) Deformation distribution (Unit: mm)

3.2 Double-Pole Bamboo Beam without Interfacial Slippage

Fig. 17 depicts the FE model of a double-pole bamboo beam. The modeling strategy was identical to that of a single-pole bamboo beam. The axial connector was adopted to simulate the tangential interaction of the top and bottom bamboo poles. The normal interaction was determined as a hard contact. Two bamboo poles were evenly divided into thirty sections along the axis and thirty connectors were installed. For different spans, each connector joined different beam lengths. When the spans were 3 m, 4.5 m, and 6 m, the connected lengths l were 100 mm, 150 mm, and 200 mm, respectively. Coupling was used to link the connector and corresponding parts. Two bamboo poles in the FE model were placed in opposite directions to negate the poles’ taper. The bamboo joints in the top and bottom bamboo poles were evenly distributed along the axis. To investigate the flexural behavior of a double-pole bamboo beam without slippage, the stiffness of the axis connectors was set as infinite.

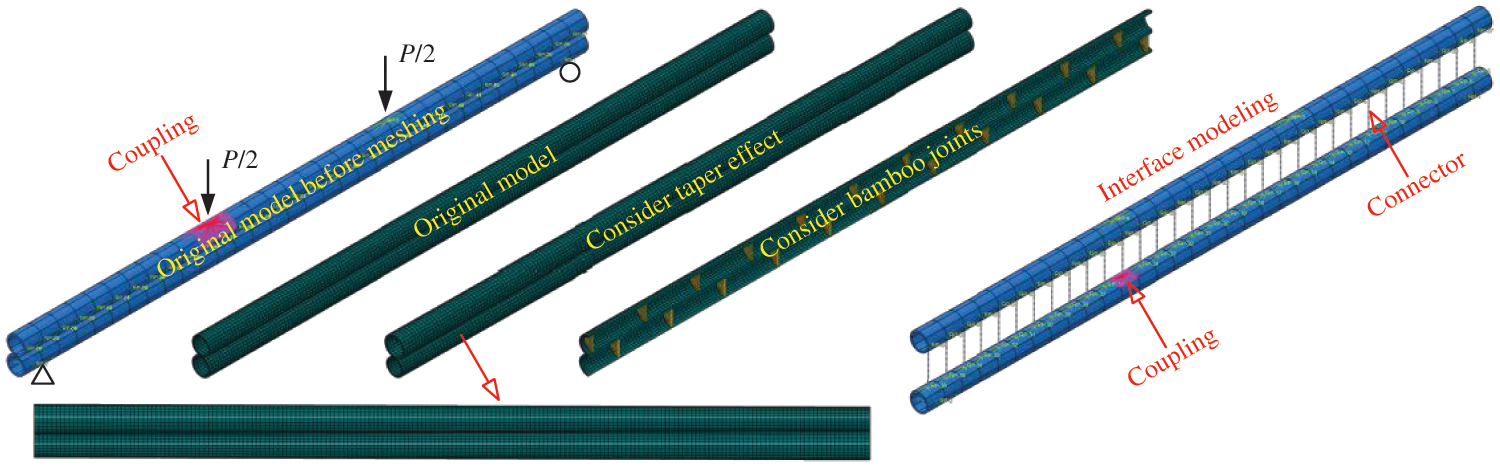
Figure 17: FE model of a double-pole bamboo beam

Fig. 18 compares the theoretical and FE results for a double-pole bamboo beam. The FE results match well with the theoretical results Eqs. (63)–(67). The deformation capacity of single-pole bamboo beams is twice that of double-pole bamboo beams. Therefore, the influence of geometric nonlinearity is not obvious in double-pole bamboo beams. Owing to the reverse placement of two poles, the influence of the taper is reduced.

Figure 18: Comparison of theoretical and FE results for double-pole bamboo beams. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

Fig. 19 displays the ultimate state of double-pole bamboo beams with a span of 3 m. Interfacial slippage is not observed in the four FE models and the neutral axis moves downward. The horizontal displacement of the support points is small and the influence of geometric nonlinearity is restricted. The stress and deformation distributions also illustrate that the taper has little effect on the flexural behavior of double-pole bamboo beams.

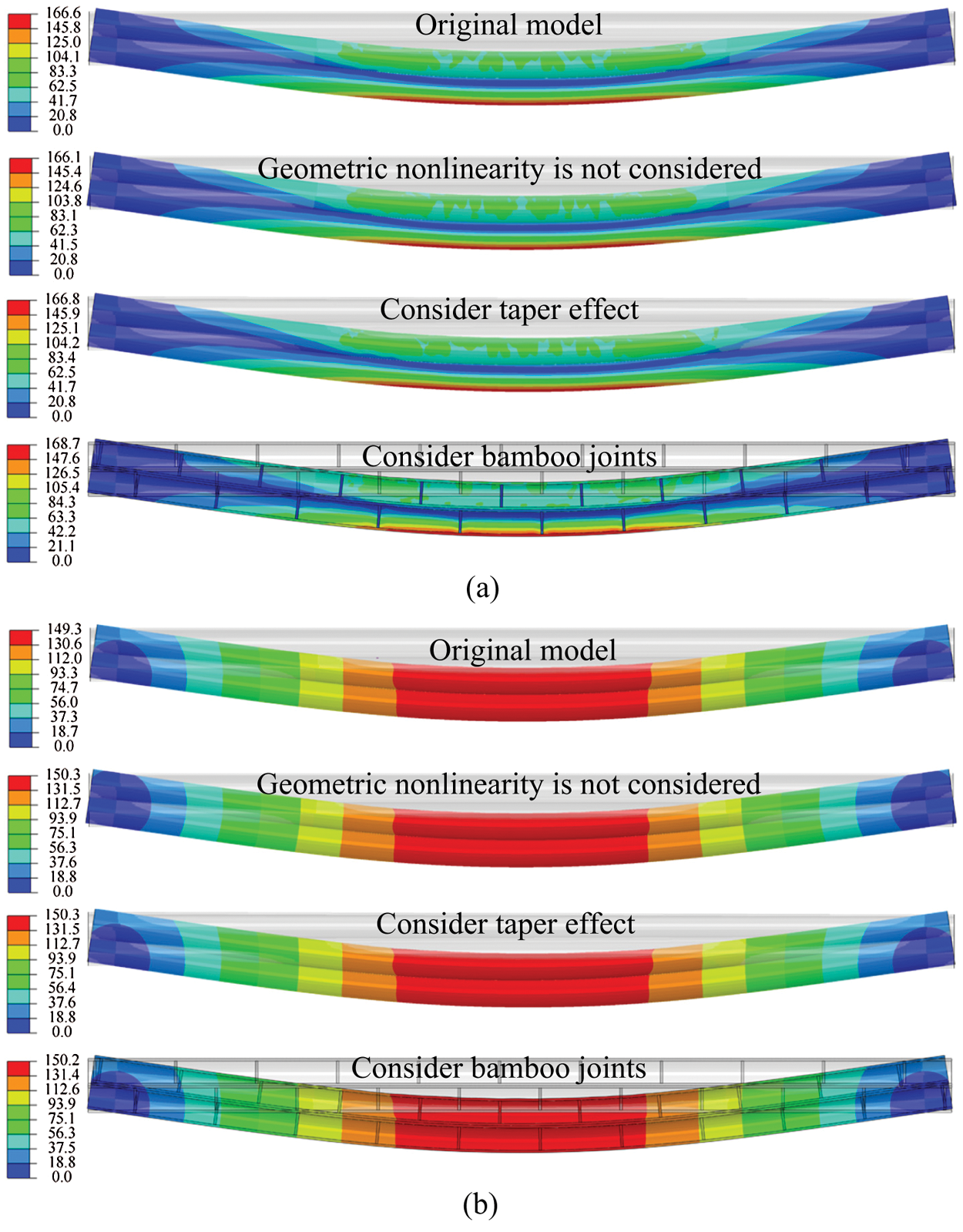
Figure 19: Ultimate state of double-pole bamboo beams (L = 3 m). (a) Stress distribution (Unit: MPa) and (b) Deformation distribution (Unit: mm)

3.3 Double-Pole Bamboo Beam with Interfacial Slippage

The theoretical analysis of the interfacial slippage is only suitable for the elastic stage [39–41]. The FE analysis can compensate for this. Four FE models, with different connector stiffnesses S , were established. The individual stiffnesses were set as 6.4 kN/mm, 1.6 kN/mm, 0.4 kN/mm, and 0 kN/mm. Fig. 20 depicts the load-deflection curves with interfacial slippage. The FE results agree well with the theoretical results in the elastic stage. The connector stiffness S has a significant influence on the flexural behavior of double-pole bamboo beams. When the stiffness is 6.4 kN/mm, the load-deflection curve is close to that when slippage is not considered. The shear stiffness of the interface per unit length K is the ratio of the connector stiffness S to the connected length l (Eq. (68)). Thus, the K requirement decreases with increasing span.

K=S/l (68)

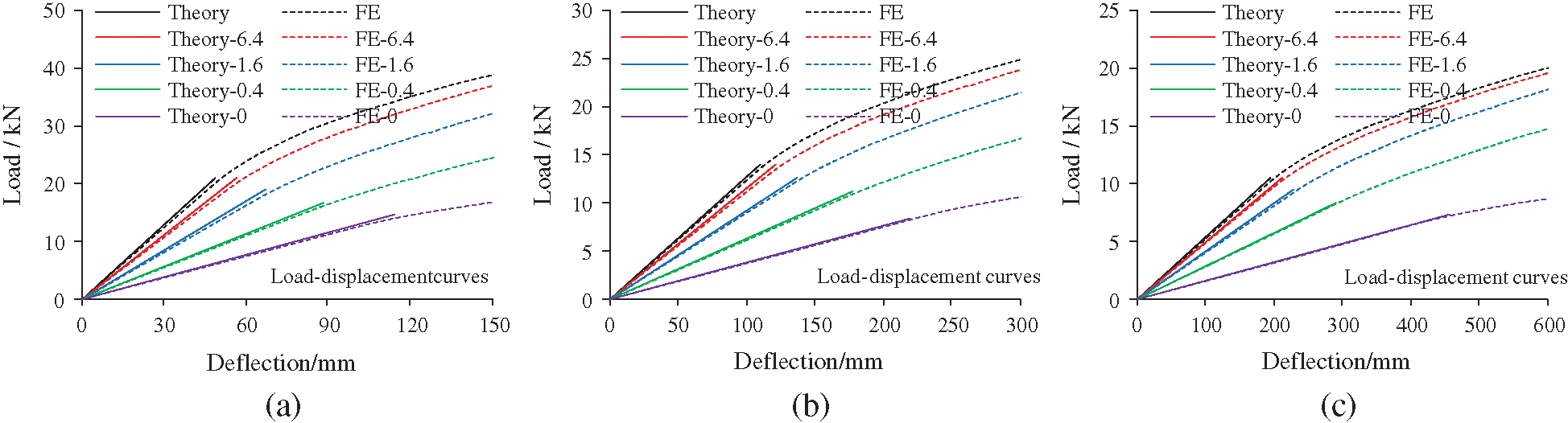
Figure 20: Load-deflection curves with interfacial slippage. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

Fig. 21 compares the slippage distribution of double-pole bamboo beams in the elastic stage. The theoretical analysis of interfacial slippage proves to be correct and reasonable. The slippage is concentrated at the beam ends. With increasing connector stiffness, the interfacial slippage gradually decreases. Fig. 22 shows the interfacial shear force of the double-pole bamboo beams when the connector stiffness is 6.4 kN/mm. The interfacial shear force is equal to the axial force on the connectors. Considering symmetry, only the axial force of the fifteen connectors on the left side of the bamboo beam are presented. The connectors are numbered from left to right. Connector 1 is near the left support point and the axial force there is the greatest. Fig. 23 displays the ultimate states of the double-pole bamboo beams when the connector stiffness is 0 kN/mm. Two neutral axes are observed in the top and bottom bamboo poles. The stress distribution is similar to that of a single-pole bamboo beam. Significant interfacial slippages are observed at both ends of the double-pole bamboo beams.

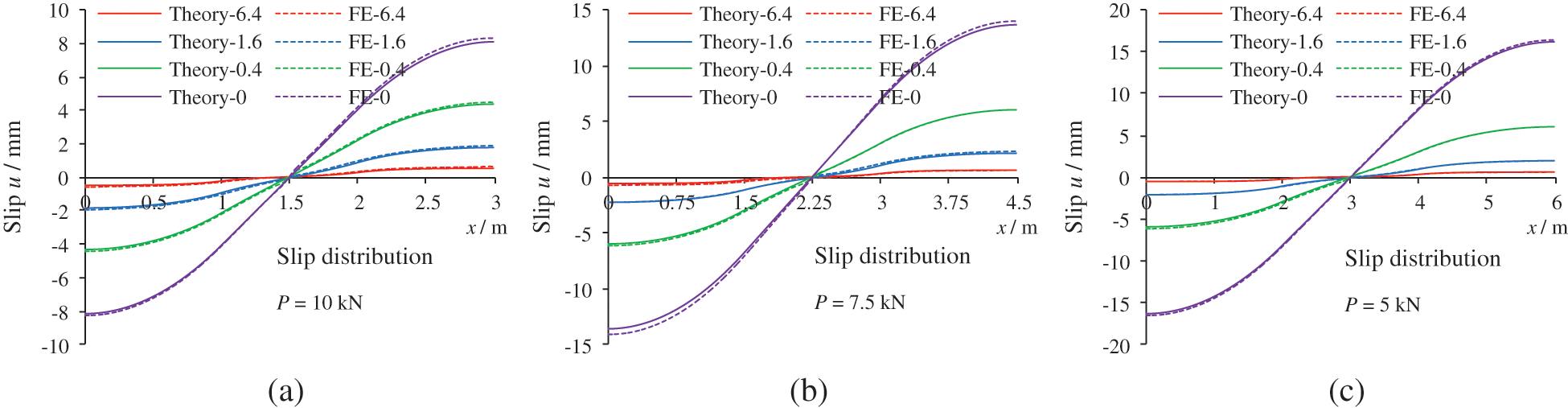
Figure 21: Slippage distribution of the double-pole bamboo beams. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

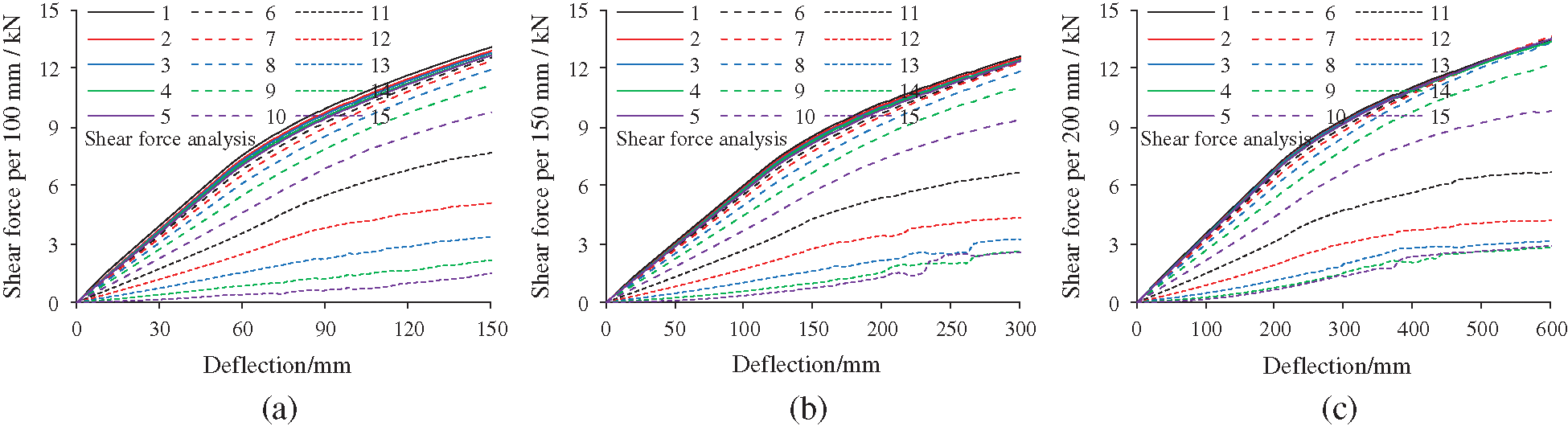
Figure 22: Shear force analysis of the double-pole bamboo beams. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

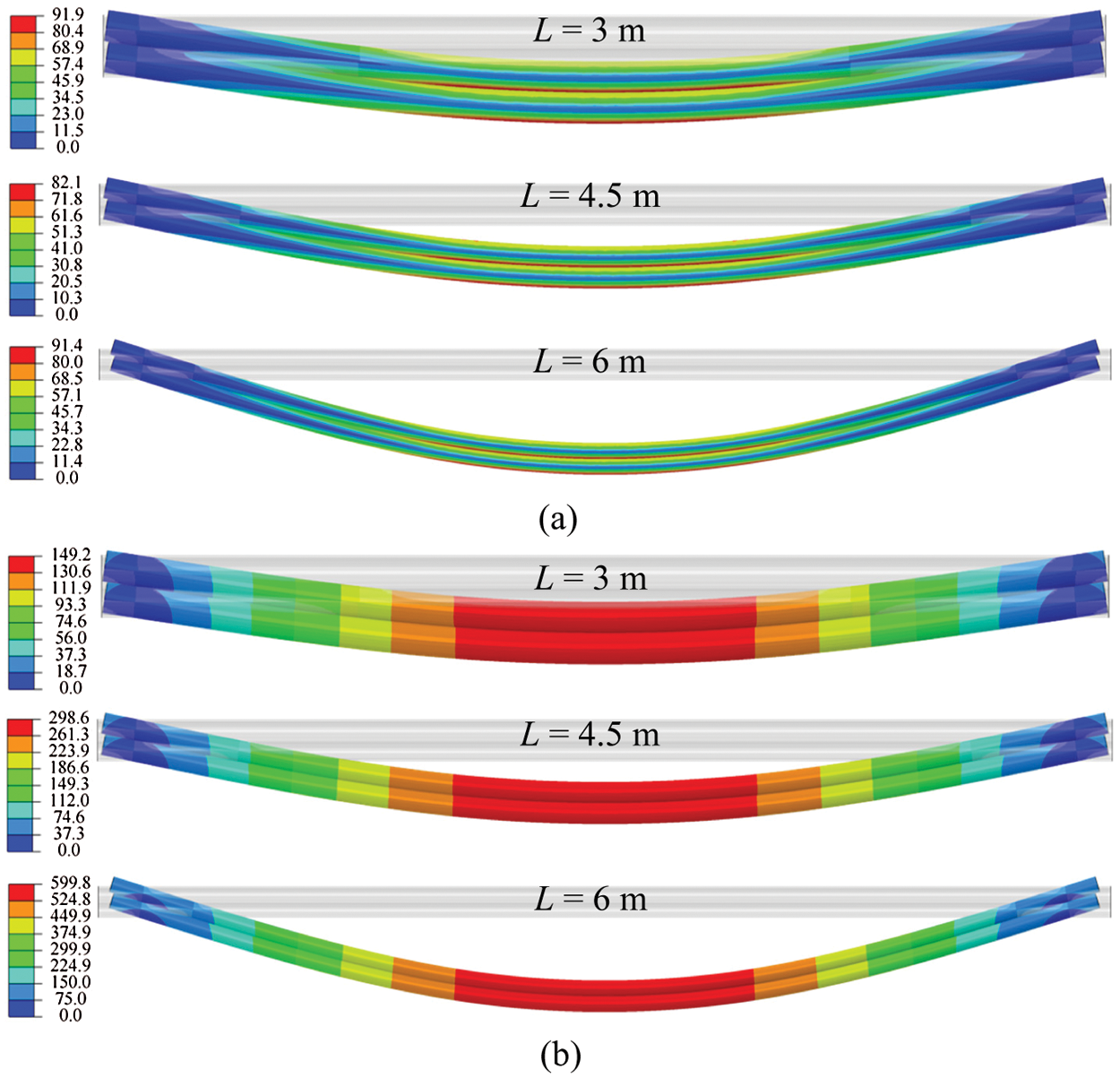
Figure 23: Ultimate state of double-pole bamboo beams with interfacial slippage. (a) Stress distribution (Unit: MPa) and (b) Deformation distribution (Unit: mm)

3.4 Novel Configuration to Avoid Slippage

Fig. 24 proposes a novel configuration to avoid slippage. Two bamboo poles are bound together using diagonal steel bands. The inclination of the steel band conforms to the direction of slippage. Owing to the diagonal arrangement, the tension stiffness of the steel bands is converted to horizontal shear stiffness between the two bamboo poles. The top and bottom areas of the steel band compressing the bamboo poles should be firmly fixed to the poles. Structural adhesives and pneumatic nails are two recommended methods. The methods should not damage the poles [24,25].

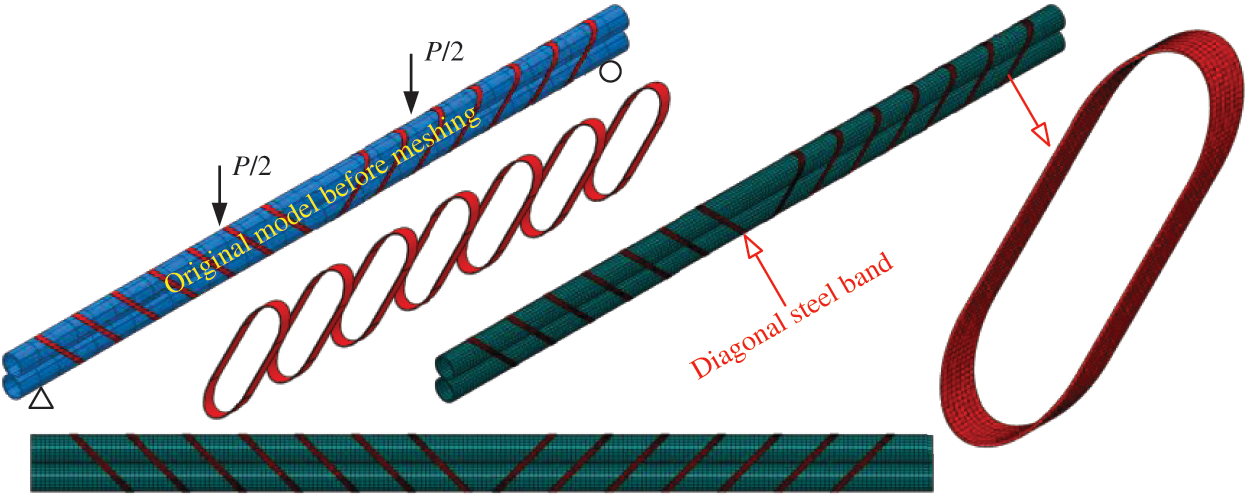
Figure 24: FE model of a double-pole bamboo beam with diagonal steel bands (L = 3 m)

The diagonal steel bands should meet the two requirements of stiffness and strength. Fig. 25 shows the stiffness of a single diagonal steel band S , which can be calculated using Eq. (69). The ultimate bearing capacity of the steel band Fu is also deduced using Eq. (70). Moreover, the quantity of steel in the diagonal area m is an important economic consideration, as shown in Eq. (71), since it contributes to the cost of these measures.

S=F/δ=2Ebwtbcos2θbsin⁡θb/D (69)

Fu=2fywtbcos⁡θb (70)

m=2wtbD/sin⁡θb (71)

where Eb and fy are the elastic modulus and yield stress of the steel band, respectively. w and tb represent the width and thickness of the band, respectively. θb refers to the inclination angle.

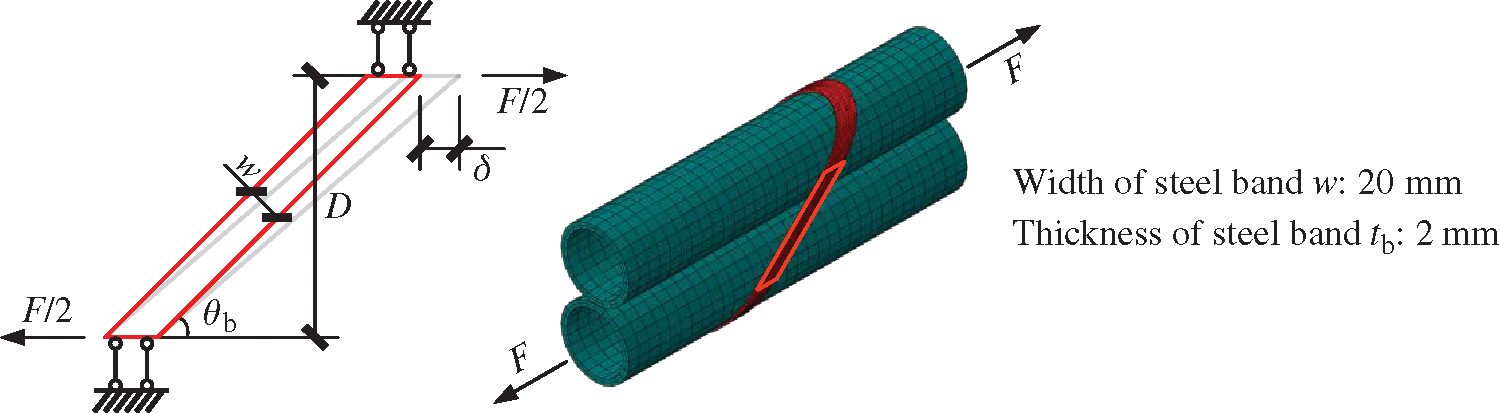
Figure 25: Mechanical diagram and FE model of a single diagonal steel band

The steel band cannot be sufficiently broad for the shear deformation to cause uneven stretching. Thus, a steel band width of 20 mm is suggested. The thickness of 2 mm is decided to facilitate fabrication. An ideal elastic-plastic constitutive model is adopted for the following analysis and the yield stress of the steel band is 235 MPa. Fig. 26 shows the results of the theoretical analysis. The ratio of S to m and the ratio of Fu to m are greatest when the inclination angle θb is 45°. Thus, it is cost-effective for θb to be 45°. At this moment, the stiffness of a single diagonal steel band S is 58.3 kN/mm and the ultimate bearing capacity Fu is 13.3 kN. Fig. 27 presents the FE results of a single diagonal steel band, which agree well with those of the theoretical analysis.

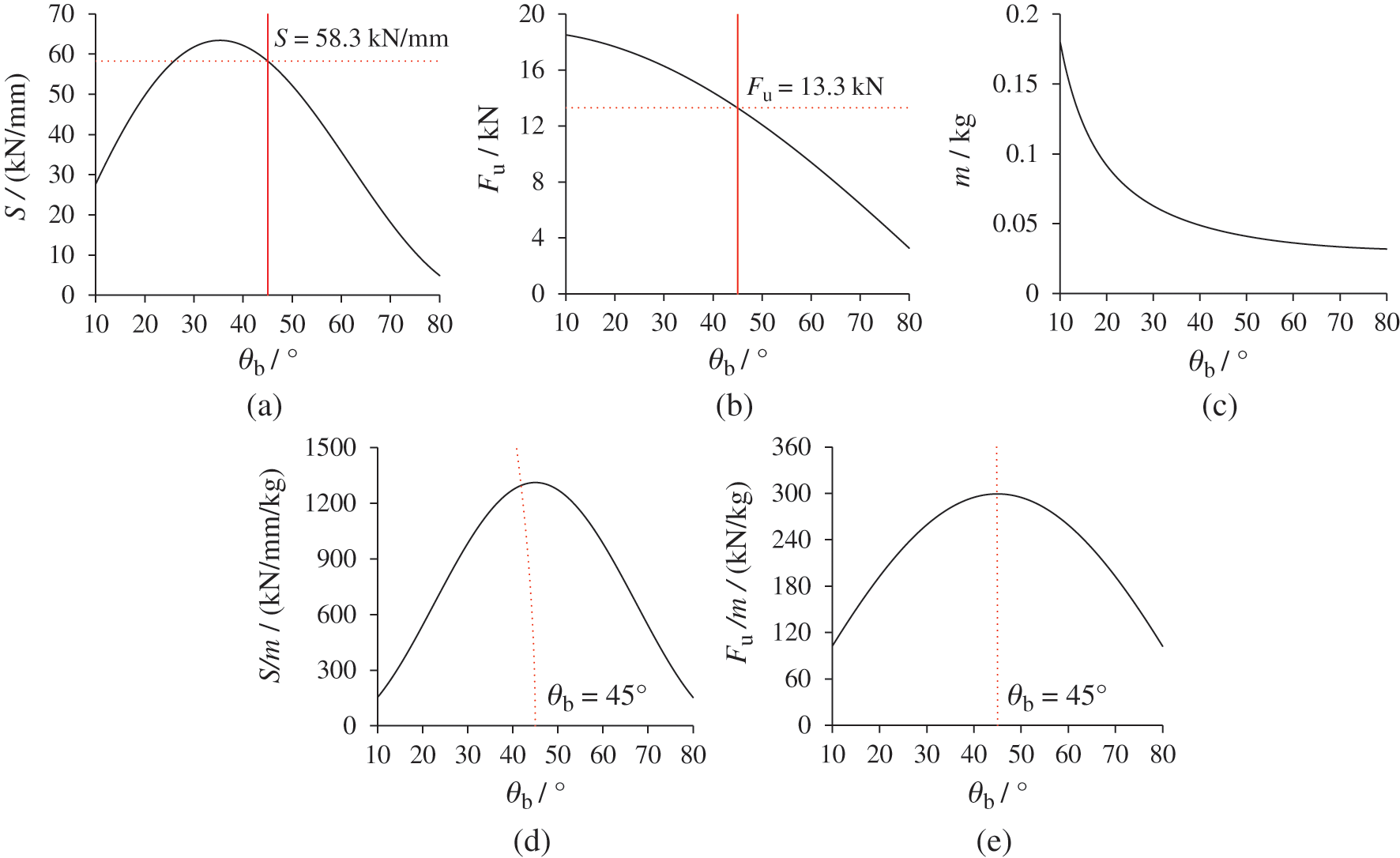
Figure 26: Results of theoretical analysis for a single diagonal steel band. (a) S – θb, (b) Fu – θb, (c) m – θb, (d) S/m – θb and (e) Fu/m – θb

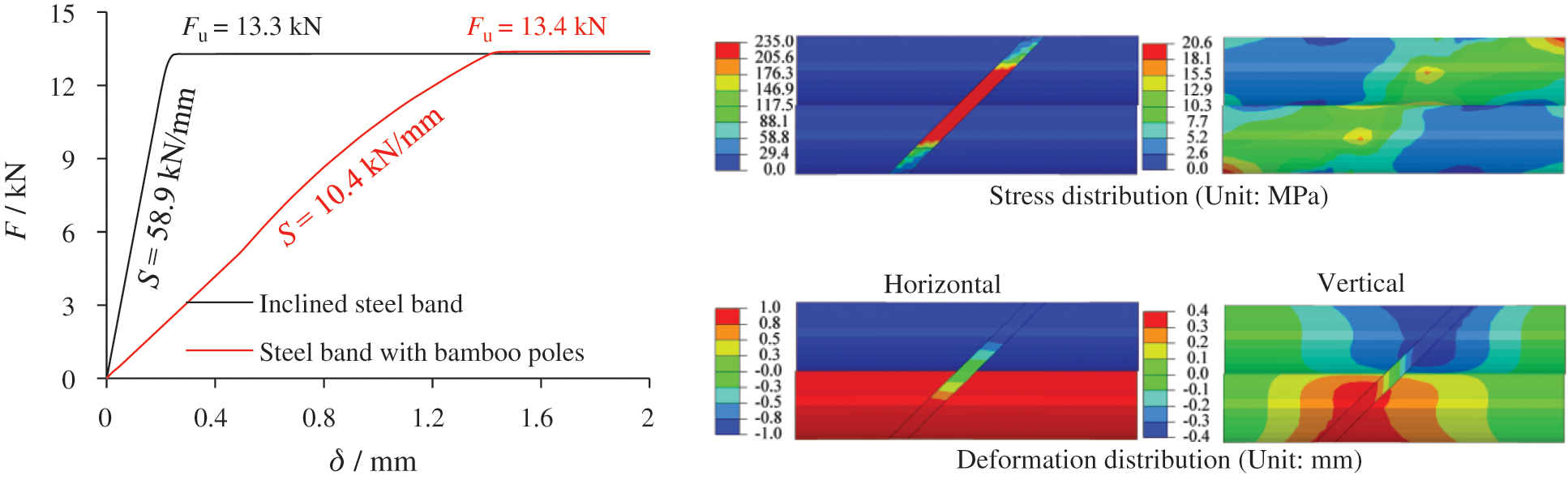
Figure 27: FE results of a single diagonal steel band

The steel band combined with bamboo is also simulated. The FE model is illustrated in Fig. 25. The length of the bamboo sample is 500 mm, which is sufficient to analyze the stiffness of the diagonal steel band in working conditions. The steel band is attached to the top and bottom surfaces of the bamboo. A shell element, S4R, with a mesh size of 4 mm, is implemented for the steel band. Fig. 27 presents the FE results. The stiffness S decreases to 10.4 kN/mm due to the bamboo’s deformation. The ultimate bearing capacity Fu is slightly affected and remains almost unchanged. These are the application parameters for diagonal steel bands.

Based on the model FE-0, a double-pole bamboo beam with diagonal steel bands is assembled, as shown in Fig. 24. The spacing of the diagonal steel bands is equivalent to the connected length l , which is set to 200 mm in Fig. 24. The modeling strategy for the steel bands is identical to that in Fig. 25. Meanwhile, the FE models with different spacings are simulated, presented in Tab. 2. The spacing enlarges as the span increases. Fig. 28 compares the load-deflection curves. Note that the ultimate load and displacement are smaller than those of the FE model without steel bands. Fig. 29 shows the ultimate state of the model with steel bands, demonstrating that the steel band yielded completely and is not fastened to the bamboo poles.

When the span is 3 m and the spacing is 100 mm, the interfacial slippage can be restrained. Its load-deflection curve is consistent with that of the FE model without slippage. The shear stiffness of the interface per unit length K is 104 N/mm2, which is greater than that of FE-6.4. As shown in Fig. 22, it is sufficient that the ultimate bearing capacity of the steel band Fu is 13.4 kN. To reduce costs, the spacing should be increased to 200 mm. The initial slope of the load-deflection curve only reduces by 13.9%. A spacing of 200 mm is recommended when the span is 3 m. In this case, K is 52 N/mm2, which is close to that of FE-6.4.

Table 2: Details of a double-pole bamboo beam with diagonal steel bands

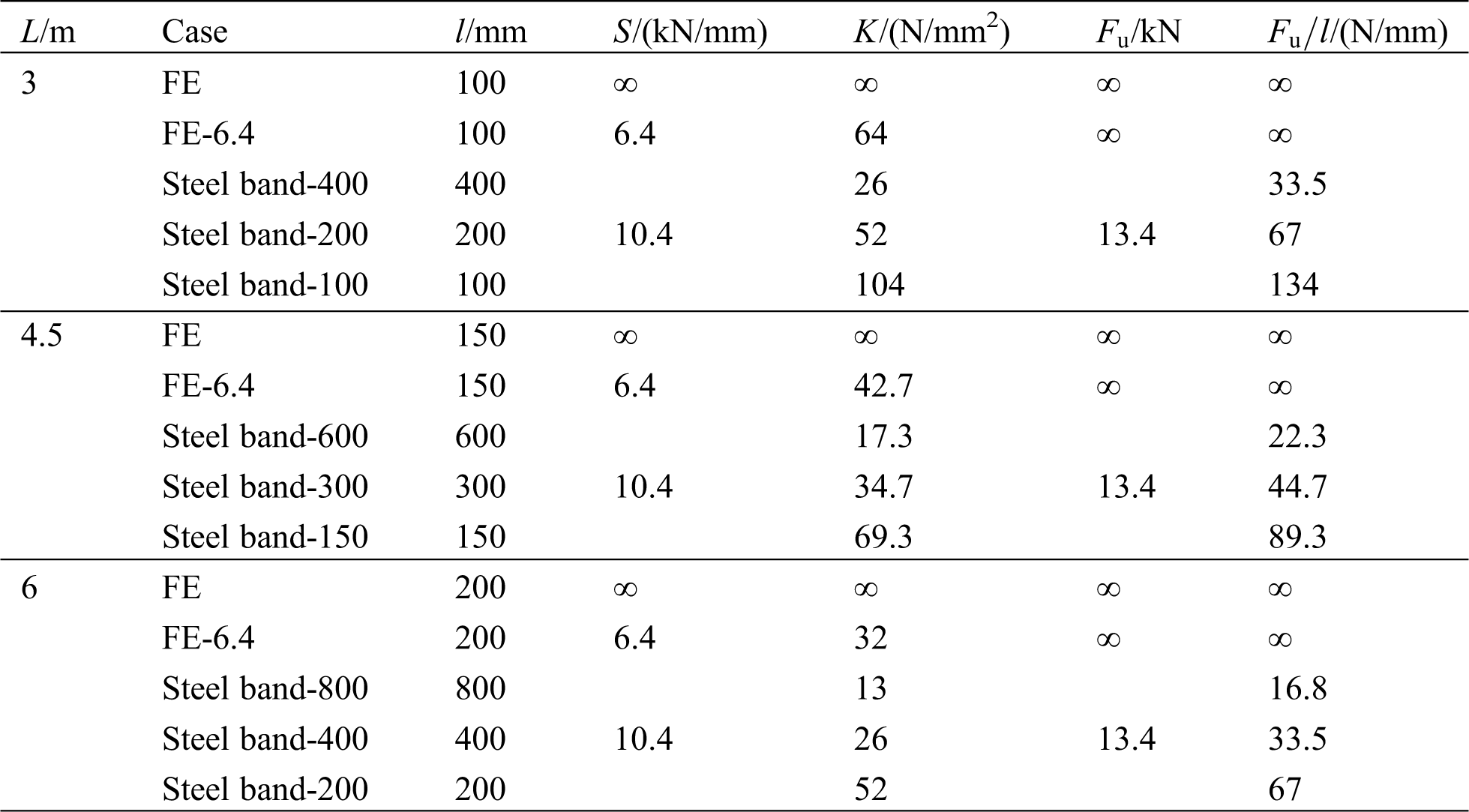
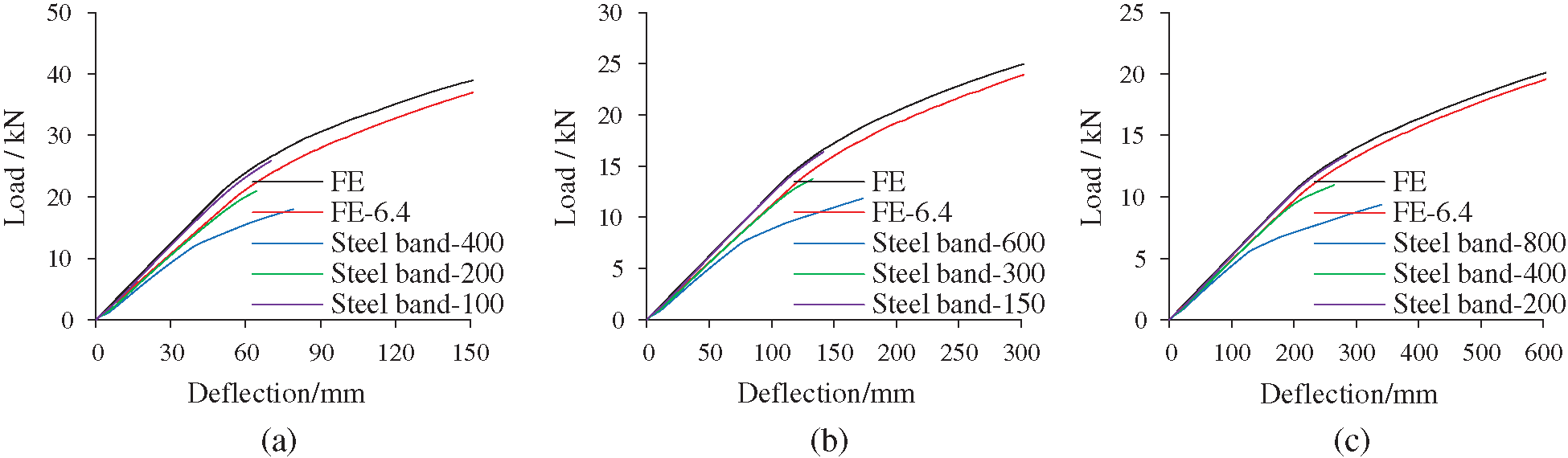
Figure 28: Load-deflection curves with diagonal steel bands. (a) L = 3 m, (b) L = 4.5 m and (c) L = 6 m

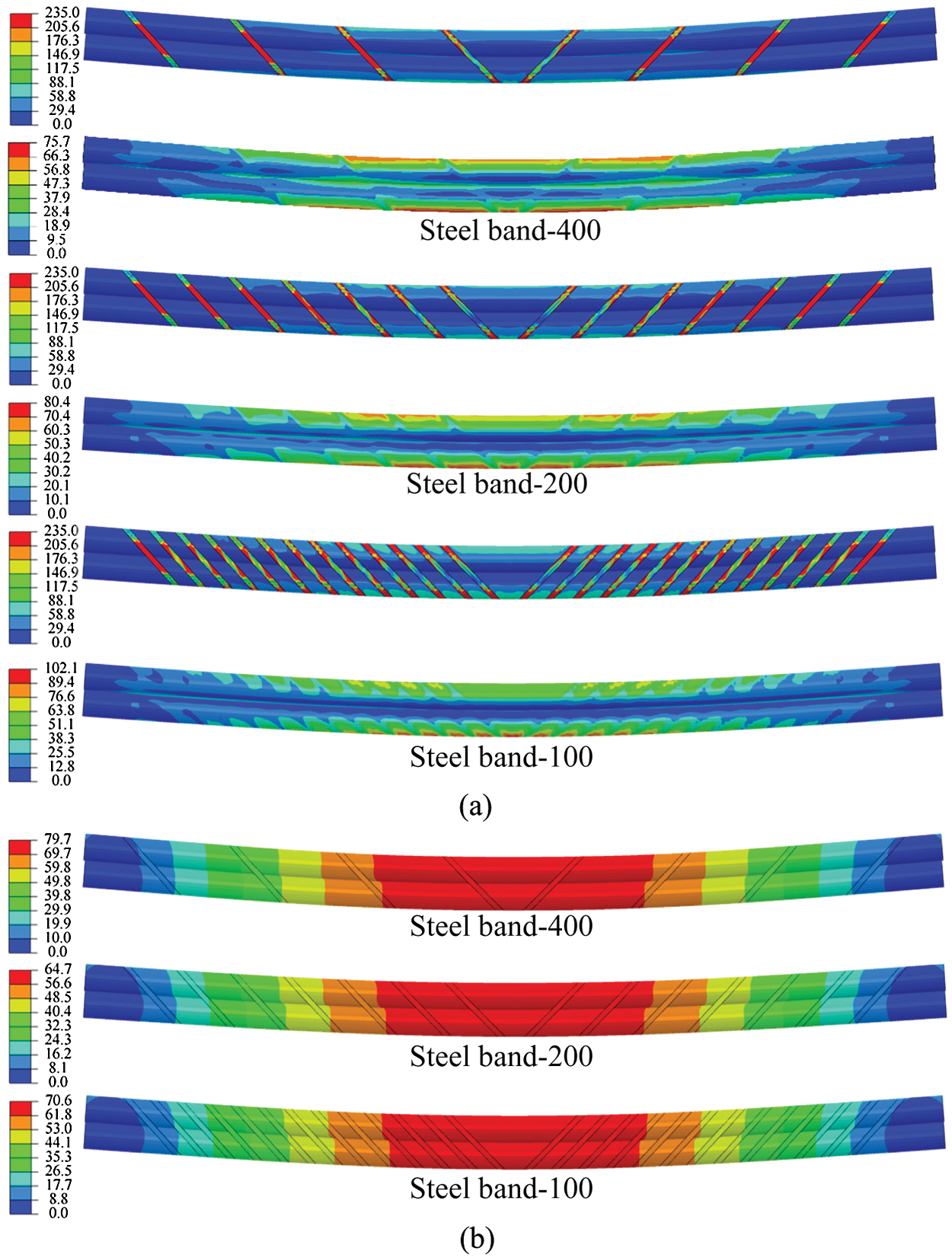
Figure 29: Ultimate state of double-pole bamboo beams with diagonal steel bands (L = 3 m). (a) Stress distribution (Unit: MPa) and (b) Deformation distribution (Unit: mm)

4  Conclusions

In this study, the flexural behavior of single-pole and double-pole bamboo beams was investigated via theoretical analysis. Simultaneously, the influence of interfacial slippage was analyzed. Then, an effective configuration to avoid slippage was proposed and validated. The following conclusions can be drawn:

1.   A method for calculating the load-deflection curve is proposed, considering the material’s nonlinearity. FE analysis is conducted to validate the calculation method. The FE results match well with the theoretical results. Moreover, the FE results indicate that the influences of the poles’ natural taper and bamboo joints are limited.

2.   The interfacial slippage between the two bamboo poles is difficult to avoid in double-pole bamboo beams. The shear stiffness of the interface per unit length has a significant influence on the flexural behavior of double-pole bamboo beams. For different shear stiffnesses, the load-deflection curves are obtained via theoretical and FE analyses.

3.   A novel configuration using diagonal steel bands is presented to avoid slippage. Owing to the diagonal arrangement, the tension stiffness of the steel band can be converted to horizontal shear stiffness between the two bamboo poles. An inclination angle of 45° is suggested to adequately develop the stiffness and bearing capacity of the band.

Funding Statement: This research was supported by the National Key Research and Development Program of China (Grant No. 2017YFC0703502), the National Natural Science Foundation of China (Grant No. 51608433), the Key Lab of Plateau Building and Eco-Community in Qinghai (KLKF-2020-001), and the Shaanxi Province Youth Science and Technology New Star Program (Grant No. 2019KJXX-040). These financial supports are greatly acknowledged.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.