Journal of Renewable Materials

DOI: 10.32604/jrm.2021.015544

ARTICLE

Prediction of Mechanical Properties of Structural Bamboo and Its Relationship with Growth Parameters

Pengcheng Liu, Ping Xiang, Qishi Zhou*, Hai Zhang, Jiefu Tian and Misganu Demis Argaw

School of Civil Engineering, Central South University, Changsha, 410075, China
*Corresponding Author: Qishi Zhou. Email: qishizhou@csu.edu.cn
Received: 26 December 2020; Accepted: 24 February 2021

1  Introduction

Bamboo is a natural, renewable, fast-growing green building material with wide distribution and excellent mechanical properties [1–6]. China is the center of the distribution of bamboo resources in the world, and bamboo is the most commonly used species in structure and has been applied in engineering [7–9]. A clear understanding of the mechanical properties is crucial to the engineering application of structural bamboo. It is also important for steam softening and bamboo flattening technology [10]. Due to the inhomogeneous and anisotropic characteristics of bamboo, the mechanical properties of bamboo with different sizes, directions and heights are not the same, which leads to a large amount of time and cost of bamboo performance test in engineering application.

In order to explore the correlation between bamboo properties and predict the mechanical properties, experiments were carried out to study the correlation between the physical and mechanical properties of bamboo and relevant results were obtained. Sá Ribeiro et al. [11] carried out a bending test of bamboo stalks and obtained a model for predicting bending strength through bending elastic modulus and predicting the elastic modulus and bending strength through the density. Ren et al. [12] and Kumar et al. [13] studied the relationship between the compressive strength, bending strength, tensile strength, and density of bamboo along the grain, and the results showed that the mechanical properties of bamboo have a correlational relationship with density. Dixon et al. [14] studied the relationship between the axial compressive properties of moso bamboo and the density, and the results showed that a linear correlation existed between them. At present, there are few literatures on the prediction of the mechanical properties of bamboo, and some of them are mainly based on a small number of samples to study the tensile, compressive and bending performance indicators along the grain. There is still a lack of research on the relationship between the mechanical properties of moso bamboo materials and growth parameters, conversion model between different mechanical properties of bamboo has not been put forward.

In order to systematically study the mechanical properties of bamboo, predict the mechanical properties through growth parameters, and facilitate the conversion between mechanical properties, this paper mainly carried out the following works on the bamboo materials produced in China: (1) considering the influence of bamboo nodes, a system was developed on moso bamboo longitudinal compression, bending resistance, longitudinal shear, longitudinal tensile, transverse compression, and transverse tensile performance test; (2) the relationship between the mechanical properties of moso bamboo and wall thickness and perimeter was fitted by univariate and multiple regression methods, and the prediction formula was given; (3) the conversion parameters between the various mechanical properties of moso bamboo materials are derived, proposed and verified. In addition, through the prediction formula and conversion method proposed in this paper, the mechanical properties of bamboo can be predicted by using simple size measuring tools.

2  Materials and Methods

2.1 Bamboo Selection and Mechanical Performance Test

The moso bamboo materials used in this research is obtained from Chenzhou, Hunan, China. In a bamboo forest, 160 straight bamboos with the age of 3–4 years, diameters of around 100 mm, and the height of 6 m were randomly collected in December (Fig. 1a), from which 25 samples were selected for mechanical properties tests. The cut bamboos were transported to the test site and stacked in the shading shed. The location of the stacked bamboos was ventilated and irritable to avoid mildew.

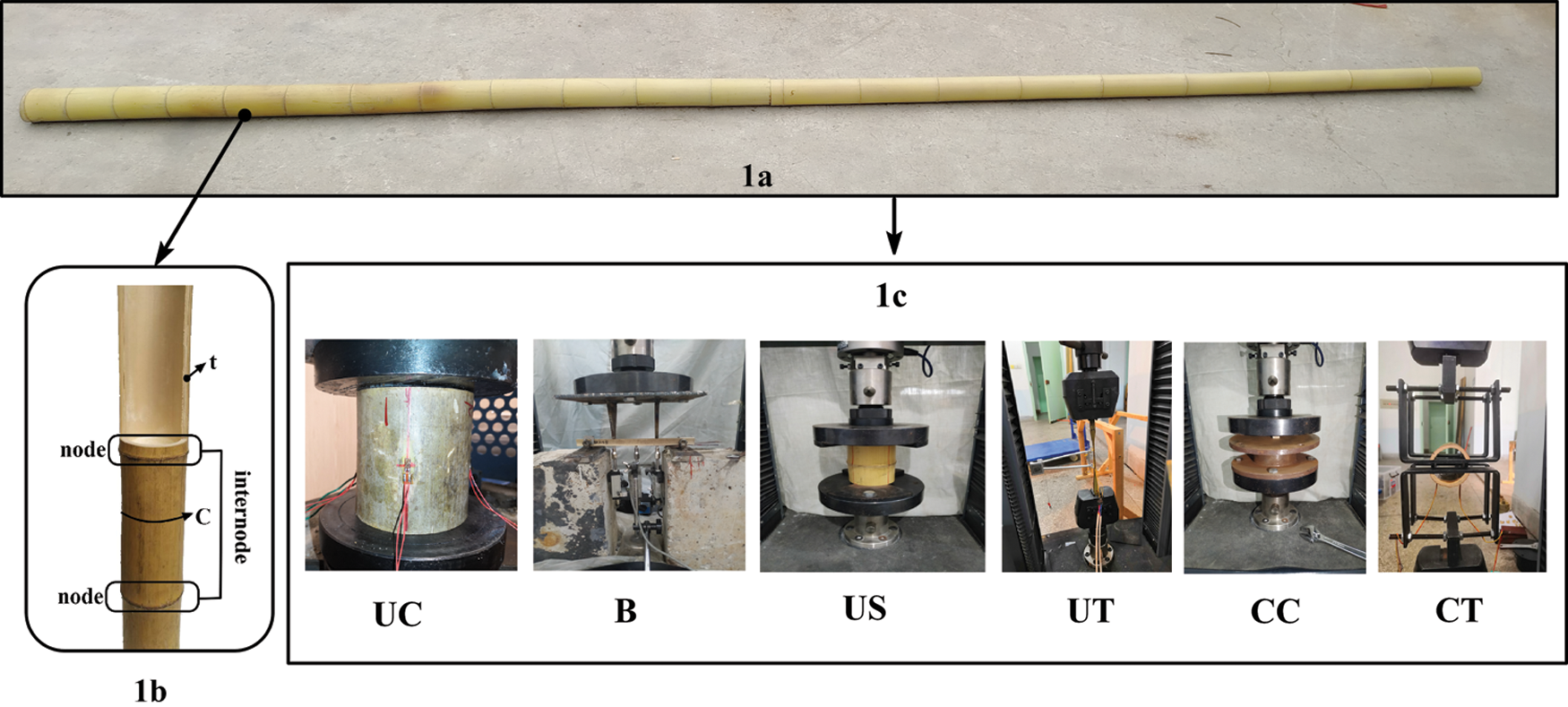
Figure 1: Schematic diagram of material selection and loading

As shown in the Fig. 1b of bamboo structure, t and C are the wall thickness and outer circumference of the bamboo, respectively. The preparation of each specimen is under full consideration of factors such as bamboo nodes and height, and the sampling is based on the principle of uniform distribution of the whole bamboo stalk along the height.

Refer to the standard JG/T199-2007 [15] and ISO 22157-1-2019 [16], six types of specimens of longitudinal compression (UC), bending (B), longitudinal shear (US), longitudinal tensile (UT), transverse compression (CC) and transverse tensile (CT) were made for the investigation of mechanical properties. The ratio of length to diameter of the UC and US specimens is 1, the size of the B specimen is 220 mm × 15 mm × t mm, the size of the UT specimen is 330 mm × 15 mm × t mm, the size of the CC specimen is 15 mm × 15 mm × t mm, and the length of CT specimen is 100 mm.

The mechanical performance tests was carried out according to the standards [15,16]. Universal testing machines were used to load various specimens for mechanical properties as shown in Fig. 1c. During the loading, the UC, US, and UT test loading rate is 0.01 mm/s, the B test loading rate is 150 N/mm2 per minute, the CC test loading rate is 20 N/mm2 per minute, and the CT test loading rate is 0.005 mm/s. The formula of the strength and elastic modulus of the specimen is as follows:

fW=PmaxA (1)

EW=20ΔPAΔl (2)

MORW=150Pmaxtb2 (3)

MOEW=1920000ΔP8δmtb3 (4)

where, fW is the strength of UC, US, UT, CC and CT specimens with the moisture content W (MPa); EW is the elastic modulus along the grain with the moisture content W (MPa); MORW is the bending strength with the moisture content W (MPa); MOEW is the flexural modulus of elasticity with the moisture content W (MPa); Pmax is the failure load (N); A is the surface area ( mm2 ); t is the thickness of the specimen (mm); b is the specimen height (mm); ∆P is the difference between the upper and lower limit loads (N); ∆l is the difference between the deformation values of the specimen under the upper and lower limit loads (mm); δm is the pure bending deflection value of the specimen under the action of ∆P (mm).

2.2 The Adjustment of Moisture Content

After the failure of specimens, a test specimen with a mass of not less than 1.5 g near the damage zone was collected immediately for the moisture content test. The moisture content is calculated according to formula (5). As the moisture content has a significant impact on the mechanical properties of bamboo [17], in this study, the value of the mechanical properties was uniformly adjusted to the value under the standard moisture content (12%). The adjustment formula (6) is shown in equation [15].

W=m1−m0m0×100 (5)

M12=KWMW (6)

KW=1a+becw (7)

where, W is the air-dry moisture content (%); m1 and m0 are the air-dry and full-dry mass (g), respectively; M12 is the strength or elastic modulus of the specimen under the standard moisture content (12%); MW is the strength or elastic modulus of the specimen when the moisture content is W; KW is the moisture content correction coefficient, which is related to the specific mechanical properties and moisture content. Parameter a, b and c refer to Standard [15].

3  Results and Discussion

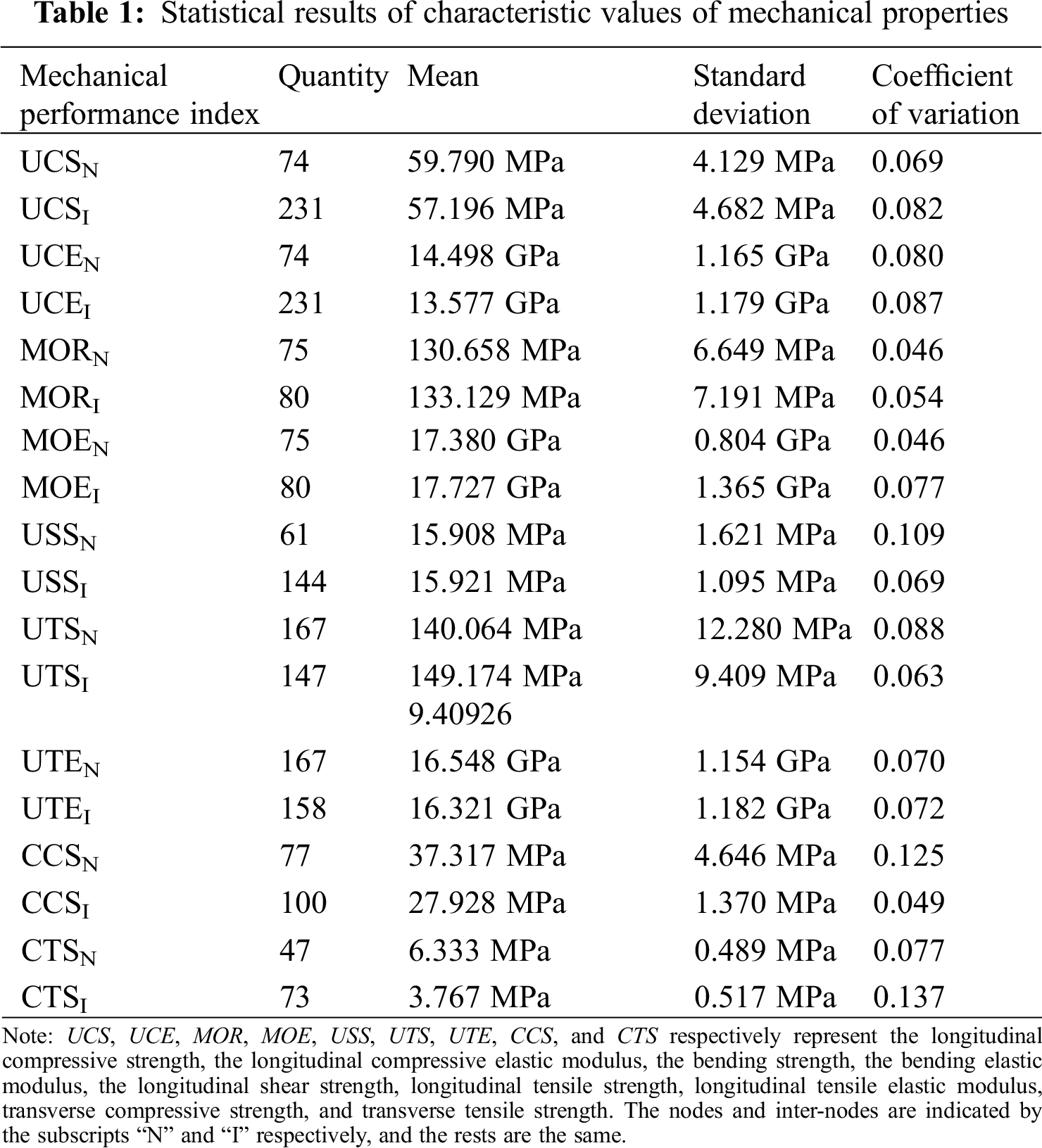
3.1 Statistical Analysis of Characteristic Values

Statistics of various mechanical properties of bamboo under standard moisture content (12%) are shown in the box diagram Fig. 2. Excluding the outliers in the box chart, the results are obtained and shown in Tab. 1. It can be seen from the results that the mechanical properties of bamboo show significant anisotropy, and the longitudinal tensile, longitudinal compressive and bending properties are particularly excellent. The longitudinal tensile and longitudinal compressive strengths are significantly greater than the transverse tensile and transverse compressive strengths. The longitudinal tensile strength is slightly greater than the bending strength. The longitudinal tensile and bending strengths are obviously greater than the longitudinal compressive strength. The transverse compressive strength is obviously greater than the transverse tensile strength. Meanwhile, the bamboo nodes have a certain influence on the value of various mechanical properties, especially the mechanical properties in the transverse direction.

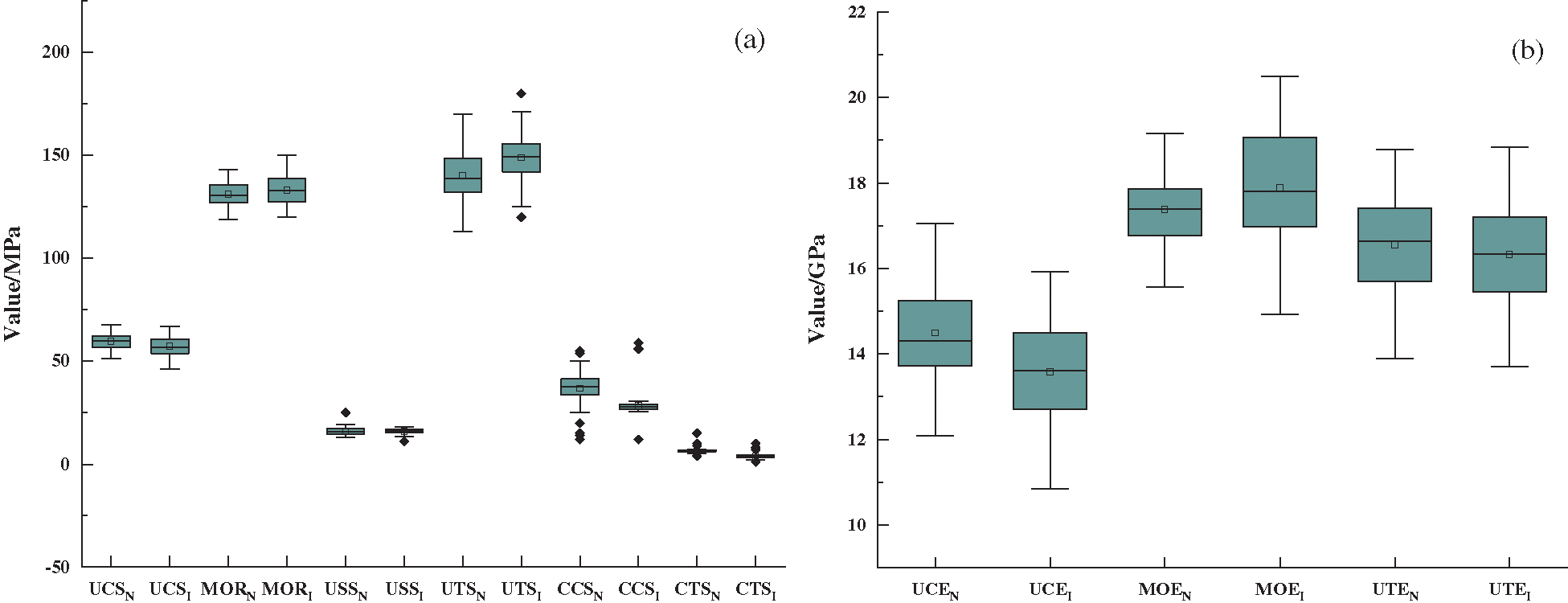
Figure 2: Statistical box diagram of mechanical properties: (a) Strength; (b) Elastic modulus

3.2 The Relationship between Growth Parameters

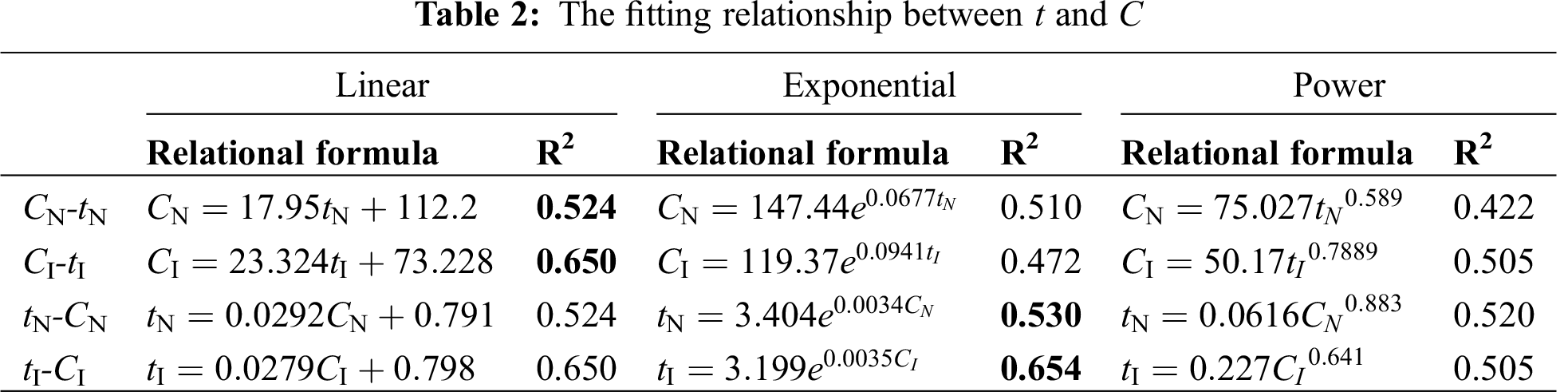
Based on the least-squares method, considering the differences between the nodes and the inter-nodes, the linear function, exponential function, and power function are used to fit t and C to obtain the fitting curve as shown in Fig. 3. The curve and relationship in the figure are the best fitting curves with its relational expression. The fitting relational expressions are shown in Tab. 2. The coefficient of determination R2 is used to evaluate the fitting effect, and the results show that t and C have a strong correlation, and from the relationship in Tab. 2, t and C can be converted.

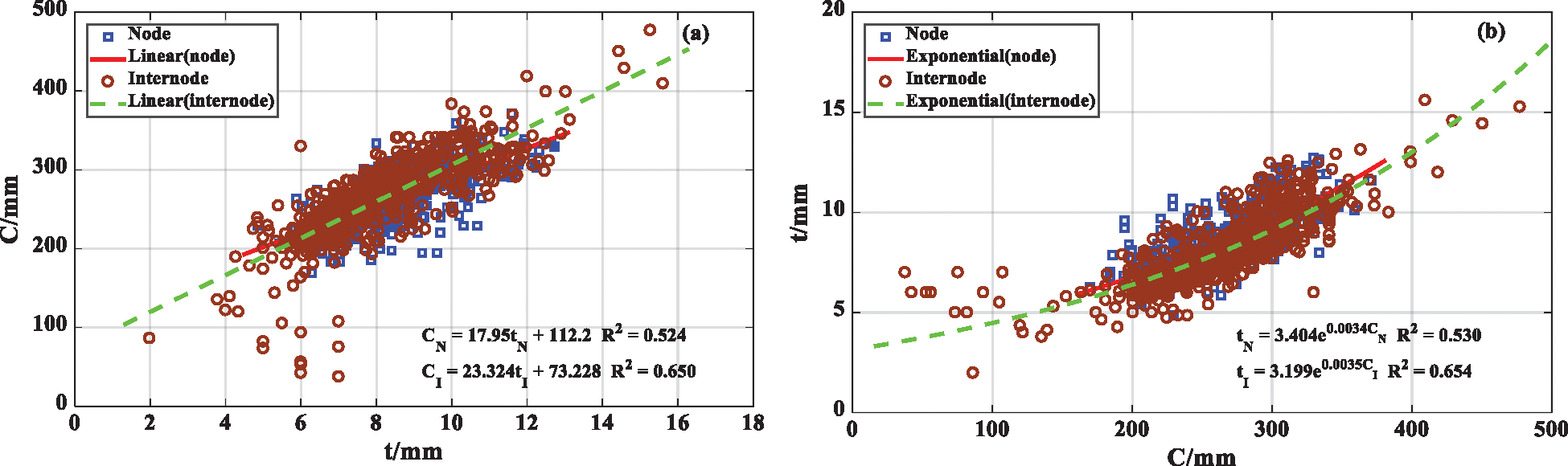
Figure 3: Fitting curve between t and C: (a) C-t; (b) t-C

3.3 The Relationship between Mechanical Properties and Wall Thickness

By equating the UCS, UCE, MOR, MOE, USS, UTS, UTE, CCS and CTS of bamboo materials to t, respectively, the fitting curve shown in Fig. 4 and the fitting relationship are obtained and shown in Tab. 3. The results show that the R2 values fitted by the three functions are relatively close, and the best fitting functions under different fitting materials are different. The longitudinal mechanical properties, the bending resistance, and the CCS of the inter-node specimens decrease with the increase of t. The CCS of node specimen and CTS of the node & inter-node specimens increase with the increase of t. The result clearly shows that bamboo joints have a significant effect on CCS and CTS.

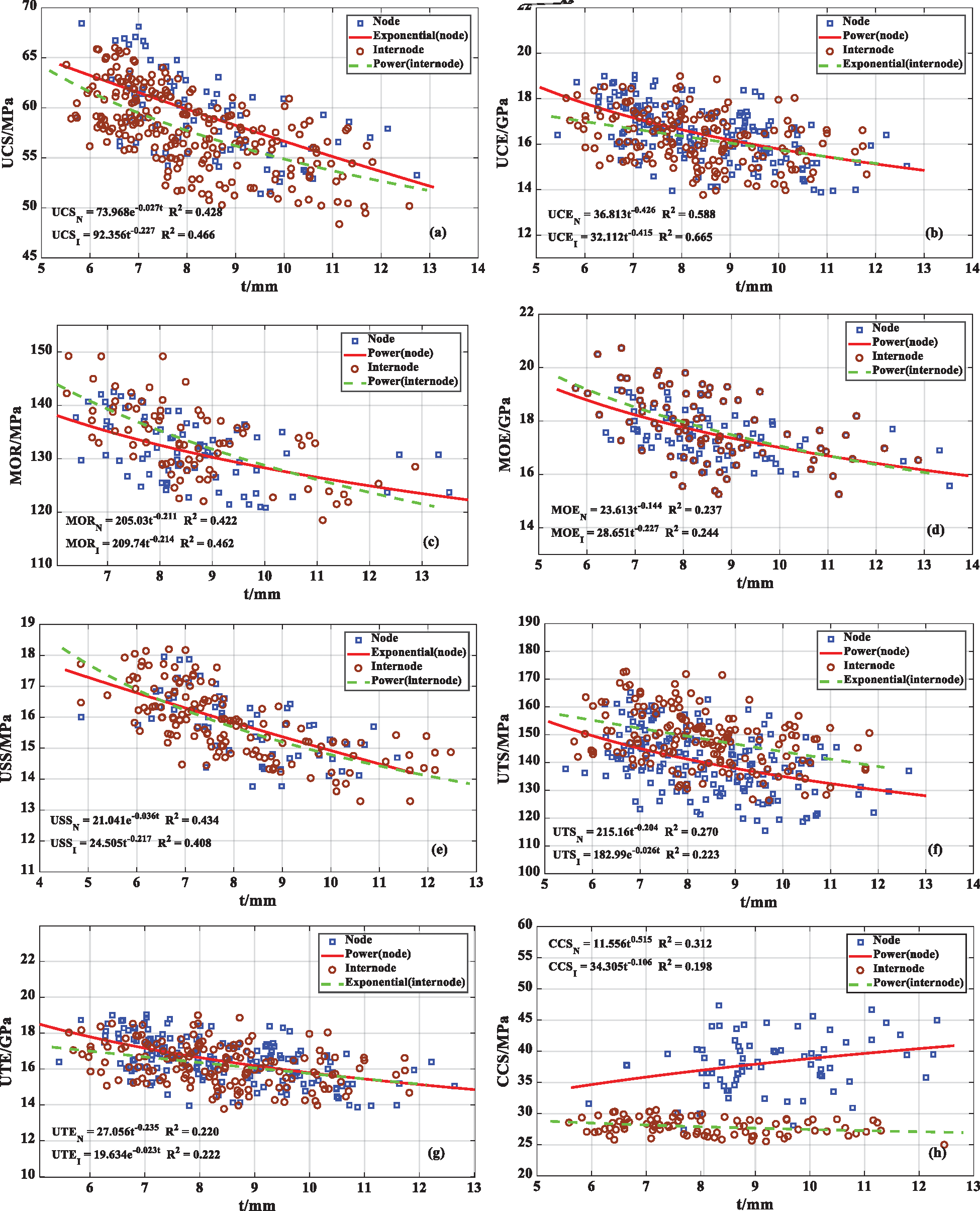
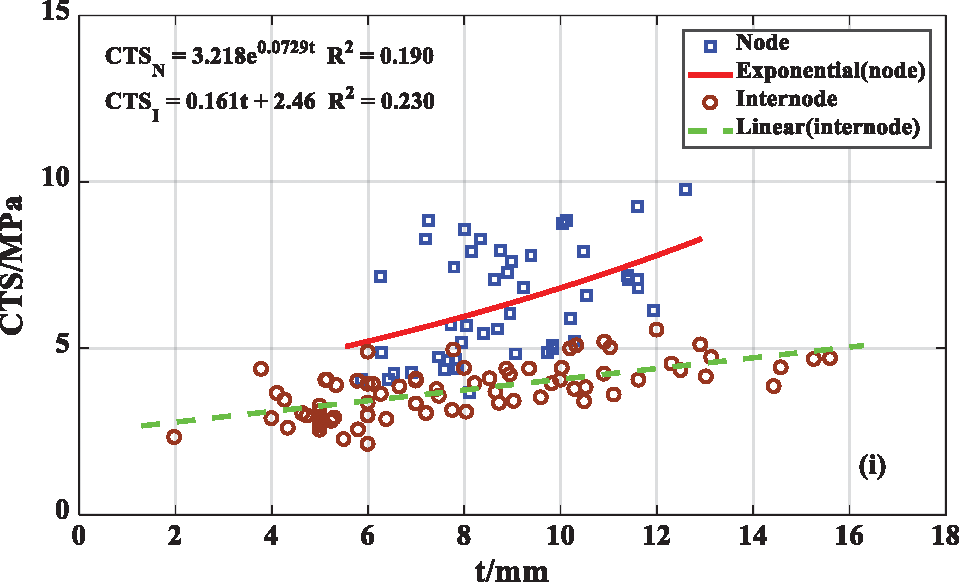
Figure 4: The fitting curve of mechanical properties and t: (a) UCS; (b) UCE; (c) MOR; (d) MOE; (e) USS; (f) UTS; (g) UTE; (h) CCS; (i) CTS

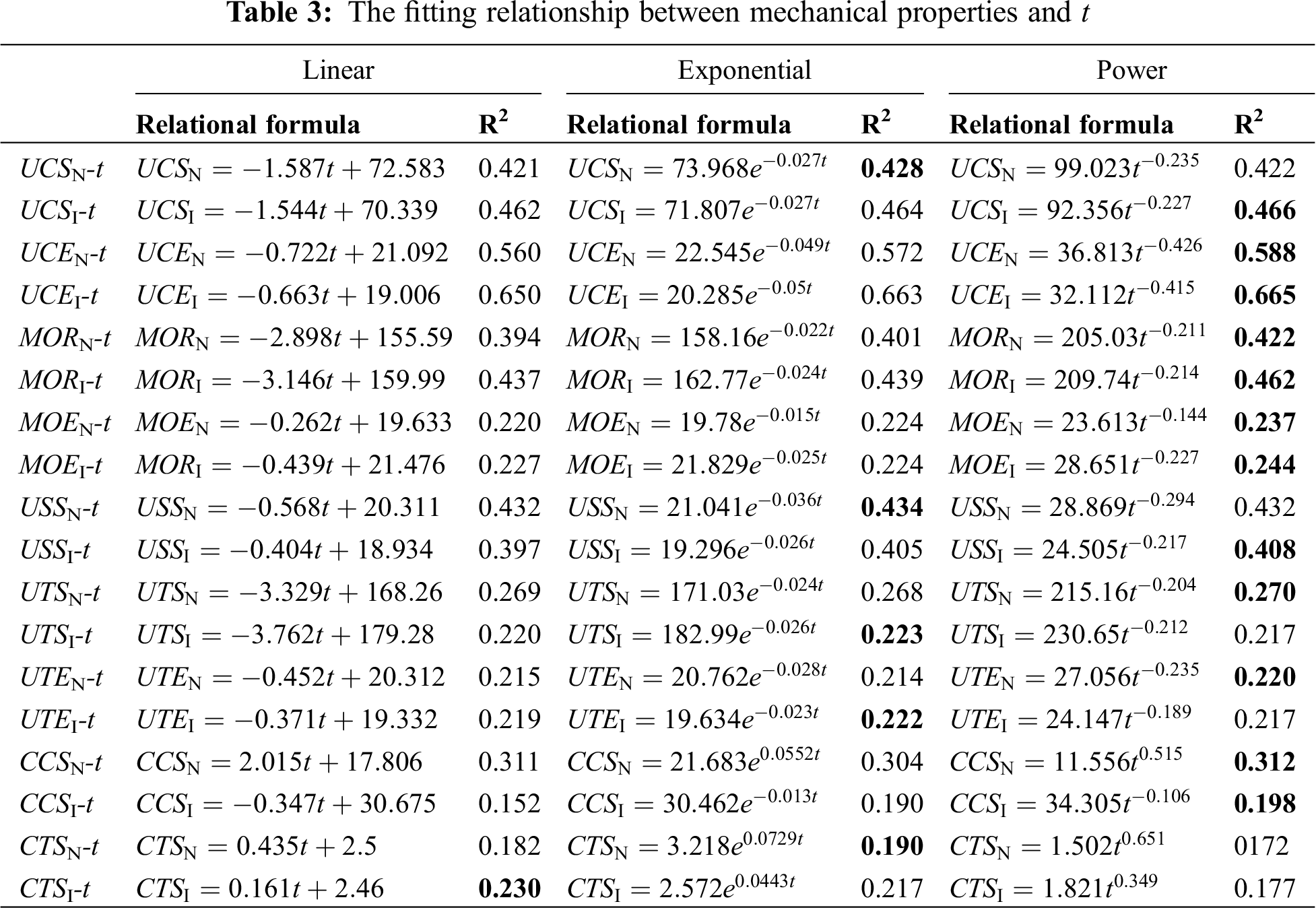
The relationship between the mechanical properties of bamboo and t shows the above rules and is related to the structure of bamboo. Bamboo is mainly composed of vascular bundles that play a bearing role and basic tissues that connect and transfer loads [18]. With the increase of t, that is, with the decrease of bamboo height h, the density of bamboo vascular bundles gradually decreases. Because the vascular bundles play a decisive role in the stress along the grain direction, the vascular bundles against the growth direction of bamboo stalk are not orderly arranged and the photosynthesis is weaker, which makes the mechanical properties of bamboo along the grain direction decrease with the increase of t. The vascular bundle also plays a major role in bending and transverse compression, so the MOR and CCS of the inter-node specimens gradually decrease with the increase of t. However, due to the polishing treatment of node during the production of CC specimens, the proportion of polished vascular bundles decreases with the increase of t. Thus, the CCS of the node specimens gradually increase as t increases. As the basic tissue plays a major role in the transverse tension, the proportion of basic tissue increases with the increase of t, so CTS and t are positively correlated.

3.4 The Relationship between Mechanical Properties and the Outer Circumference

The relationship between the mechanical properties of bamboo and C, and the relationship between the mechanical properties of bamboo and t are similar. The fitting results are shown in Fig. 5 and Tab. 4. The mechanical properties along the grain and the CCS of the inter-node specimens decrease with the increase of C, while the CCS of node specimen and CTS of the node & inter-node specimens increase with the increase of C. Obviously, the bamboo joints have a significant effect on the CCS and CTS specimens; and the R2 value and the best fitting function of the same mechanical properties obtained by fitting t and C are not the same.

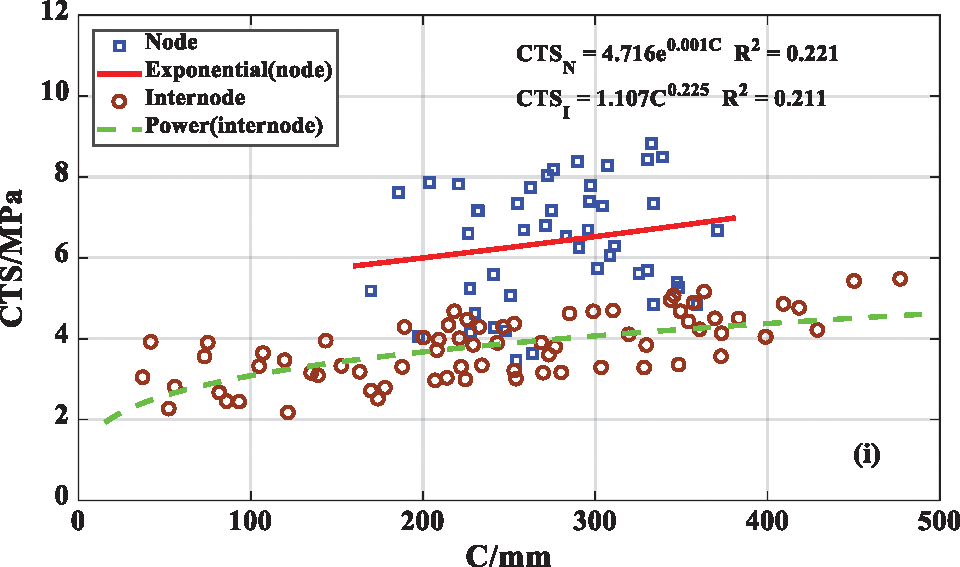
Figure 5: The fitting curve of mechanical properties and C: (a) UCS; (b) UCE; (c) MOR; (d) MOE; (e) USS; (f) UTS; (g) UTE; (h) CCS; (i) CTS

3.5 Multiple Regression Analysis of Mechanical Properties, Wall Thickness, and the Outer Circumference

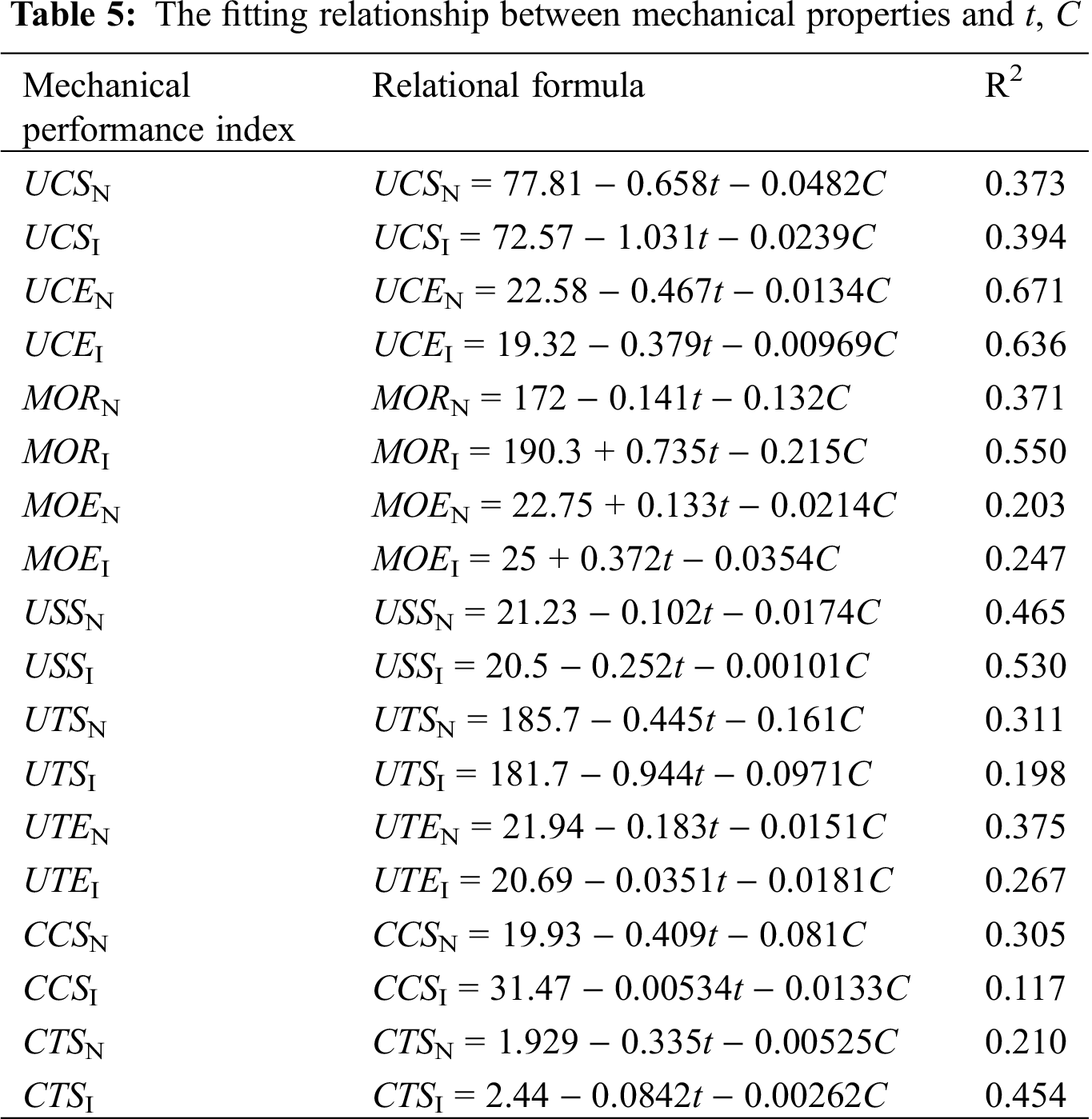
To compare the effects of multiple regression fitting and univariate fitting, a binary linear function was used to fit the mechanical properties of bamboo with t and C, and the results were obtained and presented in Fig. 6 and Tab. 5. The comparison of R2 of the best-fitting relations in Tabs. 3 and 4 and the results in Tab. 5 shows that using t to predict UCS (including UCSN and UCSI), UCEI, MOEN, and CCSI is better, and C is used to predict MOR, MOEI, USSN, UTS, UTEI, CCSN, and CTSN, which also has better prediction effects. The use of t and C bivariate has a better fitting effect for the prediction of UCEN, USSI, UTEN, and CTSI. In general, the relationship between bamboo material and growth parameter plays a significant role in fitting and analysis [11,14]. Through the univariate and bivariate fitting relations of mechanical properties and growth parameters, simple size measurement tools can be used. In the absence of test conditions, the mechanical properties of bamboo can be tested efficiently and quickly. The best prediction effect can be obtained by using the relationship formula with the highest R2 value in the univariate and bivariate fitting.

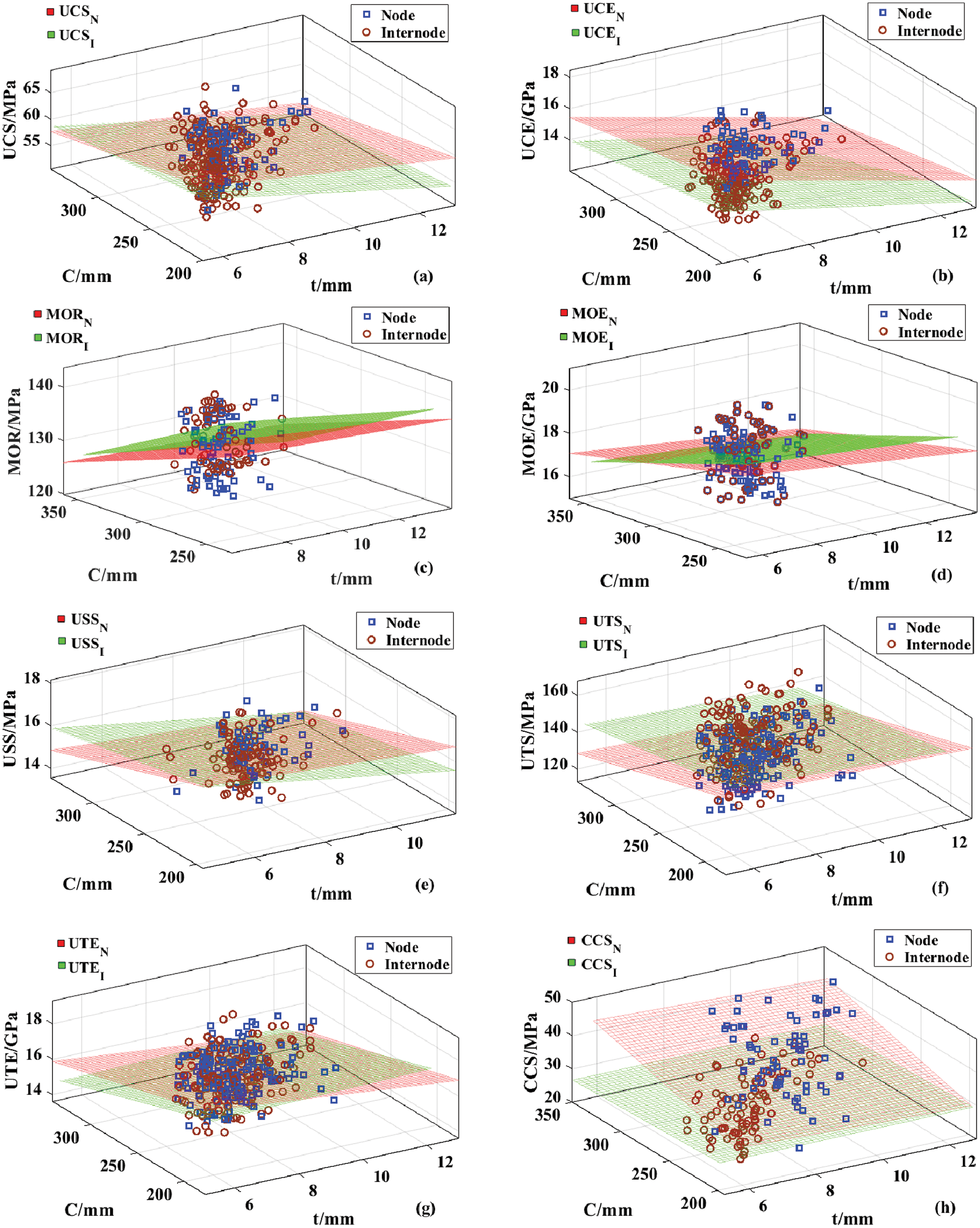
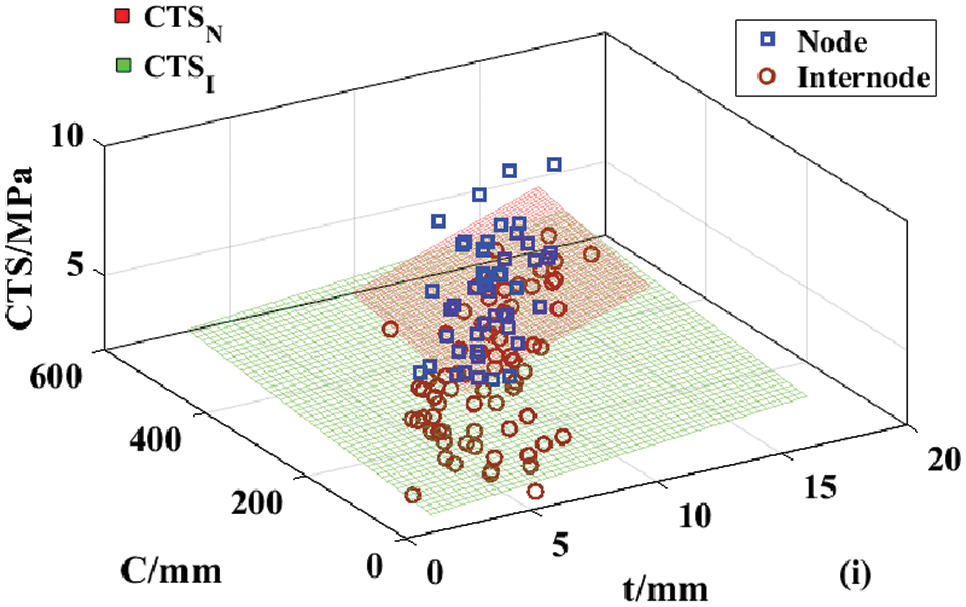
Figure 6: Multiple regression results of mechanical properties and t, C: (a) UCS; (b) UCE; (c) MOR; (d) MOE; (e) USS; (f) UTS; (g) UTE; (h) CCS; (i) CTS

3.6 The Relationship between the Mechanical Properties of Nodes and Inter-Nodes

Based on the fitting results between the mechanical properties and t & C in this research, a method is proposed to derive the relationship between the mechanical properties of bamboo through the linear fitting relationship between the mechanical properties and the growth parameters. The average value of the determination coefficient R2 of the linear fitting of various mechanical properties of bamboo with t (Tab. 3) is 0.333, and the average value of the determination coefficient R2 for linear fitting with C (Tab. 4) is 0.356. Among the 18 linear fitting relationships between mechanical properties and growth parameters, the larger values of R2 of t and C are 6 and 12, respectively. Generally, the linear relationship between mechanical properties and C is used to derive the relationship between the mechanical properties of bamboo, and the operability of measuring C is also stronger in practical engineering. Based on the linear relationship between the mechanical properties of the node and inter-node specimens and C, the relationship between the mechanical properties of the node and inter-node specimens at the same position of the bamboo is deduced and shown in Tab. 6.

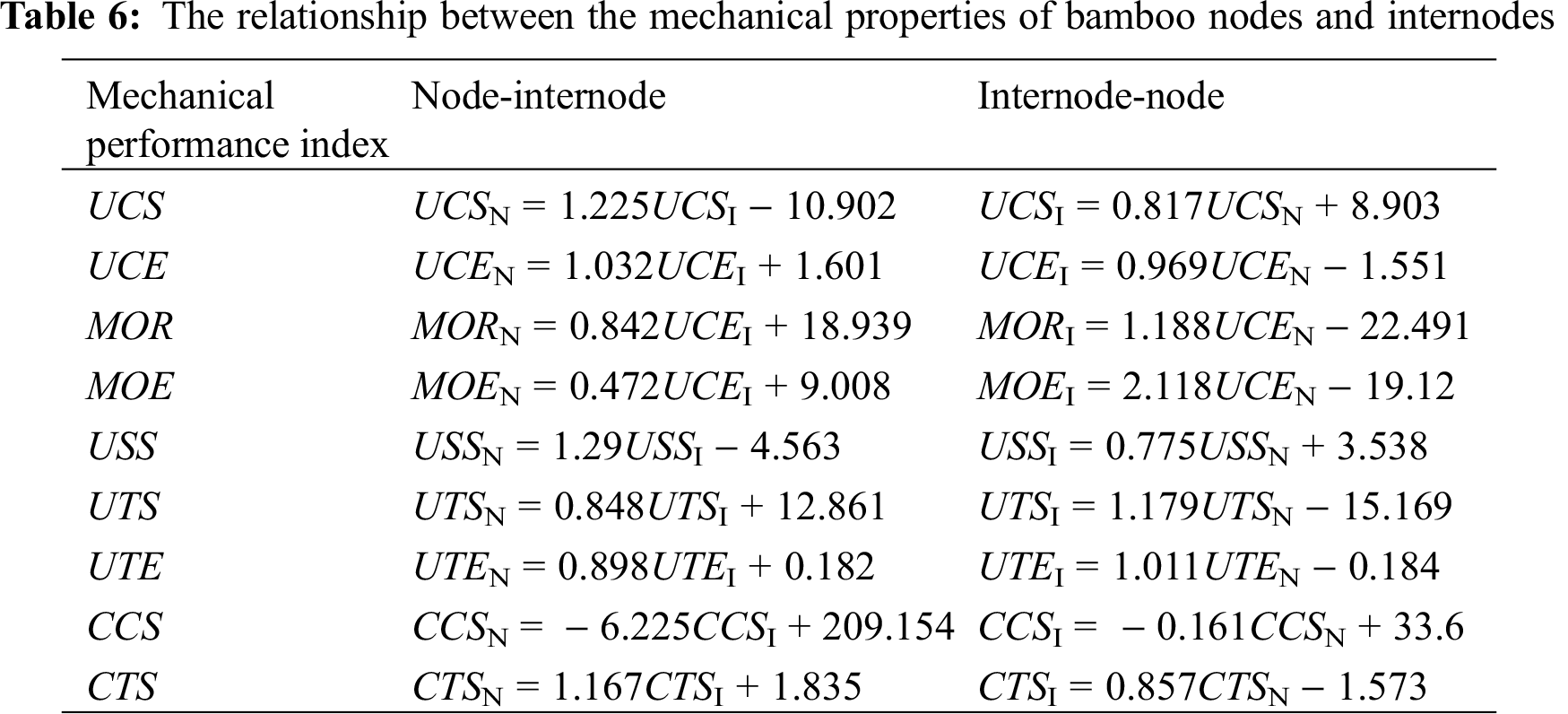
3.7 Conversion Parameters of Mechanical Properties

It is impossible to measure multiple mechanical properties of test specimens at the same time, and the relationship between the mechanical properties plays a vital role in the establishment of the mechanical property evaluation system of bamboo. The establishment of the relationship between the mechanical properties can greatly reduce the consumption and testing of materials cost. To solve this problem, a linear relationship between mechanical properties and C is used to derive the relationship between multiply mechanical properties. Eq. (7) is the conversion formula between mechanical properties.

M2=ηM1+θ (7)

where, M1 and M2 are mechanical performance indicators.

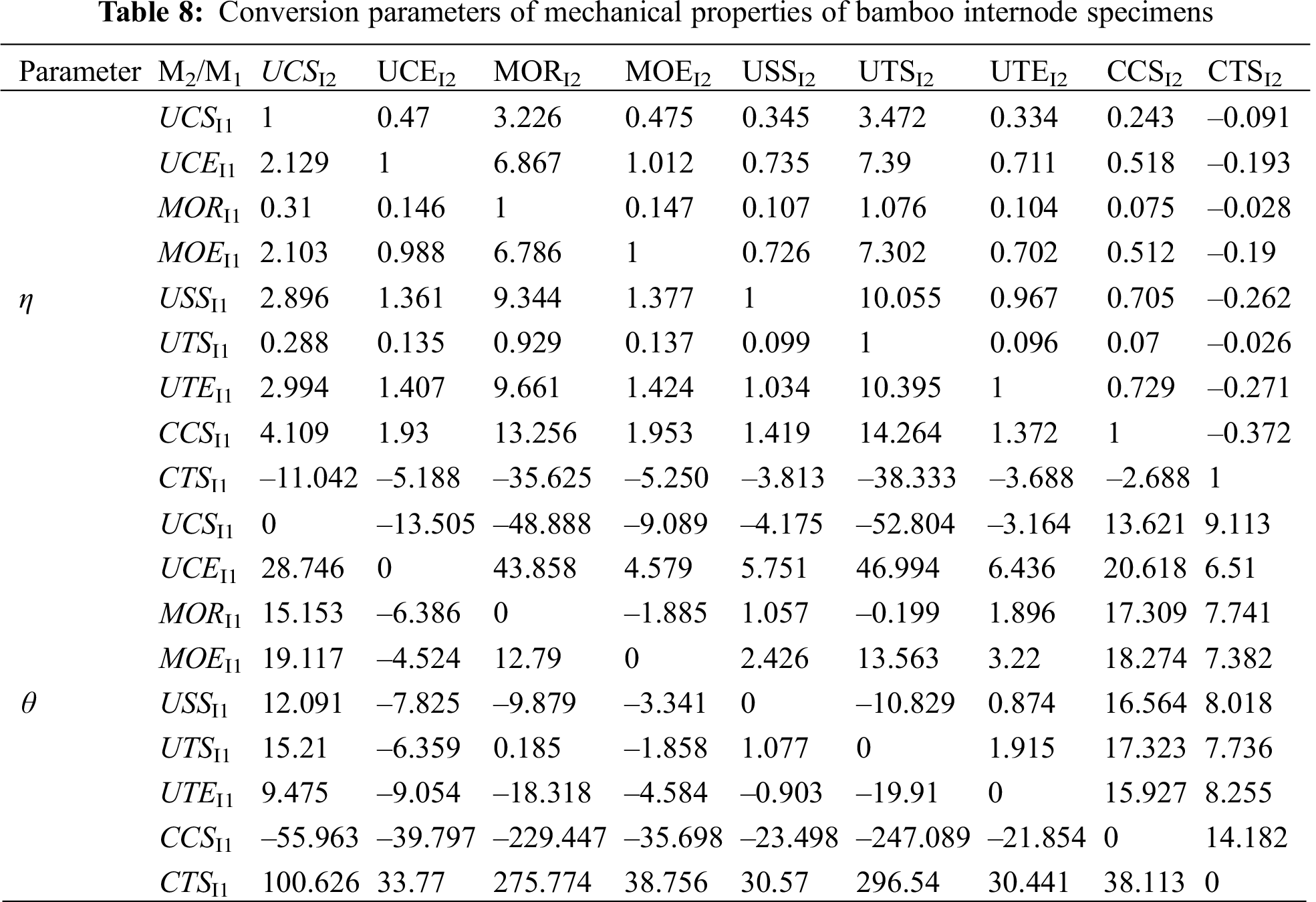
η and θ are defined as the conversion parameters of bamboo mechanical properties, as shown in Tabs. 7 and 8 for details. Since a large number of bamboo stalk materials are used in the application of the original bamboo structure, predicting the mechanical properties of a batch of bamboo stalks through a certain mechanical property can save a lot of materials, time and test costs. Also, the conversion parameters can provide a reference for the prediction of the mechanical properties of bamboo.

3.8 Verification of Prediction Formula

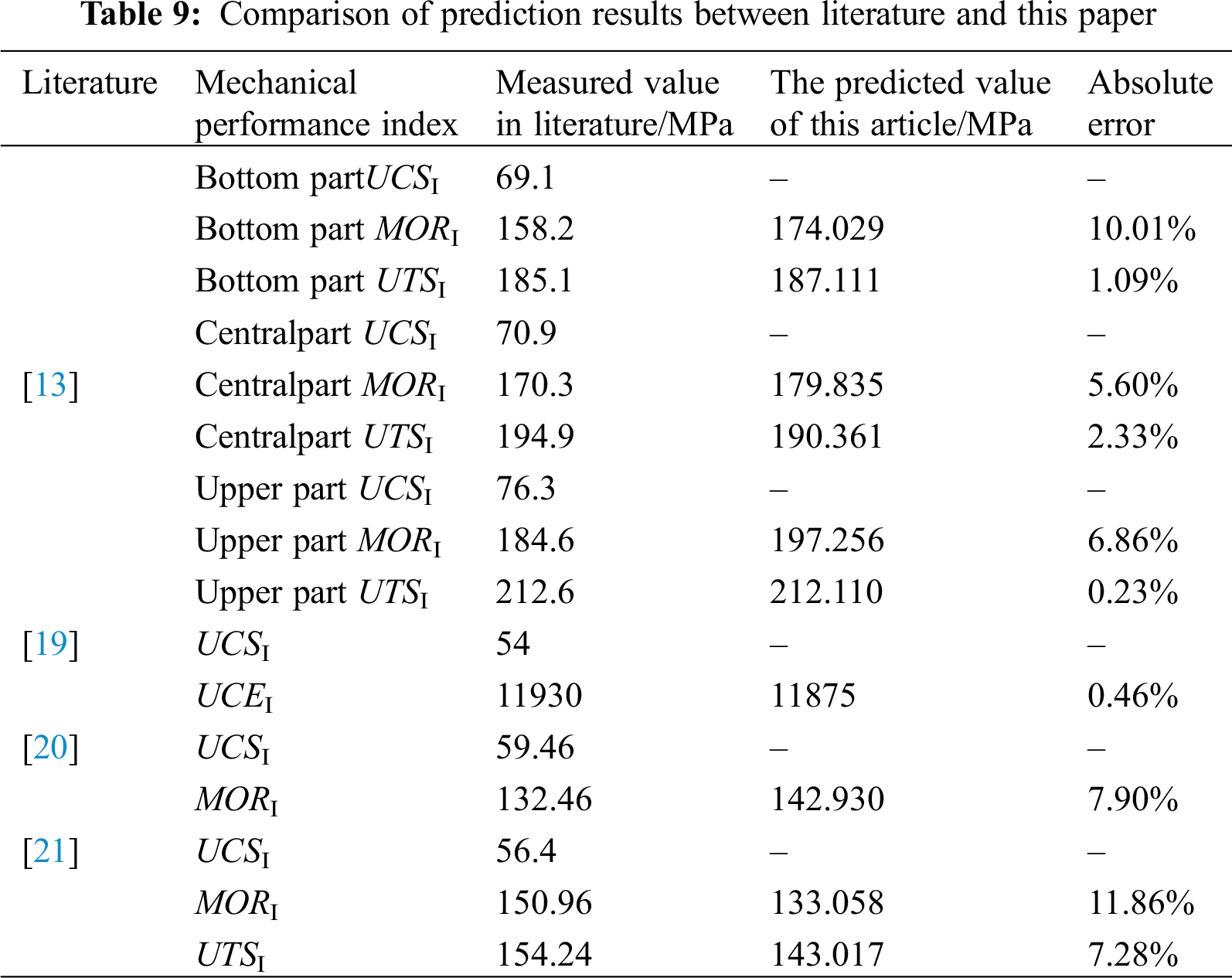
In order to verify the accuracy of the proposed prediction formula, UCSI is used as an independent variable, and the measured values of the mechanical properties of bamboo in the literature [12,19–21] are compared with the predicted values obtained in Tab. 8. The comparison results are summarized in Tab. 9. It can be seen that the predicted results by the proposed method are relatively close to the measured values of experiment, indicating that the prediction formula and conversion parameters of mechanical properties of moso bamboo obtained in this research have certain applicability and accuracy, and are useful for the application of bamboo structure.

4  Conclusions

1.    In this research, the mechanical performance tests of bamboo were carried out for longitudinal compression, bending, longitudinal shear, transverse compression and transverse tension. In addition, the mechanical characteristic values of bamboo nodes and inter-nodes specimens were statistically analyzed. The results show that the mechanical properties of bamboo show significant anisotropy. The longitudinal tensile and compressive strengths are obviously greater than the transverse tensile and compressive strengths, and the longitudinal tensile strength is slightly greater than the bending strength. The longitudinal tensile and bending strengths are obviously greater than the longitudinal compressive strengths, and the transverse compressive strength is obviously greater than the transverse tensile strength. However, the bamboo nodes have a certain influence on the value of various mechanical properties, especially the mechanical properties in the transverse direction.

2.    The Linear function, Exponential function and Power function were used to fit the performance indicators and growth parameters (wall thickness and outer circumference) of bamboo. It is concluded from the results that longitudinal mechanical properties, bending, and the transverse compressive strength of inter-node specimens decrease with the increase of wall thickness and outer circumference, while transverse compressive strength of nodal specimen and transverse tensile strength of nodal and inter-node specimen increase with the increase of wall thickness and outer circumference.

3.    There is a good correlation between the growth parameters, mechanical properties and the univariate and bivariate fitting of growth parameters. It is better to use wall thickness to predict the longitudinal compressive strength, the compressive elastic modulus of the inter-nodes, the bending elastic modulus of the nodes and the transverse compressive strength of the inter-nodes. On the other hand, it is better to use the outer circumference for the prediction of bending strength, the inter-node bending elastic modulus, node longitudinal shear strength, the inter-node longitudinal tensile strength, the node transverse compressive strength and node transverse tensile strength. The bivariate linear function of wall thickness and outer circumference is effective in predicting the longitudinal compressive elastic modulus of the node specimen, the longitudinal inter-node shear strength, the node longitudinal tensile elastic modulus and the inter-node transverse tensile strength.

4.    Comparing the fitting effects of mechanical properties by using wall thickness and outer perimeter, the linear fitting relationship between mechanical properties and outer perimeter is used to derive the bamboo mechanical property conversion parameter, which provides a reference for the prediction of the mechanical properties of bamboo. By comparing with the relevant results in literatures, the applicability and accuracy of the prediction formula and conversion parameters proposed in this work are verified.

Acknowledgement: The work described in this paper is supported by grants from the National Key R&D Program of China “Green Ecological Wooden bamboo structure and Demonstration Application”.

Funding Statement: This research was funded by the National Key Research & Development Program (Grant No. 2017YFC0703500).

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Li, H., Zhang, H., Qiu, Z., Su, J., Wei, D. et al. (2020). Mechanical properties and stress strain relationship models for bamboo scrimber. Journal of Renewable Materials, 8(1), 13–27. DOI 10.32604/jrm.2020.09341. [Google Scholar] [CrossRef]

 2.  Wang, F., Shao, Z. (2020). Study on the variation law of bamboo fibers’ tensile properties and the organization structure on the radial direction of bamboo stem. Industrial Crops and Products, 152(4), 112521. DOI 10.1016/j.indcrop.2020.112521. [Google Scholar] [CrossRef]

 3.  Li, H., Qiu, Z., Wu, G., Wei, D., Lorenzo, R. et al. (2019). Compression behaviors of parallel bamboo strand lumber under static loading. Journal of Renewable Materials, 7(7), 583–600. DOI 10.32604/jrm.2019.07592. [Google Scholar] [CrossRef]

 4.  Zhang, T., Wang, A., Wang, Q., Guan, F. (2019). Bending characteristics analysis and lightweight design of a bionic beam inspired by bamboo structures. Thin-Walled Structures, 142(3), 476–498. DOI 10.1016/j.tws.2019.04.043. [Google Scholar] [CrossRef]

 5.  Li, X., Lei, B., Lin, Z., Huang, L., Tan, S. et al. (2014). The utilization of bamboo charcoal enhances wood plastic composites with excellent mechanical and thermal properties. Materials & Design, 53, 419–424. DOI 10.1016/j.matdes.2013.07.028. [Google Scholar] [CrossRef]

 6.  Rassiah, K., Megat Ahmad, M., Ali, A. (2014). Mechanical properties of laminated bamboo strips from Gigantochloa Scortechinii/polyester composites. Materials & Design, 57(8), 551–559. DOI 10.1016/j.matdes.2013.12.070. [Google Scholar] [CrossRef]

 7.  Jiao, J., Tang, P. (2019). Application of bamboo in a design-build course: Lianhuadang Farm project. Frontiers of Architectural Research, 8(4), 549–563. DOI 10.1016/j.foar.2019.09.003. [Google Scholar] [CrossRef]

 8.  Crolla, K. (2017). Building indeterminacy modelling–the ‘ZCB Bamboo Pavilion’ as a case study on nonstandard construction from natural materials. Visualization in Engineering, 5(1), 1–12. [Google Scholar]

 9.  Das, S., Mukhopadhyay, P. (2018). Multi-hazard disaster resilient housing with bamboo-based system. Procedia Engineering, 212(12), 937–945. DOI 10.1016/j.proeng.2018.01.121. [Google Scholar] [CrossRef]

10. Lou, Z., Yuan, C., Li, Y., Shen, D., Yang, L. et al. (2020). Effects of saturated steam heat treatment on the chemical composition and crystallinity of bamboo bundles. Journal of Forestry Engineering, 5(2), 29–35 (In Chinese). [Google Scholar]

11. Sá Ribeiro, R., Sá Ribeiro, M., Miranda, I. (2017). Bending strength and nondestructive evaluation of structural bamboo. Construction and Building Materials, 146(1), 38–42. DOI 10.1016/j.conbuildmat.2017.04.074. [Google Scholar] [CrossRef]

12. Ren, H., Li, X., Wang, X., Zhou, Q., Lou, W. (2008). Research on physical and mechanical properties of moso bamboo. Chinese Forestry Science and Technology, 7(3), 50–55. [Google Scholar]

13. Kumar, A., Vlach, T., Laiblova, L., Hrouda, M., Kasal, B. et al. (2016). Engineered bamboo scrimber: Influence of density on the mechanical and water absorption properties. Construction and Building Materials, 127(2), 815–827. DOI 10.1016/j.conbuildmat.2016.10.069. [Google Scholar] [CrossRef]

14. Dixon, P., Gibson, L. (2014). The structure and mechanics of Moso bamboo material. Journal of the Royal Society Interface, 11(99), 20140321. DOI 10.1098/rsif.2014.0321. [Google Scholar] [CrossRef]

15. Chinese Standards Agenc, Testing methods for physical and mechanical properties of bamboo used in building. JG/T199-2007 (in Chinese). [Google Scholar]

16. [ISO] International Standards (2019). Bamboo–Determination of physical and mechanical properties–Part I: Requirements ISO 22157-1: 2019 (EInternational Organization for Standardization. Geneva (SZ). [Google Scholar]

17. Jakovljević, S., Lisjak, D. (2019). Investigation into the effects of humidity on the mechanical and physical properties of bamboo. Construction and Building Materials, 194(5), 386–396. DOI 10.1016/j.conbuildmat.2018.11.030. [Google Scholar] [CrossRef]

18. Zhou, A., Huang, D. S., Che, S. S., Zhang, P. D. (2012). Distribution of bamboo vascular bundles and their tensile mechanical properties. Journal of Building Materials, 15(5), 730–734 (in Chinese). [Google Scholar]

19. Lorenzo, R., Godina, M., Mimendi, L., Li, H. (2020). Determination of the physical and mechanical properties of moso, guadua and oldhamii bamboo assisted by robotic fabrication. Journal of Wood Science, 66(1), 40. DOI 10.1186/s10086-020-01869-0. [Google Scholar] [CrossRef]

20. Zhang, D., Wang, G., Zhang, W., Cheng, H. (2012). Study on the mechanical properties of Phyllostachys pubescens and round bamboo. Journal of Central South University of Forestry and Technology, 32(7), 119–123 (in Chinese). [Google Scholar]

21. Shao, Z., Huang, S., Wu, F., Zhou, L., Clement, A. (2008). Study on the structure and strength difference between internodes and internodes of Phyllostachys pubescens. Bamboo Research Transactions, (2), 48–52 (in Chinese). [Google Scholar]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.