
This journal publishes original research papers of reasonable permanent intellectual value, in the areas of computer modeling in engineering & Sciences, including, but not limited to computational mechanics, computational materials, computational mathematics, computational physics, computational chemistry, and computational biology, pertinent to solids, fluids, gases, biomaterials, and other continua spanning from various spatial length scales (quantum, nano, micro, meso, and macro), and various time scales (picoseconds to hours) are of interest. Papers which deal with multi-physics problems, as well as those which deal with the interfaces of mechanics, chemistry, and biology, are particularly encouraged. Novel computational approaches and state-of-the-art computation algorithms, such as soft computing, artificial intelligence-based machine learning methods, and computational statistical methods are welcome.
Science Citation Index (Web of Science): 2023 Impact Factor 2.2; Current Contents: Engineering, Computing & Technology; Scopus Citescore (Impact per Publication 2023): 3.8; SNIP (Source Normalized Impact per Paper 2023): 0.67; RG Journal Impact (average over last three years); Engineering Index (Compendex); Applied Mechanics Reviews; Cambridge Scientific Abstracts: Aerospace and High Technology, Materials Sciences & Engineering, and Computer & Information Systems Abstracts Database; CompuMath Citation Index; INSPEC Databases; Mathematical Reviews; MathSci Net; Mechanics; Science Alert; Science Navigator; Zentralblatt fur Mathematik; Portico, etc...
 Open Access
Open Access
REVIEW
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1-50, 2025, DOI:10.32604/cmes.2025.062113 - 11 April 2025
(This article belongs to the Special Issue: Environment Modeling for Applications of Mobile Robots)
Abstract Legged robots have always been a focal point of research for scholars domestically and internationally. Compared to other types of robots, quadruped robots exhibit superior balance and stability, enabling them to adapt effectively to diverse environments and traverse rugged terrains. This makes them well-suited for applications such as search and rescue, exploration, and transportation, with strong environmental adaptability, high flexibility, and broad application prospects. This paper discusses the current state of research on quadruped robots in terms of development status, gait trajectory planning methods, motion control strategies, reinforcement learning applications, and control algorithm integration. It More >
 Open Access
Open Access
REVIEW
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 51-113, 2025, DOI:10.32604/cmes.2025.057788 - 11 April 2025
(This article belongs to the Special Issue: Data-Driven and Physics-Informed Machine Learning for Digital Twin, Surrogate Modeling, and Model Discovery, with An Emphasis on Industrial Applications)
Abstract Cyber-Physical Systems (CPS) represent an integration of computational and physical elements, revolutionizing industries by enabling real-time monitoring, control, and optimization. A complementary technology, Digital Twin (DT), acts as a virtual replica of physical assets or processes, facilitating better decision making through simulations and predictive analytics. CPS and DT underpin the evolution of Industry 4.0 by bridging the physical and digital domains. This survey explores their synergy, highlighting how DT enriches CPS with dynamic modeling, real-time data integration, and advanced simulation capabilities. The layered architecture of DTs within CPS is examined, showcasing the enabling technologies and… More >
 Open Access
Open Access
REVIEW
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 115-152, 2025, DOI:10.32604/cmes.2025.061551 - 11 April 2025
Abstract Response analysis of structures involving non-probabilistic uncertain parameters can be closely related to optimization. This paper provides a review on optimization-based methods for uncertainty analysis, with focusing attention on specific properties of adopted numerical optimization approaches. We collect and discuss the methods based on nonlinear programming, semidefinite programming, mixed-integer programming, mathematical programming with complementarity constraints, difference-of-convex programming, optimization methods using surrogate models and machine learning techniques, and metaheuristics. As a closely related topic, we also overview the methods for assessing structural robustness using non-probabilistic uncertainty modeling. We conclude the paper by drawing several remarks through More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 153-183, 2025, DOI:10.32604/cmes.2025.062426 - 11 April 2025
Abstract Given the growing concern over global warming and the critical role of carbon dioxide (CO2) in this phenomenon, the study of CO2-induced alterations in coal strength has garnered significant attention due to its implications for carbon sequestration. A large number of experiments have proved that CO2 interaction time (T), saturation pressure (P) and other parameters have significant effects on coal strength. However, accurate evaluation of CO2-induced alterations in coal strength is still a difficult problem, so it is particularly important to establish accurate and efficient prediction models. This study explored the application of advanced machine learning (ML)… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 185-223, 2025, DOI:10.32604/cmes.2025.061865 - 11 April 2025
Abstract In this present work, we propose the expected Bayesian and hierarchical Bayesian approaches to estimate the shape parameter and hazard rate under a generalized progressive hybrid censoring scheme for the Kumaraswamy distribution. These estimates have been obtained using gamma priors based on various loss functions such as squared error, entropy, weighted balance, and minimum expected loss functions. An investigation is carried out using Monte Carlo simulation to evaluate the effectiveness of the suggested estimators. The simulation provides a quantitative assessment of the estimates accuracy and efficiency under various conditions by comparing them in terms of More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 225-254, 2025, DOI:10.32604/cmes.2025.062642 - 11 April 2025
Abstract The integration of technologies like artificial intelligence, 6G, and vehicular ad-hoc networks holds great potential to meet the communication demands of the Internet of Vehicles and drive the advancement of vehicle applications. However, these advancements also generate a surge in data processing requirements, necessitating the offloading of vehicular tasks to edge servers due to the limited computational capacity of vehicles. Despite recent advancements, the robustness and scalability of the existing approaches with respect to the number of vehicles and edge servers and their resources, as well as privacy, remain a concern. In this paper, a lightweight… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 255-287, 2025, DOI:10.32604/cmes.2025.061683 - 11 April 2025
Abstract Global mortality rates are greatly impacted by malignancies of the brain and nervous system. Although, Magnetic Resonance Imaging (MRI) plays a pivotal role in detecting brain tumors; however, manual assessment is time-consuming and susceptible to human error. To address this, we introduce ICA2-SVM, an advanced computational framework integrating Independent Component Analysis Architecture-2 (ICA2) and Support Vector Machine (SVM) for automated tumor segmentation and classification. ICA2 is utilized for image preprocessing and optimization, enhancing MRI consistency and contrast. The Fast-Marching Method (FMM) is employed to delineate tumor regions, followed by SVM for precise classification. Validation on More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 289-334, 2025, DOI:10.32604/cmes.2025.063954 - 11 April 2025
Abstract This study investigates the Maximum Power Point Tracking (MPPT) control method of offshore wind-photovoltaic hybrid power generation system with offshore crane-assisted. A new algorithm of Global Fast Integral Sliding Mode Control (GFISMC) is proposed based on the tip speed ratio method and sliding mode control. The algorithm uses fast integral sliding mode surface and fuzzy fast switching control items to ensure that the offshore wind power generation system can track the maximum power point quickly and with low jitter. An offshore wind power generation system model is presented to verify the algorithm effect. An offshore More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 335-349, 2025, DOI:10.32604/cmes.2025.062228 - 11 April 2025
Abstract This paper presents an allowable-tolerance-based group search optimization (AT-GSO), which combines the robust GSO (R-GSO) and the external quality design planning of the Taguchi method. AT-GSO algorithm is used to optimize the heat transfer area of the heat exchanger system. The R-GSO algorithm integrates the GSO algorithm with the Taguchi method, utilizing the Taguchi method to determine the optimal producer in each iteration of the GSO algorithm to strengthen the robustness of the search process and the ability to find the global optima. In conventional parameter design optimization, it is typically assumed that the designed… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 351-371, 2025, DOI:10.32604/cmes.2025.063196 - 11 April 2025
Abstract Maximizing the efficiency of thermal engineering equipment involves minimizing entropy generation, which arises from irreversible processes. This study examines thermal transport and entropy generation in viscous flow over a radially stretching disk, incorporating the effects of magnetohydrodynamics (MHD), viscous dissipation, Joule heating, and radiation. Similarity transformations are used to obtain dimensionless nonlinear ordinary differential equations (ODEs) from the governing coupled partial differential equations (PDEs). The converted equations are then solved by using the BVP4C solver in MATLAB. To validate the findings, the results are compared with previously published studies under fixed parameter conditions, demonstrating strong… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 373-411, 2025, DOI:10.32604/cmes.2025.062837 - 11 April 2025
Abstract Visual question answering (VQA) is a multimodal task, involving a deep understanding of the image scene and the question’s meaning and capturing the relevant correlations between both modalities to infer the appropriate answer. In this paper, we propose a VQA system intended to answer yes/no questions about real-world images, in Arabic. To support a robust VQA system, we work in two directions: (1) Using deep neural networks to semantically represent the given image and question in a fine-grained manner, namely ResNet-152 and Gated Recurrent Units (GRU). (2) Studying the role of the utilized multimodal bilinear… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 413-448, 2025, DOI:10.32604/cmes.2025.059406 - 11 April 2025
Abstract Thyroid nodules, a common disorder in the endocrine system, require accurate segmentation in ultrasound images for effective diagnosis and treatment. However, achieving precise segmentation remains a challenge due to various factors, including scattering noise, low contrast, and limited resolution in ultrasound images. Although existing segmentation models have made progress, they still suffer from several limitations, such as high error rates, low generalizability, overfitting, limited feature learning capability, etc. To address these challenges, this paper proposes a Multi-level Relation Transformer-based U-Net (MLRT-UNet) to improve thyroid nodule segmentation. The MLRT-UNet leverages a novel Relation Transformer, which processes… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 449-476, 2025, DOI:10.32604/cmes.2025.061635 - 11 April 2025
(This article belongs to the Special Issue: Advances in Mathematical Modeling: Numerical Approaches and Simulation for Computational Biology)
Abstract Streptococcus suis (S. suis) is a major disease impacting pig farming globally. It can also be transferred to humans by eating raw pork. A comprehensive study was recently carried out to determine the indices through multiple geographic regions in China. Methods: The well-posed theorems were employed to conduct a thorough analysis of the model’s feasible features, including positivity, boundedness equilibria, reproduction number, and parameter sensitivity. Stochastic Euler, Runge Kutta, and Euler Maruyama are some of the numerical techniques used to replicate the behavior of the streptococcus suis infection in the pig population. However, the dynamic… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 477-498, 2025, DOI:10.32604/cmes.2025.061339 - 11 April 2025
(This article belongs to the Special Issue: Applied Artificial Intelligence: Advanced Solutions for Engineering Real-World Challenges)
Abstract In robotics and human-robot interaction, a robot’s capacity to express and react correctly to human emotions is essential. A significant aspect of the capability involves controlling the robotic facial skin actuators in a way that resonates with human emotions. This research focuses on human anthropometric theories to design and control robotic facial actuators, addressing the limitations of existing approaches in expressing emotions naturally and accurately. The facial landmarks are extracted to determine the anthropometric indicators for designing the robot head and is employed to the displacement of these points to calculate emotional values using Fuzzy… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 499-513, 2025, DOI:10.32604/cmes.2025.061804 - 11 April 2025
(This article belongs to the Special Issue: Innovative Computational Methods and Applications of Nanofluids in Engineering)
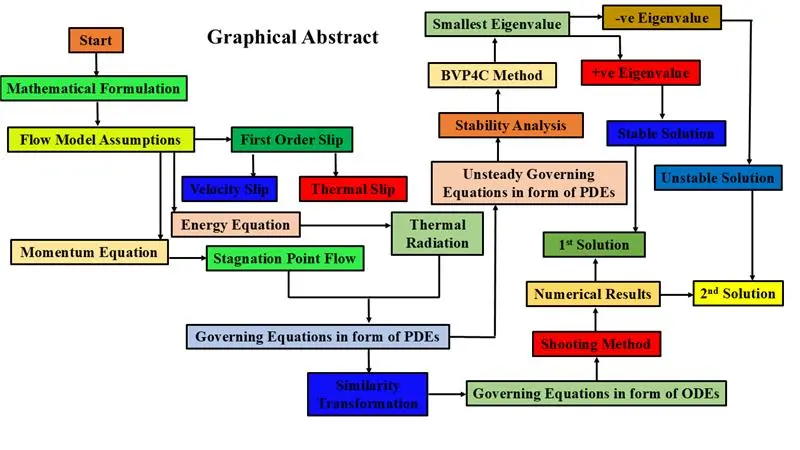
Abstract This paper discusses the model of the boundary layer (BL) flow and the heat transfer characteristics of hybrid nanofluid (HNF) over shrinking/stretching disks. In addition, the thermal radiation and the impact of velocity and thermal slip boundary conditions are also examined. The considered hybrid nano-fluid contains silver (Ag) and iron oxide (Fe3O4) nanoparticles dispersed in the water to prepare the Ag-Fe3O4/water-based hybrid nanofluid. The requisite posited partial differential equations model is converted to ordinary differential equations using similarity transformations. For a numerical solution, the shooting method in Maple is employed. Moreover, the duality in solutions is… More >
Graphic Abstract
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 515-538, 2025, DOI:10.32604/cmes.2025.061897 - 11 April 2025
(This article belongs to the Special Issue: Theoretical and Computational Modeling of Advanced Materials and Structures-II)
Abstract The nonlinear post-buckling response of functionally graded (FG) copper matrix plates enforced by graphene origami auxetic metamaterials (GOAMs) is investigated in the current work. The auxetic material properties of the plate are controlled by graphene content and the degree of origami folding, which are graded across the thickness of the plate. The material properties of the GOAM plate are evaluated using genetic micro-mechanical models. Governing nonlinear eigenvalue problems for the post-buckling response of the GOAM composite plate are derived using the virtual work principle and a four-variable nonlinear shear deformation theory. A novel differential quadrature More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 539-567, 2025, DOI:10.32604/cmes.2025.062301 - 11 April 2025
(This article belongs to the Special Issue: Advances in AI-Driven Computational Modeling for Image Processing)
Abstract Glaucoma, a leading cause of blindness, demands early detection for effective management. While AI-based diagnostic systems are gaining traction, their performance is often limited by challenges such as varying image backgrounds, pixel intensity inconsistencies, and object size variations. To address these limitations, we introduce an innovative, nature-inspired machine learning framework combining feature excitation-based dense segmentation networks (FEDS-Net) and an enhanced gray wolf optimization-supported support vector machine (IGWO-SVM). This dual-stage approach begins with FEDS-Net, which utilizes a fuzzy integral (FI) technique to accurately segment the optic cup (OC) and optic disk (OD) from retinal images, even More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 569-592, 2025, DOI:10.32604/cmes.2025.060484 - 11 April 2025
(This article belongs to the Special Issue: Advances in AI-Driven Computational Modeling for Image Processing)
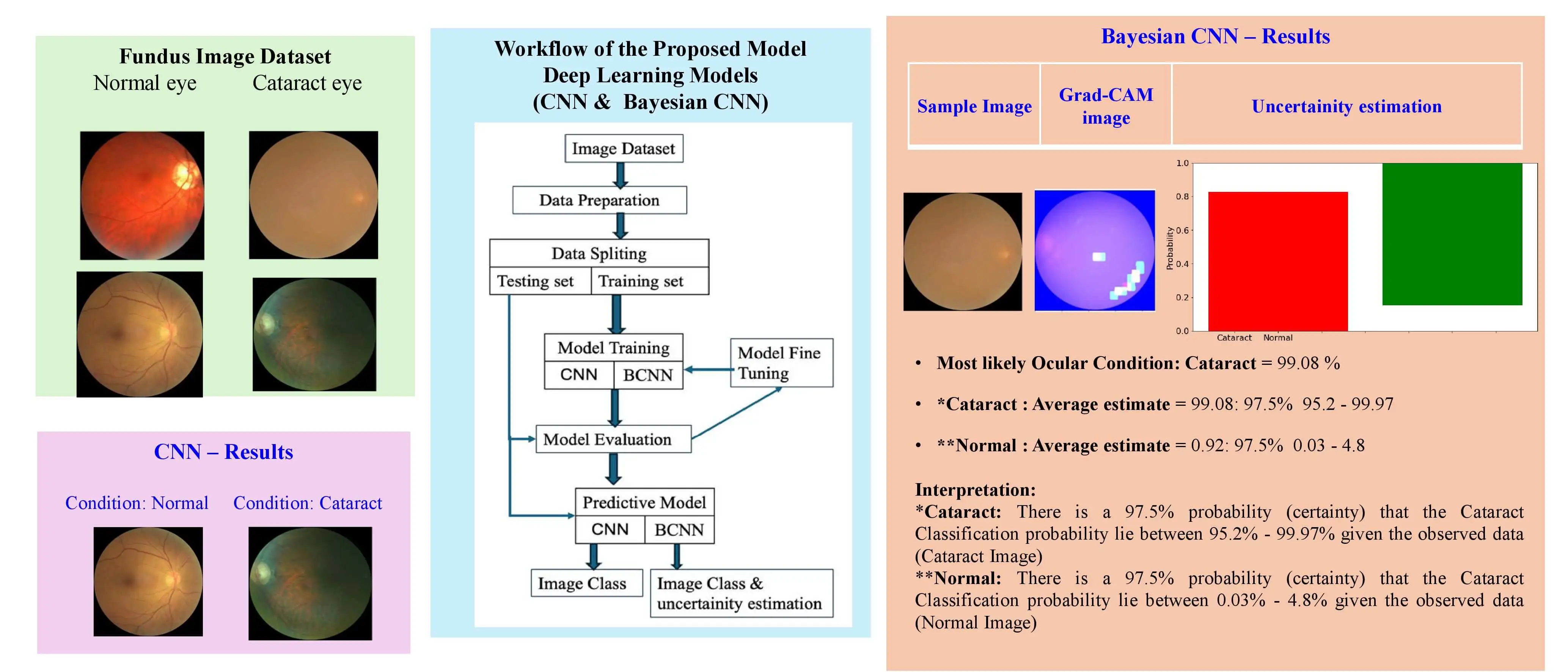
Abstract The effective and timely diagnosis and treatment of ocular diseases are key to the rapid recovery of patients. Today, the mass disease that needs attention in this context is cataracts. Although deep learning has significantly advanced the analysis of ocular disease images, there is a need for a probabilistic model to generate the distributions of potential outcomes and thus make decisions related to uncertainty quantification. Therefore, this study implements a Bayesian Convolutional Neural Networks (BCNN) model for predicting cataracts by assigning probability values to the predictions. It prepares convolutional neural network (CNN) and BCNN models. More >
Graphic Abstract
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 593-616, 2025, DOI:10.32604/cmes.2025.062264 - 11 April 2025
(This article belongs to the Special Issue: Advances in AI-Driven Computational Modeling for Image Processing)
Abstract Recent years have seen a surge in interest in object detection on remote sensing images for applications such as surveillance and management. However, challenges like small object detection, scale variation, and the presence of closely packed objects in these images hinder accurate detection. Additionally, the motion blur effect further complicates the identification of such objects. To address these issues, we propose enhanced YOLOv9 with a transformer head (YOLOv9-TH). The model introduces an additional prediction head for detecting objects of varying sizes and swaps the original prediction heads for transformer heads to leverage self-attention mechanisms. We… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 617-637, 2025, DOI:10.32604/cmes.2025.061242 - 11 April 2025
(This article belongs to the Special Issue: Incomplete Data Test, Analysis and Fusion Under Complex Environments)
Abstract A Bayesian network reconstruction method based on norm minimization is proposed to address the sparsity and iterative divergence issues in network reconstruction caused by noise and missing values. This method achieves precise adjustment of the network structure by constructing a preliminary random network model and introducing small-world network characteristics and combines L1 norm minimization regularization techniques to control model complexity and optimize the inference process of variable dependencies. In the experiment of game network reconstruction, when the success rate of the L1 norm minimization model’s existence connection reconstruction reaches 100%, the minimum data required is… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 639-671, 2025, DOI:10.32604/cmes.2025.062788 - 11 April 2025
(This article belongs to the Special Issue: Leveraging AI and ML for QoS Improvement in Intelligent Programmable Networks)
Abstract Intrusion attempts against Internet of Things (IoT) devices have significantly increased in the last few years. These devices are now easy targets for hackers because of their built-in security flaws. Combining a Self-Organizing Map (SOM) hybrid anomaly detection system for dimensionality reduction with the inherited nature of clustering and Extreme Gradient Boosting (XGBoost) for multi-class classification can improve network traffic intrusion detection. The proposed model is evaluated on the NSL-KDD dataset. The hybrid approach outperforms the baseline line models, Multilayer perceptron model, and SOM-KNN (k-nearest neighbors) model in precision, recall, and F1-score, highlighting the proposed More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 673-721, 2025, DOI:10.32604/cmes.2025.061699 - 11 April 2025
(This article belongs to the Special Issue: Computational Intelligent Systems for Solving Complex Engineering Problems: Principles and Applications-II)
Abstract This study examines various issues arising in three-phase unbalanced power distribution networks (PDNs) using a comprehensive optimization approach. With the integration of renewable energy sources, increasing energy demands, and the adoption of smart grid technologies, power systems are undergoing a rapid transformation, making the need for efficient, reliable, and sustainable distribution networks increasingly critical. In this paper, the reconfiguration problem in a 37-bus unbalanced PDN test system is solved using five different popular metaheuristic algorithms. Among these advanced search algorithms, the Bonobo Optimizer (BO) has demonstrated superior performance in handling the complexities of unbalanced power… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 723-745, 2025, DOI:10.32604/cmes.2025.059625 - 11 April 2025
(This article belongs to the Special Issue: Computational Intelligent Systems for Solving Complex Engineering Problems: Principles and Applications-II)
Abstract Promoting the high penetration of renewable energies like photovoltaic (PV) systems has become an urgent issue for expanding modern power grids and has accomplished several challenges compared to existing distribution grids. This study measures the effectiveness of the Puma optimizer (PO) algorithm in parameter estimation of PSC (perovskite solar cells) dynamic models with hysteresis consideration considering the electric field effects on operation. The models used in this study will incorporate hysteresis effects to capture the time-dependent behavior of PSCs accurately. The PO optimizes the proposed modified triple diode model (TDM) with a variable voltage capacitor… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 747-766, 2025, DOI:10.32604/cmes.2025.062390 - 11 April 2025
(This article belongs to the Special Issue: Computational Intelligent Systems for Solving Complex Engineering Problems: Principles and Applications-II)
Abstract In contemporary geotechnical projects, various approaches are employed for forecasting the settlement of shallow foundations (Sm). However, achieving precise modeling of foundation behavior using certain techniques (such as analytical, numerical, and regression) is challenging and sometimes unattainable. This is primarily due to the inherent nonlinearity of the model, the intricate nature of geotechnical materials, the complex interaction between soil and foundation, and the inherent uncertainty in soil parameters. Therefore, these methods often introduce assumptions and simplifications, resulting in relationships that deviate from the actual problem’s reality. In addition, many of these methods demand significant investments of… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 767-808, 2025, DOI:10.32604/cmes.2025.061763 - 11 April 2025
(This article belongs to the Special Issue: Machine Learning-Assisted Structural Integrity Assessment and Design Optimization under Uncertainty)
Abstract The traditional first-order reliability method (FORM) often encounters challenges with non-convergence of results or excessive calculation when analyzing complex engineering problems. To improve the global convergence speed of structural reliability analysis, an improved coati optimization algorithm (COA) is proposed in this paper. In this study, the social learning strategy is used to improve the coati optimization algorithm (SL-COA), which improves the convergence speed and robustness of the new heuristic optimization algorithm. Then, the SL-COA is compared with the latest heuristic optimization algorithms such as the original COA, whale optimization algorithm (WOA), and osprey optimization algorithm… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 809-826, 2025, DOI:10.32604/cmes.2025.059700 - 11 April 2025
(This article belongs to the Special Issue: Analytical and Numerical Solution of the Fractional Differential Equation)
Abstract Fractional discrete systems can enable the modeling and control of the complicated processes more adaptable through the concept of versatility by providing system dynamics’ descriptions with more degrees of freedom. Numerical approaches have become necessary and sufficient to be addressed and employed for benefiting from the adaptability of such systems for varied applications. A variety of fractional Layla and Majnun model (LMM) system kinds has been proposed in the current work where some of these systems’ key behaviors are addressed. In addition, the necessary and sufficient conditions for the stability and asymptotic stability of the… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 827-847, 2025, DOI:10.32604/cmes.2025.061178 - 11 April 2025
(This article belongs to the Special Issue: Machine learning and Blockchain for AIoT: Robustness, Privacy, Trust and Security)
Abstract Integrating Artificial Intelligence of Things (AIoT) in healthcare offers transformative potential for real-time diagnostics and collaborative learning but presents critical challenges, including privacy preservation, computational efficiency, and regulatory compliance. Traditional approaches, such as differential privacy, homomorphic encryption, and secure multi-party computation, often fail to balance performance and privacy, rendering them unsuitable for resource-constrained healthcare AIoT environments. This paper introduces LMSA (Lightweight Multi-Key Secure Aggregation), a novel framework designed to address these challenges and enable efficient, secure federated learning across distributed healthcare institutions. LMSA incorporates three key innovations: (1) a lightweight multi-key management system leveraging Diffie-Hellman… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 849-873, 2025, DOI:10.32604/cmes.2025.062549 - 11 April 2025
(This article belongs to the Special Issue: Machine learning and Blockchain for AIoT: Robustness, Privacy, Trust and Security)
Abstract The Internet of Things (IoT) is emerging as an innovative phenomenon concerned with the development of numerous vital applications. With the development of IoT devices, huge amounts of information, including users’ private data, are generated. IoT systems face major security and data privacy challenges owing to their integral features such as scalability, resource constraints, and heterogeneity. These challenges are intensified by the fact that IoT technology frequently gathers and conveys complex data, creating an attractive opportunity for cyberattacks. To address these challenges, artificial intelligence (AI) techniques, such as machine learning (ML) and deep learning (DL),… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 875-909, 2025, DOI:10.32604/cmes.2025.061623 - 11 April 2025
(This article belongs to the Special Issue: Advanced Computational Intelligence Techniques, Uncertain Knowledge Processing and Multi-Attribute Group Decision-Making Methods Applied in Modeling of Medical Diagnosis and Prognosis)
Abstract Heart disease prediction is a critical issue in healthcare, where accurate early diagnosis can save lives and reduce healthcare costs. The problem is inherently complex due to the high dimensionality of medical data, irrelevant or redundant features, and the variability in risk factors such as age, lifestyle, and medical history. These challenges often lead to inefficient and less accurate models. Traditional prediction methodologies face limitations in effectively handling large feature sets and optimizing classification performance, which can result in overfitting poor generalization, and high computational cost. This work proposes a novel classification model for heart… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 911-943, 2025, DOI:10.32604/cmes.2025.062524 - 11 April 2025
(This article belongs to the Special Issue: Algorithms, Models, and Applications of Fuzzy Optimization and Decision Making)
Abstract This paper introduces fuzzy N-bipolar soft (FN-BS) sets, a novel mathematical framework designed to enhance multi-criteria decision-making (MCDM) processes under uncertainty. The study addresses a significant limitation in existing models by unifying fuzzy logic, the consideration of bipolarity, and the ability to evaluate attributes on a multinary scale. The specific contributions of the FN-BS framework include: (1) a formal definition and set-theoretic foundation, (2) the development of two innovative algorithms for solving decision-making (DM) problems, and (3) a comparative analysis demonstrating its superiority over established models. The proposed framework is applied to a real-world case More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 945-965, 2025, DOI:10.32604/cmes.2025.062315 - 11 April 2025
(This article belongs to the Special Issue: Advances in Deep Learning for Time Series Forecasting: Research and Applications)
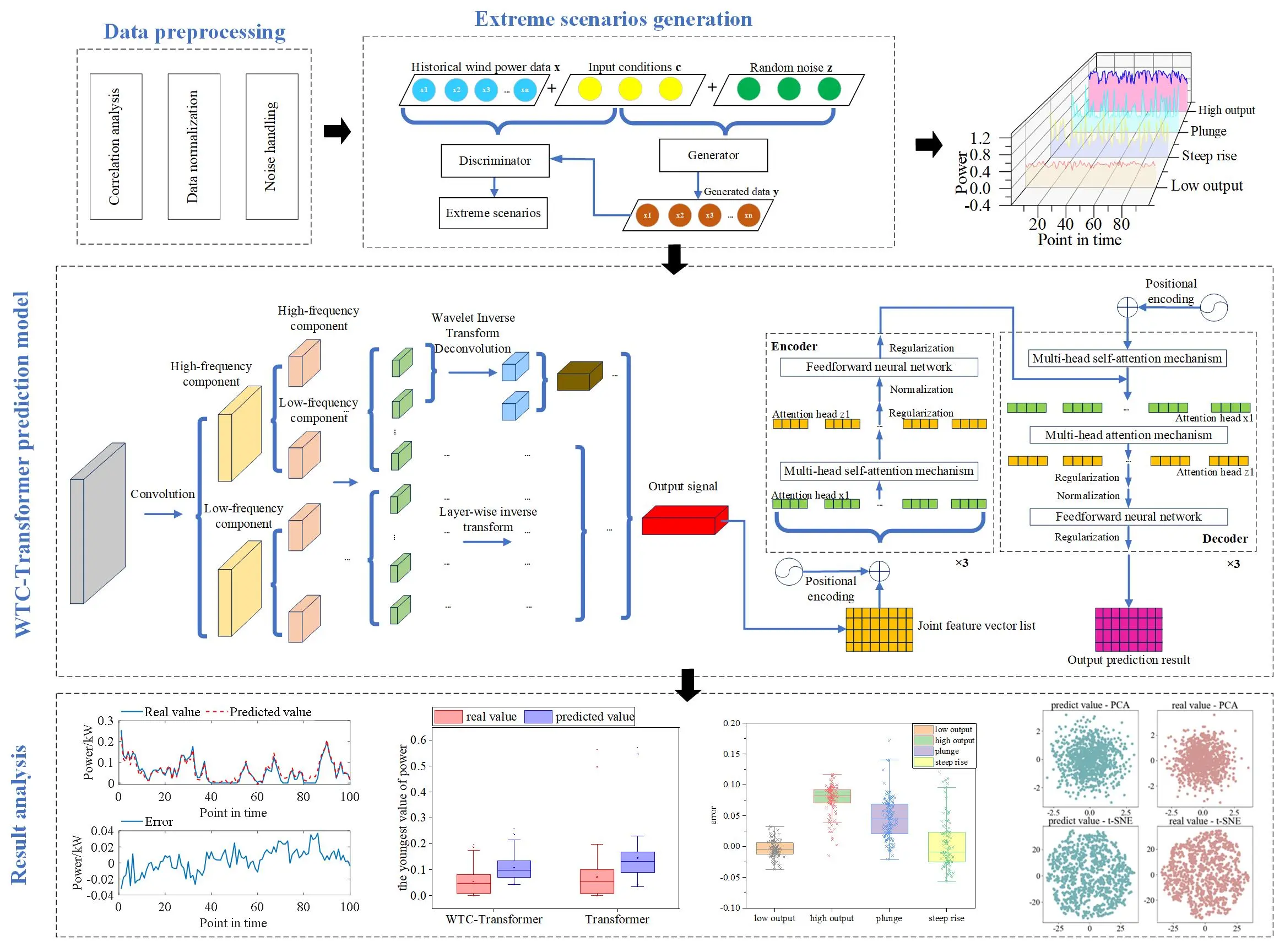
Abstract Wind power generation is subjected to complex and variable meteorological conditions, resulting in intermittent and volatile power generation. Accurate wind power prediction plays a crucial role in enabling the power grid dispatching departments to rationally plan power transmission and energy storage operations. This enhances the efficiency of wind power integration into the grid. It allows grid operators to anticipate and mitigate the impact of wind power fluctuations, significantly improving the resilience of wind farms and the overall power grid. Furthermore, it assists wind farm operators in optimizing the management of power generation facilities and reducing… More >
Graphic Abstract
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 967-1008, 2025, DOI:10.32604/cmes.2025.062332 - 11 April 2025
(This article belongs to the Special Issue: Swarm and Metaheuristic Optimization for Applied Engineering Application)
Abstract Multi-objective optimization is critical for problem-solving in engineering, economics, and AI. This study introduces the Multi-Objective Chef-Based Optimization Algorithm (MOCBOA), an upgraded version of the Chef-Based Optimization Algorithm (CBOA) that addresses distinct objectives. Our approach is unique in systematically examining four dominance relations—Pareto, Epsilon, Cone-epsilon, and Strengthened dominance—to evaluate their influence on sustaining solution variety and driving convergence toward the Pareto front. Our comparison investigation, which was conducted on fifty test problems from the CEC 2021 benchmark and applied to areas such as chemical engineering, mechanical design, and power systems, reveals that the dominance approach More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1009-1027, 2025, DOI:10.32604/cmes.2025.058352 - 11 April 2025
(This article belongs to the Special Issue: Emerging Technologies in Information Security )
Abstract In the current digital era, new technologies are becoming an essential part of our lives. Consequently, the number of malicious software or malware attacks is rapidly growing. There is no doubt, the majority of malware attacks can be detected by most antivirus programs. However, such types of antivirus programs are one step behind malicious software. Due to these dilemmas, deep learning become popular in the detection and classification of malicious data. Therefore, researchers have significantly focused on finding solutions for malware attacks by analyzing malicious samples with the help of different techniques and models. In More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1029-1048, 2025, DOI:10.32604/cmes.2025.061787 - 11 April 2025
(This article belongs to the Special Issue: Emerging Technologies in Information Security )
Abstract Data compression plays a vital role in data management and information theory by reducing redundancy. However, it lacks built-in security features such as secret keys or password-based access control, leaving sensitive data vulnerable to unauthorized access and misuse. With the exponential growth of digital data, robust security measures are essential. Data encryption, a widely used approach, ensures data confidentiality by making it unreadable and unalterable through secret key control. Despite their individual benefits, both require significant computational resources. Additionally, performing them separately for the same data increases complexity and processing time. Recognizing the need for More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1049-1070, 2025, DOI:10.32604/cmes.2025.063405 - 11 April 2025
(This article belongs to the Special Issue: Emerging Technologies in Information Security )
Abstract Satellite images are widely used for remote sensing and defence applications, however, they are subject to a variety of threats. To ensure the security and privacy of these images, they must be watermarked and encrypted before communication. Therefore, this paper proposes a novel watermarked satellite image encryption scheme based on chaos, Deoxyribonucleic Acid (DNA) sequence, and hash algorithm. The watermark image, DNA sequence, and plaintext image are passed through the Secure Hash Algorithm (SHA-512) to compute the initial condition (keys) for the Tangent-Delay Ellipse Reflecting Cavity Map (TD-ERCS), Henon, and Duffing chaotic maps, respectively. Through More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1071-1093, 2025, DOI:10.32604/cmes.2025.062902 - 11 April 2025
(This article belongs to the Special Issue: Emerging Technologies in Information Security )
Abstract Managing sensitive data in dynamic and high-stakes environments, such as healthcare, requires access control frameworks that offer real-time adaptability, scalability, and regulatory compliance. BIG-ABAC introduces a transformative approach to Attribute-Based Access Control (ABAC) by integrating real-time policy evaluation and contextual adaptation. Unlike traditional ABAC systems that rely on static policies, BIG-ABAC dynamically updates policies in response to evolving rules and real-time contextual attributes, ensuring precise and efficient access control. Leveraging decision trees evaluated in real-time, BIG-ABAC overcomes the limitations of conventional access control models, enabling seamless adaptation to complex, high-demand scenarios. The framework adheres to the… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1095-1128, 2025, DOI:10.32604/cmes.2025.058821 - 11 April 2025
(This article belongs to the Special Issue: Exploring the Impact of Artificial Intelligence on Healthcare: Insights into Data Management, Integration, and Ethical Considerations)
Abstract Predicting hospital readmission and length of stay (LOS) for diabetic patients is critical for improving healthcare quality, optimizing resource utilization, and reducing costs. This study leverages machine learning algorithms to predict 30-day readmission rates and LOS using a robust dataset comprising over 100,000 patient encounters from 130 hospitals collected over a decade. A comprehensive preprocessing pipeline, including feature selection, data transformation, and class balancing, was implemented to ensure data quality and enhance model performance. Exploratory analysis revealed key patterns, such as the influence of age and the number of diagnoses on readmission rates, guiding the More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1129-1155, 2025, DOI:10.32604/cmes.2025.063765 - 11 April 2025
(This article belongs to the Special Issue: Exploring the Impact of Artificial Intelligence on Healthcare: Insights into Data Management, Integration, and Ethical Considerations)
Abstract Cancer is a formidable and multifaceted disease driven by genetic aberrations and metabolic disruptions. Around 19% of cancer-related deaths worldwide are attributable to lung and colon cancer, which is also the top cause of death worldwide. The malignancy has a terrible 5-year survival rate of 19%. Early diagnosis is critical for improving treatment outcomes and survival rates. The study aims to create a computer-aided diagnosis (CAD) that accurately diagnoses lung disease by classifying histopathological images. It uses a publicly accessible dataset that includes 15,000 images of benign, malignant, and squamous cell carcinomas in the lung.… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1157-1184, 2025, DOI:10.32604/cmes.2025.062841 - 11 April 2025
(This article belongs to the Special Issue: Exploring the Impact of Artificial Intelligence on Healthcare: Insights into Data Management, Integration, and Ethical Considerations)
Abstract The Internet of Medical Things (IoMT) connects healthcare devices and sensors to the Internet, driving transformative advancements in healthcare delivery. However, expanding IoMT infrastructures face growing security threats, necessitating robust Intrusion Detection Systems (IDS). Maintaining the confidentiality of patient data is critical in AI-driven healthcare systems, especially when securing interconnected medical devices. This paper introduces SNN-IoMT (Stacked Neural Network Ensemble for IoMT Security), an AI-driven IDS framework designed to secure dynamic IoMT environments. Leveraging a stacked deep learning architecture combining Multi-Layer Perceptron (MLP), Convolutional Neural Networks (CNN), and Long Short-Term Memory (LSTM), the model optimizes data management More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1185-1214, 2025, DOI:10.32604/cmes.2025.064636 - 11 April 2025
(This article belongs to the Special Issue: Meta-heuristic Algorithms in Materials Science and Engineering)
Abstract Hybrid renewable energy systems (HRES) offer cost-effectiveness, low-emission power solutions, and reduced dependence on fossil fuels. However, the renewable energy allocation problem remains challenging due to complex system interactions and multiple operational constraints. This study develops a novel Multi-Neighborhood Enhanced Harris Hawks Optimization (MNEHHO) algorithm to address the allocation of HRES components. The proposed approach integrates key technical parameters, including charge-discharge efficiency, storage device configurations, and renewable energy fraction. We formulate a comprehensive mathematical model that simultaneously minimizes levelized energy costs and pollutant emissions while maintaining system reliability. The MNEHHO algorithm employs multiple neighborhood structures… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1215-1248, 2025, DOI:10.32604/cmes.2025.063193 - 11 April 2025
(This article belongs to the Special Issue: Advanced Artificial Intelligence and Machine Learning Methods Applied to Energy Systems)
Abstract This research investigates the influence of indoor and outdoor factors on photovoltaic (PV) power generation at Utrecht University to accurately predict PV system performance by identifying critical impact factors and improving renewable energy efficiency. To predict plant efficiency, nineteen variables are analyzed, consisting of nine indoor photovoltaic panel characteristics (Open Circuit Voltage (Voc), Short Circuit Current (Isc), Maximum Power (Pmpp), Maximum Voltage (Umpp), Maximum Current (Impp), Filling Factor (FF), Parallel Resistance (Rp), Series Resistance (Rs), Module Temperature) and ten environmental factors (Air Temperature, Air Humidity, Dew Point, Air Pressure, Irradiation, Irradiation Propagation, Wind Speed, Wind… More >
 Open Access
Open Access
ARTICLE
CMES-Computer Modeling in Engineering & Sciences, Vol.143, No.1, pp. 1249-1272, 2025, DOI:10.32604/cmes.2025.062339 - 11 April 2025
(This article belongs to the Special Issue: Soft Computing Applications of Civil Engineering including AI-based Optimization and Prediction)
Abstract Accurate daily suspended sediment load (SSL) prediction is essential for sustainable water resource management, sediment control, and environmental planning. However, SSL prediction is highly complex due to its nonlinear and dynamic nature, making traditional empirical models inadequate. This study proposes a novel hybrid approach, integrating the Adaptive Neuro-Fuzzy Inference System (ANFIS) with the Gradient-Based Optimizer (GBO), to enhance SSL forecasting accuracy. The research compares the performance of ANFIS-GBO with three alternative models: standard ANFIS, ANFIS with Particle Swarm Optimization (ANFIS-PSO), and ANFIS with Grey Wolf Optimization (ANFIS-GWO). Historical SSL and streamflow data from the Bailong… More >