Intelligent Automation & Soft Computing DOI:10.32604/iasc.2021.017391
Article

Game-Theory Based Graded Diagnosis Strategies of Craniocerebral Injury

Yiming Liu1, Ke Chen1, Lanzhen Bian2, Lei Ren3, Jing Hu4,* and Jinyue Xia5

1Department of neurosurgery, Children’s Hospital of Nanjing Medical University, Nanjing, 210008, China
2Department of surgery, Children’s Hospital of Nanjing Medical University, Nanjing, 210008, China
3Department of Nursing, Children’s Hospital of Nanjing Medical University, Nanjing, 210008, China
4Department of rehabilitation medicine, Children’s Hospital of Nanjing Medical University, Nanjing, 210008, China
5International Business Machines Corporation (IBM), NY, USA
*Corresponding Author: Jing Hu. Email: njetyykfk@163.com
Received: 29 January 2021; Accepted: 03 March 2021

Abstract: Craniocerebral injury is a common surgical emergency in children. It has the highest mortality and disability rate, and the second highest incidence rate. Accidental injuries due to falls, sports and traffic accidents are the main causes of craniocerebral injury. In recent years, the incidence rate of craniocerebral injury in children has continued to rise, which injury stretches out the limited medical resources. Moreover, it is very difficult to deal with complex craniocerebral trauma in the hospital of county town, in which is not rich in medical resources because of the lack of experienced doctors and nurses. In addition, some children with mild craniocerebral injury go to tertiary hospitals directly, which takes up a lot of medical resources and leads to the waste of medical resources. To solve the problem, the Game Model is used to model the graded diagnosis and treatment strategies of craniocerebral injury in this paper. The results show that the diversion of some children who are identified as mild to moderate craniocerebral injury can increase the turnover of hospital beds in a tertiary hospital. Accordingly, the limited medical resources can be used to treat those children who are in critical conditions. In addition, the data also verifies the effectiveness of graded diagnosis and treatment strategies.

Keywords: Graded diagnosis; craniocerebral injury; game theory

1  Introduction

Craniocerebral injury is a mechanical injury caused by blunt force acting on the human brain [1, 2]. Accidental injuries caused by falls, sports and traffic accidents are the main causes of craniocerebral injury in children. Due to the anatomical and physiological characteristics of children at different stages of development, craniocerebral trauma has significantly different characteristics and its consequences from adults [3,4]. The Department of Neurosurgery of Nanjing Children’s Hospital affiliated to Nanjing Medical University, as a provincial and municipal clinical key specialty, provides its clinical experiences in the diagnosis and treatment of various diseases in pediatric neurosurgery, especially in craniocerebral injury. Every year, because the number of patients in various parts of the country reach tens of thousands, the utilization rate of the hospital beds is 100-120%. From January 2015 to June 2020, the total of craniocerebral trauma is 5476 cases. And the males were treated 3398 cases, the females were 2078. In our department, the children with craniocerebral trauma accounted for 60% of all children, in which it includes a large number of children with mild craniocerebral injury. Because all patients from mild to critical conditions were all treated on the same ward with limited beds, the children with mild craniocerebral injury occupy a lot of limited medical resources. This had not only increased the cost of environmental control for the ward, such as more experienced doctors and nurses are needed, but also increased the risk of cross-infection in children because of the children's running. Among all admitted children with craniocerebral injury, mild and medium craniocerebral injury are the main diagnosed condition. Children with mild craniocerebral injury can mostly improve by themselves after observation and symptomatic treatments. Most hospitalized patients can recover after treatments, and less than ten percentage of admitted patients have poor prognosis and even death [5–7].

Therefore, how to allocate the limited medical resources in tertiary hospitals becomes significant to critically ill children who may require immediate and urgent treatments more than non-critically ill children. We use the Game Theory to model the graded diagnosis and treatment strategies of craniocerebral injury and hypothesize the research question that triaging children with mild and medium craniocerebral injury can improve the turnover of hospital beds in a level-3 hospital.

The roadmap of this paper is organized as follows. Section 2 introduces the knowledge of game theory and craniocerebral injury. Section 3 presents the status and analysis of the problem. In Section 4, the grading diagnosis strategy of craniocerebral injury based on game theory is analyzed. Finally, the conclusion is given in Section 5.

2  Related Works

2.1 Game Theory

Game Theory first appeared in microeconomics. The concept of Nash equilibrium was first proposed in 1951, demonstrating that one or more Nash equilibria can be found in any finite non-cooperative game [8–10].

Game Theory is an incentive mechanism that applies mathematical theory to simulate and analyze. Each participant tries to choose the best strategy for himself according to the strategy chosen by other participants to obtain more benefits [11–13]. In a cooperative game, the model strategy, once selected, will not be changed again. In contrast, nodes in non-cooperative games can change their strategies at any time to maximize their gains. The participants in a single-stage game can only interact once, while participants in a repeated game can interact multiple times [14–16]. Repeating games can be composed of finite and infinite repeating games. In a finite repetition game, the number of interactions between participants is limited, while in an infinite repetition game, participants can think that there is always an expectation of interaction.

In recent years, the relevant knowledge of Game Theory is often used to solve practical problems in all walks of life. Nash Equilibrium is an important milestone in the development of game theory [17–20]. In the process of game, rational participants choose the strategy to maximize their own benefits through the behavior of other participants, and finally obtain Nash Equilibrium [21–25]. Game Theory provides the corresponding analysis tools to predict the behavior of rational nodes, and these rational entities obtain the maximum benefit by choosing the corresponding strategies.

2.2 Craniocerebral Injury

Craniocerebral injury is a mechanical injury caused by blunt force acting on human brain. Blunt objects range from bare hands to household goods and production tools [26–30]. Generalized blunt force injuries include stick injuries, masonry injuries, axe-hammer injuries, bare-handed injuries, high-drop injuries, crush injuries, traffic injuries, etc. The forms of injury can be divided into three types [31–36]: the moving object hitting the stationary body, such as the moving wound strikes the stationary human body; the moving human body strikes the stationary object, such as the high fall injury, or the secondary collision between the human body and the ground after the automobile collision; an injury caused by movement collides with a moving human body, such as a speeding car hitting pedestrians. Craniocerebral injury accounted for 34% of total body injuries, but brain injuries accounted for 68% of all injury deaths. Every year in China, about 600,000 people suffer from craniocerebral trauma, of which about 100,000 die, resulting in direct and indirect economic losses of up to 10 billion RMB. The surviving patients also often have more serious sequela, bringing a huge burden to the family and society.

3  Choice of Medical Institutions

At present, various resources of large cities are rich. And there are many tertiary hospitals with rich experience of doctors and nurses in large cities. Therefore, patients will concentrate on the tertiary hospitals in big cities, and less to secondary and first-class hospitals [37–40]. This situation is shown in the Fig. 1. This has led to a waste of resources at tertiary hospitals to treat children with mild and moderate symptoms. And the tertiary hospitals cannot concentrate medical resources to treat critically ill children. Moreover, the medical resources of first-level and second-level medical institutions are idle. And the doctors and nurses have no opportunity to participate in more treatment work, which results in low professional ability.

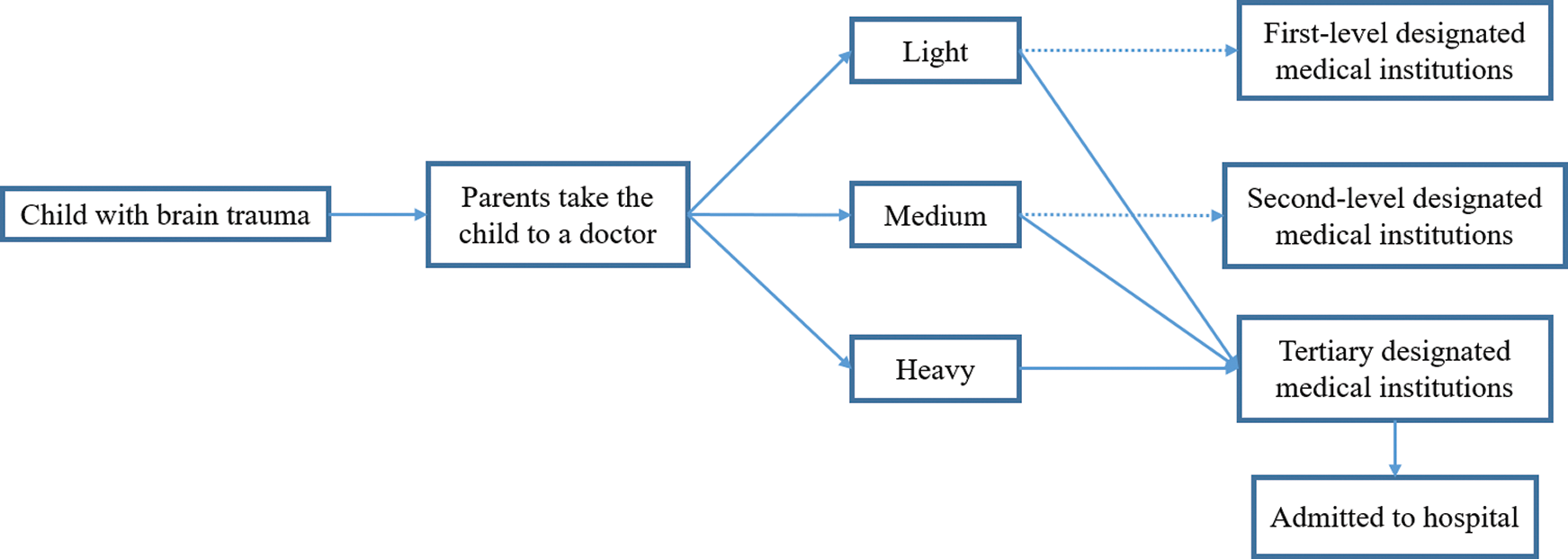
Figure 1: Selected medical institutions by patients without classified diagnosis and treatment

In an ideal state, a grading diagnosis and treatment strategy should be adopted, in order to solve the problem of excessive waste of resources in tertiary hospitals in big cities. Children with mild symptoms directly enter the first-level medical institutions, the children with moderate symptoms enter the second-level medical institutions, and only severe children enter the tertiary medical institutions. This situation is shown in the Fig. 2. This can make the doctors and nurses of the first-level and second-level medical institutions not only participate in the medical work of children, but also can improve the technical level. More importantly, it can reduce the burden of tertiary medical institutions, especially for the treatment of critically ill children. Because children want to get the treatment of tertiary hospitals, so in this case, the game between patients and medical institutions is formed. Thus, this paper uses game theory to analyze this situation, in order to analyze the effect of grading diagnosis and treatment.

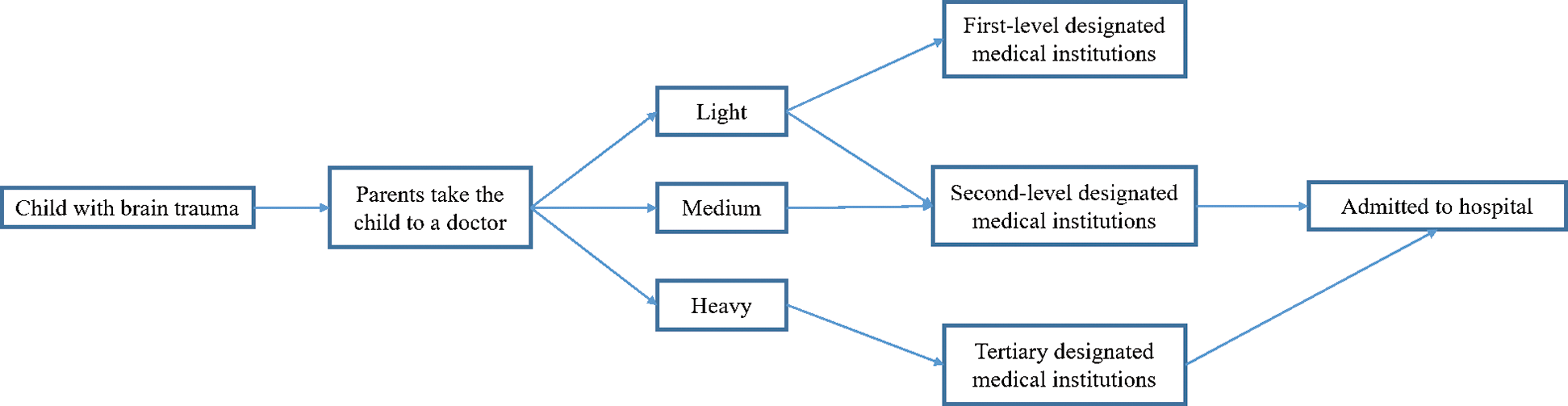
Figure 2: Selected medical institutions by patients with classified diagnosis and treatment

4  Grading Diagnosis Strategy of Craniocerebral Injury Based on Game Theory

4.1 Assumption Based on Game Theory

For the establishment of the game model, we first make the following basic assumptions.

Assumption 1: Participants in the game are the upper-level children's specialist hospital, the lower-level hospital’s pediatric department or children's specialist hospital, and the child patient.

Assumption 2: Each participant in the game has two choices: the upper-level children's specialist hospital chooses "receiving the child patient" or "recommends transfer the child patient to the lower-level hospital’s pediatric department or children’s specialist hospital to get treatment". The lower-level hospital’s pediatric department or children’s specialist hospital chooses "receiving the child patient" or "recommends transfer the child patient to the upper-level children’s specialist hospital to get treatment", the child patients’ parents choose" go to the lower-level hospital’s pediatric department or children's specialist hospital" or "go to the upper-level children's specialist hospital".

Assumption 3: The rate of the upper-level children’s specialist hospital chooses "receiving the child patient" is x(0<x<1) ; the rate of the lower-level hospital’s pediatric department or children’s specialist hospital chooses "receiving the child patient" is y(0<y<1) ; the rate of child patients’ parents choose "go to the lower-level hospital’s pediatric department or children’s specialist hospital" is z(0<z<1) ; and the rate of child patients above the heavy type is m(0<m<1) .

Assumption 4: The upper-level children's specialist hospital chooses "receiving children", set the cost of treating child patients above the heavy type as L1 , set the cost of treating child patients with light and medium type as L2 ; the lower-level hospital’s pediatric department or children's specialist hospital chooses "receiving children", set the cost of treating child patients with light and medium type as D1 , set the cost of treating child patients above heavy type (and cannot be completely cured) as D2 . When child patients above heavy type first get treatment in the lower-level hospital’s pediatric department or children's specialist hospital, then transferred to upper-level children’s specialist hospital to get treatment, set the increased cost of treatment for time delay as ε .

Assumption 5: If the upper-level children's specialist hospital chooses to "recommend transfer the child patient to the lower-level hospital’s pediatric department or children’s specialist hospital to get treatment", and the recommend child patient is above heavy type, set the medical resources saved by the upper-level children’s specialist hospital as L1 . If the recommend child patient is light and medium type, set the medical resources saved by the upper-level children’s specialist hospital as L2 . If the lower-level hospital’s pediatric department or children’s specialist hospital chooses "recommends transfer the child patient to the upper-level children's specialist hospital to get treatment", and the recommend child patient is above heavy type, set the medical resources saved by it as D1 ; otherwise, the recommend child patient is light and medium type, set the medical resources saved by it as D2 .

Assumption 6: If the upper-level children's specialist hospital chooses to "receive the child patient," for child patient with heavy-type above, set the increased Social evaluation as S1 ; for child patients with light and medium-type, set the increased Social evaluation as S2 . If the lower-level hospital’s pediatric department or children’s specialist hospital chooses to "receive the child patient,” and the cure is successful for child patients with heavy-type above, set the increased Social evaluation as I1 ; for child patients with light and medium-type, set the increased Social evaluation as I2 . When the child is successfully treated, set the cure for child patients with heavy-type above as T1 , set the cure for child patients with light and medium-type as T2 .

Assumption 7: When child patients above heavy-type are not treated or treated successfully, the social evaluation of the loss of the upper-level children's specialist hospital and lower-level hospital’s pediatric department or children’s specialist hospital are set as S1 and I1 , respectively. The loss of patient is set as T1 . When child patients with light and medium type are not treated or treated successfully, the social evaluation of the loss of the upper-level children's specialist hospital and lower-level hospital’s pediatric department or children’s specialist hospital are set as S2 and I2 , respectively. The loss of patient is set as T2 .

Assumption 8: Only consider the treatment process of a child patient in the early stages of symptoms, regardless of the transfer process in the later stages of his or her cure, and do not consider the transfer cost of "going to the lower-level hospital’s pediatric department or children's specialist hospital" and "going to the upper-level of children's specialist hospital".

4.2 Grading Diagnosis Strategy of Craniocerebral Injury Based on Game Theory

Based on the above assumptions, in the whole hospital system, the upper-level children's specialist hospital, the lower-level hospital’s pediatric department or children's specialist hospital and children make game combinations, game income statement shows in Tab. 1. There are eight combinations of this game strategy, namely (the lower-level hospital’s pediatric department or children's specialist hospital, treatment, treatment), (the lower-level hospital’s pediatric department or children's specialist hospital, treatment, recommendation), the lower-level hospital’s pediatric department or children's specialist hospital, recommendation, treatment), (the upper-level of children's specialist hospital, recommendation, recommendation), ([the upper-level of children's specialist hospital, treatment, treatment, the upper-level of children's specialist hospital, treatment, recommendation, the upper-level of children's specialist hospital, recommendation, treatment, the upper-level of children's specialist hospital, recommendation, recommendation]), in which child patients with light and medium type can go to the lower-level hospital’s pediatric department or children's specialist hospital and get treatment to be considered in the following two conditions, (the lower-level hospital’s pediatric department or children's specialist hospital, treatment, treatment), (the lower-level hospital’s pediatric department or children's specialist hospital, treatment, recommendations).

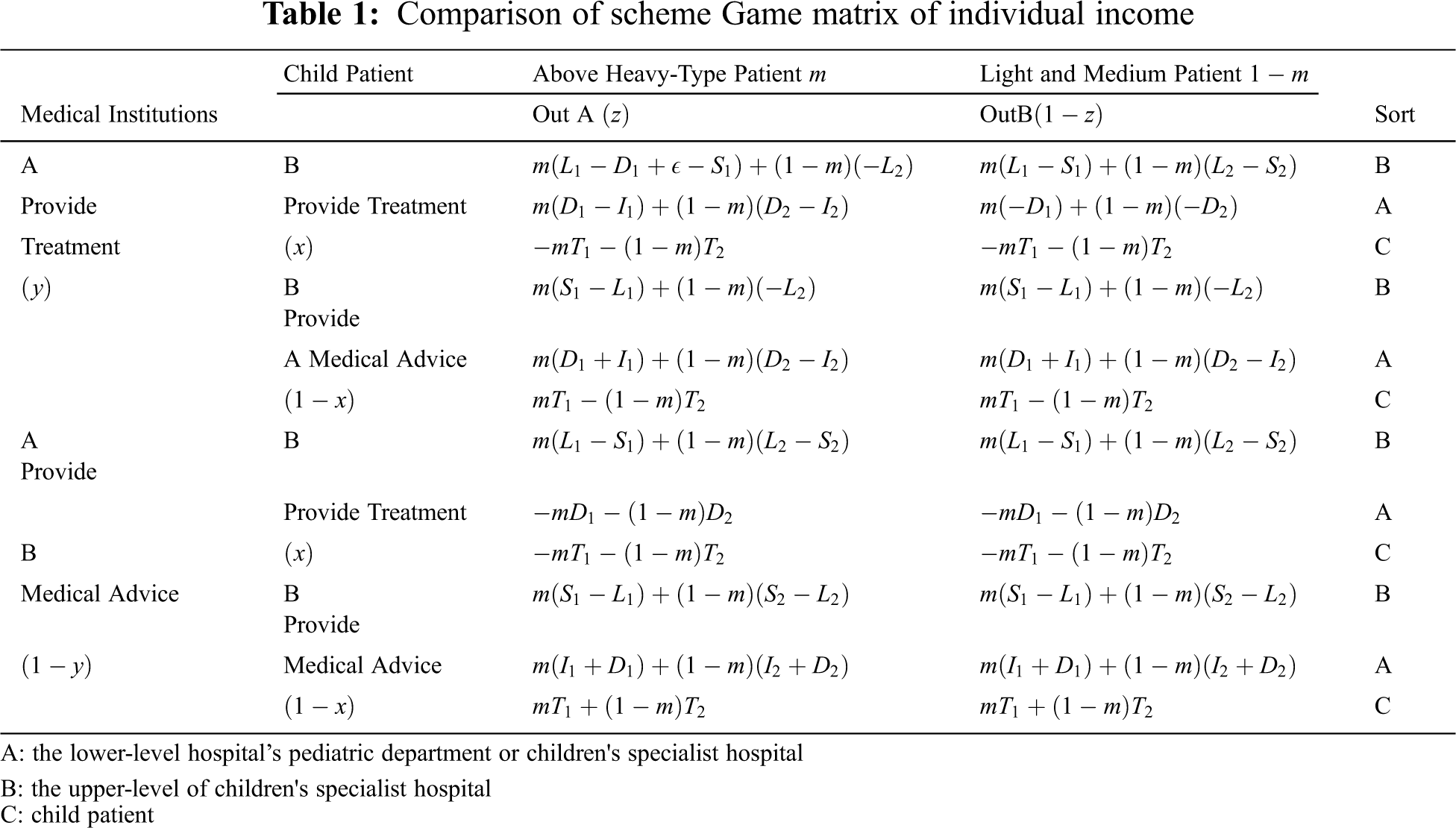
Replication dynamic analysis of the upper-level of children’s specialist hospital: according to Tab. 1, the expected and average benefits of the upper-level of children's specialist hospital provides treatment and provides lower-level hospital’s pediatric department or children's specialist hospital medical advice are G′,G′′ and G¯ , respectively.

G′=yz[m(L1−D1+ε−S1)+(1−m)(−L2)]+y(1−z)[m(L1−S1)+(1−m)(L2−S2)]+(1−y)z[m(L1−S1)+(1−m)(L2−S2)]+(1−y)(1−z)[m(L1−S1)+(1−m)(L2−S2)]

=yz[m(2L1−S2−D1+ε)+(S2−2L2)]+[m(L1−L2+S2−S1)+(L2−S2) (1)

G′′=yz[m(S1−L1)+(1−m)(−L2)]+y(1−z)[m(S1−L1)+(1−m)(−L2)]+(1−y)z[m(S1−L1)+(1−m)(S2−L2)]+(1−y)(1−z)[m(S1−L1)+(1−m)(S2−L2)]

=[m(S1−L1+L2)−L2]+(1−y)S2(1−m) (2)

G¯=xG′+(1−x)G′′ (3)

According to the above formulas, the game replication dynamic process of upper-level of children's specialist hospital as follows.

F(x)=dxdt=x(G′−G¯)=x(1−x){yz[m(2L1−S2−D1+ε)+(S2−2L2)] (4)

m∗=(L2+G1−G2−L1)/(S+L2−L1) (5)

4.3 Data Validation of Grading Diagnosis and Treatment of Craniocerebral Injury

According to the game model, our department performed triage and screening for hospitalized children. And the number of hospitalized children with craniocerebral injury has been distinctly decreasing since 2019, as shown in Fig. 3.

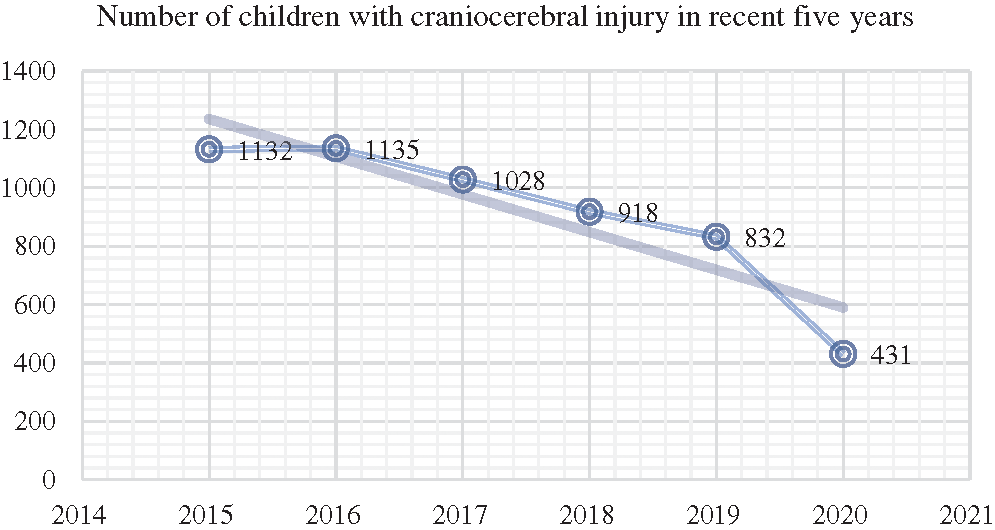
Figure 3: The number of children with craniocerebral injury in recent five years

In the Fig. 1, from January 1 to June 30, 2020, the number of hospitalized children with craniocerebral injury has decreased.

In the Fig. 4, the number of patients with craniocerebral injury in 2020 was counted from January 1 to June 30.

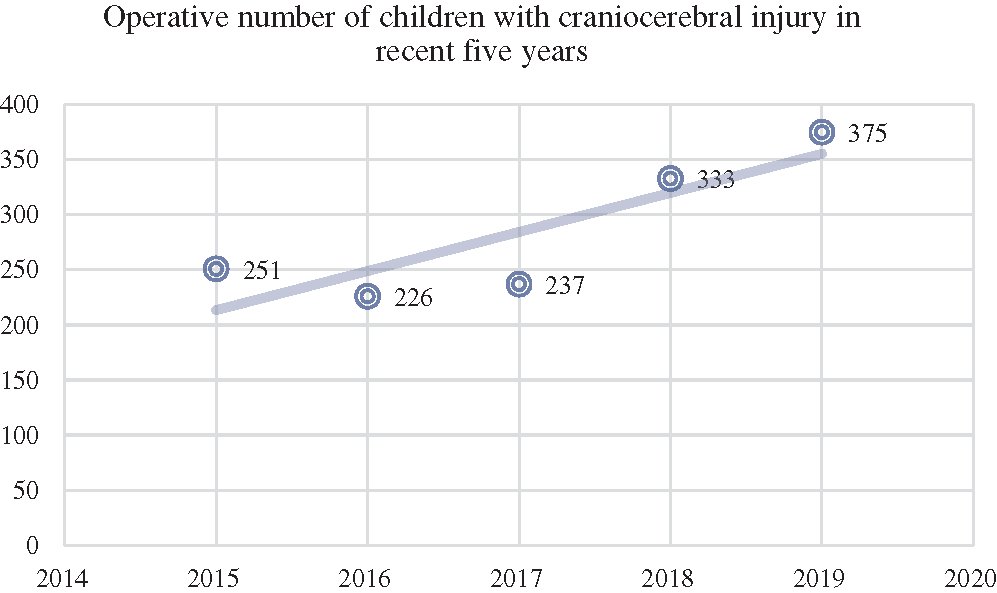
Figure 4: Operative number of children with craniocerebral injury in recent five years

As it can be seen from the above figure: although the number of children admitted to the department of neurosurgery in our hospital has decreased significantly, the number of children with craniocerebral injury undergoing surgery has increased year by year.

For children with craniocerebral injury, the general prognosis will be controlled as long as the treatments are in time and accurate. For the Doctor of Children’s specialty or children's hospital in the lower-level hospital, doctors can examine patients whether there is nervous system damage, and through physical examination and necessary auxiliary examination, the degree and location of the injury were further identified. For children with mild and moderate craniocerebral injury, they can be treated in hospitals. While children with severe craniocerebral injury should be treated in a upper-level specialized hospital immediately. On the other hand, if a upper-level children's hospital receives children with mild or moderate craniocerebral injury, they can also be transferred to the lower-level hospital when the diagnosis is clear and the condition is stable. Thus, it can not only ensure that the treatment of children is effective and continuous, but also timely can use the limited ward bed resources for children who need more beds.

This game will inevitably exist for a long time, which is related to the consciousness type of children's parents and the specialized knowledge level of doctors in primary and secondary hospitals. As a result, how to carry out effective health education for each guardian and reduce the incidence of craniocerebral injury, and how to deal with it timely and effectively after the occurrence of injury is a long and arduous task.

5  Conclusion

Optimization of medical resources in tertiary hospitals in China has always been a challenge. In this paper, the game model is adapted to model the graded diagnosis and treatment strategies of craniocerebral injury. Grading diagnosis and treatment can be classified according to the severity of the disease and the priority of diagnosis and treatment. The upper-level hospitals mainly focus on providing treatment for critical patients, while the lower-level medical institutions mainly deal with patients with mild illness. At the same time, it can also improve the lower-level medical institutions through the increase of cases treated, so as to better serve people's health. The results show training based on graded diagnosis and treatments is effective in improving hospital bed turnover rate.

Acknowledgement: We thank the director of nursing department in the children’s hospital of Nanjing medical university (Mei Li).

Funding Statement:This work is supported by science and technology development fund of Nanjing Medical University (Grand No. NMUB2018095). Yiming Liu received the grant and the URLs to sponsors’ websites is https://www.njmu.edu.cn. This study is also supported by Medical research projects of Jiangsu Health Commission (No. 2019178). Lei Ren received the grant and the URLs to sponsors’ websites is http://wjw.jiangsu.gov.cn/index.html.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study. Yiming Liu and Ke Chen are the co-first authors.

References

 1.  B. Jin, Y. Chen, M. Cao, J. Liu and M. Han, “Clinical features and risk factors of post-concussion syndromes after mild traumatic brain injury,” Chinese Journal of Neuromedicin, vol. 18, no. 6, pp. 570–574, 2019. [Google Scholar]

 2.  Z. Zhang, M. Saraswati and R. Koehler, “A new rabbit model of pediatric traumatic brain injury,” Neurotrauma, vol. 32, no. 17, pp. 1369–1379, 2017. [Google Scholar]

 3.  Z. Zhou, “Clinical characteristics and treatment of craniocerebral trauma in children,” Guide of China Medicine, vol. 16, no. 6, pp. 169–170, 2018. [Google Scholar]

 4.  J. Pace, N. Parry and K. Voge, “A clinical prediction model for raised intracranial pressure in patients with traumatic brain injuries,” Journal of Trauma and Acute Care Surgery, vol. 85, no. 2, pp. 380–386, 2018. [Google Scholar]

 5.  S. Neeg and A. Lee, “Traumatic brain injuries: pathophysiology and potential therapeutic targets,” Front Cell Neurosis, vol. 27, no. 13, pp. 528–538, 2019. [Google Scholar]

 6.  J. Dai, J. Cao, N. Le and Y. Ma, “Advance in experimental models of pediatric traumatic brain injury,” Chinese Journal of Pediatric Surgery, vol. 39, no. 1, pp. 71–75, 2018. [Google Scholar]

 7.  Y. Ren, J. Qi, Y. Cheng, J. Wang and O. Asama, “Digital continuity guarantee approach of electronic record based on data quality theory,” Computers, Materials & Continua, vol. 63, no. 3, pp. 1471–1483, 2020. [Google Scholar]

 8.  Z. Rong, X. Xu and Z. Wu, “Experiment research on the evolution of cooperation and network game theory,” Scientia Sinica (Physica Mechanica & Astronomica), vol. 45, no. 1, pp. 114–128, 2020. [Google Scholar]

 9.  E. Simhon and D. Starobiinski, “Advance reservation games,” ACM Transactions on Modeling and Performance Evaluation of Computing Systems, vol. 2, no. 2, pp. 1–21, 2017. [Google Scholar]

10. C. Ge, Z. Liu, J. Xia and L. Fang, “Revocable identity-based broadcast proxy re-encryption for data sharing in clouds,” IEEE Transactions on Dependable and Secure Computing, vol. 19, no. 3, pp. 1–12, 2019. [Google Scholar]

11. A. Kabir and T. Jun, “The role of advanced and late provisions in a co-evolutionary epidemic game model for assessing the social triple-dilemma aspect,” Journal of Theoretical Biology, vol. 503, no. 6, pp. 110399, 2020. [Google Scholar]

12. Y. Ren, Y. Leng, J. Qi, K. S. Pradip, J. Wang et al., “Multiple cloud storage mechanism based on blockchain in smart homes,” Future Generation Computer Systems, vol. 115, no. 3, pp. 304–313, 2021. [Google Scholar]

13. Y. Zhai, J. Liu, L. Liu and J. Chen, “Analysis of private cloud resource allocation management based on game theory in spatial data center,” Computer Science, vol. 47, no. 11, pp. 373–379, 2020. [Google Scholar]

14. Y. Peng, S. Zhang, H. Zhu and Q. Li, “D2D communication relay selection algorithm based on game theory,” Journal of Applied Sciences, vol. 38, no. 6, pp. 871–881, 2020. [Google Scholar]

15. G. Zhang and M. Zhang, “The stability of industry-university-research innovation alliance based on evolutionary game,” Operations Research and Management Science, vol. 29, no. 12, pp. 67–73, 2020. [Google Scholar]

16. L. Fang, C. Yin, L. Zhou, Y. Li, C. Su et al., “A physiological and behavioral feature authentication scheme for medical cloud based on fuzzy-rough core vector machine,” Information Sciences, vol. 507, no. 1, pp. 143–160, 2020. [Google Scholar]

17. L. Cheng and T. Yu, “Decision-making behavior investigation for general multi-strategy evolutionary games in the spontaneous formation of long-term bidding equilibria of a power generation market,” Proc. of The Chinese Society for Electrical Engineering, vol. 40, no. 21, pp. 6936–6955, 2020. [Google Scholar]

18. J. Hu, G. Hu, J. Cai, L. Xu and Q. Wang, “Hospital bed allocation strategy based on queuing theory during the COVID-19 epidemic,” Computers, Materials & Continua, vol. 66, no. 1, pp. 793–803, 2020. [Google Scholar]

19. J. Y. Ren, Y. Liu, S. Ji, A. K. Sangaiah and J. Wang, “Incentive mechanism of data storage based on blockchain for wireless sensor networks,” Mobile Information Systems, vol. 2018, no. 8, pp. 1–10, 2018. [Google Scholar]

20. W. Zhao, J. Liu, H. Guo and T. Hara, “ETC-IoT: edge-node-assisted transmitting for the cloud-centric internet of things,” IEEE Network, vol. 32, no. 3, pp. 101–107, 2018. [Google Scholar]

21. Y. Li and J. Feng, “Cooperative evolution under the direct sum game model,” Control Theory and Applications, vol. 37, no. 8, pp. 1717–1726, 2020. [Google Scholar]

22. T. Li, Y. Ren and J. Xia, “Blockchain queuing model with non-preemptive limited-priority,” Intelligent Automation & Soft Computing, vol. 26, no. 5, pp. 1111–1122, 2020. [Google Scholar]

23. J. Y. Ren, J. Qi, Y. Liu, J. Wang and G. Kim, “Integrity verification mechanism of sensor data based on bilinear map accumulator,” ACM Transactions on Internet Technology, vol. 21, no. 1, pp. 1–19, 2021. [Google Scholar]

24. X. Gu Jin Wang, W. Liu, A. K. Sangaiah and H. Kim, “An empower Hamilton loop based data collection algorithm with mobile agent for WSNs,” Human-Centric Computing and Information Sciences, vol. 18, no. 9, pp. 1–14, 2019. [Google Scholar]

25. Y. Ren, Y. Leng, Y. Cheng and J. Wang, “Secure data storage based on blockchain and coding in edge computing,” Mathematical Biosciences and Engineering, vol. 16, no. 4, pp. 1874–1892, 2019. [Google Scholar]

26. J. Mu, C. Ni, M. Wu, W. Fan, F. Xu et al., “Factors related to cognitive dysfunction after traumatic brain injury,” Chinese Journal of Physical Medicine and Rehabilitation, vol. 42, no. 6, pp. 500–504, 2020. [Google Scholar]

27. J. Wang, W. Chen, L. Wang, Y. Ren and R. S. Sherratt, “Blockchain-based data storage mechanism for industrial Internet of Things,” Intelligent Automation & Soft Computing, vol. 26, no. 5, pp. 1157–1172, 2020. [Google Scholar]

28. H. Wang and Z. Wang, “Analysis of influencing factors of cognitive impairment after moderate traumatic brain injury,” Chinese Journal of Neurosurgery, vol. 35, no. 11, pp. 1143–1147, 2019. [Google Scholar]

29. C. Ge, W. Susilo, Z. Liu, J. Xia, P. Szalachowski et al., “Secure keyword search and data sharing mechanism for cloud computing,” IEEE Transactions on Dependable and Secure Computing, vol. 20, no. 6, pp. 1–12, 2020. [Google Scholar]

30. L. Xiang, W. Wu, X. Li and C. Yang, “A linguistic steganography based on word indexing compression and candidate selection,” Multimedia Tools and Applications, vol. 77, no. 21, pp. 28969–28989, 2018. [Google Scholar]

31. J. Wang and J. Zhang, “Research progress of cerebral vasospasm after craniocerebral injury,” Chinese Journal of Neurosurgery, vol. 36, no. 1, pp. 95–98, 2020. [Google Scholar]

32. Y. Liu, L. Ma, F. Huang, Z. Liu and Y. Zhou, “Progress in functional magnetic resonance imaging of emotion dysregulation due to traumatic brain injury,” Chinese Journal of Behavioral Medicine and Brain Science, vol. 29, no. 2, pp. 189–192, 2020. [Google Scholar]

33. Y. Ren, F. Zhu, K. S. Pradip, J. Wang, T. Wang et al., “Data query mechanism based on hash computing power of blockchain in internet of things,” IEEE Sensors Journal, vol. 20, no. 1, pp. C1, 2020. [Google Scholar]

34. L. Gong, B. Yang, T. Xue, J. Chen and W. Wang, “Secure rational numbers equivalence test based on threshold cryptosystem with rational numbers,” Information Sciences, vol. 466, no. 5, pp. 44–54, 2018. [Google Scholar]

35. J. Xu, Y. J. Zhang, K. Y. Fu and S. Peng, “SGX-based secure indexing system,” IEEE Access, vol. 19, no. 7, pp. 77923–77931, 2019. [Google Scholar]

36. X. Chen, J. Chen and Z. Sha, “Edge detection based on generative adversarial networks,” Journal of New Media, vol. 2, no. 2, pp. 61–77, 2020. [Google Scholar]

37. H. Wang, Z. Huang, Y. Qian, L. Yuan and A. Yuan, “Community residents' needs of general medical services and preferred healthcare institutions for treatment during the implementation of a three-tiered healthcare system,” Chinese General Practice, vol. 24, no. 7, pp. 805–811, 2021. [Google Scholar]

38. M. Ma, C. Wei and Z. Li, “Referral and government investment decision-making study based on hierarchical medical system,” Journal of Quantitative Economics, vol. 37, no. 4, pp. 130–140, 2020. [Google Scholar]

39. W. Chen, Z. Zhang and Z. Hua, “Analysis of two-tier public service systems under a government subsidy policy,” Computers & Industrial Engineering, vol. 90, no. 2, pp. 146–157, 2015. [Google Scholar]

40. Z. Hua, W. Chen and Z. Zhang, “Competition and coordination in two-tier public service systems under government fiscal policy,” Production and Operations Management, vol. 25, no. 8, pp. 1430–1448, 2016. [Google Scholar]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.