 Open Access
Open Access
ARTICLE
Wellbore Cleaning Degree and Hydraulic Extension in Shale Oil Horizontal Wells
1 College of Petroleum Engineering, China University of Petroleum (Beijing), Beijing, China
2 Daqing Oil Field Company Limited, Daqing, China
* Corresponding Author: Xin Ai. Email:
(This article belongs to the Special Issue: Fluid Flow and Materials Strength related to the Wellbore Safety)
Fluid Dynamics & Materials Processing 2024, 20(3), 661-670. https://doi.org/10.32604/fdmp.2023.026819
Received 27 September 2022; Accepted 25 May 2023; Issue published 12 January 2024
Abstract
The efficient development and exploitation of shale oil depends on long-distance horizontal wells. As the degree of cleaning of the wellbore plays a key role in these processes, in this study, this problem is investigated experimentally by focusing on the dimensionless cuttings bed height. A method is proposed to calculate the horizontal-well hydraulic extension taking into account the influence of the wellbore cleaning degree on the wellbore pressure distribution and assess the effect of a variety of factors such as the bottom hole pressure, the circulating pressure drop, the drilling pump performance and the formation properties. The analysis shows that the hydraulic extension of horizontal wells decreases with an increase in the cuttings bed height, and the higher the displacement of drilling fluid, the faster the hydraulic extension declines. The annular pressure drop of the horizontal section increases with the increase of the cuttings bed height, resulting in a higher bottom-hole pressure. Several arguments are provided to guide the safe drilling of shale oil horizontal wells and overcome the limits of current technological approaches.
Keywords
Nomenclature
| A | Annular overflow area, A = π(D2-d02)/4, m2 |
| A0 | Sectional area of nozzle exit, cm3 |
| d1 | Inner diameter of drill pipe, mm |
| a,b | Coefficient, dimensionless |
| n | Flow pattern index of drilling fluid, dimensionless |
| B | The flow coefficient of the nozzle, which is related to the resistance coefficient of the nozzle, and its value is always less than 1, dimensionless |
| Cec | Eccentricity coefficient of drill string, dimensionless |
| Ccb | Coefficient of cuttings bed, dimensionless |
| D | Wellbore hole diameter, m |
| do | Outside diameter of drill string, m |
| E | Eccentricity, dimensionless |
| e | Eccentric distance, the distance between drill string axis and wellbore axis, mm |
| f | Friction coefficient of drilling fluid, dimensionless |
| fcb | Friction coefficient between cuttings bed and drilling fluid, dimensionless |
| H | Dimensionless height of cuttings bed, dimensionless |
| hv | Height of the liquid column, m |
| k | Consistency index of drilling fluid, Pa∙sn |
| Lm | Hydraulic extension ability of the horizontal section, m |
| L1 | The length of horizontal section under the constraint of the formulas (1) and (2), m |
| L2 | The length of horizontal section under the constraint of the formula (3), m |
| Li | Length of the first horizontal well in i section, m |
| pf | Formation fracture pressure, MPa |
| pbh | Bottom hole pressure, MPa |
| pc | Formation collapse pressure, MPa |
| ph | Hydrostatic column pressure, MPa |
| Cb | Annular pressure loss factor, dimensionless |
| pp | Formation pore pressure, MPa |
| Q | The displacement of drilling fluid, m3/s |
| Qr | Rated displacement of pump, L/s |
| Pr | Rated power of pump, kw |
| | Pressure drop in the annulus of i section, MPa/m |
| Rec | Critical Reynolds number, dimensionless |
| Re | Reynolds number of drilling fluid, dimensionless |
| va | Annulus flow rate of drilling fluid, va = Q/A, m/s |
| ρf | Liquid density, g/cm3 |
| ρs | Cutting density, g/cm3 |
| ∆pg | Pressure loss of surface manifold, MPa |
| ∆pz | Bit pressure loss, MPa |
| ∆pa | Annular pressure loss, MPa |
| ∆pd | Internal pressure loss of drill string, MPa |
Shale oil is an important part of unconventional oil and gas resources, showing great resource potential in the region of Gulong in Daqing, Aoxian formation in Jiyang, Jimsar in Xinjiang and the region of Longdong in Changqing, as well as in Eagle Ford, USA. Due to the low permeability of shale oil reservoirs, long-distance horizontal wells have gradually become one of the key technologies for the efficient development of shale oil reservoirs [1,2], and the length of the horizontal section has become an important technical index [3,4]. However, the further extension of long-distance horizontal wells also faces severe challenges, which are highlighted in three aspects: open hole extension [5], mechanical extension [6] and hydraulic extension [4]. Open hole extension mainly engages in the study of the stability of wellbore holes [7]. The mechanical extension mainly engages in lowering the pipe string, which involves the transmission of weight on bit (WOB) and the buckling of the pipe string. At present, the research of the mechanical extension has been quite sufficient, which can be solved by measures such as drag reduction tools, low friction drilling fluid and floating casing running. Hydraulic extension mainly engages in the migration and return of cuttings in the wellbore, involving wellbore cleaning and wellbore pressure distribution. The cuttings bed of the horizontal section is formed by gravity [8], and the removal of the cuttings bed mainly relies on traditional measures such as reaming and large displacement circulation. Because of the limited effort of cuttings removal tools [9] and the hole cleaning degree is low, with the increase of the length for horizontal section, the cuttings accumulation problem becomes more and more serious [10–12], which will affect the circulating pressure consumption in the wellbore annulus, and then feed back to the bottom hole pressure distribution, resulting the increase of the equivalent circulating density of drilling fluid (ECD).
To solve the problem of hydraulic extension, the authors, Gulraiz et al. [13–15] have established a mathematical model about cutting migration and wellbore cleanliness. Li et al. [16] have established the drilling hydraulic extension model about the influence of formation fracture pressure. Liang et al. [17] have pointed out that the extension ability of horizontal wells is limited by the height of the cuttings bed. The authors, Wang et al. [18–20] have established a correction method of annular pressure loss considering the height of the cuttings bed and eccentricity of the drill string through experiments. The existing researches have not yet formed a calculation method of the hydraulic extension ability of shale oil horizontal wells that considers the cleanness of the wellbore. This paper analyzes the coupling relationship between cuttings bed and wellbore pressure distribution, establishes a calculation method of hydraulic extension ability, and gives the relationship between wellbore pressure distribution and hydraulic extension ability under different cuttings bed heights, which provides a theoretical basis for efficient drilling for the long-distance horizontal wells of shale oil.
2 Causation Analysis of Cuttings Bed
The deposition and bed formation of cuttings are related to the forces exerted on cuttings particles. The forces exerted on individual cutting particles mainly include the gravity, the buoyancy generated by drilling fluid, the lifting force generated by the rotation of drill string, the drag force and the driving force generated by the hydraulic pressure drop in drilling fluid. The inter-particle force is also generated when the particles contact each other, however, with the collision of particles, the momentum exchange occurs, so it could be neglected. Taking the shale reservoir of Daqing Gulong as an example, the relative density of shale cuttings is generally from 2.42 to 2.48 g/cm3. In order to avoid leakage and improve efficiency, the density of drilling fluid used is generally from 1.70 to 1.73 g/cm3. The buoyancy of drilling fluid alone is not enough to balance the gravity of cuttings in the horizontal section, and the cuttings tend to deposit to the bottom of the wellbore. When the lifting force, dragging force and thrust force are insufficient to push cuttings to saltate or roll along the lower section of the horizontal interval, the cuttings bed has formed. Generally, the ratio of cuttings bed height to wellbore diameter is used as a quantitative index to measure wellbore cleanliness, which is called dimensionless cuttings bed height. The monitoring of ECD, friction, torque, hook load, returned cuttings volume, and gas drilling, as well as the analysis of cuttings shape and cuttings particle size distribution can be used to evaluate the cleanness of the wellbore [17].
3 Establishment of Hydraulic Extension Ability Model for the Horizontal Well of Shale Oil Considering Wellbore Cleaning
3.1 Definition of the Hydraulic Extension Ability for Horizontal Well
In the process of horizontal well drilling, in order to avoid the danger of well out of control or wellbore instability, it is necessary to control the bottom hole pressure to be greater than the formation pore pressure and the formation collapse pressure. This relationship can be expressed by formula (1):
At the same time, it is necessary to control the bottom hole pressure not to exceed the formation fracture [21] pressure to avoid drilling fluid leakage. This relationship can be expressed by formula (2):
The circulation of drilling fluid is driven by the ground pump, so the total consumption for the circulating pressure of drilling fluid cannot be higher than the rated working pressure of the pump, which can be expressed by formula (3):
∆pL-total consumption for the circulating pressure, MPa; ppw-rated working pressure of the pump, MPa.
As both the bottom hole pressure and the total consumption for the circulating pressure of drilling fluid increases with the increase of the horizontal section, the maximum length of the horizontal section is limited, and the minimum value is taken as the hydraulic extension ability of the horizontal well, as shown in Fig. 1, which can be expressed by formula (4):
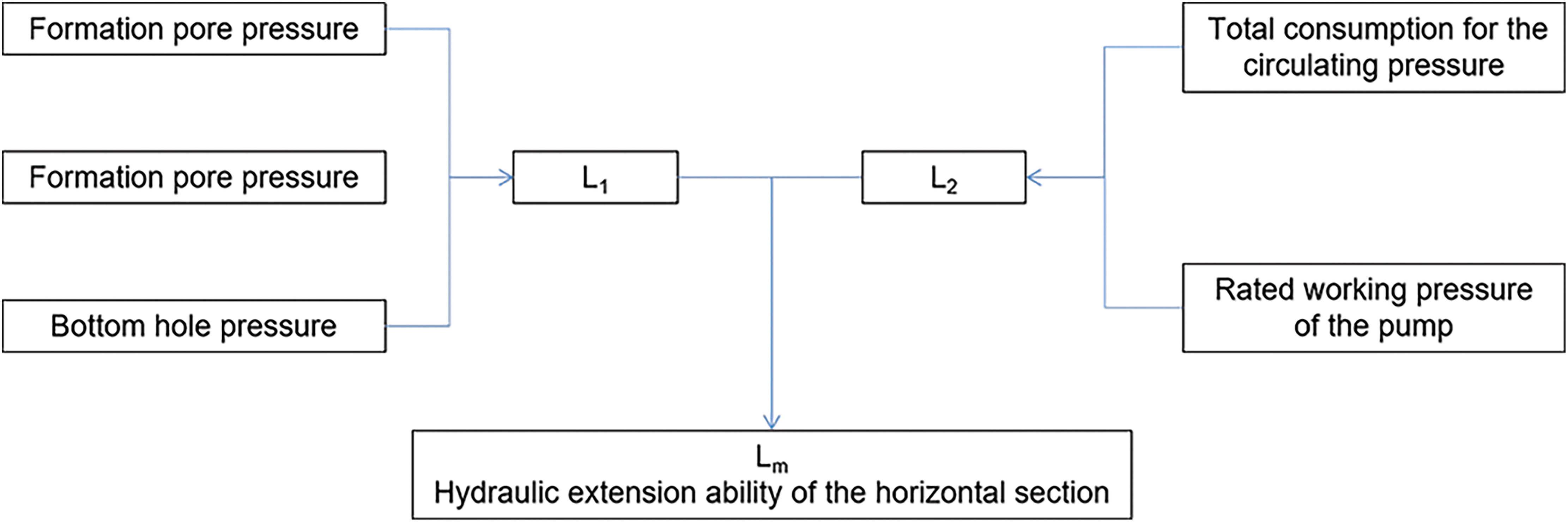
Figure 1: Sketch map
3.2 Relationship between the Length of Horizontal Section and the Distribution of Bottom Hole Pressure
Drilling fluid is injected into the bottom hole through the inner of the drill string by the bit port, and then returns to the surface through the annulus, so the bottom hole pressure is mainly composed of hydrostatic column pressure and annular circulation pressure considering the influence of cuttings, which can be expressed by formula (5):
The hydrostatic pressure can be calculated from the liquid density and the vertical height of the liquid column, as shown in formula (6):
The annular pressure drop of horizontal and vertical sections without considering the influence of the cuttings bed can be calculated by Funning-Darcy formula [18], as shown in formula (7):
The friction coefficient in formula (7) is related to the flow regime of drilling fluid, and the calculation is shown in formula (8):
The fluid regime of drilling fluid is judged as shown in formulas (9) and (10) [19]. If Re < Rec, it is judged as laminar flow and if Re > Rec, it is judged as turbulent flow:
3.3 Mechanism of the Influence from Wellbore Cleaning Degree on Hydraulic Extension Ability of Horizontal Wells
The wellbore cleaning degree mainly affects the hydraulic extension ability of horizontal wells by affecting the annular pressure loss. At the same time, the drill string in the horizontal section is frequently eccentric, which will also affect the loss of annular pressure. To sum up, the factor of the annular pressure loss in formula (5) can be calculated in two cases, that is, vertical section and horizontal section, which can be expressed by formula (11):
The eccentricity coefficient of the drill string can be obtained by the empirical formula [20], as shown in formula (12):
Eccentricity is related to the position of the drill string in the wellbore, as shown in formula (13):
The cutting bed height can be measured by cutting logging and calculation, like ECD monitoring of friction, torque and hook load, volume monitoring of returned rock cuttings. The coefficient of the cuttings bed [18] is mainly related to the dimensionless height of the cuttings bed, as shown in formula (14):
The friction coefficient between the cuttings bed and drilling fluid, as shown in formula (15):
3.4 Total Circulating Pressure Loss and Driving Pressure
In addition to the loss of annular circulating pressure, the total circulating pressure loss also includes the pressure loss generated in the surface manifold, drill bit and drill string. The calculation of pressure loss in drill string is similar to that in the annulus. The pressure loss of the surface manifold can be obtained by actual measurement. Therefore, the total circulating pressure loss is calculated as shown in formulas (16)–(19):
The drilling pump is the power source of drilling fluid circulation, and it has two working modes, which are rated pressure and rated power [4]. The actual working pressure is shown in formula (20):
Well-SYH2 is a horizontal shale oil well located in Northeast China, which the position of the deviation point is 2,155 m and the vertical depth is 2448.16 m. The formation pressure pp, fracture pressure pf and collapse pressure pc of shale reservoir are 34.79, 58.75 and 38.39 MPa, respectively. The Structure of the wellbore is shown in Fig. 2, and other calculation parameters are shown in Table 1.
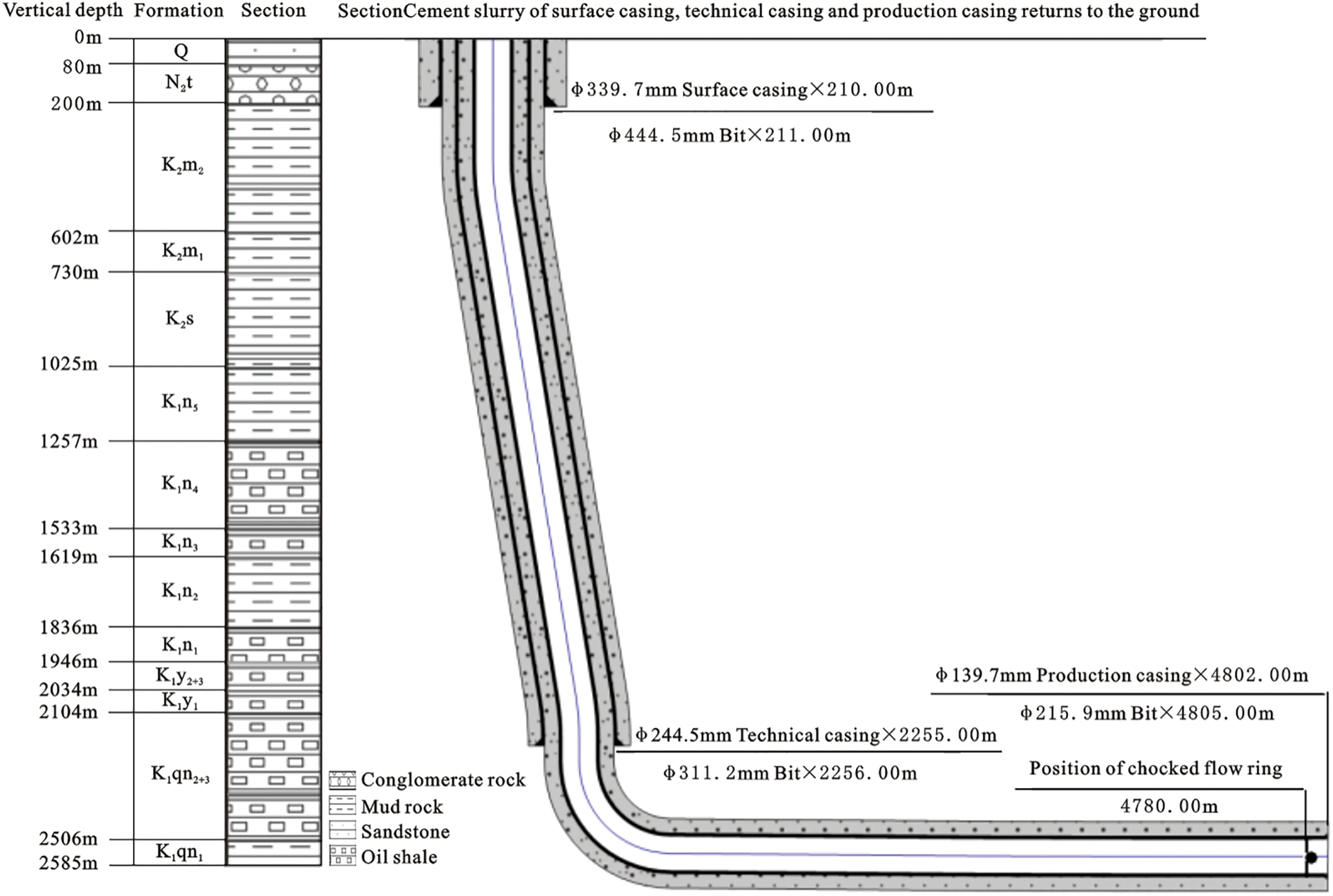
Figure 2: Structure of wellbore pipes
Fig. 3 shows the variation curve of hydraulic extension ability of horizontal section with the height of the dimensionless cuttings bed under different displacement conditions. On the whole, the hydraulic extension ability decreases with the increase of the height of the dimensionless cuttings bed and decreases with the increase of the displacement as well. When the dimensionless cuttings bed height increases from 0 to 50 and the drilling fluid displacement is 65 L/s, the length of hydraulic extension decreases from 542.40 to 400.95 m. When the displacement of drilling fluid is 30 L/s, the hydraulic extension length decreases from 10182 to 7770.68 m. Therefore, the combination of improving wellbore cleanliness and controlling the displacement of the drilling fluid can improve the hydraulic extension ability of shale oil horizontal wells. However, the reduction of the displacement will easily lead to debris deposition, so it is necessary to take other measures to clean up the debris bed. In this case, during the drilling process in the horizontal section, the displacement of drilling fluid reduces from 70 to 35 L/s, and the measures such as circulating well washing are taken to improve the cleanness of the wellbore, and the drilling of the horizontal section for 2000 m is successfully completed. According to the calculation, when the drilling fluid displacement is 50 L/s, the length of the hydraulic extension of this well is still over 2000 m. Considering that the large displacement drilling is beneficial to improve the wellbore cleaning degree and the penetration rate, the drilling fluid displacement of shale oil horizontal wells in this area is increased to 50 L/s, and the horizontal sections are drilled efficiently, which verifies the reliability of the model calculation.
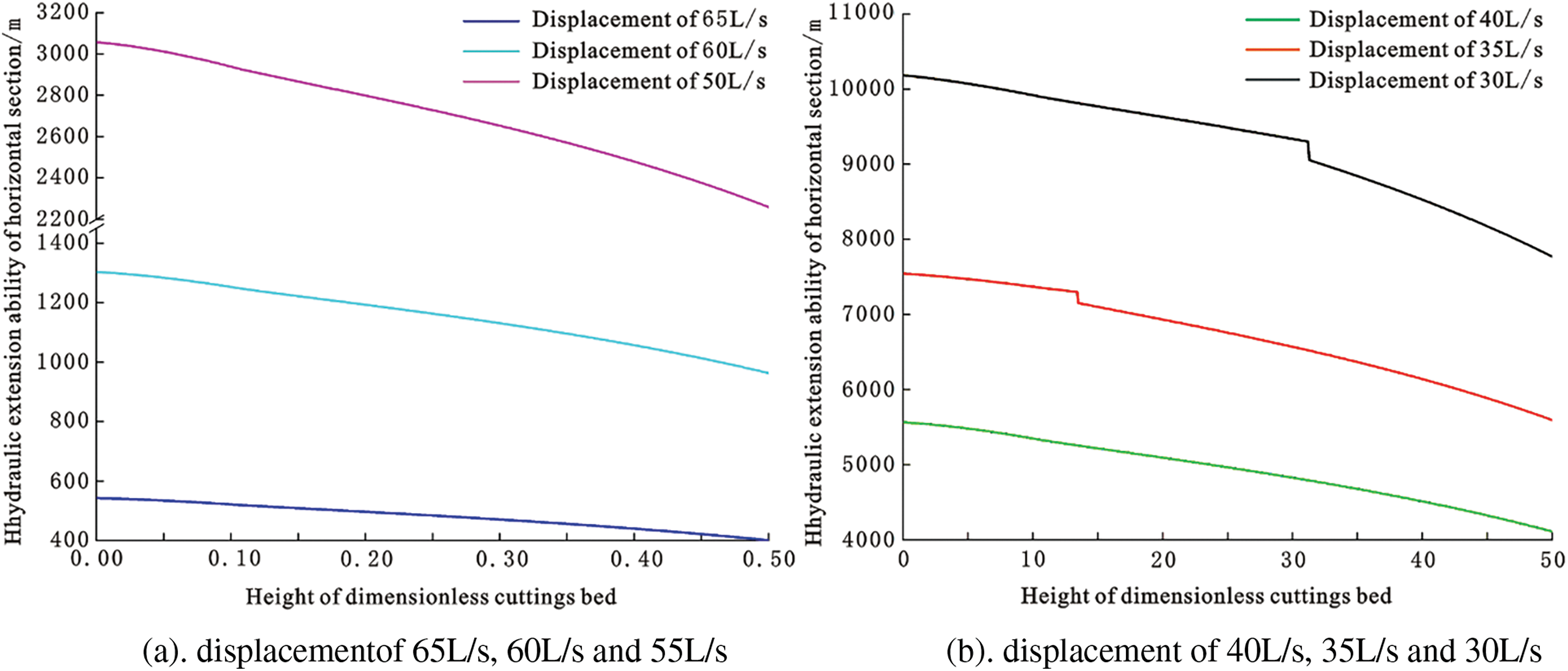
Figure 3: Changing rule of hydraulic extension ability of horizontal section as dimensionless cutting bed height
Fig. 4 shows the change in pressure distribution in the wellbore. According to the analysis, with the increase of the height of the dimensionless cuttings bed, the annular pressure loss in the horizontal section will increase, which will lead to an increase of the bottom hole pressure. In this process, the total circulating pressure loss remains unchanged, because the rated working pressure of the drilling pump limits the increase of the total circulating pressure loss. When the displacement is 30 L/s, the maximum of the total circulating pressure loss is the rated pump pressure, that is, 38.1 MPa. When the displacement is 65 L/s, the maximum of the total circulating pressure loss is 33.0 MPa, because the displacement exceeds the rated displacement of the drilling pump. Therefore, for shale oil horizontal wells, improving the performance parameters of drilling pumps can enhance the hydraulic extension ability of horizontal wells under the condition of high displacement.
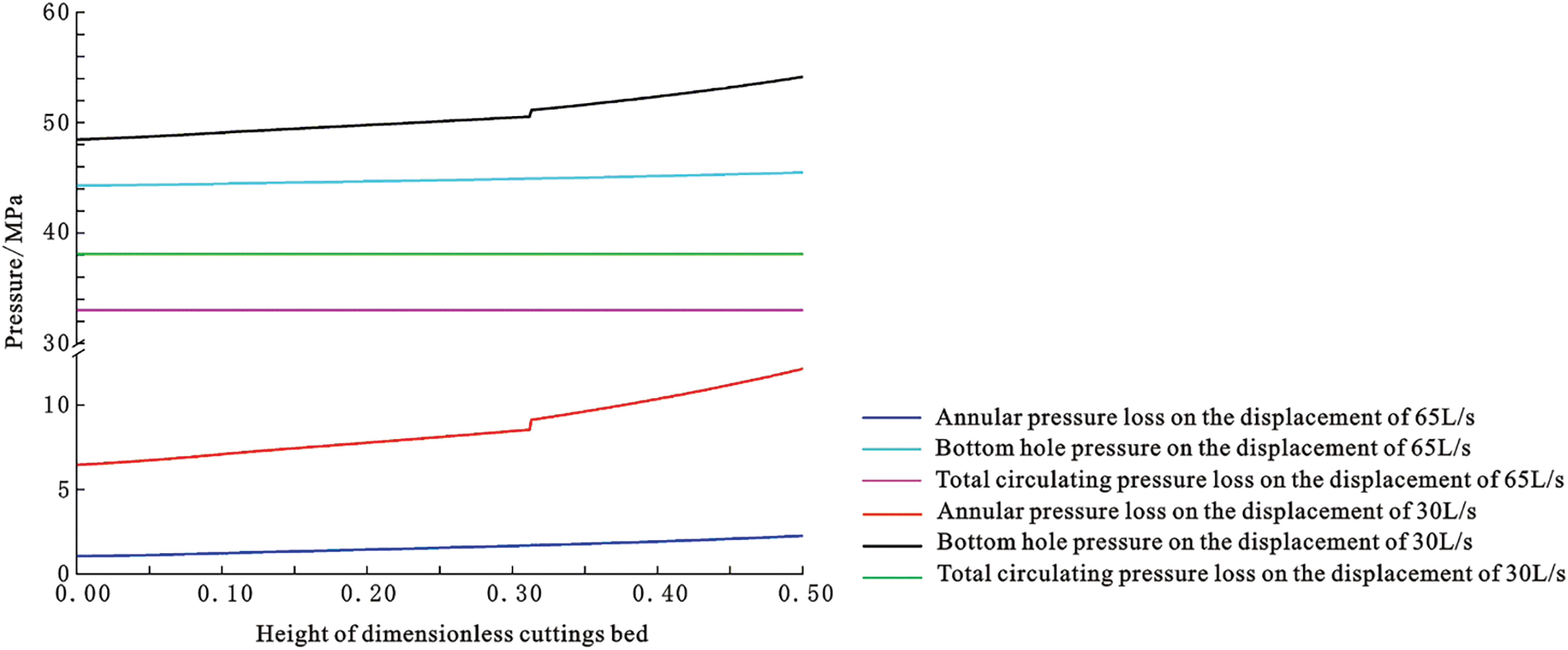
Figure 4: Changing rule of wellbore pressure parameters as dimensionless cutting bed height
(1) This paper provides a calculation method considering the cleanness of the wellbore hole and the ultimate length of the horizontal section under certain engineering conditions, The calculation method of horizontal well hydraulic extension ability constrained by formation character, bottom hole pressure, total circulating pressure loss and drilling pump performance is established, and the influence mechanism of wellbore cleaning on horizontal well hydraulic extension ability is revealed.
(2) With the increase of cuttings bed height, the hydraulic extension ability of shale oil horizontal wells decreases. The combination of improving wellbore cleaning degree and controlling displacement can improve the hydraulic extension ability of shale oil horizontal wells. It is necessary to take other measures to clean the cuttings bed. Considering that large displacement for the drilling is beneficial to improve the cleanness of the wellbore and the penetration rate, the displacement of drilling fluid should be increased as much as possible to meet the requirement of the length of the horizontal section.
(3) Annular pressure loss in the horizontal section increases with the height of the cuttings bed, which results in the increase of bottom hole pressure. For shale oil horizontal wells, improving the performance parameters of drilling pumps can enhance the hydraulic extension ability of horizontal wells under the condition of high displacement.
(4) The coupling of mechanical extension ability and hydraulic extension are not considered as the limitation of the model. In fact, the statistical results show that there is no direct relationship between the output of horizontal wells and the length of the horizontal section, which reflects the importance of geology, drilling rate and transformation efficiency of geology and engineering sweet heart. Therefore, the optimal length of the horizontal section should be determined comprehensively by considering equipment capacity, geological conditions and benefit factors.
Acknowledgement: This study thanks to the support of China University of Petroleum (Beijing).
Funding Statement: The project is supported by CNPC Key Core Technology Research Projects (2022ZG06) and project funded by China Postdoctoral Science Foundation (2021M693508). Basic research and strategic reserve technology research fund project of institutes directly under CNPC.
Author Contributions: Ai Xin: Formal analysis, Writing-original draft preparation, Writing-review & editing, Funding acquisition; Chen Mian: Methodology, Conceptualization, Project administration, Supervision.
Availability of Data and Materials: The original data and materials presented in the study are included in the article. Further inquiries can be directed to the corresponding authors.
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
References
1. Saputra, W., Kirati, W., Patzek, T. (2020). Physical scaling of oil production rates and ultimate recovery from all horizontal wells in the bakken shale. Energies, 13(8), 2052. [Google Scholar]
2. Medeiros, F., Kurtoglu, B., Ozkan, E., Kazemi, H. (2010). Analysis of production data from hydraulically fractured horizontal wells in shale reservoirs. SPE Reservoir Evaluation & Engineering, 13(3), 559–568. [Google Scholar]
3. Joshi, S. D. (1988). Augmentation of well productivity with slant and horizontal wells (includes associated papers 24547 and 25308). Journal of Petroleum Technology, 40(6), 729–739. [Google Scholar]
4. Lu, N., Zhang, B., Wang, T., Fu, Q. (2021). Modeling research on the extreme hydraulic extension length of horizontal well: Impact of formation properties, drilling bit and cutting parameters. Journal of Petroleum Exploration and Production, 11(3), 1211–1222. [Google Scholar]
5. Gao, D. (2018). Large-scale of the cluster horizontal wells project and high-efficiency development mode of shale gas in mountainous areas. Natural Gas Industry, 38(8), 1–7. [Google Scholar]
6. Huang, W., Gao, D., Liu, Y. (2018). Buckling analysis of tubular strings with connectors constrained in vertical and inclined wellbores. SPE Journal, 23(2), 301–327. [Google Scholar]
7. Allawi, R. H., Al-Jawad, M. S. (2021). Wellbore instability management using geomechanical modeling and wellbore stability analysis for Zubair shale formation in Southern Iraq. Journal of Petroleum Exploration and Production Technology, 11, 4047–4062. [Google Scholar]
8. Amanna, B., Movaghar, M. R. K. (2016). Cuttings transport behavior in directional drilling using computational fluid dynamics (CFD). Journal of Natural Gas Science and Engineering, 34, 670–679. [Google Scholar]
9. Duan, M., Miska, S., Yu, M., Takach, N., Ahmed, R. et al. (2009). Critical conditions for effective sand-sized solids transport in horizontal and high-angle wells. SPE Drilling & Completion, 24(2), 229–238. [Google Scholar]
10. Naganawa, S., Suzuki, M., Ikeda, K., Inada, N., Sato, R. (2021). Modeling cuttings lag distribution in directional drilling to evaluate depth resolution of mud logging. SPE Drilling & Completion, 36(1), 63–74. [Google Scholar]
11. Badrouchi, F., Rasouli, V., Badrouchi, N. (2022). Impact of hole cleaning and drilling performance on the equivalent circulating density. Journal of Petroleum Science and Engineering, 211, 110150. [Google Scholar]
12. Dokhani, V., Ma, Y., Yu, M. (2016). Determination of equivalent circulating density of drilling fluids in deepwater drilling. Journal of Natural Gas Science and Engineering, 34, 1096–1105. [Google Scholar]
13. Gulraiz, S., Gray, K. E. (2021). Modeling cuttings transport without drillpipe rotation while using the concepts of static and dynamic yield stresses. SPE Drilling & Completion, 36(3), 494–509. [Google Scholar]
14. Lin, P., Zheng, W., Wu, X. (2021). Influencing factors of the wellbore cleaning efficiency in extended reach wells based on seawater drilling fluid. Arabian Journal for Science and Engineering, 46, 6979–6988. [Google Scholar]
15. Meng, X., Wang, J. (2019). Production performance evaluation of multifractured horizontal wells in shale oil reservoirs: An analytical method. Journal of Energy Resources Technology, 141(10), 102907. [Google Scholar]
16. Li, X., Gao, D., Zhou, Y., Zhang, H. (2016). A model for extended-reach limit analysis in offshore horizontal drilling based on formation fracture pressure. Journal of Petroleum Science and Engineering, 146, 400–408. [Google Scholar]
17. Liang, H., Xu, S., Zhao, W. (2013). Monitoring methods of borehole cleanliness for underbalanced horizontal CBM gas wells. Natural Gas Industry, 33(10), 85–88. [Google Scholar]
18. Wang, H., Liu, X., Ding, G. (1996). Development of model for pressure drop in annular space of horizontal well. Journal of China University of Petroleum (Edition of Natural Science), 20(2), 30–35 (In Chinese). [Google Scholar]
19. Erge, O., Ozbayoglu, E. M., Miska, S. Z., Yu, M., Takach, N. et al. (2015). Laminar to turbulent transition of yield power law fluids in annuli. Journal of Petroleum Science and Engineering, 128, 128–139. [Google Scholar]
20. Kelessidis, V. C., Dalamarinis, P., Maglione, R. (2011). Experimental study and predictions of pressure losses of fluids modeled as Herschel-Bulkley in concentric and eccentric annuli in laminar, transitional and turbulent flows. Journal of Petroleum Science and Engineering, 77(3–4), 305–312. [Google Scholar]
21. Ponomareva, I. N., Galkin, V. I., Martyushev, D. A. (2021). Operational method for determining bottom hole pressure in mechanized oil producing wells, based on the application of multivariate regression analysis. Petroleum Research, 6(4), 351–360. [Google Scholar]
Cite This Article
 Copyright © 2024 The Author(s). Published by Tech Science Press.
Copyright © 2024 The Author(s). Published by Tech Science Press.This work is licensed under a Creative Commons Attribution 4.0 International License , which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.


 Submit a Paper
Submit a Paper Propose a Special lssue
Propose a Special lssue View Full Text
View Full Text Download PDF
Download PDF Downloads
Downloads
 Citation Tools
Citation Tools