 Open Access
Open Access
ARTICLE
Assessment of the Elastic-Wave Well Treatment in Oil-Bearing Clastic and Carbonate Reservoirs
1 Perm National Research Polytechnic University, Perm, 614990, Russian
2 Beijing University of Civil Engineering and Architecture, Beijing, 100049, China
3 Institute for Applied Mathematics FEB RAS, Vladivostok, 690041, Russian
* Corresponding Author: Vladimir Poplygin. Email:
(This article belongs to the Special Issue: Fluid Dynamics & Materials Processing in the Oil and Gas Industry)
Fluid Dynamics & Materials Processing 2023, 19(6), 1495-1505. https://doi.org/10.32604/fdmp.2023.022335
Received 05 March 2022; Accepted 20 July 2022; Issue published 30 January 2023
Abstract
A set of techniques for well treatment aimed to enhance oil recovery are considered in the present study. These are based on the application of elastic waves of various types (dilation-wave, vibro-wave, or other acoustically induced effects). In such a context, a new technique is proposed to predict the effectiveness of the elastic-wave well treatment using the rank distribution according to Zipf’s law. It is revealed that, when the results of elastic wave well treatments are analyzed, groups of wells exploiting various geological deposits can differ in terms of their slope coefficients and free members. As the slope coefficient increases, the average increase in the well oil production rate (after the well treatment) becomes larger. An equation is obtained accordingly for estimating the slope coefficient in the Zipf’s equation from the frequency of the elastic wave. The obtained results demonstrate the applicability of the Zipf’s law in the analysis of the technological efficiency of elastic-wave well treatment methods.Keywords
Nomenclature
| ω | number of occurrences of the considered characteristics |
| R | rank of the considered characteristics |
| | constant parameter |
| QDWA i | increase in the oil flow rate of the ith well under dilation-wave action |
| QDWA av. | average increase in the oil flow rate of all wells under dilation-wave action |
| QAI i | increase in the oil flow rate of the ith well under acoustic impact |
| QAI av. | average increase in the oil flow rate of all wells under acoustic impact |
| QVWA i | increase in the oil flow rate of the ith well under vibration wave action |
| QVWA av. | average increase in the oil production rate of all wells under vibration wave action |
| DWA | dilation-wave action |
| VWA | vibration wave action |
| AI | acoustic impact |
| DWAcarb | dilation-wave action in carbonate reservoirs |
| DWAclast | dilation-wave action in sandstone reservoirs |
| DWAcarb.1 | dilation-wave action in the carbonates of tectonic structure 1 |
| DWAclast.1 | dilation-wave action in the sandstones of tectonic structure 1 |
| AIBb | acoustic impact in sandstones |
| AIMl.1 | acoustic impact in the sandstone reservoirs in field 1 |
| AIT1.2 | acoustic impact in the sandstone reservoirs in field 2 |
| AIMl.3 | acoustic impact in the sandstone reservoirs in field 3 |
| VWABsh.1 | vibration wave action in field 1 |
| VWABsh.2 | vibration wave action in field 2 |
| VWABsh.3 | vibration wave action in field 3 |
The proportion of oil extracted from oil fields during flooding remains at around 35%–40%, while the rest remains in the rocks. To enhance oil recovery, various techniques have been developed and used, including injection of heat or polymers, acid treatments and hydraulic fracturing, and elastic wave treatments. However, there is no universal method of enhanced oil recovery that can be implemented in any reservoir. One of the common methods used to enhance oil recovery is the application of elastic wave action in reservoirs to increase permeability, reduce the interfacial tension between the oil and water, and reduce the capillary pressure in the pores. For example, natural seismic activity was found to significantly increase production in a field [1]. Therefore, studying the influence of elastic waves on the multiphase movement of fluid in a porous saturated material is an important topic in the field of oil production. Beresnev et al. [2] noted that well productivity increases after the application of elastic wave action. In addition, the increase in production under the action of vibration can reach 65% [3]. It has been reported that the penetration depth of some waves can reach a depth of 200 km in an oil reservoir [4]. The increase in oil production is because the vibrations increase the permeability of the rocks to formation fluids. In addition, the elastic waves intensify the movement of the fluid itself under a given permeability [5]. Beresnev et al. [6] and Beresnev et al. [7] have created models to simulate a porous medium and have found that vibrations have a positive effect on the fluid flow process when overcoming constrictions, and a lower vibration frequency is more effective than a high frequency. The experiments on elastic wave action performed by Ariadji [8] on sandstone revealed that the porosity increased by 53.9% and the permeability increased by 54.1% compared to the initial values. This technique has been reported to be less efficient in carbonate rocks than in sandstone. An optimal frequency of 10 Hz has been proposed to increase the permeability. Louhenapessy et al. [9] reported that compared to longitudinal waves, circular waves increase the oil recovery by 3% more and reduce the residual oil saturation of oil rocks. The optimal frequency for circular waves is 20 Hz, and that for longitudinal waves is 35 Hz. The influence of the elastic wave improves the porosity and permeability by up to 34%. For rock samples from the Perm region, researchers [10] have determined that the dynamic elasticity modulus of carbonate reservoir rocks subjected to wave action increased logarithmically as the frequency of the dynamic load increased from 0 to 10 Hz; while for an increase in frequency from 10 to 60 Hz, the increase in the elasticity modulus followed a power law for each load amplitude. An important conclusion was drawn: at high strain rates, the rock becomes more rigid compared to when the rock is subjected to dynamic loading at a lower strain rate. Several studies have shown that high-frequency ultrasonic treatment is also quite effective. While modeling the effects of elastic waves with a frequency of 40 kHz on cylindrical samples of oil-saturated sandstone, an increase in oil recovery was observed [11]. Hamidi et al. [12] reported that under ultrasonic action, the oil viscosity decreased. Alhomadhi et al. [13] reported the results of a wave with a frequency of 50 kHz and noted that the application of wave action to a high-permeability core resulted in a higher oil recovery than application to a low-permeability core. However, there was a general increase in the oil recovery from both cores, and as the treatment time increased, the effect increased. Recently, Agi et al. [14] applied a combination of ultrasonic treatment with a frequency of 40 kHz and the injection of surfactants, which increased the oil recovery by 12%. Some technologies can increase the flow rate of oil wells by 91% and reduce the water cut by 20% [15]. It should be noted that the major portion of such research was conducted using small-scale models of oil reservoirs. It is well known that elastic wave treatments are followed by wave attenuation. Ma et al. [16] found that for siltstones, the attenuation intensity increases as the permeability and clay content increase; while for dolomites, the attenuation increases as the permeability, porosity, and water saturation increase. Wave attenuation is the strongest in sandstone at high water saturation.
Riabokon [17] presented field data for wells treated using oscillators and various operating principles. Because it has been reported to have the highest intensity observed effects, acoustic well treatment in the ultrasonic range has become the most widely used. An ultrasonic downhole oscillator operating at frequencies of 20–27 kHz and the mechanism of its effect on the fluid flow in the near-wellbore zone were investigated by Mullakaev et al. [18]. To achieve the maximum effect of the vibration treatment in the near-wellbore zone, Pérez-Arancibia et al. [19] proposed a mathematical model that allows the user to select the optimal values of the oscillation of the vibration unit in a perforated well. Another mathematical model for evaluating the fluid flow in the field of elastic oscillation in a perforated well was developed by Prachkin et al. [20]. In a recent field application review, Meribout [21] suggested that since the application of elastic oscillations is one of the most promising enhanced oil recovery (EOR) methods, combining a vibration treatment with the injection of gas, liquid, and other chemicals into the near-wellbore zone should produce excellent results. According to previous studies [22,23], elastic oscillations have the following effects on rocks: capillary effects, poroelastic motion, thermal effects, increased degassing, and changes in the oil viscosity, mobility ratio, oil displacement by water, and rock permeability. According to the review conducted by Avvaru et al. [24], the effect of cavitation is one of the most important effects influencing the stimulation of the flow of oil into the well. Exposure to elastic waves leads to destruction of paraffin compounds and a decrease in the oil viscosity. Mullakaev et al. [25] designed an experimental system that simulates a borehole on the actual scale. They concluded that the increase in the oil flow rate was caused by the reduction in the effective viscosity. Regarding acoustic cleaning, Wong et al. [26] built an experimental setup containing a linear cell. They conducted tests at a frequency of 20 kHz on Berea sandstone samples (diameter of 7.5 cm and length of 20 cm) containing a mud filter cake. They regained 100% of the permeability lost due to formation damage.
The results of previous studies do not provide an unambiguous conclusion regarding the effectiveness of the exposure of reservoir rocks to high and low frequencies. Therefore, prior to application in an oil field, it is worth conducting a series of experiments on saturated rocks to select the optimal conditions for elastic wave treatments.
To assess the effectiveness of the wave action techniques, the laws of rank distribution were used. The applicability of the rank distribution method for describing and understanding processes based on a set of interconnected heterogeneous phenomena and their quantitative and qualitative characteristics has been studied [27]. The rank distributions include the rank (number) of the object R and the frequency
The application of the laws of rank distribution was applied to wells in a specific area where elastic wave stimulation techniques were actively being used to increase well flow rates. The elastic wave well treatment was carried out using various techniques and high and low frequencies. This paper presents the results of the application of these techniques and evaluates their effectiveness based on the rank distribution.
2 Elastic Wave Well Treatment Techniques
Three types of elastic wave techniques were carried out for well treatment in the area of interest. The dilation-wave action (DWA) technique consists of creating a zone of expansion (decompaction) in the rocks around the well within the perforated interval due to the weight of the tubing string. When the pumping pipes rest on the rocks through a special liner, low-frequency elastic vibrations are excited in the formation rocks. These vibrations are created in the liner and through it into the rocks by a column of pumped liquid during the operation of the sucker rod pump. The DWA is characterized by frequencies of 1–10 Hz. The vibration wave action (VWA) is carried out by placing a hydrodynamic transmitter (pressure wave generator) at the bottom of the well, which excites pressure fluctuations in the flow of the agent injected into the formation. The average vibration frequency is 66 Hz. The VWA + Acid consists of vibration wave exposure via oil-acid compositions. The acoustic impact (AI) is the long-term impact of the acoustic waves on the bottomhole zone of the well. The wave generating device is operated within the well. The average exposure frequency is 25 kHz. The results of the technologies applied are presented in Table 1.
The high-frequency impact of the AI technique resulted in an additional oil production of 877 tons. The results in Table 1 show that as the average impact frequency increased, the incremental oil production decreased. This result might be because under low-frequency exposure, the amplitude was several megapascals, while it was only 0.3 MPa under high-frequency exposure.
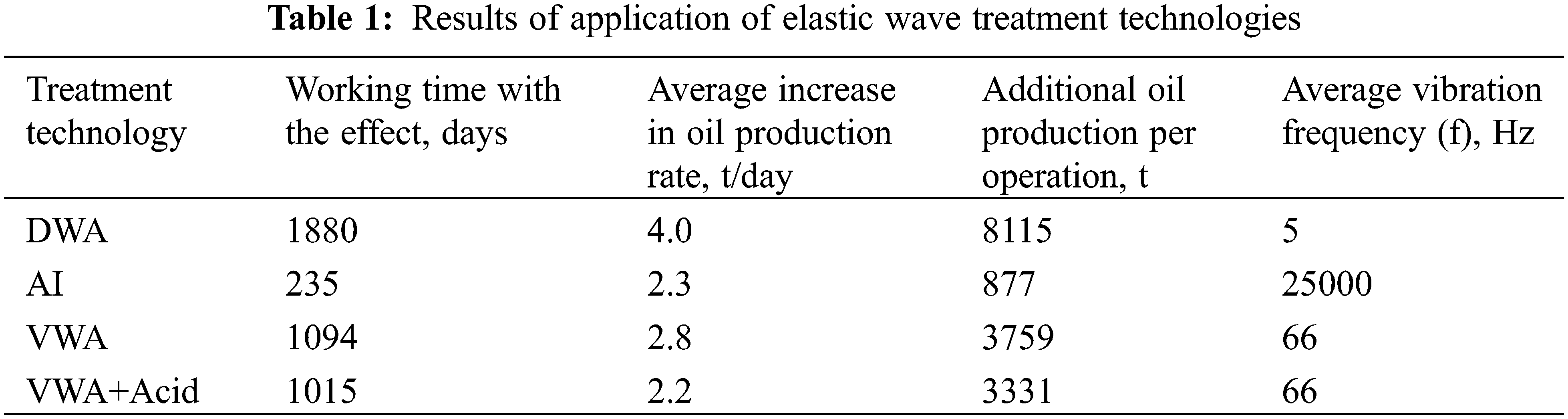
The DWA technique was applied to 13 wells. Six of the wells produced oil from carbonate reservoirs (Fig. 1a) and seven of the wells were in clastic (sandstone) reservoirs (Fig. 1b). In the wells producing oil from carbonate reservoirs, the additional oil production amounted to 5981 tons, and the duration of the additional effect was 1133 days. In the wells producing oil from clastic reservoirs, the additional oil production amounted to 2175 tons, and the duration of the additional effect was 716 days. The results of the application of the DWA technique indicate that the technique is more efficient when applied to carbonate rocks. The higher efficiency for carbonate rocks is associated with the presence of a system of pores and fractures. The fluid flow from the reservoir to the well occurs along the fractures, and the bulk of the oil is contained in the pore blocks. Under wave action, an additional pressure drop occurs between the blocks and fractures, which contributes to the flow of oil from the blocks into the fractures and then into the well.
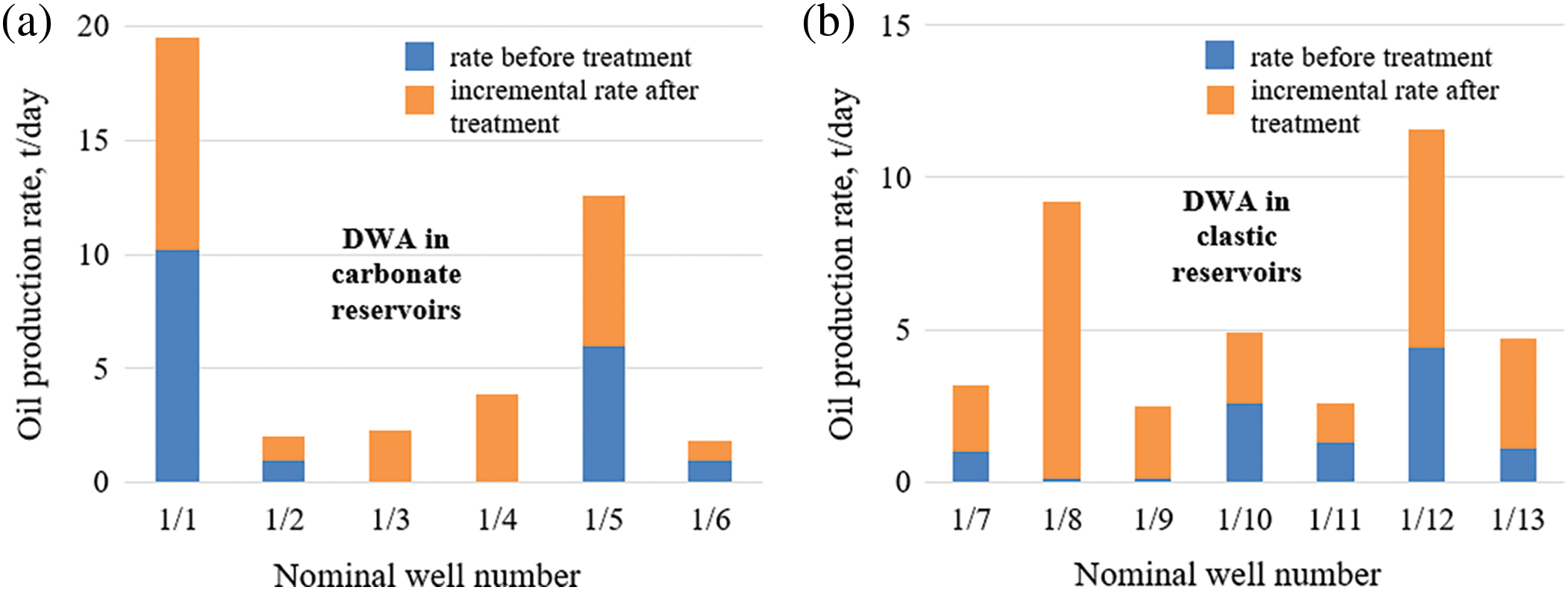
Figure 1: Changes in oil rates of production wells after well treatments with DWA technology: (a) in carbonate reservoirs; (b) in clastic reservoirs
To assess the technological efficiency of the elastic wave action in the wells using the DWA and in accordance with the idea of well rank analysis, the results were arranged in ascending order of the absolute value of
We prepared the rank distribution of the elastic wave treatments and obtained the regions with different slopes (Fig. 2). The slope of the dependence of the natural logarithm of the rank on the logarithm relative to the additional oil production for the carbonate reservoirs (DWAcarb) had a larger slope. The wells exploiting clastic reservoirs were divided into two sets (DWAclast and DWAclast.1). These sets had similar slopes, but their free terms differed. The wells belonging to these two sets exploited deposits confined in different tectonic structures. Accordingly, the geological confinement is an important criterion for the effectiveness of the DWA method.
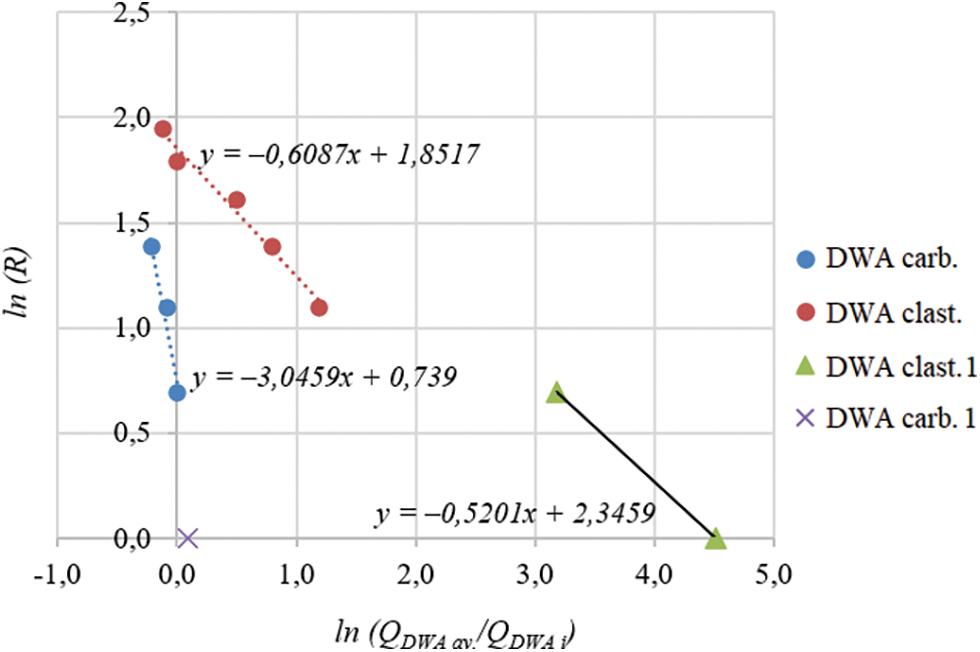
Figure 2: Changes in the logarithm of the rank from the logarithm of the relative average oil production rate for clastic and carbonate reservoirs after DWA treatment
The AI technique was applied to 25 wells in clastic reservoirs (Fig. 3). The additional oil production amounted to 740 tons, and the duration of the additional effect was 213 days. The high efficiency for the clastic rocks is associated with the presence of deposits in the pore flow channels, which under high-frequency exposure become suspended, and the flow area increases. The areas with different angular coefficients can be distinguished using Eq. (1) for the clastic reservoirs (Fig. 4). For DWA technique, the areas correspond to different geological structures (oil reservoirs).
Figure 3: Changes in oil rates of production wells after well treatments with AI technology in clastic reservoirs
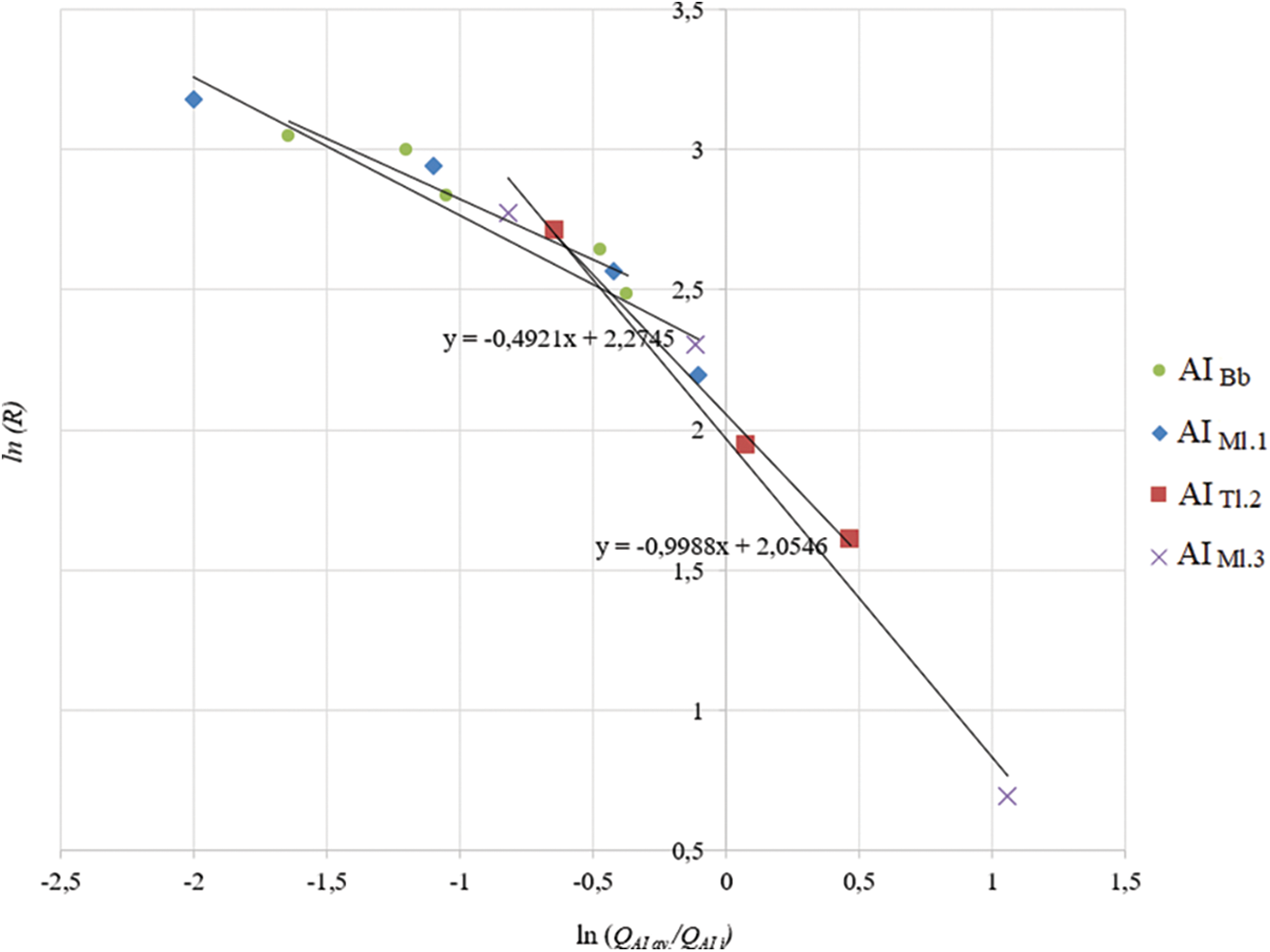
Figure 4: Change in the logarithm of the rank from the logarithm of the relative average oil production rate for clastic reservoirs after AI treatment
2.3 VWA and VWA + Acid Techniques
The VWA and VWA + acid techniques were applied to 65 wells. Forty-six of the wells were in carbonate reservoirs (Fig. 5a), and 19 of the wells were in clastic sandstone reservoirs (Fig. 5b). The additional oil production in the wells producing oil from carbonate reservoirs amounted to 3646 tons, and the duration of the additional effect was 2097 days. The additional oil production in the wells producing oil from clastic formations amounted to 917 tons, and the duration of the additional effect was 302 days. The results of the application of VWA and VWA + acid techniques indicate that they both have a greater efficiency when applied to carbonate rocks. The values of
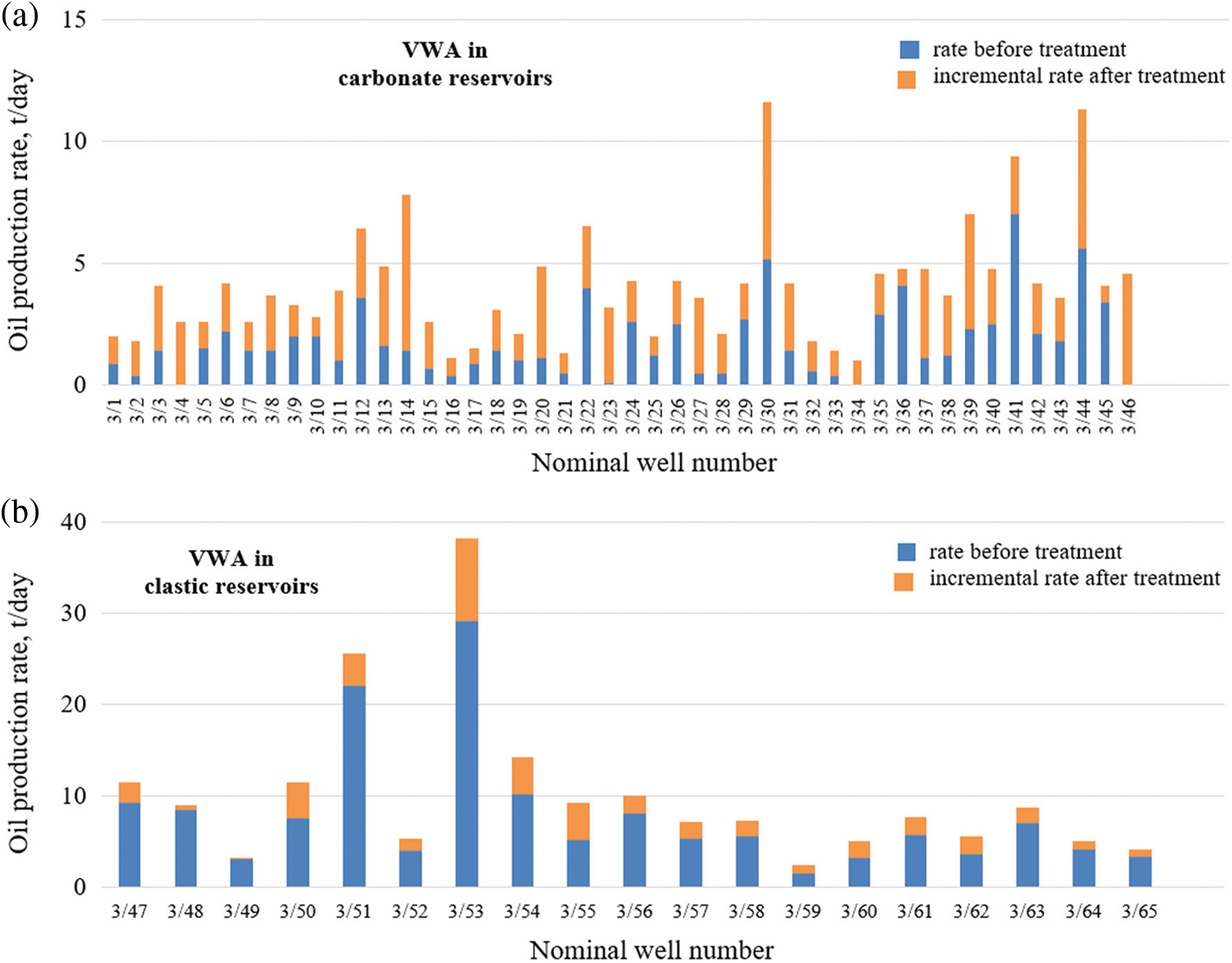
Figure 5: Changes in oil rates of production wells after well treatments with VWA and VWA+acid technology: (a) in carbonate reservoirs; (b) in clastic reservoirs
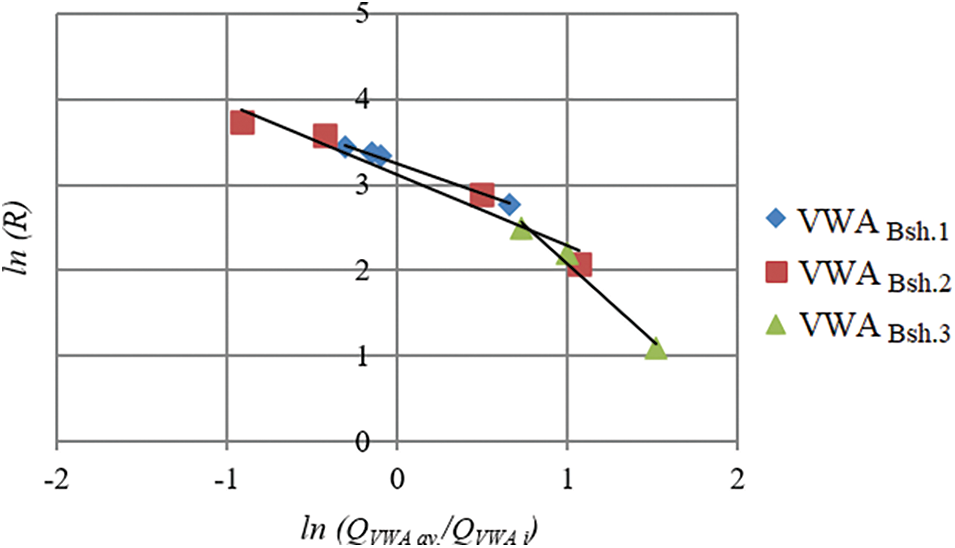
Figure 6: Logarithm rank dependence on the relative average oil rate for carbonate reservoirs after VWA treatment
The average slopes in Eq. (1) for the DWA treatment are the largest compared to the AI and VVA techniques. The amplitudes and frequencies of the pressure changes of the techniques differ. The change in the average slope in Eq. (1) for the average frequency of the elastic wave action was studied. For the considered frequency interval, an equation for the preliminary slope after wave action was developed:
The changes in the duration of the effect of the technique and the relative increase in the well production rate have similar characteristics. As the logarithm of the impact frequency increases, the duration of the effect of the technique decreases, and the relative increase in the well production also decreases. Based on the constant parameter
The results obtained from the analysis of the field data are comparable to the results of laboratory studies [9]. That is, a 60.54% increase in oil production was obtained when using intermittent longitudinal waves, an increase of 63.53% was obtained when using constant longitudinal waves, and an increase of 64.76% was obtained when using circular waves.
When studying the plane-parallel liquid flow through a porous medium under conditions of wave processing, Marfin et al. [29] demonstrated that in the fields of the elastic waves, the ratio of the permeability of a porous medium to the viscosity of a liquid increases by 300% or more. Since well productivity is directly proportional to the increase in the well flow rate, the average change in the flow rate will also be 300%.
Tests of the impact of high-frequency acoustic waves in field applications have been reported to have an efficiency of approximately 179% in terms of the well productivity [30], which is consistent with the results of this study.
The effect of elastic wave action decreases with increasing distance from the emitter. Therefore, the effect of the application of an elastic wave treatment to a saturated porous medium near the well using an emitter located inside the well will be more effective at a high frequency. When moving deeper into the oil reservoir, the efficiency of the elastic wave treatment will decrease with increasing frequency. According to Hamida et al. [31], the penetration depth of an elastic wave with a frequency of 20 kHz does not exceed 10 cm.
These conclusions are consistent with the analysis of field data after elastic wave treatment. Wave stimulation using the DWA technique was performed on well 433, with a frequency of up to 5 Hz and an amplitude of approximately 3 MPa (Fig. 7). The average distance between wells in this area was 500 m, and the permeability of the plate was 494 mD. During the observation period, the flow rate of well 433 increased by 133%, and it did not tend to decrease. In the first 2 months after the start of the elastic wave treatment in wells 431, 107, and 100, an increase in the fluid flow rate of up to 130% was observed. The change in the fluid flow rates of the wells at a distance of 500 m indicates deep penetration of the low-frequency waves into the reservoir.

Figure 7: Location of wells in an oil field
When analyzing the results of the impact of high-frequency acoustic waves, no changes in the production rates of the surrounding wells were observed.
Studying the mechanism of elastic wave well treatments and its influence on oil production is of great practical importance in the oil industry. In this study, the results of elastic wave well treatments in a specific area were analyzed. The DWA, AI, and VWA techniques were applied, and the results were assessed. The DWA technique is more efficient in carbonate formations. In addition, during the application of the DWA technique, a pressure amplitude of several megapascals occurs, which allows the wave to penetrate deep into the reservoir and to affect the surrounding wells. The AI technique is most effective in clastic rocks (sandstones). The penetration depth of the wave under high-frequency treatment is small, and the properties only change near the wellbore. The VWA and VWA + acid techniques are most efficient in carbonate rocks. The change in the relative average oil production according to Zipf’s law was studied. The wells were clustered according to the oil reservoirs they encountered. The well groups were characterized by a linear change in the logarithms of R and
Funding Statement: The reported study was supported by the Government of Perm Krai, Research Project No. C-26/628 dated 05/04/2021.
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
References
1. Mirzaei-Paiaman, A., Nourani, M. (2012). Positive effect of earthquake waves on well productivity: A case study: Iranian carbonate gas condensate reservoir. Scientia Iranica, 19(6), 1601–1607. DOI 10.1016/j.scient.2012.05.009. [Google Scholar] [CrossRef]
2. Beresnev, I. A., Johnson, P. A. (1994). Elastic-wave stimulation of oil production: A review of methods and results. Geophysics, 59(6), 1000–1017. DOI 10.1190/1.1443645. [Google Scholar] [CrossRef]
3. Dai, L., Zhang, Y. (2019). Effects of low frequency external excitation on oil slug mobilization and flow in a water saturated capillary model. Petroleum, 5(4), 375–381. DOI 10.1016/j.petlm.2019.03.001. [Google Scholar] [CrossRef]
4. Nikolaevskiy, V. N., Lopukhov, G. P., Liao, Y., Economides, M. J. (1996). Residual oil reservoir recovery with seismic vibrations. SPE Production and Facilities, 11(2), 89–94. DOI 10.2118/29155-PA. [Google Scholar] [CrossRef]
5. Graham, D. R., Higdon, J. J. L. (2000). Oscillatory flow of droplets in capillary tubes. Part 2. Constricted tubes. Journal of Fluid Mechanics, 425, 55–77. DOI 10.1017/S0022112000002032. [Google Scholar] [CrossRef]
6. Beresnev, I. A., Deng, W. (2010). Viscosity effects in vibratory mobilization of residual oil. Geophysics, 75(4), 79–85. DOI 10.1190/1.3429999. [Google Scholar] [CrossRef]
7. Beresnev, I. A., Gaul, W., Vigil, R. D. (2011). Direct pore-level observation of permeability increase in two-phase flow by shaking. Geophysical Research Letters, 38(20), L20302. DOI 10.1029/2011GL048840. [Google Scholar] [CrossRef]
8. Ariadji, T. (2005). Effect of vibration on rock and fluid properties: On seeking the vibroseismic technology mechanisms. SPE Asia Pacific Oil and Gas Conference and Exhibition, pp. 161–168. Jakarta, Indonesia. DOI 10.2118/93112-MS. [Google Scholar] [CrossRef]
9. Louhenapessy, S. C., Ariadji, T. (2020). The effect of type waves on vibroseismic implementation of changes properties of rock, oil viscosity, oil compound composition and enhanced oil recovery. Petroleum Research, 5(4), 304–314. DOI 10.1016/j.ptlrs.2020.05.001. [Google Scholar] [CrossRef]
10. Riabokon, E., Turbakov, M., Popov, N., Kozhevnikov, E., Poplygin, V. et al. (2021). Study of the influence of nonlinear dynamic loads on elastic modulus of carbonate reservoir rocks. Energies, 14, 8559. DOI 10.3390/en14248559. [Google Scholar] [CrossRef]
11. Hamida, T., Babadagli, T. (2005). Effect of ultrasonic waves on the capillary-imbibition recovery of oil. SPE Asia Pacific Oil and Gas Conference and Exhibition, pp. 37–48. Jakarta, Indonesia. DOI 10.2118/92124-MS. [Google Scholar] [CrossRef]
12. Hamidi, H., Mohammadian, E., Rafati, R., Azdarpour, A. (2013). A role of ultrasonic waves on oil viscosity changes in porous media. CEAT 2013–2013. IEEE Conference on Clean Energy and Technology, pp. 1–6. Langkawi, Malaysia. DOI 10.1109/CEAT.2013.6775589. [Google Scholar] [CrossRef]
13. Alhomadhi, E., Amro, M., Almobarky, М (2014). Experimental application of ultrasound waves to improved oil recovery during waterflooding. Journal of King Saud University-Engineering Sciences, 26(1), 103–110. DOI 10.1016/j.jksues.2013.04.002. [Google Scholar] [CrossRef]
14. Agi, A., Junin, R., Shirazi, R., Afeez, G., Yekeen, N. (2019). Comparative study of ultrasound assisted water and surfactant flooding. Journal of King Saud University-Engineering Sciences, 31, 296–303. DOI 10.1016/j.jksues.2018.01.002. [Google Scholar] [CrossRef]
15. Abramov, V. O., Abramova, A. V., Bayazitov, V. M., Marnosov, A. V., Kuleshov, S. P. et al. (2016). Selective ultrasonic treatment of perforation zones in horizontal oil wells for water cut reduction. Applied Acoustics, 103(B), 214–220. DOI 10.1016/j.apacoust.2015.06.017. [Google Scholar] [CrossRef]
16. Ma, R., Ba, J., Lebedev, M., Gurevich, B., Sun, Y. (2021). Effect of pore fluid on ultrasonic S-wave attenuation in partially saturated tight rocks. International Journal of Rock Mechanics and Mining Sciences, 147, 104910. DOI 10.1016/j.ijrmms.2021.104910. [Google Scholar] [CrossRef]
17. Riabokon, E. P. (2020). Methodology for forecasting the oil rate change while elastic wave propagation in the near-wellbore zone of clastic reservoirs. Neftyanoe Khozyaystvo-Oil Industry, 6, 76–79. DOI 10.24887/0028-2448-2020-6-76-79. [Google Scholar] [CrossRef]
18. Mullakaev, M. S., Abramov, V. O., Pechkov, A. A. (2009). Ultrasonic unit for restoring oil wells. Chemical and Petroleum Engineering, 45, 133–137. DOI 10.1007/s10556-009-9160-9. [Google Scholar] [CrossRef]
19. Pérez-Arancibia, C., Godoy, E., Durán, M. (2018). Modeling and simulation of an acoustic well stimulation method. Wave Motion, 77, 214–228. DOI 10.1016/j.wavemoti.2017.12.005. [Google Scholar] [CrossRef]
20. Prachkin, V. G., Mullakaev, M. S., Asylbaev, D. F. (2014). Improving the productivity of wells by means of acoustic impact on high-viscosity oil in the channels of the face zone of a well. Chemical and Petroleum Engineering, 50(9–10), 571–578. DOI 10.1007/s10556-014-9943-5. [Google Scholar] [CrossRef]
21. Meribout, M. (2018). On using ultrasonic-assisted enhanced oil recovery (EORRecent practical achievements and future prospects. IEEE Access, 6, 51110–51118. DOI 10.1109/ACCESS.2018.2859774. [Google Scholar] [CrossRef]
22. Cidoncha, J. G. (2007). Application of acoustic waves for reservoir stimulation. Society of Petroleum Engineers-2nd International Oil Conference and Exhibition in Mexico, pp. 258–264. Veracruz, Mexico. DOI 10.2118/108643-MS. [Google Scholar] [CrossRef]
23. Riabokon, E. P. (2020). Laboratory study on the effect of elastic wave treatment on geomechanical and capillary properties of clastic reservoirs. Neftyanoe Khozyaystvo-Oil Industry, 4, 54–57. DOI 10.24887/0028-2448-2020-4-54-57. [Google Scholar] [CrossRef]
24. Avvaru, B., Venkateswaran, N., Uppara, P., Iyengar, S. B., Katti, S. S. (2018). Current knowledge and potential applications of cavitation technologies for the petroleum industry. Ultrasonics Sonochemistry, 42, 493–507. DOI 10.1016/j.ultsonch.2017.12.010. [Google Scholar] [CrossRef]
25. Mullakaev, M. S., Abramov, O. V., Abramov, V. O., Gradov, O. M., Pechkov, A. A. (2009). An ultrasonic technology for productivity restoration in low-flow boreholes. Chemical and Petroleum Engineering, 45(3–4), 203–210. DOI 10.1007/s10556-009-9171-6. [Google Scholar] [CrossRef]
26. Wong, S. W., Van Der Bas, F., Groenenboom, J., Zuiderwijk, P. (2003). Near wellbore stimulation by acoustic waves. SPE European Formation Damage Conference, The Hague, Netherlands. DOI 10.2118/82198-MS. [Google Scholar] [CrossRef]
27. Guzev, M. A., Nikitina, E. Y., Chernysh, E. V. (2021). V.P. maslov’s approach to the analysis of rank distributions. Russian Journal of Mathematical Physics, 28, 56–65. DOI 10.1134/S1061920821010064. [Google Scholar] [CrossRef]
28. Maslov, V. P., Maslova, T. V. (2006). On Zipf’s Law and rank distributions in linguistics and semiotics. Mathematical Notes, 80(5), 679–691. DOI 10.1007/s11006-006-0189-1. [Google Scholar] [CrossRef]
29. Marfin, E., Gavrilov, A., Abdrashitov, A., Kadyirov, A. (2019). Pressure build-up test under elastic-wave action on the reservoir. AIP Conference Proceedings, vol. 2181, no. 1, 020018. Crimea, Russia. DOI 10.1063/1.5135678. [Google Scholar] [CrossRef]
30. Mullakaev, M. S., Abramov, V. O., Abramova, A. V. (2017). Ultrasonic automated oil well complex and technology for enhancing marginal well productivity and heavy oil recovery. Journal of Petroleum Science and Engineering, 159, 1–7. DOI 10.1016/j.petrol.2017.09.019. [Google Scholar] [CrossRef]
31. Hamida, T., Babadagli, T. (2008). Displacement of oil by different interfacial tension fluids under ultrasonic waves. Colloid Surface A, 316(1–3), 176–189. DOI 10.1016/j.colsurfa.2007.09.012. [Google Scholar] [CrossRef]
Cite This Article
 Copyright © 2023 The Author(s). Published by Tech Science Press.
Copyright © 2023 The Author(s). Published by Tech Science Press.This work is licensed under a Creative Commons Attribution 4.0 International License , which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.


 Submit a Paper
Submit a Paper Propose a Special lssue
Propose a Special lssue View Full Text
View Full Text Download PDF
Download PDF Downloads
Downloads
 Citation Tools
Citation Tools