Fluid Dynamics & Materials Processing

---

DOI: 10.32604/fdmp.2022.021345

ARTICLE

Numerical Simulation of a Two-Phase Flow with Low Permeability and a Start-Up Pressure Gradient

Xuanyu Dong1,* and Jingyao Yang2

1School of Geosciences, Yangtze University, Wuhan, 430100, China
2Gas Production Plant 5, Changqing Oilfield Company of PetroChina, Xi’an, 710000, China
*Corresponding Author: Xuanyu Dong. Email: dongxuanyu2022@163.com
Received: 10 January 2022; Accepted: 20 April 2022

Nomenclature

ρo, ρw:	Density of oil and water respectively (kg/m3);
krw, kro:	Relative permeability of water phase and oil phase (mD);
μo, μw:	Viscosity of oil Phase and Water Phase MPa s;
po, pw:	Pressure of oil phase and water phase in Reservoir (MPa);
Sw, So:	Saturation of water phase and oil phase;
t:	Production time (day);
qosc, qwsc:	Source-sink phase under standard conditions (m3/day);
G:	Phase starting pressure gradient;
v:	flow velocity.

1  Introduction

Due to the rapid growth of global oil and gas consumption and the rapid development of the oil industry, traditional medium and high permeability oil and gas resources are becoming increasingly depleted. As a result, many ultra-low permeability reservoirs are being constructed and used [1]. With the increasing demand for energy, it is more important than ever to study the construction of low-permeability reservoirs [2].

Darcy’s law has long been considered a cornerstone of reservoir engineering and flow dynamics. Reservoirs with limited permeability differ from ordinary reservoirs in the following ways. First, the physical properties of the reservoir are unusually poor; second, the oil recovery rate and oil recovery are low [3]. This is because the rocks of low-permeability reservoirs are compact, stress-sensitive, and have other physicochemical characteristics. Due to the weaknesses of these low permeability reservoir rocks, formation fluid flow is impeded. As a result, the fluid flow does not follow Darcy’s law and can only flow when a specific initial pressure gradient is overcome. The lack of natural energy in the reservoir leads to a rapid decrease in information pressure and a rapid decrease in production [4]. According to previous studies, the formation pressure drop in low permeability reservoirs ranges from 3 to 10 MPa per 1% of geologic reserves, while the formation pressure drop in selected high-pressure anomalous reservoirs reaches 10 MPa per 1% of geologic reserves. In low permeability reservoirs, the classical version of Darcy’s law is not sufficient to describe the flow pattern. Compared to reservoirs with moderate or high permeability, field development practice shows that. Wells with low permeability produce very little oil per day. Without the fracturing process, there is no natural production capacity. Production is quickly depleted and the ability to sustain production is limited. Water injection wells have extremely limited water absorption capacity [5]. As a result of these problems, development capacity is compromised and ultimately the efficiency of oil recovery is compromised. Therefore, to stabilize and increase the production of low permeability reservoirs, we must invest significant resources to study the flow mechanisms and numerical simulations of ultra-low permeability reservoirs. At present, the reservoir numerical simulation software based on Darcy’s linear flow equation has shown remarkable performance in reservoir engineering calculations for medium and high permeability fields. However, since it does not consider the flow characteristics of low-permeability reservoirs, it cannot meet the production needs and its applicability is somewhat limited [6].

The characteristics of low permeability reservoirs are very different from those of Darcy’s law. Darcy’s law does not apply to the rational evolution of reservoirs with limited permeability. Song et al. [7] also studied the two-phase flow of water-driven oil in low-permeability reservoirs using Buckley Leverett’s unmixed two-phase flow displacement theory. They studied the effect of the initial pressure gradient. They determined the analytical solution formula for oil-water two-phase flow in low-permeability reservoirs under different water-driven conditions. Ji [8] studied the pressure propagation and distribution characteristics of non-Darcy flow in low-permeability reservoirs. In endless formations, when single injection and production wells are used, the pressure in medium or high permeability reservoirs decreases rapidly at the wellbore and gradually between injection and production wells. In low permeability reservoirs, the pressure drops rapidly between injection and production wells, and between near wells as well. Radius limits for low permeability reservoirs are determined after modeling endless formations. The limit radius decreases as the initial pressure gradient increases and the pressure wave stop decreasing as it approaches the limit radius. In addition, because the low-rate non-Darcy’s law is different from Darcy’s law, its flow law, production equation, and well design are incompatible with low-permeability and ultra-low-permeability reservoirs. Helset et al. [9] studied relative permeability when capillary forces were considered and proposed that the flow of injected water under reservoir conditions could be simulated at low injection rates to accurately reflect relative permeability measurements. Torabi et al. [10] studied the effect of temperature effect on the permeability profiles of heavy oil and water phases. It was found that the relative permeability of oil and water increases when the viscosity of the oil phase decreases, and the increase in the injection flow rate leads to high relative permeability of the oil and low relative permeability of the water. The Corey equation was modified by experimental data and the accuracy of the equation was verified by theoretical studies, and the modified equation was more consistent with the relative permeability curves of heavy oil and water than the Corey equation. Prada et al. [11] derived the flow curve by single-phase flow experiments on artificial cemented cores and natural cores with different permeability. It is noted that the flow curve has a nonlinear connection at low velocity flow. As the flow rate increases, the flow curve becomes a linear segment. The different flow parameters of the same liquid in different media indicate that the pore structure characteristics of porous media have a great influence on the flow rate of low-velocity non-Darcy flow.

Pore size, pore throat radius, shape, and distribution of reservoir rocks all have an impact on fluid flow capacity. The nonlinear flow associated with low permeability rocks is caused by the pore throat size, unconnectedness, and the permeability of low permeability rocks. According to Li et al. [12], in porous media with low permeability, the size of the pore throat can cause the Jamin effect, blockage, and other phenomena that are not conducive to smooth flow. When the fluid passes through the pore throat of the rock, the capillary force increases sharply due to the small radius of the throat. Prada et al. [11] conducted flow experiments with low concentration brine on natural and artificial cores of different permeability and confirmed that different pore structures correspond to different flow characteristics, which can fully explain the flow characteristics caused by the pore structure of the reservoir rock.

Numerical reservoir simulation is an important technical tool and a basic tool for reservoir management such as oil field development programs, adjustment programming, and dynamic forecasting. In a sense, reservoir numerical simulation is one of the important tools for modern reservoir development [13–15]. In recent years, due to the rapid development of computers, reservoir numerical simulation technology has made great progress. Especially, in terms of calculation methods, program design, and image processing of calculation results. Therefore, reservoir numerical simulation plays an increasingly important role in modern reservoir development and management. At present, the more advanced reservoir numerical simulation software, such as eclipse, workbench, CMG, VIP, etc., are almost all based on the Darcy flow model, which is very suitable for developing oil and gas fields with medium and high permeability. On the other hand, when the fluid flows through the ultra-low permeability porous media, the flow pattern is nonlinear and the initial pressure gradient is small. Numerous scholars have enhanced the current conventional reservoir numerical simulation techniques to investigate the nonlinear flow characteristics of fluids in ultra-low permeability reservoirs. Currently, fluid flow in ultra-low permeability porous media is a nonlinear flow with a minimum initial pressure gradient. Therefore, the classical Darcy’s law cannot fully represent the flow law of low-permeability reservoirs. The numerical simulation software based on Darcy’s flow model has some limitations in the application of ultra-low permeability reservoirs [16]. Although the variable permeability numerical simulation method can accurately describe the nonlinear flow law of ultra-low permeability porous media. However, it cannot yet reflect the continuity and smoothness of the equation of state, so this numerical simulation method is still in the research stage [17].

This work discusses the characteristics of oil-water two-phase nonlinear flow in low-permeability reservoirs, as well as the initial pressure gradient. In a low permeability reservoir, we have performed numerical simulations of oil-water two-phase nonlinear flow. In this study, a one-dimensional conceptual example and a two-dimensional heterogeneous low-permeability example were developed to justify the simulation calculations.

2  Mathematical Model

2.1 Basic Assumptions of the Model

a) the phases are not mixed during the oil drive; b) the effect of the starting pressure gradient is considered; c) the flow of water and oil follows the modified Darcy’s law; d) the formation contains only oil-water two-phase fluids with the same direction of motion. The rocks are water-wet considering the microscopic compressibility of rocks and fluids; e) the effects of capillary forces and gravity are not considered; f) the formation is homogeneous in direction and thickness; g) the reservoir temperature is kept constant.

2.2 Continuity Equation

The mathematical model of oil-water two-phase motion equation can be written as follows, without considering the influence of capillary force and gravity:

∇[λo(∇P−Go)]+qosc=∂[ϕρoSo]∂t (1)

∇[λw(∇P−Gw)]+qwsc=∂[ϕρwSw]∂t (2)

2.3 Auxiliary Equation

For the above differential equation of oil and water continuous flow, the following auxiliary equations must be given

So+Sw=1 (3)

Relative permeability equation:

Kro=Kro(Sw),Krw=(Sw) (4)

2.4 Initial and Boundary Conditions

The beginning conditions are the saturation and pressure distributions at various sites in the reservoir at the start of production or from a specific period. The law of fluid on the outer geometric boundary is an example of an outer boundary condition; the internal pinch out, the flow law of fault, production well, and water injection well are examples of an inner boundary condition.

3  Low-Velocity Non-Darcy Flow Model

3.1 Solution of the Continuity Equation

The left and right ends of expansion Eqs. (1) and (2) can obtain:

∇[(λon+λwn)∇(Po−Go)n+1]−[ϕ(CoSo+CwSw)Δt]n(Pon+1−Pon)=0 (5)

∇(λwn∇(P−Gw)n+1)−(ϕΔt)n(Swn+1−Swn)=0 (6)

For Eqs. (5) and (6), the IMPES method is adopted, that is, the pressure is solved implicitly and the saturation is solved explicitly. However, in the treatment of variable parameters, there is no unique method to select the values of and Q. generally speaking, when selecting these values, the most accurate flow (including the cumulative flow in the block and the flow into the grid block) should be given. In this paper, the upstream weight method is used to obtain the variable parameters. The grid system is solved by a block central grid, which is shown in Fig. 1.

Figure 1: Block center grid

In reservoir numerical simulation, the solution method of linear equations is the most important stage. The ultra-low permeability reservoir nonlinear flow mathematical model is a system of nonlinear partial differential equations. Using the various separation and discretization methods outlined above, nonlinear partial differential equations are turned into nonlinear algebraic equations. The nonlinear algebraic equations are turned into linear equations after coefficient linearization, and the matrix is solved to yield the requisite pressure and saturation distribution.

First, the pressure solution is obtained by using the definite solution conditions, replace the pressure into Eq. (6) to explicitly calculate the saturation. This value is the pressure and saturation value of the corresponding period. After calculation Swn+1 , calculate the new pressure Pn+1 , and the pressure will be explicitly used in the next time step. Many coefficients at the right end of the equation are in unknown time order and must be iterated. The amplification coefficient must also be updated at any time during the iteration.

3.2 Low-Velocity Non-Darcy Flow Curve

In this paper, the quasi-starting pressure algorithm is adopted, which is a widely used method at present. The basic expression is:

{v=kμ(ΔPL−G)v=0ΔPL≥GΔPL<G (7)

This method considerably lowers the problem’s complexity and gives a straightforward solution for the theoretical analysis of flow in porous media with low permeability. The downside is that the curved portion with a tiny pressure gradient is omitted, which means that the genuine flow pattern of low-velocity non-Darcy flow is not captured in the calculation and simulation. It is included in the simulator as an optional computation module because it is very simple to use and frequently utilized at the moment.

4  Example Verification

The oil-water two-phase nonlinear flow model of a low permeability reservoir has been developed, with the effects of the initial pressure gradient and gravity taken into account. This is the foundation for the mathematical model of nonlinear oil-water two-phase flow in a low permeability reservoir. Many examples will be utilized throughout this chapter to evaluate the model and calculate the program’s validity.

4.1 One-Dimensional Model

Firstly, an injection production model is established for simulation calculation. From Table 1, we can see the main parameters of the reservoir static geological model and water injection development simulation. The size of the model is 510 m * 20 m * 20 m, and the grid is divided into 51 * 1 * 1, a total of 51 grids. The oil-water phase permeability used in the reservoir is shown in Fig. 2.

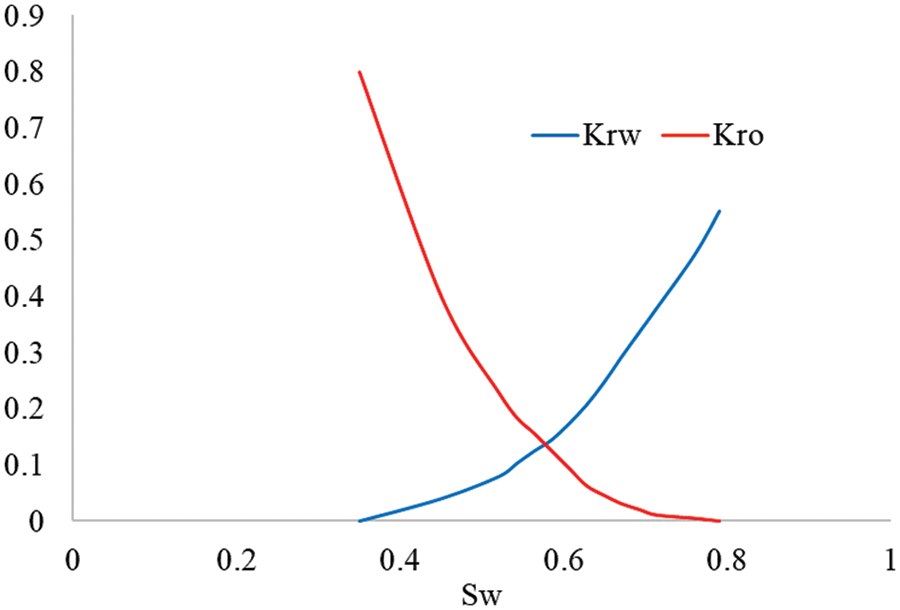
Figure 2: Relative permeability curve

The initial permeability of the reservoir is shown in Fig. 3. It can be seen that the permeability in this paper is homogeneous and the permeability is 0.5 MD. The example is used for simulation calculation for 12 years. The fixed injection volume of the injection well is 10 m3/day, and the fixed production volume of the production well is 10 m3/day. The calculated daily oil production curve and water cut of the single well are shown in Figs. 4 and 5.

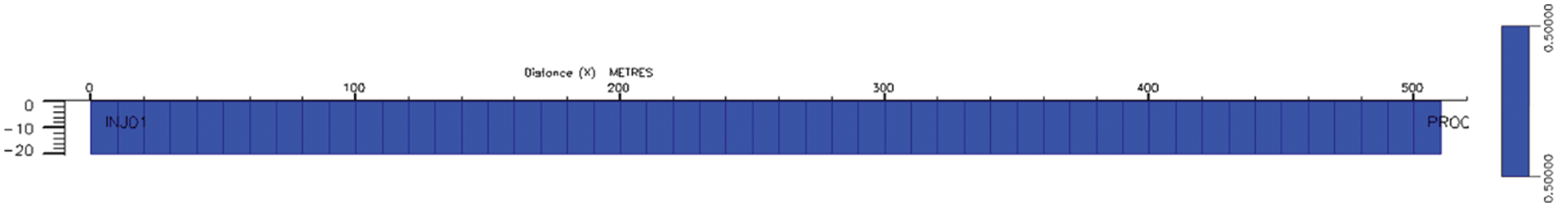
Figure 3: Permeability distribution field

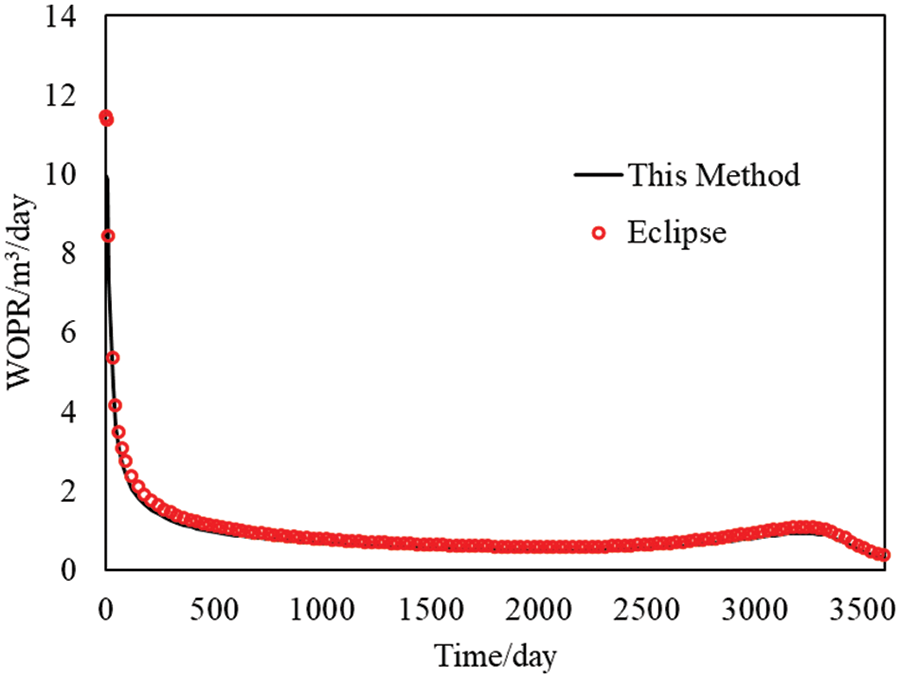
Figure 4: Single well daily oil production curve

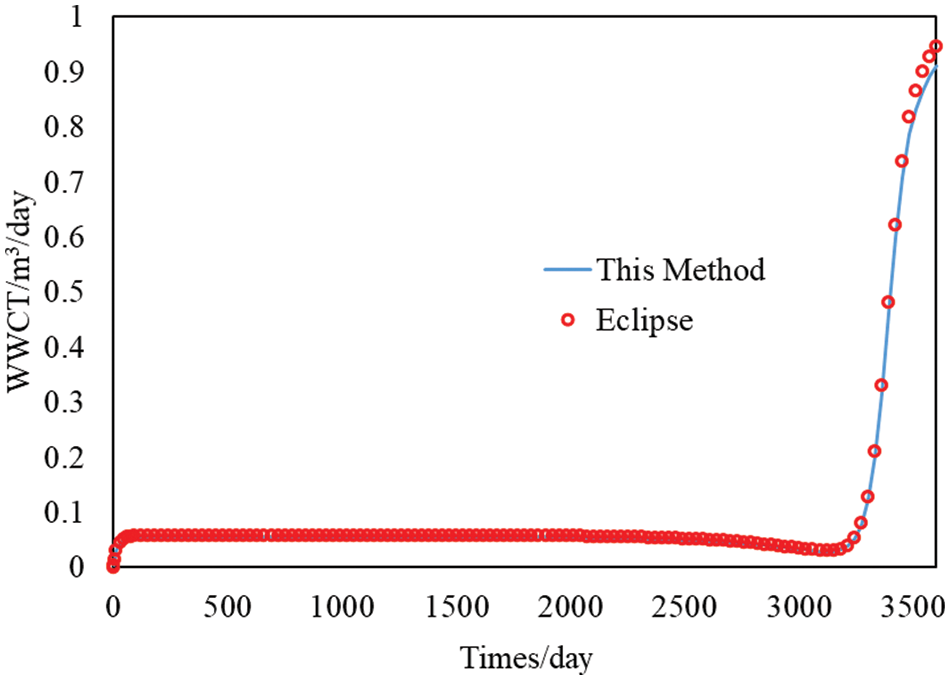
Figure 5: Single well water cut curve

It can be seen from Figs. 4 and 5 that the production wells are exhausted at the initial stage of production, and the daily oil production decreases greatly. And by comparing with the traditional commercial numerical simulator Eclipse, we found that the calculation results of this paper are almost the same as the commercial numerical simulator. When the production time is about 3000 days, the production well begins to see water, and the water cut rises sharply. Daily oil production increased slightly and then began to decline. This is mainly because the pressure front just reaches the production well, which will temporarily increase production. However, because of water, oil production will decline rapidly.

4.2 Parameter Sensitivity Analysis

In this paper, a two-dimensional heterogeneous low-permeability reservoir model is constructed, and its permeability distribution is shown in Fig. 6, with the unit of MD. The size of the reservoir model is 210 m * 210 m * 10 m, and the model grid is divided into 21 * 21 * 1, a total of 421 grids. The relative permeability curve of the model is shown in Fig. 7. The well group of one injection and four productions is used for production. The injection rate is 12 m3/day and the recovery rate is 3 m3/day. Some physical properties can see from Table 2.

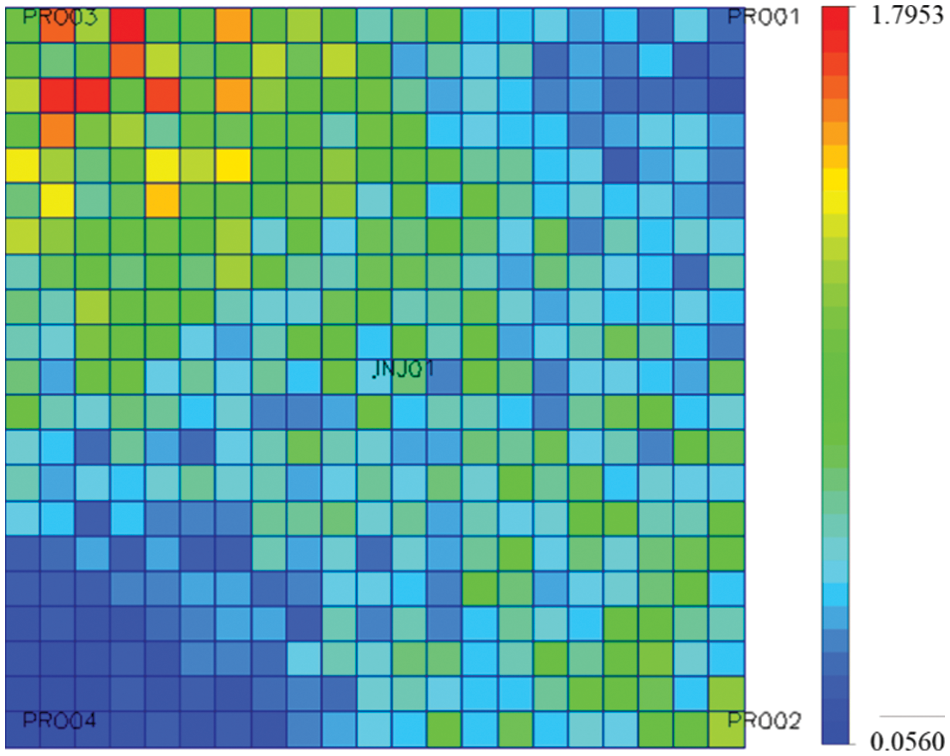
Figure 6: Reservoir permeability distribution

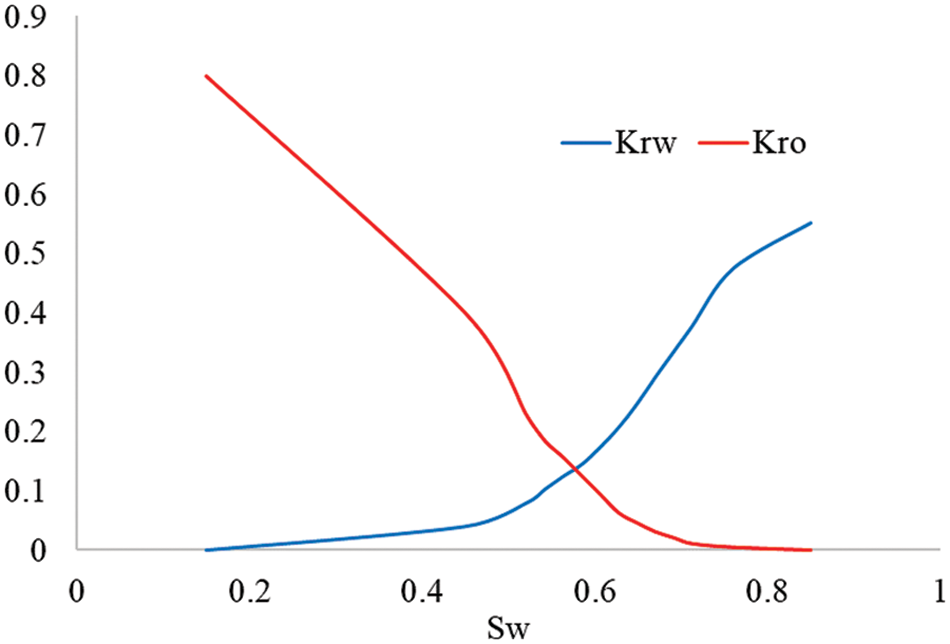
Figure 7: Relative permeability curve

4.3 Initial Water Saturation

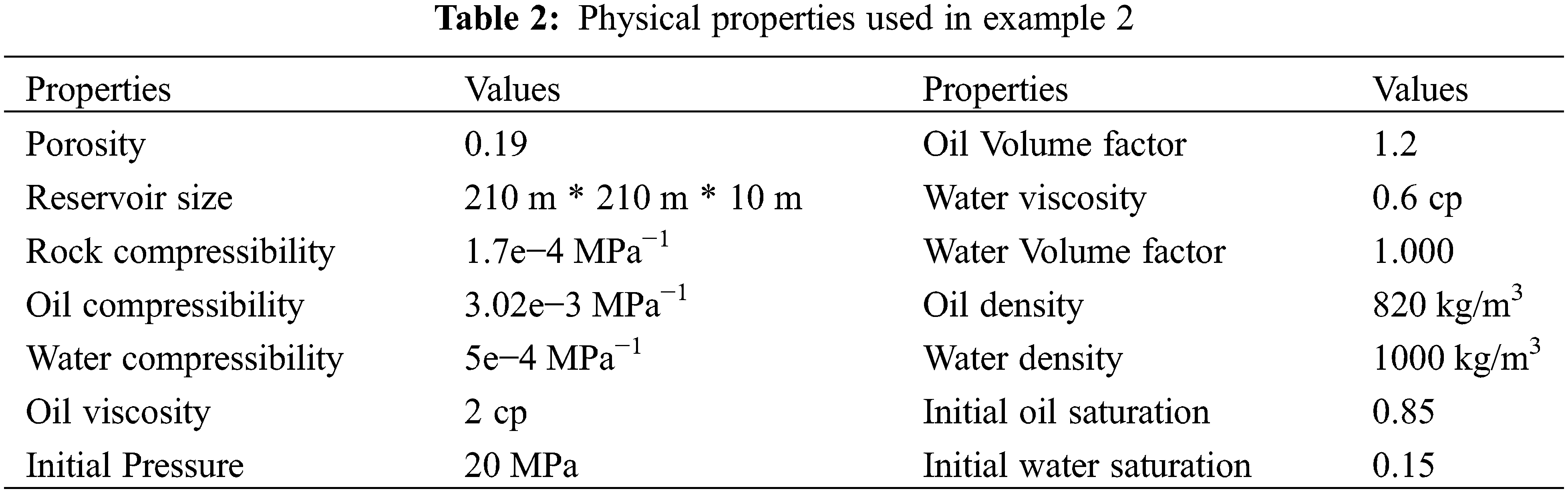
The original water saturation of a low permeability reservoir is high, and the size of water saturation has a direct impact on oilfield development. In order to study the influence of water saturation on oil well water production, the water saturation is designed to be 0.15, 0.25, 0.35, and 0.45, respectively.

As can be seen from Figs. 8 and 9, when the initial water saturation increases from 0.15 to 0.45, crude oil production gradually decreases. When the initial water saturation is 0.15 and 0.25, the cumulative oil production is 19,539 and 15,556 m3. When the original water saturation increases from 0.25 to 0.35, the cumulative oil production decreases to about 5000 m3, and the water cut increases by about 25%. When the original water saturation is 0.45, the cumulative oil production decreases rapidly to 6750 m3 and the water cut increases rapidly to 0.94.

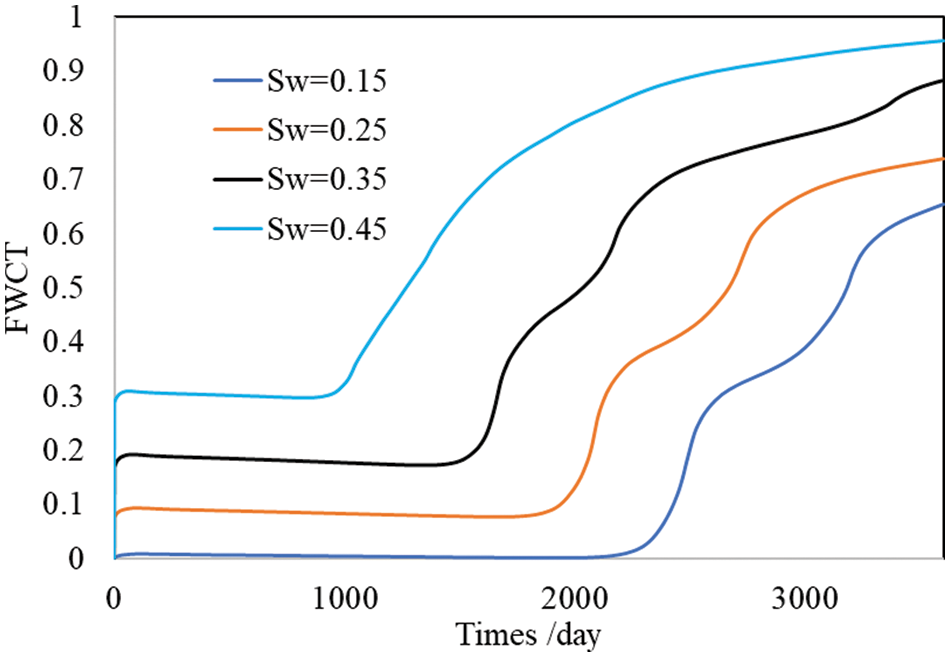
Figure 8: Block water cut curve

Figure 9: Block cumulative oil production curve

It can be seen from Fig. 10 that the daily oil production rate of the four oil-producing wells decreases significantly with the increase of water saturation. Due to the low permeability of well pro1 and well PRO4, their daily oil production belongs to depletion development from the beginning. With the continuous production, the pressure spread here, and the daily oil production increased slightly. The daily oil production at well PRO2 decreased first and then increased from exhaustion development to pressure spread. However, after the water breakthrough in the later stage, the daily oil production rate decreased significantly. The permeability at well PRO3 is relatively high, so the daily oil production rate is high. However, it was also faster to see water, and the output decreased significantly in the later stage.

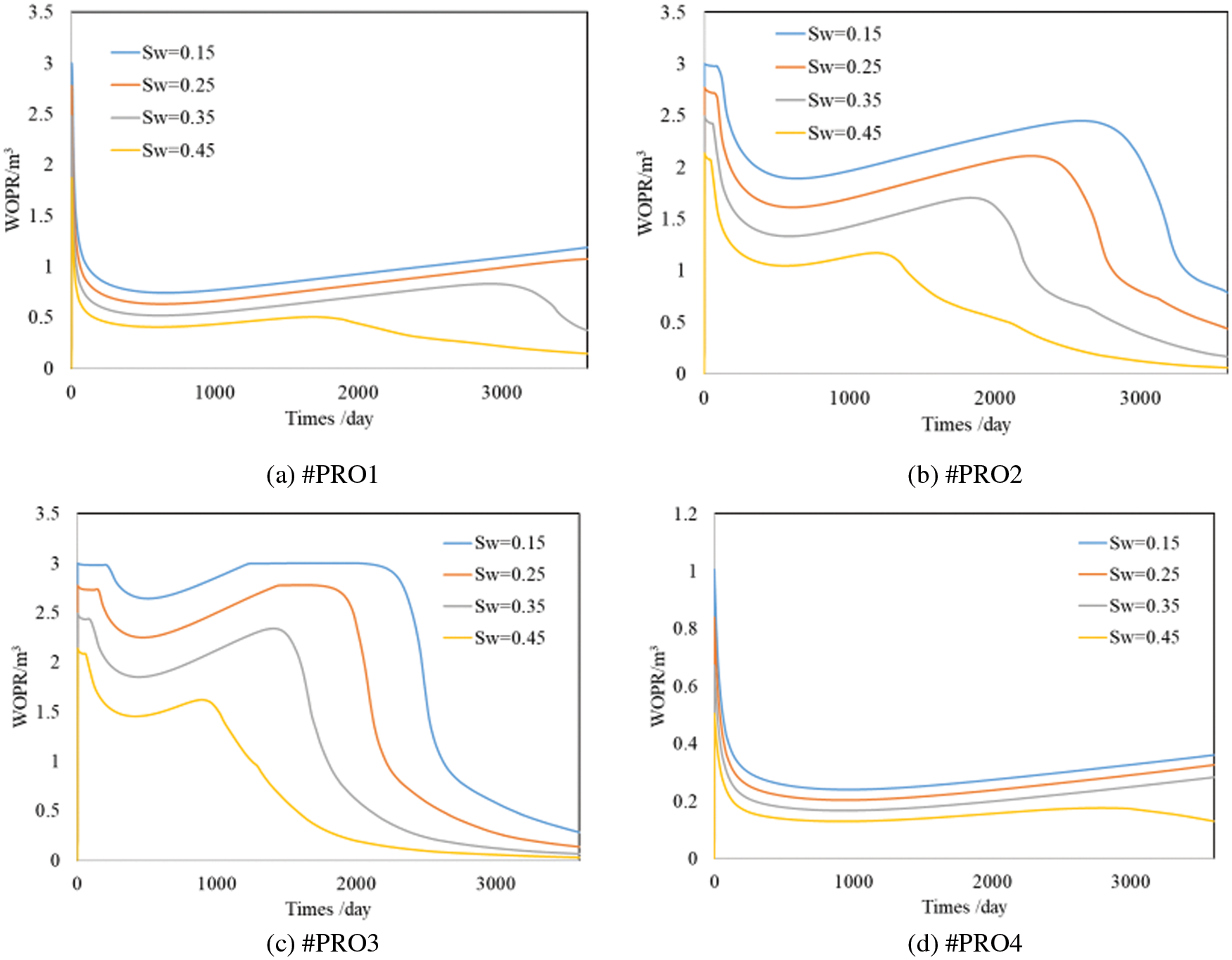
Figure 10: Comparison of daily oil production from a single well

5  Conclusion

Numerical simulations of oil-water two-phase nonlinear flow in low-permeability reservoirs were performed using the characteristics of oil-water two-phase nonlinear flow in low-permeability reservoirs and the starting pressure gradient. The following results and conclusions are presented:

1)   A mathematical model of oil-water two-phase nonlinear flow in a low-permeability reservoir with an onset pressure gradient is established, a numerical algorithm for the grid system is constructed, and a numerical example is carried out using nonlinear flow theory and the oil-water two-phase nonlinear flow model in a low-permeability reservoir with an onset pressure gradient.

2)   In this study, a one-dimensional homogeneous model is developed. The daily oil production and water cut of a single low-permeability model well were examined to demonstrate the accuracy of the algorithm. Depletion development was found to be the early stage, during which the daily oil production rate of the reservoir declined rapidly. Although the pressure sweep boosted production at the beginning, it declined rapidly after the water break.

3)   In this work, a two-dimensional heterogeneous low-permeability reservoir model with varying initial water saturation was constructed and investigated. When the initial water saturation grows, the water cut of the block continues to rise and the cumulative oil production gradually decreases. As the water saturation increases, the daily oil production of a single well decreases.

Acknowledgement: Thanks to Eclipse commercial numerical simulation software.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

Reference

 1.  Xu, J., Jiang, R., Xie, L., Wang, R., Shan, L. et al. (2012). Non-darcy flow numerical simulation for Low-permeability reservoirs. SPE Europec/EAGE Annual ConferenceCopenhagen, Denmark. DOI 10.2118/154890-MS. [Google Scholar] [CrossRef]

 2.  Su, H., Zhang, Y., Xiao, B., Huang, X., Yu, B. (2021). A fractal-monte carlo approach to model oil and water two-phase seepage in low-permeability reservoirs with rough surfaces. Fractals, 29(1), 2150003. DOI 10.1142/S0218348X21500031. [Google Scholar] [CrossRef]

 3.  Jiang, R., Li, L., Xu, J., Yang, R., Zhuang, Y. (2012). A nonlinear mathematical model for low-permeability reservoirs and well-testing analysis. Acta Petrolei Sinica, 33(2), 264–268. DOI 10.7623/syxb201202011. [Google Scholar] [CrossRef]

 4.  Yang, Q., Yang, Z., Wang, Y., Ji, H. (2007). Study on flow theory in ultra-low permeability oil reservoir. Drilling Production Technology, 30(6), 52. [Google Scholar]

 5.  Mahani, H., Keya, A. L., Berg, S., Nasralla, R. (2018). Electrokinetics of carbonate/brine interface in low-salinity waterflooding: Effect of brine salinity, composition, rock type, and pH on?–Potential and a surface-complexation model. SPE Journal, 22(1), 53–68. DOI 10.2118/181745-PA. [Google Scholar] [CrossRef]

 6.  Bartels, W. B., Mahani, H., Berg, S., Hassanizadeh, S. (2019). Literature review of low salinity waterflooding from a length and time scale perspective. Fuel, 236, 338–353. DOI 10.1016/j.fuel.2018.09.018. [Google Scholar] [CrossRef]

 7.  Song, F. Q., Liu, C. Q., Li, F. H. (1999). Transient pressure of percolation through one dimension porous media with threshold pressure gradient. Applied Mathematics Mechanics, 20(1), 27–35. DOI 10.1007/BF02459270. [Google Scholar] [CrossRef]

 8.  Ji, B. Y. (2006). Understanding of reservoir study in daqing oil field. Petroleum Geology Oilfield Development in Daqing, 1. [Google Scholar]

 9.  Helset, H., Nordtvedt, J., Skjaeveland, S., Virnovsky, G. (1998). Three-phase relative permeabilities from displacement experiments with full account for capillary pressure. SPE Reservoir Evaluation Engineering, 1(2), 92–98. DOI 10.2118/36684-PA. [Google Scholar] [CrossRef]

10. Torabi, F., Mosavat, N., Zarivnyy, O. (2016). Predicting heavy oil/water relative permeability using modified corey-based correlations. Fuel, 163, 196–204. DOI 10.1016/j.fuel.2015.09.035. [Google Scholar] [CrossRef]

11. Prada, A., Civan, F. (1999). Modification of Darcy’s law for the threshold pressure gradient. Journal of Petroleum Science Engineering, 22(4), 237–240. DOI 10.1016/S0920-4105(98)00083-7. [Google Scholar] [CrossRef]

12. Li, D. P., Luo, D. Q. (1994). The special development Law of Low permeability oilfield──Part 1 Development of Low permeability oilfield. Fault-Block Oil Gas Field, 4. [Google Scholar]

13. Rao, X., Xin, L., He, Y., Fang, X., Gong, R. et al. (2022). Numerical simulation of two-phase heat and mass transfer in fractured reservoirs based on projection-based embedded discrete fracture model (pEDFM). Journal of Petroleum Science Engineering, 208, 109323. DOI 10.1016/j.petrol.2021.109323. [Google Scholar] [CrossRef]

14. Rao, X., Zhan, W., Zhao, H., Xu, Y., Liu, D. et al. (2021). Application of the least-square meshless method to gas-water flow simulation of complex-shape shale gas reservoirs. Engineering Analysis with Boundary Elements, 129, 39–54. DOI 10.1016/j.enganabound.2021.04.018. [Google Scholar] [CrossRef]

15. Xu, Y., Sheng, G., Zhao, H., Hui, Y., Zhou, Y. et al. (2021). A new approach for gas-water flow simulation in multi-fractured horizontal wells of shale gas reservoirs. Journal of Petroleum Science Engineering, 199, 108292. DOI 10.1016/j.petrol.2020.108292. [Google Scholar] [CrossRef]

16. Xu, Y., Hu, Y., Rao, X., Zhao, H., Zhong, X. et al. (2021). A fractal physics-based data-driven model for water-flooding reservoir (FlowNet-fractal). Journal of Petroleum Science Engineering, 109960. [Google Scholar]

17. Zhou, Y., Xu, Y., Rao, X., Hu, Y., Liu, D. et al. (2021). Artificial neural network-(ANN-) based proxy model for fast performances’ forecast and inverse schedule design of steam-flooding reservoirs. In: Mathematical problems in engineering, vol. 2021. DOI 10.1155/2021/5527259. [Google Scholar] [CrossRef]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.