A New Approach for the Calculation of Slope Failure Probability with Fuzzy Limit-State Functions
Jianing Hao1, Dan Yang2, Guanxiong Ren1, Ying Zhao3, Rangling Cao4,*
1 Department of Engineering Management, Sichuan College of Architectural Technology, Deyang, 61800, China
2 Department of Transport and Municipal Engneering, Sichuan College of Architectural Technology, Deyang, 61800, China
3 Department of Basic Teaching, Sichuan College of Architectural Technology, Deyang, 61800, China
4 Sichuan Hongye Construction Software Co., Ltd., Chengdu, 610081, China
* Corresponding Author: Rangling Cao. Email: 
Fluid Dynamics & Materials Processing https://doi.org/10.32604/fdmp.2024.054469
Received 29 May 2024; Accepted 06 November 2024; Published online 25 November 2024
Abstract
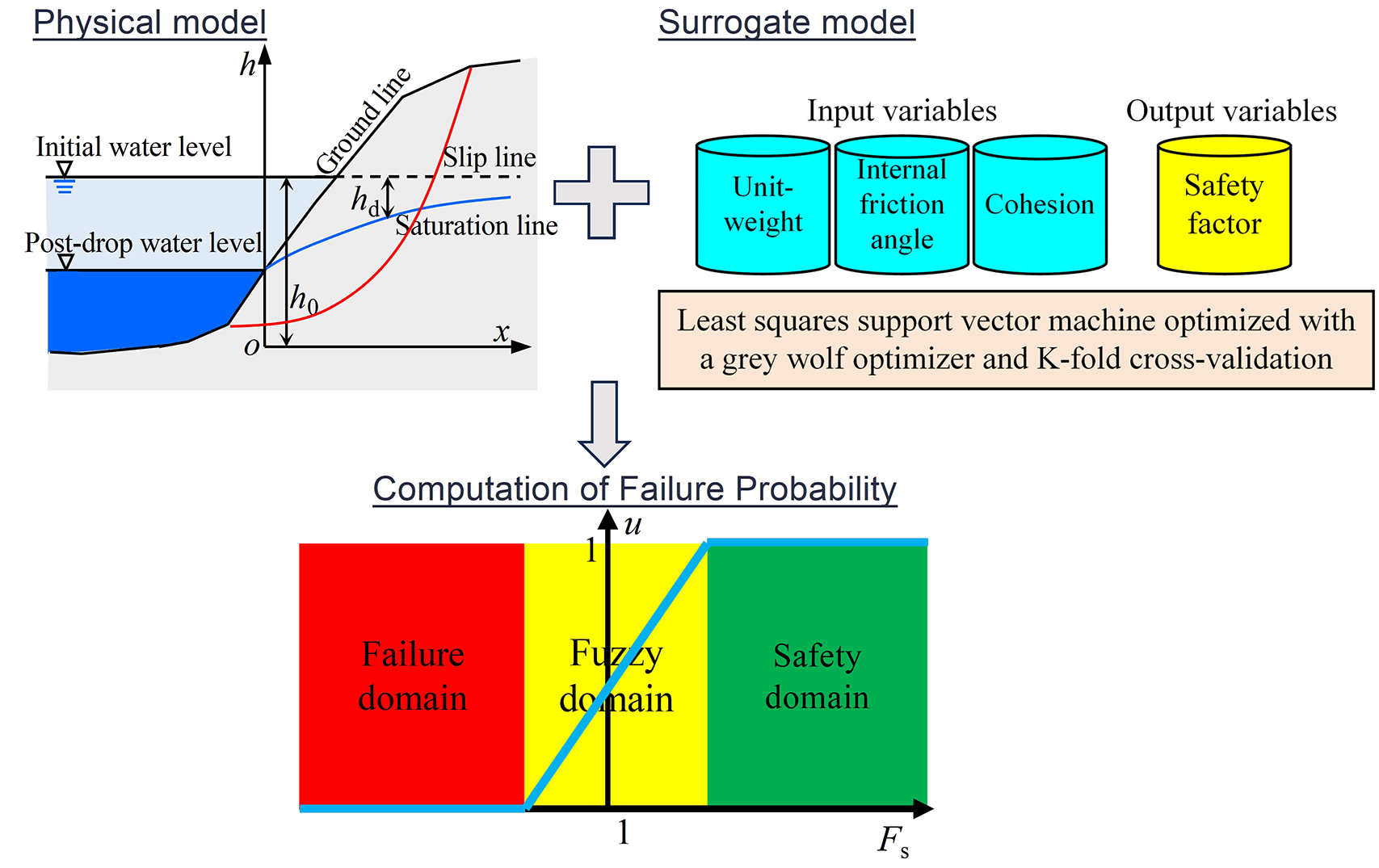
This study presents an innovative approach to calculating the failure probability of slopes by incorporating fuzzy limit-state functions, a method that significantly enhances the accuracy and efficiency of slope stability analysis. Unlike traditional probabilistic techniques, this approach utilizes a least squares support vector machine (LSSVM) optimized with a grey wolf optimizer (GWO) and K-fold cross-validation (CV) to approximate the limit-state function, thus reducing computational complexity. The novelty of this work lies in its application to one-dimensional (1D), two-dimensional (2D), and three-dimensional (3D) slope models, demonstrating its versatility and high precision. The proposed method consistently achieves error margins within 3% of Monte Carlo simulation (MCS) results, while substantially reducing computation time, particularly for 2D and 3D models. This makes the approach highly practical for real-world engineering applications. Furthermore, by applying fuzzy mathematics to handle uncertainties in geotechnical properties, the method offers a more realistic and comprehensive understanding of slope stability. As water is the main factor influencing the stability of slopes, this aspect is investigated by calculating the phreatic line after the change in water level. Relevant examples are used to show that the failure probability of a slope under water wading condition can increase by more than 20% (increase rates in 1D, 2D and 3D conditions being 25%, 27% and 31%, respectively) compared with the natural condition. The influence of diverse fuzzy membership functions—linear, normal, and Cauchy—on failure probability is also considered. This research not only provides a strategy for better calculation of the slope failure probability but also pioneers the integration of computational intelligence, fuzzy logic and fluid-dynamics in geotechnical engineering, presenting an innovative and efficient tool for slope stability analysis.
Graphical Abstract
Keywords
Least Squares Support Vector Machine (LSSVM); Grey Wolf Optimizer (GWO); slope stability analysis; fuzzy set theory; failure probability estimation
Open Access
Login
Register
Submit a Paper
Propose a Special lssue
Download PDF
Downloads
Citation Tools
