

 | Energy Engineering |  |
DOI: 10.32604/EE.2021.016413
ARTICLE
Using the Taguchi Method and Grey Relational Analysis to Optimize the Performance of a Solar Air Heater
1Department of Mechanical Engineering, School of Engineering, The University of Jordan, Amman, Jordan
2Department of Mechanical Engineering, Faculty of Engineering and Technology Al-Zaytonah University of Jordan, Amman, Jordan
3Department of Mechatronics Engineering, Faculty of Engineering, The Hashemite University, Zarqa’, Jordan
*Corresponding Author: Manar B. AL-Hajji. Email: m.hajji@ju.edu.jo
Received: 04 March 2021; Accepted: 04 June 2021
Abstract: Solar energy is regarded as one of the promising renewable energy sources in the world.The main aim of this study is to use the Taguchi-Grey relational grade analysis to optimize the performance of two Solar Air Heaters (SAHs). A typical Grey–Taguchi method was applied. The Orthogonal Array, Signal-to-Noise ratio, Grey Relational Grade, and Analysis of Variance were employed to investigate the performance characteristics of SAH. Experimental observations were made in agreement with Jordanian climate 32°00′ N latitude and 36°00′ E longitude with a solar intensity of 500 W\m2. The operating factors selected for optimization are the tilt angle (T) with three levels (0°, 22°, 45°), inlet velocity (V) with two levels (1.2, 1.8 m/s), and absorber plate material (M) with two levels (Aluminum, wood). In this study, the Grey–Taguchi approach is validated by performing 12 individual experiments. The results show that the process factors sequence required for a maximum SAH efficiency (SAH µ) is V > T > M. Using this approach, we combined the Orthogonal Array design with Grey Relational Analysis. As a result of that, the level of each operating conditions which optimizes both process responses (Temperature difference, ∆T and Solar air heater efficiency, SAH µ) can be specified with a minimum number of tests compared with classic Grey Relational Analysis. The optimal operating conditions of a SAH for multiple performance characteristics are determined as T2, M2, and V2, respectively, which are in congruence with the experimental results.
Keywords: Solar air heater; collector efficiency; thermal efficiency; grey-taguchi method; robust design
Nomenclature
| ∆T: | Temperature difference (°C) |
| yj(k): | Value of process response k at replicationj |
| Xj(k): | Normalized value of each process response |
| ξi(k): | Grey relational coefficient |
| ∆oj(k): | Absolute value difference between xo(k) and xj(k) |
| xo(k): | Reference value |
| m: | Mass flow rate of air (kg/s) |
Abbreviations
| SAH: | Solar Air Heater |
| CFD: | Computational Fluid Dynamics |
| THC-VGs: | Truncated Half Conical Vortex Generators |
| GRA: | Grey Relational Analysis |
| Re: | Reynolds number |
| p/e: | Pitch ratio |
| Nu: | Nusselt number |
| TEF: | Thermal Enhancement Factor |
| FEF: | Friction Factor Enhancement Factor |
| THPP: | Thermohydraulic performance parameter |
| DOE: | Design of Experiment science |
| f: | Friction factor |
| OA: | Orthogonal Array |
| S/N: | Signal-to-Noise ratio |
| SAHµ: | Solar Air Heater efficiency (%) |
| T: | Tilt angle (o) |
| M: | Material of solar plate |
| V: | The Inlet air velocity (m/s) |
| DOF: | Degree of Freedom |
| N: | Number of replications in each experiment |
| Cp: | Specific heat of air at constant pressure (kJ/kg.K) |
| A: | Surface area of the SAH (m2) |
| I: | Irradiance (W/m2) |
| Ac: | Constant cross section area of air outlet (m2) |
Greek Symbols
| α: | Angle of attack (o) |
| Ψ: | Distinguishing coefficient in the range 0 ≤ ψ ≤ 1 |
| γj: | Grey rational grade of experiment j |
| ρ: | Air density (kg/m3) |
Solar Air Heater (SAH) performance depends on many factors such as; collector orientation, thickness of cover materials, wind velocity, collector length, collector depth, and type of the used absorber material, etc. [1]. A wide range of research have been devoted to improving the SAHs’ performance. Some studies like [2,3] have attempted to improve the heat transfer rate by incorporating fins on absorbers plate. Several researchers [4] tried to provide roughness on the absorbing plate. Furthermore, many novel techniques such as; heat storage [5], jet impingement [6], and use of Nanofluids have been employed to improve heat transfer in different devices, including SAH [7,8].
Different optimization techniques have been used to pinpoint the proper geometric configuration for enhancing the performance factors that affect heat transfer [9,10]. Jawad et al. [11] carried out research with the goal of enhancing the performance of a SAH by adding tubes from aluminum chips, paraffin wax, and nano-SiC which have been fixed on the absorption plate of the SAH. This study was conducted according to the weather conditions of Baghdad in winter. It was found that the new composite material’s thermal conductivity increased by 18.2% when 3 wt.% of nano-Sic was added. Also, the heat capacity of the new composite decreased by 4.5% although the effect on the SAH performance did not change. The sustainability of the proposed SAH was proven by improving the performance and accelerating the heating rate. The tested SAH hit high heating degrees and went on to operate for not less than 3 h after sunset. Bensaci et al. [12] had developed a numerical and experimental study to improve the thermal and hydraulic performance of SAH by changing the baffle position inside the air channel. The numerical study investigated four cases corresponding to the different placement of baffles, regulating devices, with Reynolds numbers extending from 2370–8340. It was found that the right placement of baffles pointedly increases the thermo-hydraulic functioning of SAHs. The optimum value was obtained when the baffles were fixed in the first part of the air channel which occupies 50% of the SAH. Zhu et al. [13] carried out numerical optimization research on micro-heat pipe arrays based SAH.A3D computational fluid dynamics modeling (CFD) based on the physical heat transfer process in the SAH was introduced in this study. Airflow, how thick the air is, ambient temperature, air duct aspect ratio, and fins geometrical parameters were the process factors that were used in the optimizing the efficiency of the system and the thermal-hydraulic performance of airflow. This model was verified experimentally. It was found that the optimal operating conditions of the air heater are: an inlet velocity of the air heater with 3.3 m/s, the air layer thickness of 25 mm. The results also showed that the values investigated in the paper, including the optimal aspect ratio, the height of the fin, and the spacing of the fin are 0.25, 12, and 6 mm, respectively. Bezbaruah et al. [14] came up with a numerical study based on enhancing the thermohydraulic functioning of a SAH by adding truncated Half Conical Vortex Generators (THC-VGs) on the absorber plate. Grey Relational analysis (GRA) was used to specify the optimal configuration for the applicable range of Reynolds numbers (Re 3500–16000). The THC-VGs were adjusted at different relative pitch ratio (p/e) ranging from 2.67–6.67 while the angle of attack (α) lies between 0°–90°. Results were examined by calculating different process parameters such as; Nusselt number (Nu), Thermal Enhancement Factor (TEF), Friction factor (f), Friction Factor Enhancement Factor (FEF), and Thermohydraulic performance parameter (THPP). The results revealed a better thermal impact when VGs are placed at a 60° angle of attack. Also, it was noted that the highest percentage betterment was 187% in Nu for α = 60°. Jeffrey et al. [15] had applied the Taguchi method, GRA, and analysis of variance (ANOVA) to optimize the performance of a flat-plate collector. The process factors which were considered in this work include the material of the collector tube, endothermic plate material, the diameter of the tube, the number of collector tubes, and the type of the absorption film. Heat dissipation factor and efficiency coefficient were the response variables that were measured in this paper. The findings divulge that the mean values of heat dissipation and the efficiency coefficient dropped within a 95% confidence interval. Also, it was noticed that the absorber film type has a remarkable effect on the process responses.
The key goal of the current work is to use the Taguchi-Grey relational grade analysis to optimize the performance of two SAHs that were experimentally tested by Al Khalil et al. [16]. In the current study, we use the Taguchi–Grey method to detect the maximum setup to perform a strong statistical analysis of a SAH concerning the Jordanian climate. The operating factors selected for optimization include tilt angle with three levels (0°, 22°, 45°), inlet velocity with two levels (1.2, 1.8 m/s), and two different absorber plate (Aluminum, wood). The absorber plate of the first SAH was made of black painted wood, whereas the second one was made of the black painted Aluminum sheet.
2 Performed Experimental Research
Two SAHs were constructed of 1.0 m2 of black painted aluminum sheet and wood absorber plate. These two configurations were covered with a glass plate of 5 mm thickness. The components of each SAH are shown in Fig. 1:
a) SAH which is used to heat the atmospheric air which is introduced and flows inside the heater, and it is to leave the heater at a higher temperature.
b) Variable speed fan is used to introduce the atmospheric air into the heater at variable velocity; hence, there is a variable mass flow rate.
c) Arduino Controller from which the digital values of air velocity within each heater are obtained.
d) The inlet and outlet air temperature is measured by copper constantan thermocouples.
A metrological station was used to measure the metrological data (solar intensity and ambient temperature). For each stated air velocity at the inner space of the collector and after steady-state is reached (that is indicated for a constant air inlet and outlet air temperatures). The tilt angle was modified at different steps ranging from 15°–50° with 5° interval. This was repeated several times to determine the optimum tilt angle that gives the maximum increase in air temperature inside the collector. This procedure was repeated several times with different air velocities. Additional experiments were conducted to determine the optimal tilt angle during summer (22°) and winter (45°). Also, in order to a void shading effect, it was recommended to use 0° tilt angle.
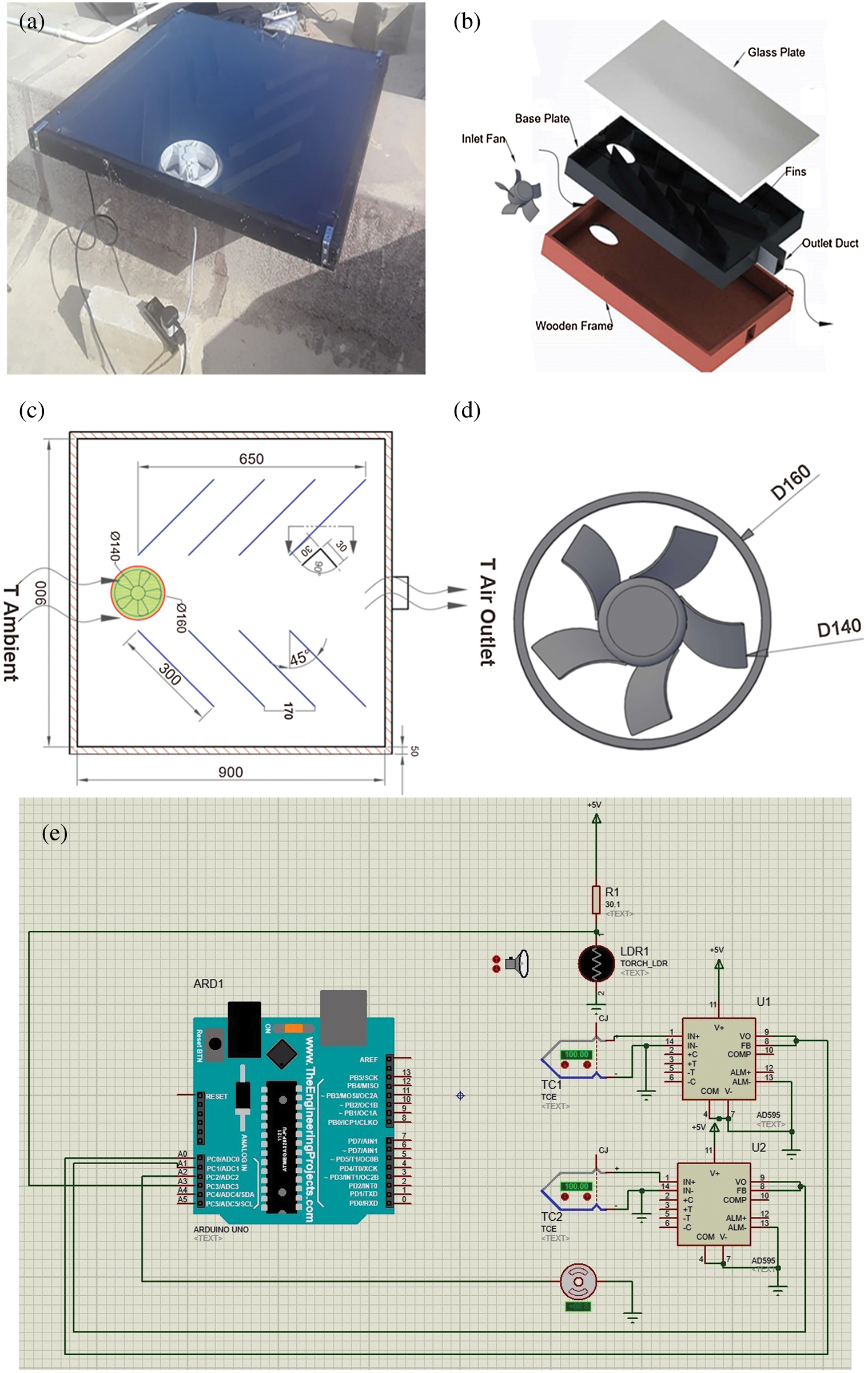
Figure 1: a) Experimental setup components, b) Solar collector components, c) Schematic illustration of SAH, d) Inlet fan cover, and e) Arduino controller
3.1 Optimization Using Grey–Taguchi
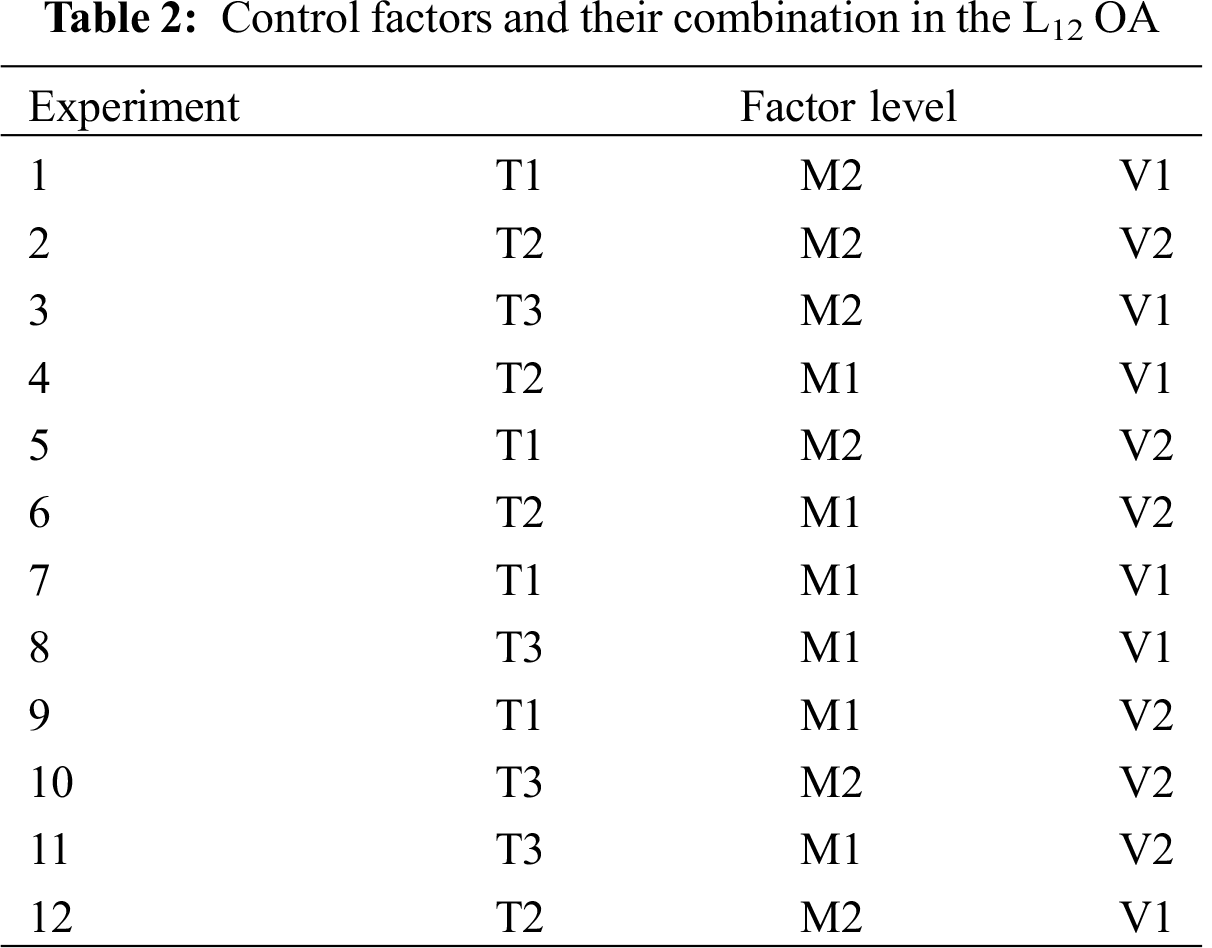
In the present study, the Taguchi technique and the GRA are employed to maximize the performance of a SAH. The Taguchi method is an analytical technique that belongs to Design of Experiment science (DOE). Taguchi method is used to reduce the number of tests that are necessary to measure the effects of different levels for certain control factors on a specified process response individually. Each test consists of one level of each control factor being used. The experimental design proposed by Taguchi involves an Orthogonal Array (OA) that organizes different parameters known to affect the process response and the levels at which they should be varied. The effect on process response is obtained by performing each experiment several times, followed by converting the results to Signal to Noise (S/N). According to this approach, experiments with the maximum S/N values represent the best levels of control factors [17,18]. Tab. 1 shows the control factors with the specified levels which are tested to optimize the performance of a SAH.
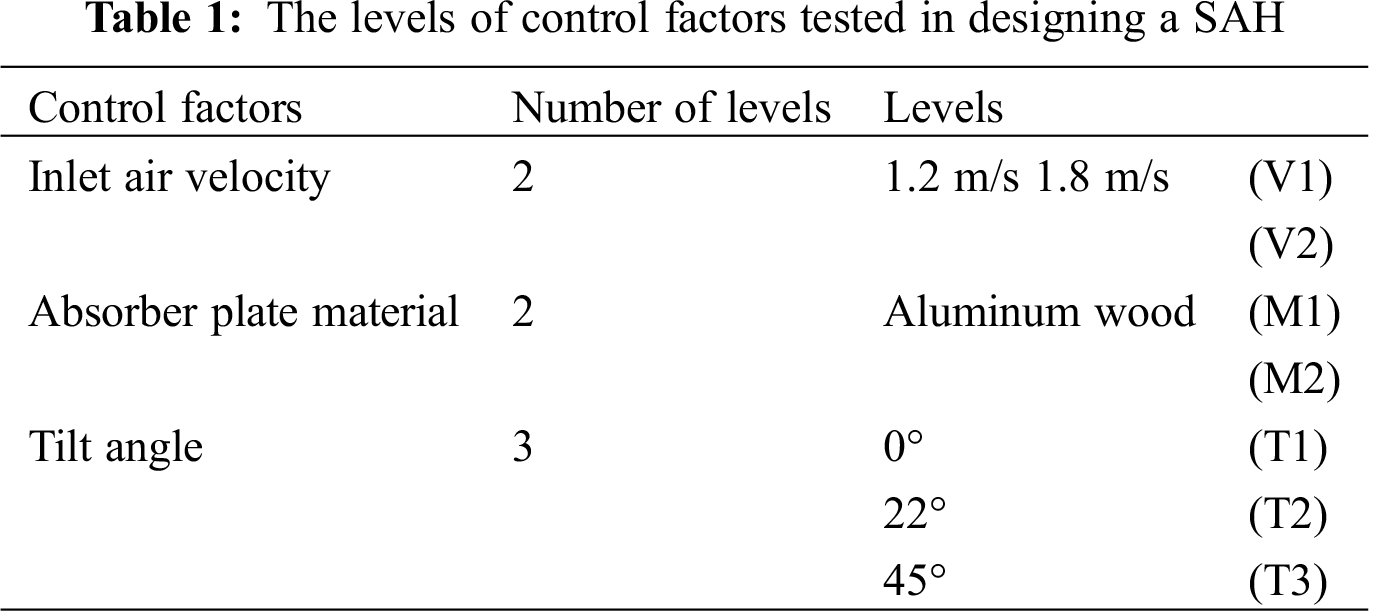
The temperature difference (∆T) and Solar Air Heater efficiency (SAHµ) are the two process responses that are considered in the current study. As it is stated in the Taguchi method, one degree of freedom (DOF) is regarded for each of the two control factors with two levels (Inlet air velocity, Absorber Plate Material respectively) and two DOFs are considered for the control factor with three levels (Tilt angle). The total DOF for the Taguchi method is (1 + 1 + 2) + 1 = 5. Therefore, the Taguchi design of a SAH must have at least 5 rows. Taguchi OA with L12 is employed in the experimental layout Tab. 2. The notation for this mixed OA is L12 (22
where n is the number of experimental measurements and yj(k) is the average measured value of process response k at replication j.
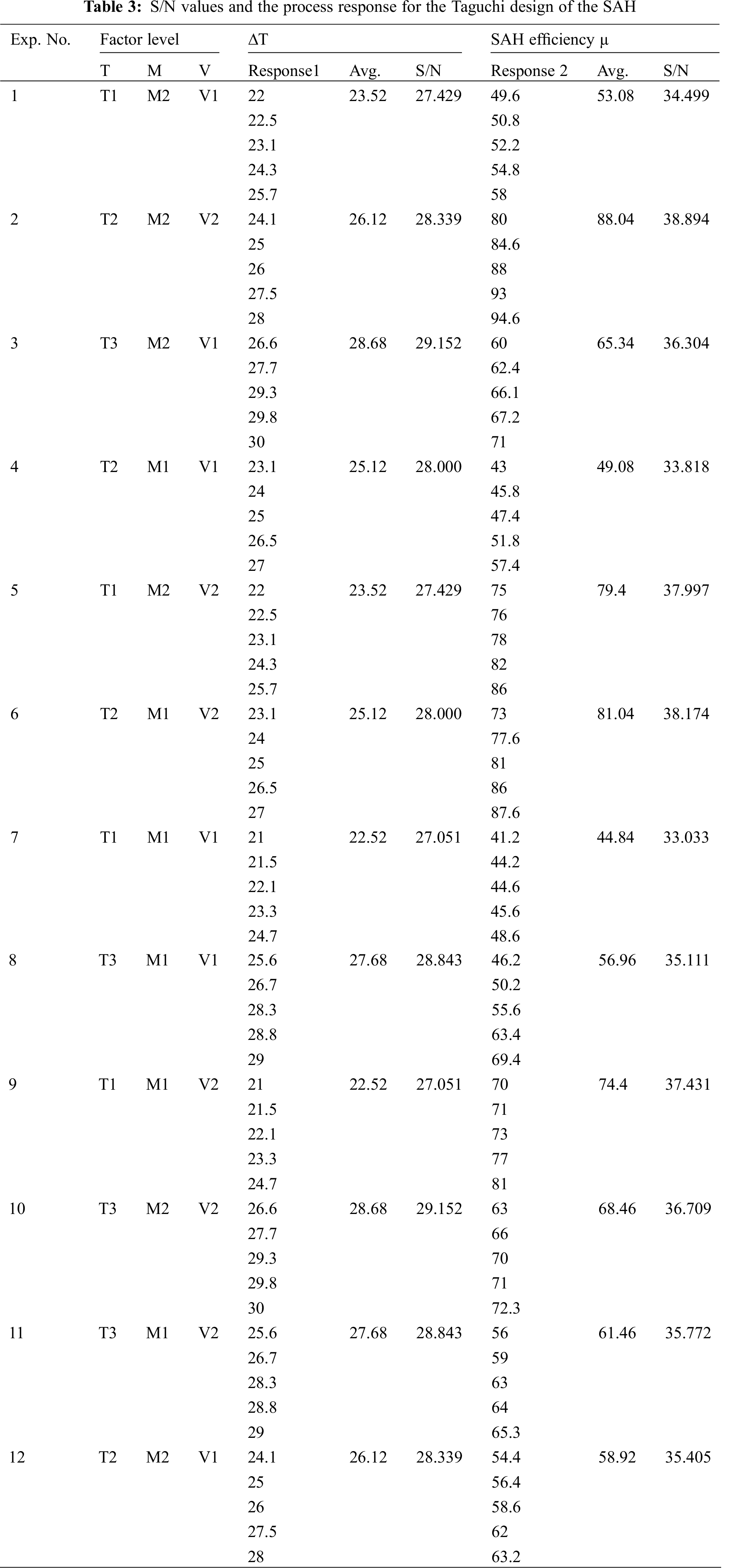
In this study of the SAH, the number of replications in all tests was set to be 1. Tab. 3 shows the average value of the response variables and the corresponding S/N ratios for all 12 experiments being carried out randomly. After that, the marginal average of ratios for each level of the control factors belonging to each process response values was found and listed in Tab. 4.
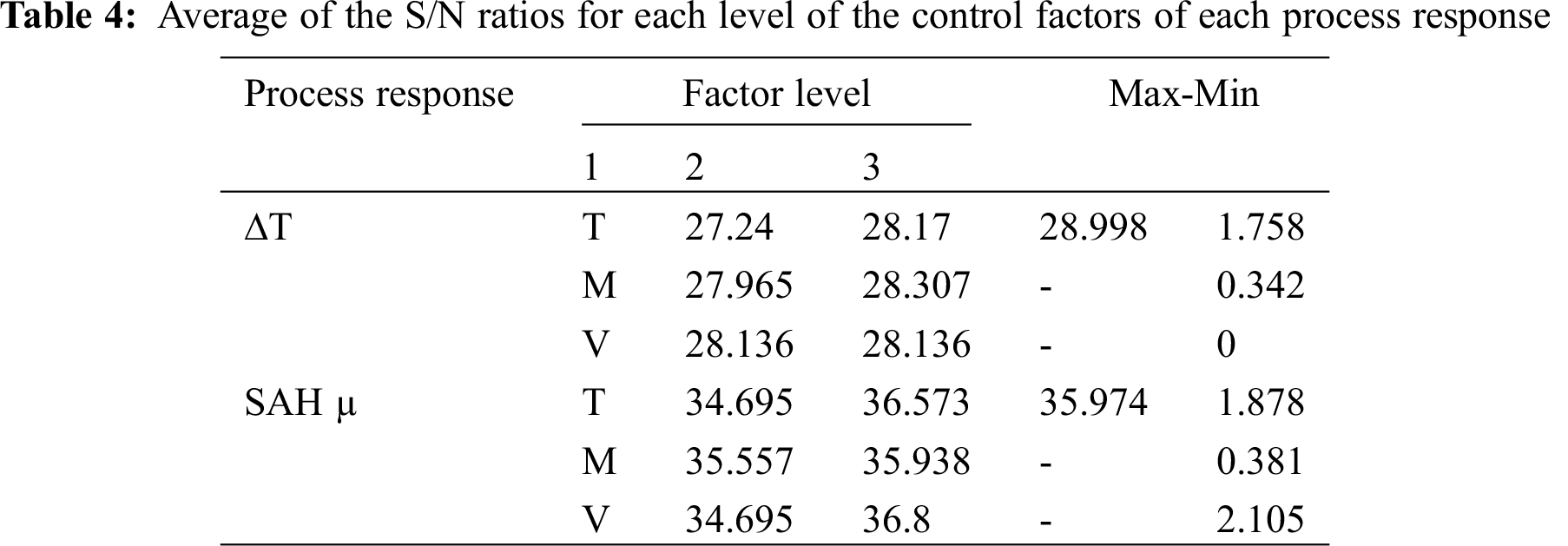
Since we have more than one process response (∆T, µ), the Taguchi approach cannot figure out the overall optimum conditions, so GRA is employed to select the best level of each control factor. By using the GRA, the observations of process response are combined into a single value called the GRG. After applying this concept, the experiment with the uppermost GRG has the best levels of the control factors. In this research, the OA which was used for the Taguchi design is applied in GRA to reduce the number of tests compared with classic GRA. The grey relation grades, which we got by the QA, were computed depending on the designated tests. After that, the GRGs were converted to S/N ratios. The technique of the Grey–Taguchi is illustrated in the following steps:
a) The value of each process response is linearly normalized in the range between zero and unity using Eq. (2).
∀j, k|k ∈ (the larger, the better the process responses).
b) For each response factor, the Grey relational coefficient ξj(k) is calculated by Eq. (3).
where ∆oj(k) is the deviation sequence = difference of the absolute value between xo(k) and Xj(k), the xo(k) is a reference value, it can be defined as the best-normalized value of process response k among all its experiments signifying that xo(k) = maxj{xj(k)}, and ∆min and ∆maxl are calculated as minj mink{∆oj} and maxj maxk{∆oj}, respectively. While ψ is a distinguishing coefficient which is defined in the range of 0 ≤ ψ ≤ 1, this value may be attuned depending on the application. In this work, the value ψ is = 0.5.
c) GRG of each experiment is found by Eq. (4).
where γj is the grey rational grade of experiment j.
For every test, the S/N ratio is computed from its GRG using the same equation (Eq. (1)) which is used for the Taguchi method. Thus, the average S/N ratio for all the levels of controlling factors or from S/N ratios of the grey relation grade is calculated. All the findings for the Grey–Taguchi design of the SAH, which were obtained from Steps (a)–(d), are listed in Tab. 5. Also, the marginal average of S/N ratios for the different levels of each control factor is shown in Tab. 6.
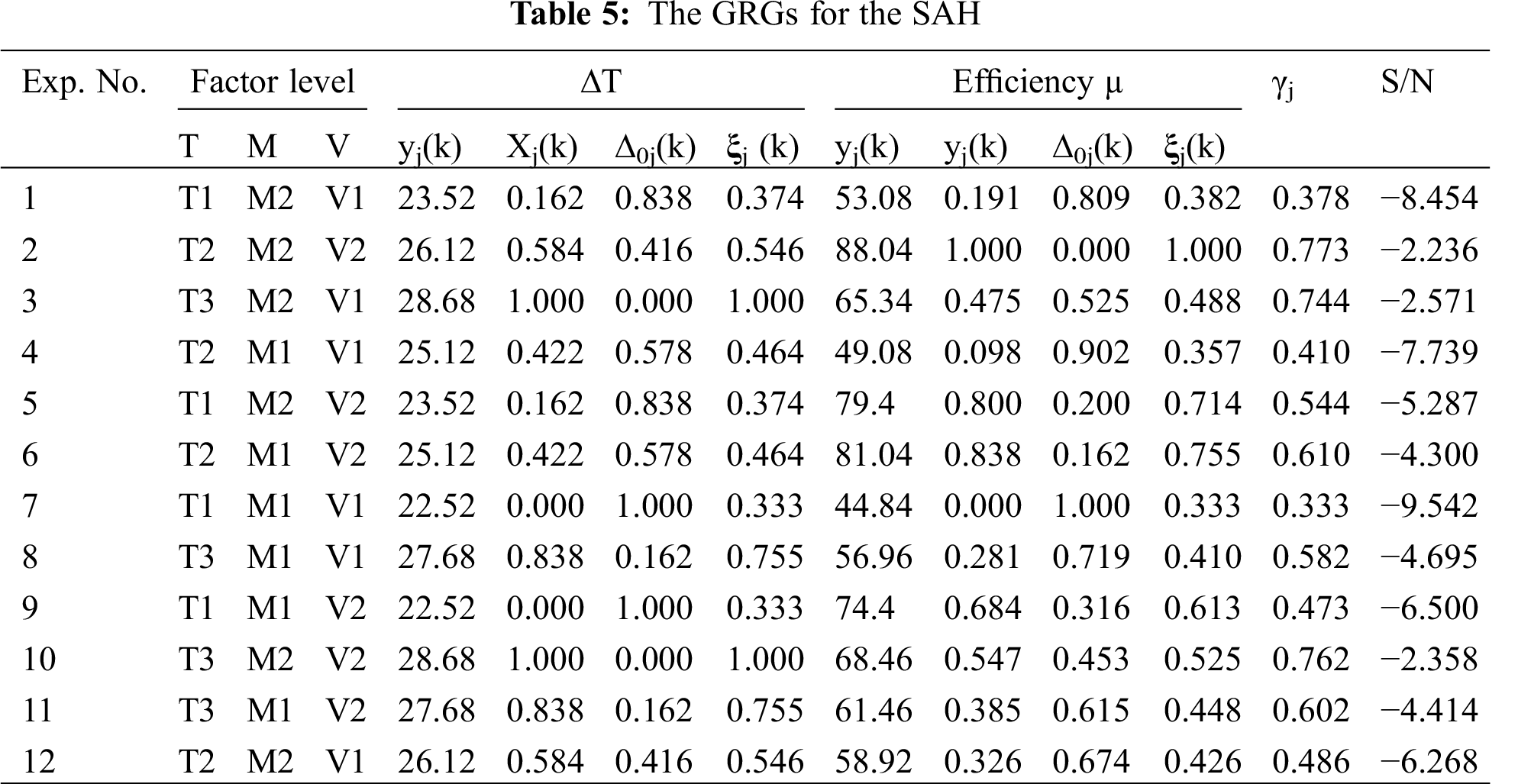
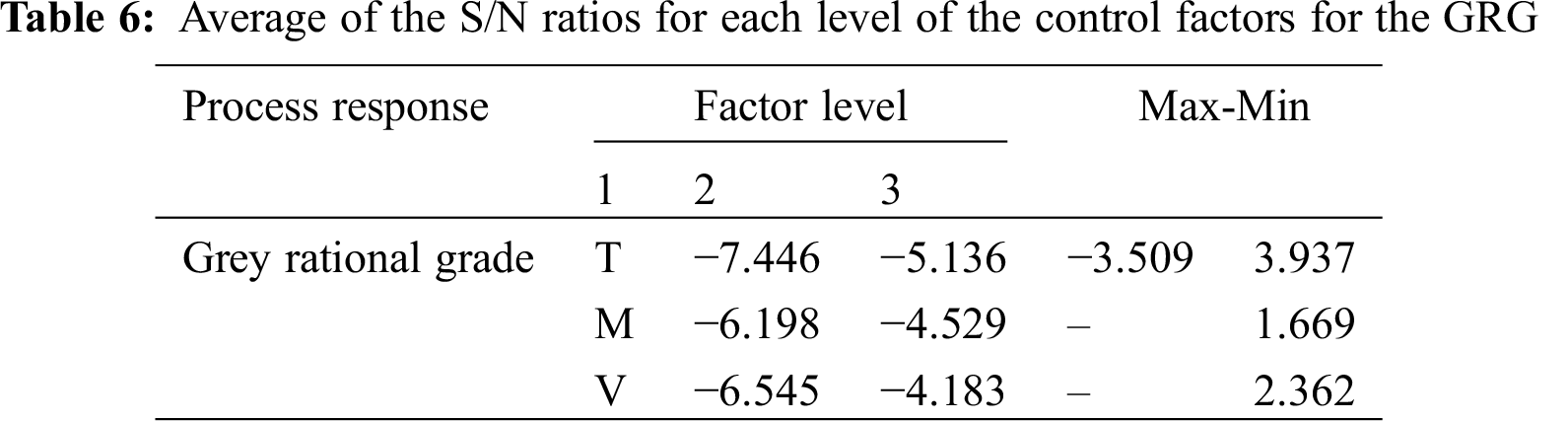
To optimize the performance of a SAH, the results in Tabs. 1–6 were analyzed to get the most effective control factor and the best level of each control factor. This analysis was performed for each process response (∆T, SAH µ, and GRG). The GRG, a combination of ∆T and SAH µ.S/N ratios, were analyzed by using two different methods to specify the most effective factor.
The first method, the dissimilarity between the highest and lowest marginal means of the levels of each factor was calculated and listed in Tab. 4 (concerning ∆T and SAH µ). A similar way was also used for the GRG and results which are listed in Tab. 6. The most effective factor in designing a SAH is considered when the difference between max and min is the highest value. Based on the above-mentioned sentence and based on the obtained results of both ∆T and SAHµ together (as shown below), the most effective factor is the tilt angle (T):
• Based upon the ∆T control factor, the most effective factor is the tilt angle (T).
• Based upon SAH µ, the most effective factor is the Inlet air velocity (V).
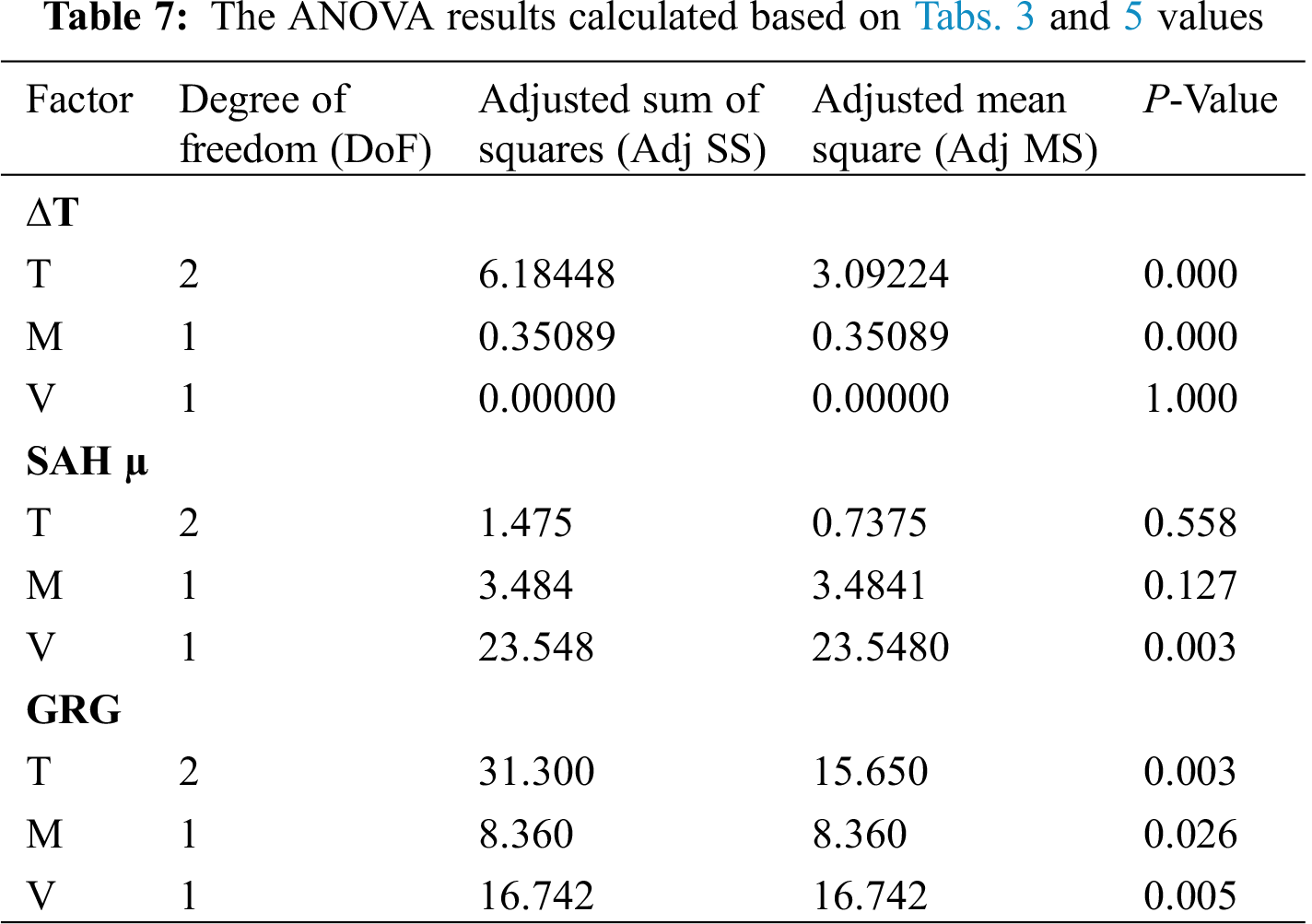
The second method, ANOVA is utilized to decide the most effective factor in designing a SAH. ANOVA is performed on S/N ratios of the Taguchi and Grey–Taguchi designs for each factor which is mentioned in Tabs. 3 and 5. According to the results listed in Tab. 7 below, the control factor with the highest contribution is chosen as the most effective factor. Based on ANOVA results which consider each control factor, the most contributing control factor is the same as what we have got by the first technique as it is stated above.
To determine the perfect level of each controlling factor, the marginal S/N ratios were being calculated for ∆T, SAH µ making use of the GRG. According to these S/N ratio values, the level with the uppermost S/N ratio is the most effective. The marginal S/N ratios were independently represented for all levels of the control factors in Fig. 2 where Fig. 2a presents the marginal S/N ratio for all levels of the factors when ∆T is considered, Fig. 2b presents the marginal S/N ratio for all levels of the factors when SAH µ is regarded, and Fig. 2c illustrates the marginal S/N ratio for all levels of the factors combining both ∆T and SAH µ.
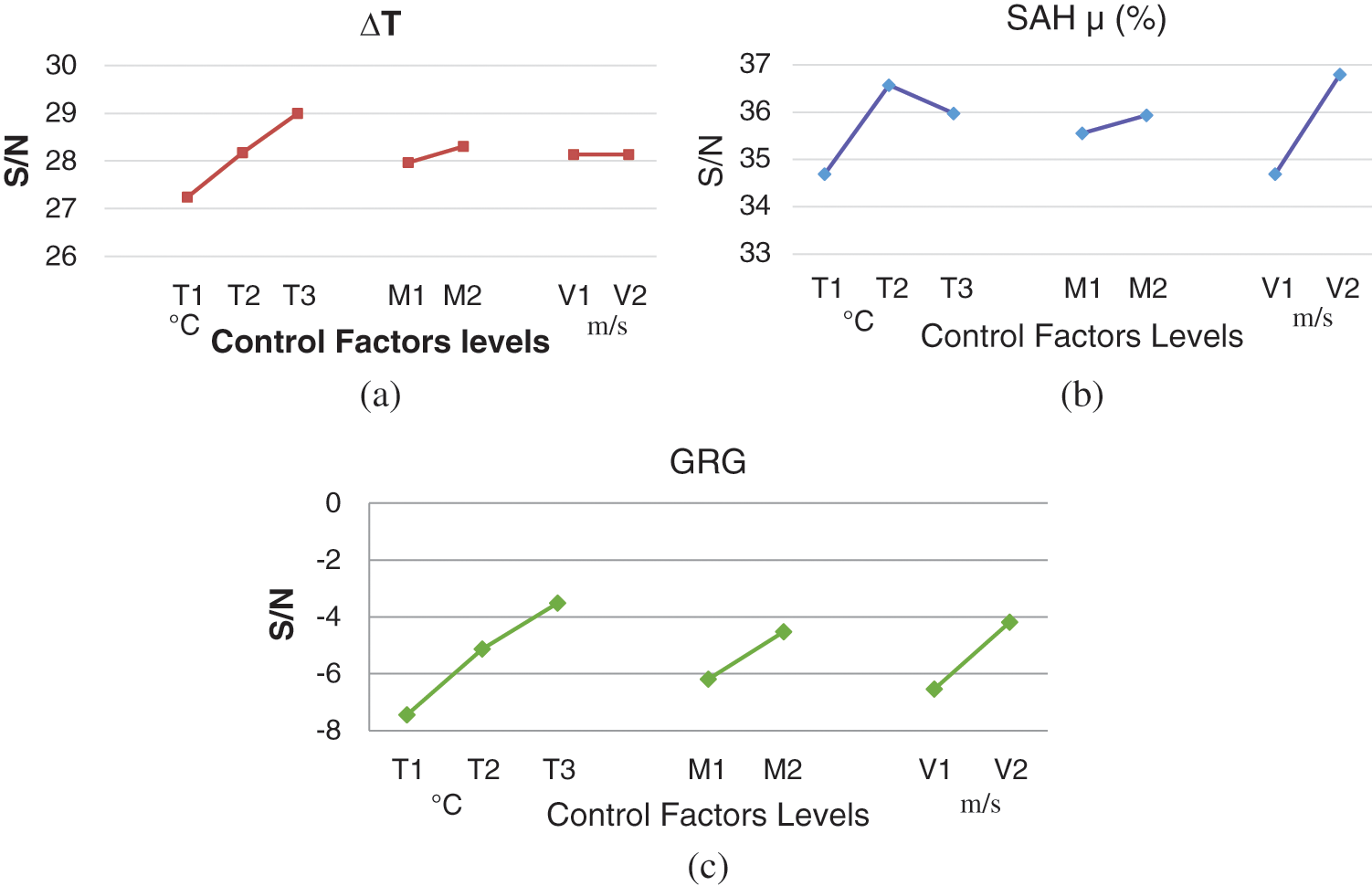
Figure 2: The marginal S/N ratio for all Levels of the factors when (a) ∆T is Considered, (b) SAH µ is Considered, and (c) Combining both ∆T and SAH µ
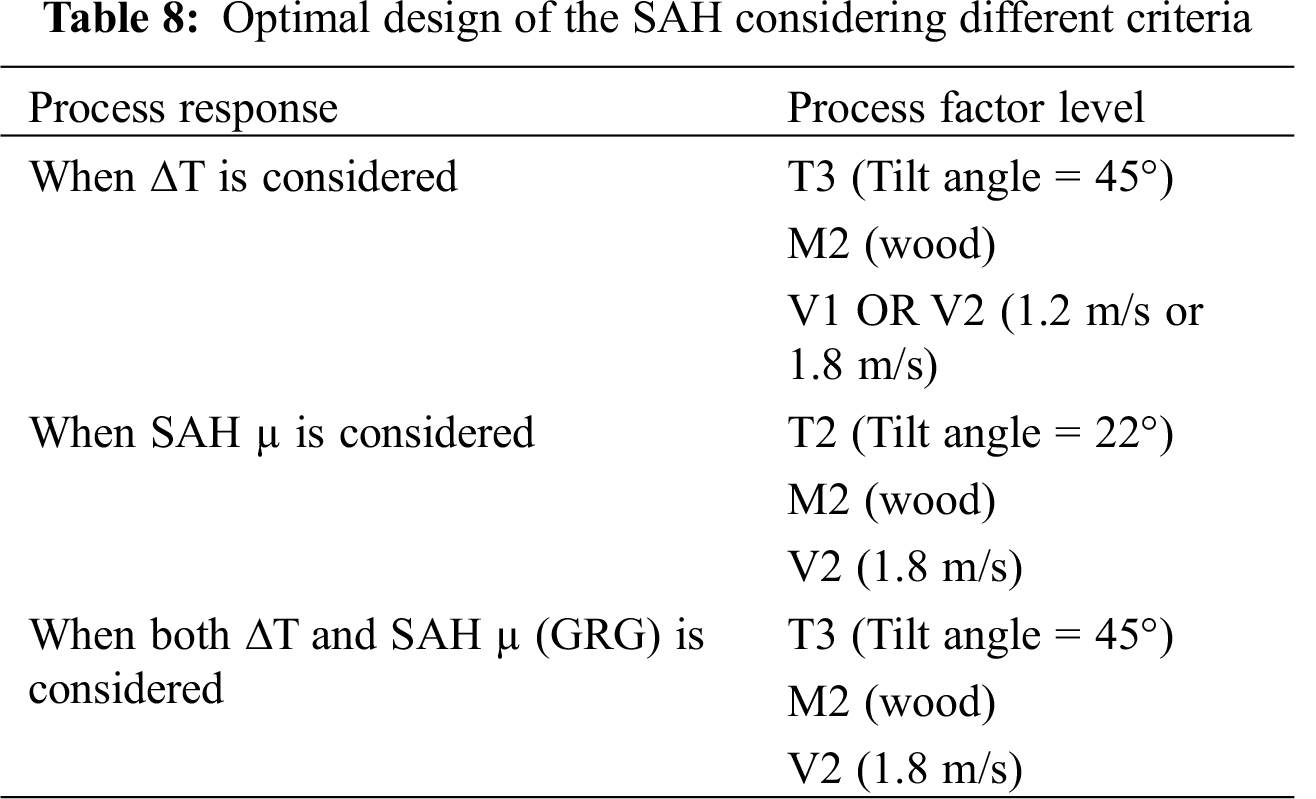
To summarize the results mentioned earlier, designing optimal SAH based upon each criterion requires selecting the factor level with the optimal S/N ratio. As a final point, the optimal Design based on ∆T, SAH µ, and GRG is individually reported in Tab. 8.
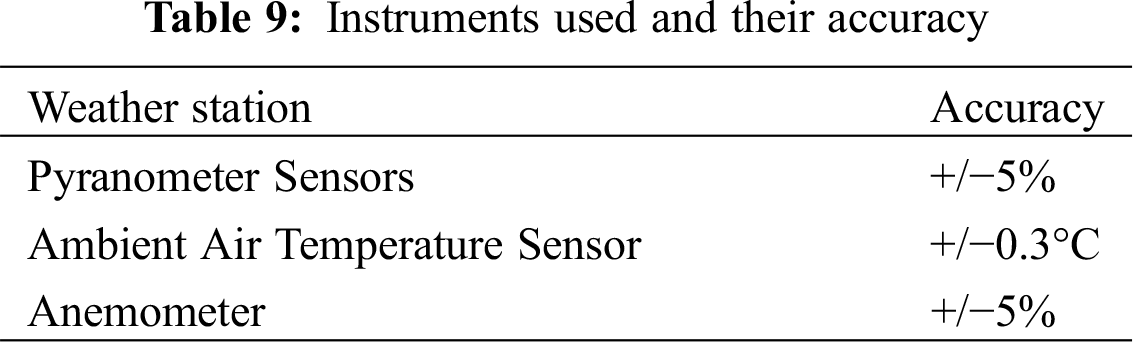
In this study, a weather station was used to measure different values (Tab. 9). Uncertainty in this experimental work comes from temperature, velocity, and irradiance measurements which differ from the true values.
In this experiment the thermal efficiency of SAH (η ) is defined as
where
Cp is the specific heat of air at constant pressure [kJ/kg.K]
A is the surface area of the SAH [m2 ].
I is the irradiance [W/m2 ].
According to the above Eq. (5) and noting that mass flow rate is defined as
where
ρ is the air density [kg/m3]
Ac is constant cross section area of air outlet in [m2]
V is the velocity of the air at the outlet [m/s]
Uncertainty of evaluating η becomes
Hence I is 500 W with
Then the percentage of uncertainty in evaluating thermal efficiency is
This study presents the comprehensive outcomes of the statistical analysis performed to acquire the optimal design of a SAH. The Taguchi method hybridized by the GRA was employed on multi-design variables (V, A, M) to optimize the process responses (∆T, SAH µ). The Grey–Taguchi method plays a considerable role in decreasing the number of required combinations of process factor levels. The analysis of the results showed that the important order of the process factors according to ∆T is determined as follows: T > M > V. While the process factors sequence, which is important for SAH µ is V > T > M, takes into consideration both process responses (∆T and SAH µ) and the effective sequence is T > V > M. The ANOVA results proved the same order for both ∆T and SAH µ (GRG), whereas M process factor is more significant than T factor for SAH µ. Furthermore, ANOVA confirmed that T and M factors are at the same level as ∆T process response. The highest and lowest levels of operating conditions of a SAH for multiple performance characteristics are determined as T2, M2, V2 and T1, M1, V1, respectively. Comparing these results with the experimental ones, we can conclude that they follow the same fashion. The experimental results demonstrated that the wood heater has a higher efficiency than that of the metal one. This observation can be ascribed to the fact that the heat loss from the wood heater to the surroundings is considerably less than the thermal losing from the metal heater to the surroundings. Hence, the loss from the wood heater leads to higher efficiency. The maximum efficiency for both the wood and metal-air heater was found to be 94.6 and 87.6, respectively.
Acknowledgement: The authors would like to express their gratitude to Mr. Muhammad Y. Abu Elrub for his valuable time and precious efforts in reviewing the language of this research.
Funding Statement: The authors received no specific funding for this study.
Conflicts of Interest: The authors declare that they have no conflicts of interest associated with this publication.
1. Bakari, R., Minja, R., Njau, K. (2014). Effect of glass thickness on performance of flat plate solar collectors for fruits drying. Journal of Energy, 2014(247287), 8. DOI 10.1155/2014/247287. [Google Scholar] [CrossRef]
2. Ho, C. D., Yeh, H. M., Cheng, T. W., Chen, T. C., Wang, R. C. (2009). The influences of recycle on performance of baffled double-pass flat-plate solar air heaters with internal fins attached. Applied Energy, 86(9), 1470–1478. DOI 10.1016/j.apenergy.2008.12.013. [Google Scholar] [CrossRef]
3. Chabane, F., Moummi, N., Benramache, S. (2013). Experimental analysis on thermal performance of a solar air collector with longitudinal fins in a region of Biskra. Algeria Journal of Power Technologies, 93(1), 52–58. [Google Scholar]
4. Karmare, S. V., Tikekar, A. N. (2009). Experimental investigation of optimum thermohydraulic performance of solar air heaters with metal rib grits roughness. Solar Energy, 83(1), 6–13. DOI 10.1016/j.solener.2008.05.017. [Google Scholar] [CrossRef]
5. Tyagi, V. V., Panwar, N. L., Rahim, N. A., Kothari, R. (2012). Review on solar air heating system with and without thermal energy storage system. Renewable and Sustainable Energy Reviews, 16(4), 2289–2303. DOI 10.1016/j.rser.2011.12.005. [Google Scholar] [CrossRef]
6. Rajaseenivasan, T., Ravi Prasanth, S., Salamon Antony, M., Srithar, K. (2017). Experimental investigation on the performance of an impinging jet solar air heater. Alexandria Engineering Journal, 56(1), 63–69. DOI 10.1016/j.aej.2016.09.004. [Google Scholar] [CrossRef]
7. Menni, Y., Chamkha, A., Lorenzini, G., Kaid, N., Ameur, H. et al. (2019). Advances of nanofluids in solar collectors—A review of numerical studies. Mathematical Modelling of Engineering Problems, 6(3), 415–427. DOI 10.18280/mmep.060313. [Google Scholar] [CrossRef]
8. Khan, M., Qayyum, S., Hayat, T., Imran, M., Alsaedi, A. et al. (2018). Entropy generation in radiative motion of tangent hyperbolic nanofluid in presence of activation energy and nonlinear mixed convection. Physics Letters A, 382(31), 2017–2026. DOI 10.1016/j.physleta.2018.05.021. [Google Scholar] [CrossRef]
9. Qayyum, S., Hayat, T., Khan, M., Imran, M., Alsaedi, A. (2018). Optimization of entropy generation and dissipative nonlinear radiative Von Karman’s swirling flow with Soret and Dufour effects. Journal of Molecular Liquids, 262(10), 261–274. DOI 10.1016/j.molliq.2018.04.010. [Google Scholar] [CrossRef]
10. Nwachukwu, N. (2010). Employing exergy-optimized pin fins in the design of an absorber in a solar air heater. Energy, 35(2), 571–575. DOI 10.1016/j.energy.2009.10.027. [Google Scholar] [CrossRef]
11. Jawad, Q., Mahdy, A., Khuder, A., Chaichan, M. (2020). Improve the performance of a solar air heater by adding aluminum chip, paraffin wax, and nano-SiC. Case Studies in Thermal Engineering, 19(3), 9. DOI 10.1016/j.csite.2020.100622. [Google Scholar] [CrossRef]
12. Bensaci, C., Moummi, A., Francisco, J., Enrique, A., Rodriguez, J. et al. (2020). Numerical and experimental study of the heat transfer and hydraulic performance of solar air heaters with different baffle positions. Renewable Energy, 155, 1231–1244. DOI 10.1016/j.renene.2020.04.017. [Google Scholar] [CrossRef]
13. Zhu, T., Zhang, J. (2021). A numerical study on performance optimization of a micro-heat pipe arrays-based solar air heater. Energy, 215(6), 119047. DOI 10.1016/j.energy.2020.119047. [Google Scholar] [CrossRef]
14. Bezbaruah, P., Das, R., Sarkar, B. (2020). Overall performance analysis and GRA optimization of solar air heater with truncated half conical vortex generators. Solar Energy, 196(1), 637–652. DOI 10.1016/j.solener.2019.12.057. [Google Scholar] [CrossRef]
15. Kuo, C. F. J., Su, T. L., Jhang, P. R., Huang, C. Y., Chiu, C. H. (2011). Using the Taguchi method and grey relational analysis to optimize the flat-plate collector process with multiple quality characteristics in solar energy collector manufacturing. Energy, 36(5), 3554–3562. DOI 10.1016/j.energy.2011.03.065. [Google Scholar] [CrossRef]
16. Alkhalil, S., Abu Shaban, N. (2018). Design and evaluation of the performance of solar air heater. International Journal of Mechanical Engineering and Technology, 9(4), 328–334. [Google Scholar]
17. Brendell, A., Disney, J., Pridmore, W. A. (1989). Taguchi methods: Applications in world industry UK. Berlin, Heidelberg: Springer. [Google Scholar]
18. Prasanth, A. S., Ramesh, R. (2017). Investigation of surface roughness and tool wear in end milling of Al7075-SiC co-continuous composite. Materials Design and Applications, 65(2), 315–327. DOI 10.1007/978-3-319-50784-2. [Google Scholar] [CrossRef]
19. Sylajakumari, P., Ramakrishnasamy, R., Palaniappan, G. (2018). Taguchi grey relational analysis for multi-response optimization of wear in co-continuous composite. Materials, 11(9), 1743. DOI 10.3390/ma11091743. [Google Scholar] [CrossRef]
 | This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. |