DOI:10.32604/cmc.2022.026682

| Computers, Materials & Continua DOI:10.32604/cmc.2022.026682 |  |
| Article |
Fuzzy Multi-criteria Decision Making for Decision Support in Port Capacity Upgrade
Department of Industrial Engineering and Management, National Kaohsiung University of Science and Technology, Taiwan
*Corresponding Author: Tien–Lin Chao. Email: tienlinchao@gmail.com
Received: 02 January 2022; Accepted: 26 April 2022
Abstract: In many port capacity upgrade projects, choosing a supplier of equipment is a complicated decision, project managers must consider many criteria to choose a supplier to ensure the project is completed on time, optimal in terms of benefit and cost. Therefore, selecting the equipment supplier in this project is a multi-criteria decision-making process. The multi-criteria decision-making (MCDM) model is applied in many fields to select the optimal solution, but there are very few studies using the MCDM model to support project managers in evaluating and selecting optimal solutions in port capacity upgrade project. In this research, the authors combine Fuzzy Analytic Network Process model and Weighted Aggregated Sum Product Assessment concepts to develop a decision support system in port capacity upgrade project. The scientific and practical contribution of this study is to successfully propose a decision support model in a fuzzy environment. The results of the study will be a useful guideline to assist decision makers in port capacity upgrading projects in Taiwan as well as in other countries around the world.
Keywords: Fuzzy theory; MCDM model; supplier selection; port capacity upgrading
In Seaport is a port located on the coast with facilities for loading and unloading goods or a place to pick up or take passengers by waterway. Seaport is an area including port land and port water area, where infrastructure is built and equipment is installed for ships entering and leaving to operate to load and unload goods, pick up and drop off passengers and carry out other services. A seaport consists of berths in a water area of a certain depth and breadth serving for ships to anchor or dock. Seaports are usually deep-water ports serving large ships with high tonnage. According to the goods served, cargo ports can be divided into many types of specialized ports such as bulk ports, container ports, and fuel ports. Services and procedures at the port include loading and unloading services, wharf and warehouse services for goods storage, transportation services in and out of the port, customs clearance.
In Taiwan, Kaohsiung Port is the largest port in Taiwan, handling an average of 10.26 million units of cargo. The port is located in the South of Taiwan, was established in 1858. Every day, the port receives 77,280 tons of cargo with the operation of 23 wharves, of which 2 bulk carriers receive 19,200 tons of cargo per day and 4,811,520 tons. each year; 26 container terminals with 51,480 TEU per day and 14,743,872 TEU per year; 12 liquid cargo terminals receive 240,000 tons of cargo every day, each year this number is up to 60,144,000 tons. 69 warehouses and transshipment yards provide a capacity of 936,089 tons of goods, 12 outdoor yards with a capacity of 41,012 tons of goods [1].
Taiwan’s government has unveiled plans to invest $1.37 billion over the next five years to upgrade the country’s seven commercial ports to support sustainable growth. With the desire to provide customers with services with increasingly improved quality, Kaohsiung Port (Taiwan) has developed a medium and long-term investment plan in infrastructure, facilities, equipment, markets, and human resources to meet the needs of customers [2].
One of the important equipment in the harbor is the container gantry crane. Gantry Crane is also known as gantry crane or Goliath crane. Includes single girder gantry crane, double girder gantry crane, container gantry crane, engineer gantry crane, semi gantry crane, and floor mounted rail operation. Container gantry crane is a kind of large wharf gantry crane at container terminals for intermodal container loading and unloading. Container gantry cranes consist of a truss structure that can traverse the length of a jetty or rail yard. Instead of a conventional hook, the container gantry crane is equipped with a specialized handling tool we commonly call a spreader, which will be lowered on top of the container and used to lock onto the four molding corners of the container [3].
According to a review of the literature, many Multi-Criteria Decision-Making models have been developed and applied to many fields of science and engineering. Among these fields, MCDM techniques have been extensively applied in solving supplier selection problems, where the decision makers must evaluate both qualitative and quantitative criteria. In this research, the authors proposed a MCDM model including Fuzzy Analytic Network Process (FANP) model and Weighted Aggregated Sum Product Assessment (WASPAS) concepts to develop a decision support system in port capacity upgrade project. FANP is a more general form of the decentralized process, which includes the feed-back and interdependencies of decision attributes and alternatives. Thus, FANP is applied for determine the weight of all criteria in the first stage of this research. In WASPAS method, a joint criterion of optimality is sought based on two criteria of optimality. The first criterion of optimality, i.e., criterion of a mean weighted success is similar to WSM method. It is a popular and well accepted MCDM approach applied for evaluating a number of alternatives with respect to a set of decision criteria. Therefore, WASPAS model is used for ranking all potential supplier in final stage. To valid the proposed model, an application case and the ranking performance of WASPAS method with respect to changing λ values is also studied.
Lin [4] proposed the fuzzy analytic net-work process (FANP) model to identify top suppliers, FANP is then integrated with FMOLP in selecting the optimal suppliers under fuzzy environment. Vinodh et al. [5] applied FANP model for supplier selection in a manufacturing organization, the results of this research showed that the application of FANP is practically feasible and adaptable in the contemporary industrial scenario.
Huang et al. [6] used Fuzzy Analytic Network Process-Goal Programming (FANP-GP) and De Novo Programming (DNP) for automotive industry supplier selection. This method helps decision maker can select the best supplier, improves corporate competitiveness, and reduces purchasing costs. Fallahpour et al. [7] proposed a fuzzy decision framework including fuzzy decision-making trial and evaluation laboratory (FDEMATEL), fuzzy best worst method (FBWM), fuzzy analytical network process (FANP) and fuzzy inference system (FIS) to investigate the sustainable supplier selection problem in palm oil industry. Dargi et al. [8] proposed a hybrid Nominated Group Technique (NGT) and A Fuzzy Analytical Network Process (FANP) concepts to support the supplier selection process in automotive industry. Samut [9] proposed an integrated FANP-f-MIGP model for selection of an optimal wind turbine supplier.
Kang et al. [10] proposed a FANP model to evaluate and select optimal sup-pliers, a case study of IC packaging company selection is presented to validate the pro-posed model. Pang et al. [11] proposed a hybrid model based on the ANP model and fuzzy synthetic evaluation under a fuzzy condition. Fuzzy synthetic evaluation is used to select a supplier option in the first stage and is then FANP method is used to calculate the weight of all criteria. Then an integrated FANP and fuzzy synthetic evaluation model is used for selecting the best suppliers in final stage.
Galankashi et al. [12] proposed a Nominal Group Technique (NGT) and Fuzzy Analytical Network Process (FANP) concepts for green supplier selection. In the first stage of this research, NGT is applied to extract the most critical performance measures, a FANP is then used to weight the extracted measures and determine their importance level. Tirkolaee [13] proposed hybrid method using fuzzy MCDM and multi-objective programming for sustainable supplier selection. In this re-search, the reliability of the supply chain was tested, and sensitivity analyses were also performed on the main parameters of the model.
Wang et al. [14] proposed hybrid multicriteria decision-making (MCDM) model using a fuzzy analytical hierarchy process (FAHP) and green data envelopment analysis (GDEA) to identifies the best supplier for edible oil production in food processing industry. Wang et al. [15] presented a MCDM model including Fuzzy ANP and DEA concept for selecting the best supplier in the rice supply chain.
The multi-criteria decision-making (MCDM) model is applied in many fields to select the optimal solution, but there are very few studies using the MCDM model to support project managers in evaluating and selecting optimal solutions in port capacity up-grade project, especially under uncertain decision-making environment. This paper aims to develop a robust and effective decision support tool to support supplier selection processes in port capacity upgrade project.
The Fuzzy MCDM procedure is shown below by steps with a diagram showing in Fig. 1 in order to create an optimal supplier selection model.
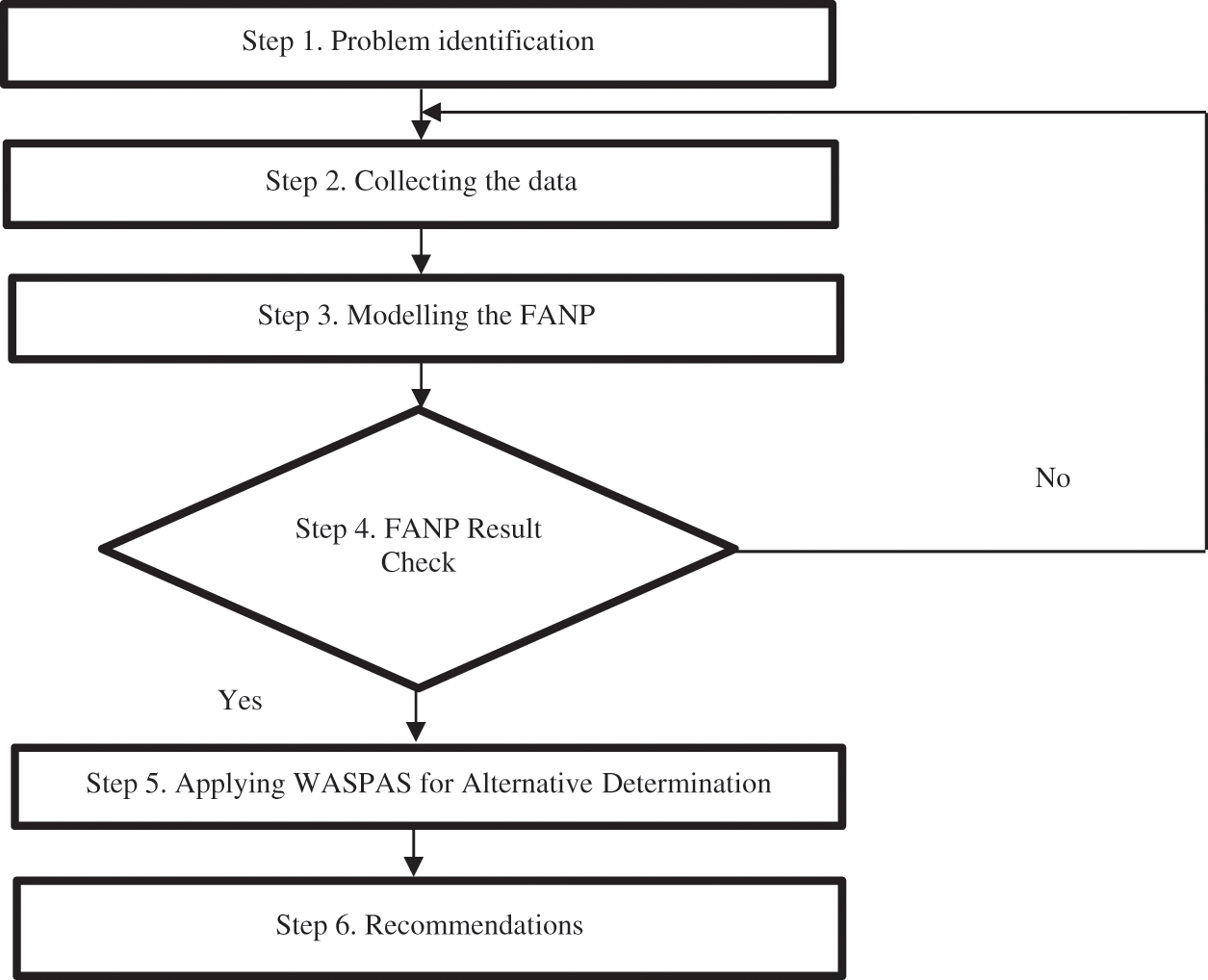
Figure 1: The research graph
Step 1: Problem identification
The first step is to learn about the company’s purchasing processes and determine the criteria for selecting suppliers to analyses and evaluate the current situation by observing the actual operating environment of supplier selection.
Step 2: Collecting the data
Collect the opinions regarding the criteria by acquiring supplier and specialists’ opinions and perspectives.
Step 3: Modelling the FANP
Apply a Fuzzy Network Analysis method (FANP). The FANP model is one of the most effective tools for defining the weight of the criteria. The weight of criteria will be calculated based on reliability, responsiveness, flexibility, cost, and assets factors. The weight of all criteria will be used in WASPAS model.
Step 4. FANP Result Check
Check the correctness of the model
Step 5: Applying WASPAS for Alternative Determination
An initial assessment will be conducted by combining the results of the FANP Model and integrate with the WASPAS Model to determine the best alternative for the study.
Step 6: Recommendations
Analyze and conclude the study results, as well as propose to relate personnel about the issues discovered and unresolved during the research process. Furthermore, models should be developed in conjunction with other approaches utilized in other decision-making domains.
3.2 An Intergrated Model for Supplier Selection
3.2.1 Fuzzy Analytic Network Process Model
The procedure for implementing the FANP method is as follows [16]:
Step 1: Building FANP model
The FANP model framework is built. Establish the analytic network method and specify the link between the criteria and the suppliers, as illustrated in Fig. 2:
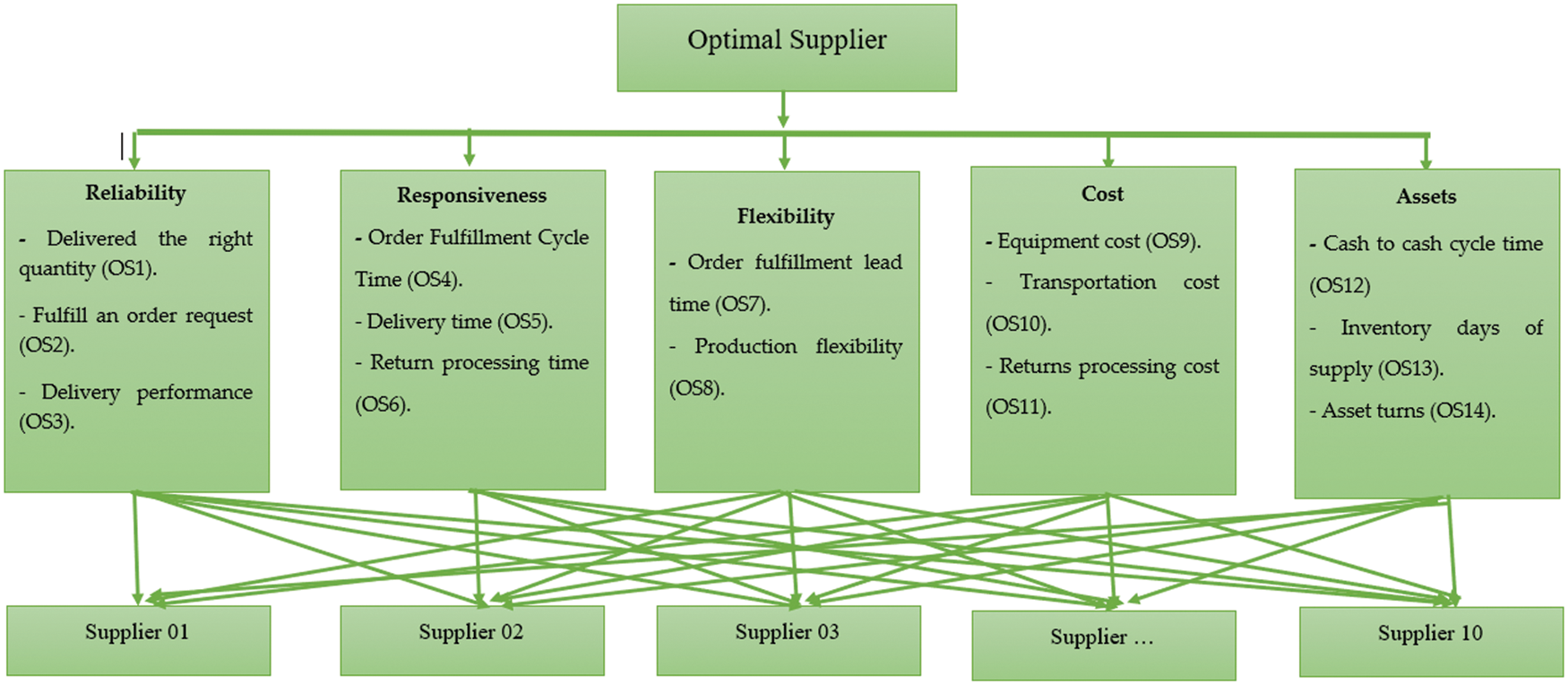
Figure 2: Supplier selection criteria
Step 2: Set up pair comparison matrix
Perform a pairwise comparison of the fuzzy numbers used with each pair between the criteria. This is represented as a matrix that is shown in (1):
where:
Triangular fuzzy trigonometric techniques for converting fuzzy integers to real numbers are described in [16]:
where:
When the diagonal matrix is directly compared, we would have:
We get a matrix that compares real numbers after comparing the fuzzy pairs matrix. This comparison is conducted between two indicators, which are then integrated into a matrix of lines and columns (a: is the number of indicators). The element demonstrates the significance of the indicator i in comparison to the column criteria.
Step 3: Calculate maximum individual value.
ANP can utilize a variety of approaches to compute the highest particular value for the indicator. The most popular is the Lambda Max (max) [17,18].
where:
N: The observations’ matrix of comparison
I: unit matrix of the same size with matrix A.
Step 4: Check consistency. Calculates the vector of the matrix
The ratio that determines the consistency of the randomness of the data is shown as:
where:
CI: Consistency Index
RI: Random Index
If CR
where:
n is the total count of indicators
3.2.2 Weighted Aggregated Sum Product Assessment
The WSM technique is incredibly basic, straightforward to apply, and simple to comprehend. It computes an alternative’s overall score as the weighted sum of the opinionated values. This is the most well-known and commonly utilized approach [20]. WPM was created in order to prevent alternatives with low attribute value. It computes the score of each choice as a product of the scale rating of each attribute to strength multiplied by the importance weight of the attribute [21].
The WASPAS method’s computational approach may be explicitly described as follows:
Step 1: Normalization of the decision matrix
The technique of normalize the decision matrix for the WASPAS approach is de-pendent on whether the choice criteria are useful or not. Normalization of the decision matrix in Tab. 1 for the beneficial choice criterion is accomplished as stated in Eq. (10) as follows:
For detrimental decision criteria as shown in Eq. (11):
Step 2: Determine the observed importance for the ith option, based on the WSM method is as follows:
Step 3: The performance index of the ith option is determined by WPM as given in Eq. (13):
Step 4: Using Eqs. (12) and (13), we build a WASPAS model to determine total relative significance.
The relative importance sum, or, more accurately, the general criterion of the weighted synthesis of the addition and multiplication procedures, is as follows:
where
Container gantry crane is a large crane located at the wharf, usually installed at specialized container ports to load, and unload containers by lifting over the ship’s railing: Lift-on/Lift-off (Lo/Lo). This crane has a sturdy frame structure, placed perpendicular to the pier, reaching across the hull during cargo handling.
One of the important equipment in the harbor is the container gantry crane. In this research, the authors combine Fuzzy Analytic Network Process (FANP) model and Weighted Aggregated Sum Product Assessment (WASPAS) concepts to select optimal container gantry crane supplier. In the first step, there are ten container gantry crane suppliers are considered. There are 14 criteria are shown in Fig. 2 and Tab. 2.
In the first stage of this research, the author applies Fuzzy Analytic Network Process (FANP) model for determining the weight of all criteria.
Then, WASPAS Model is used to determine the best alternative in final stage. The results of WASPAS model are shown in Tab. 3.
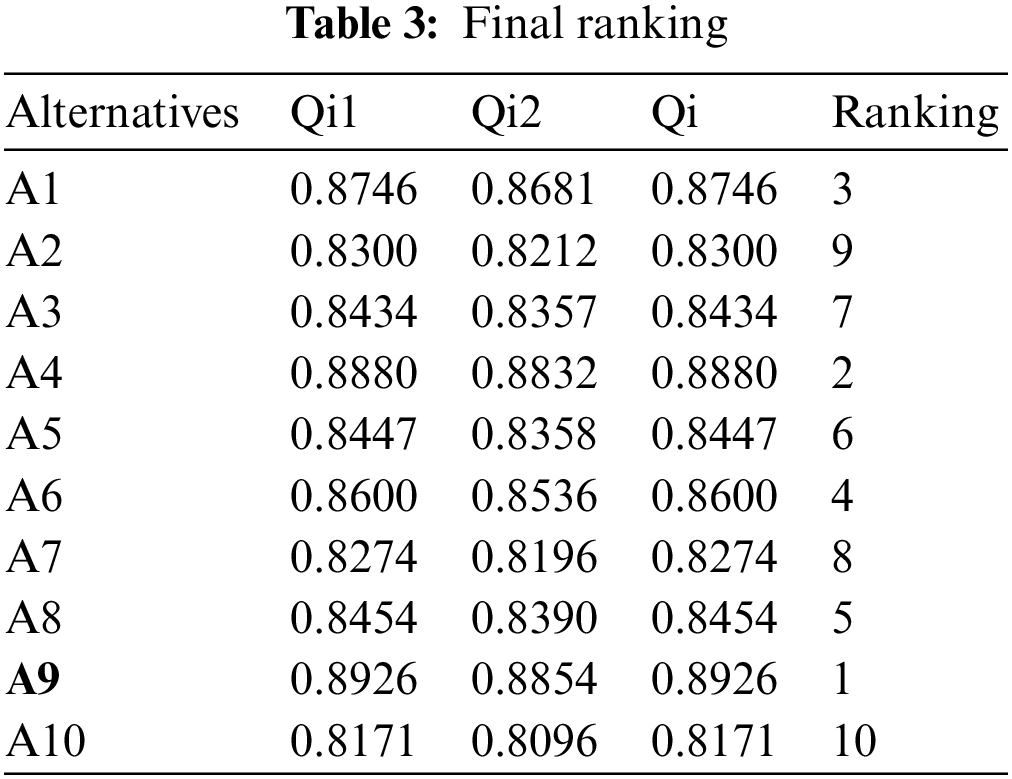
In this research, the authors proposed a hybrid model including FANP - WASPAS concepts to develop a decision support system in port capacity upgrade project. A Fuzzy ANP is applied to determine the weight of all criteria in the first stage, WASPAS model is used for ranking all potential supplier in final stage. From this Tab. 3 and Fig. 3, the ranking list of the robot alternatives is achieved as A9–A4–A1–A6–A8–A5–A3–A7–A2-A10 for a λ value of 0.5, thus supplier 9 (A9) is optimal supplier.
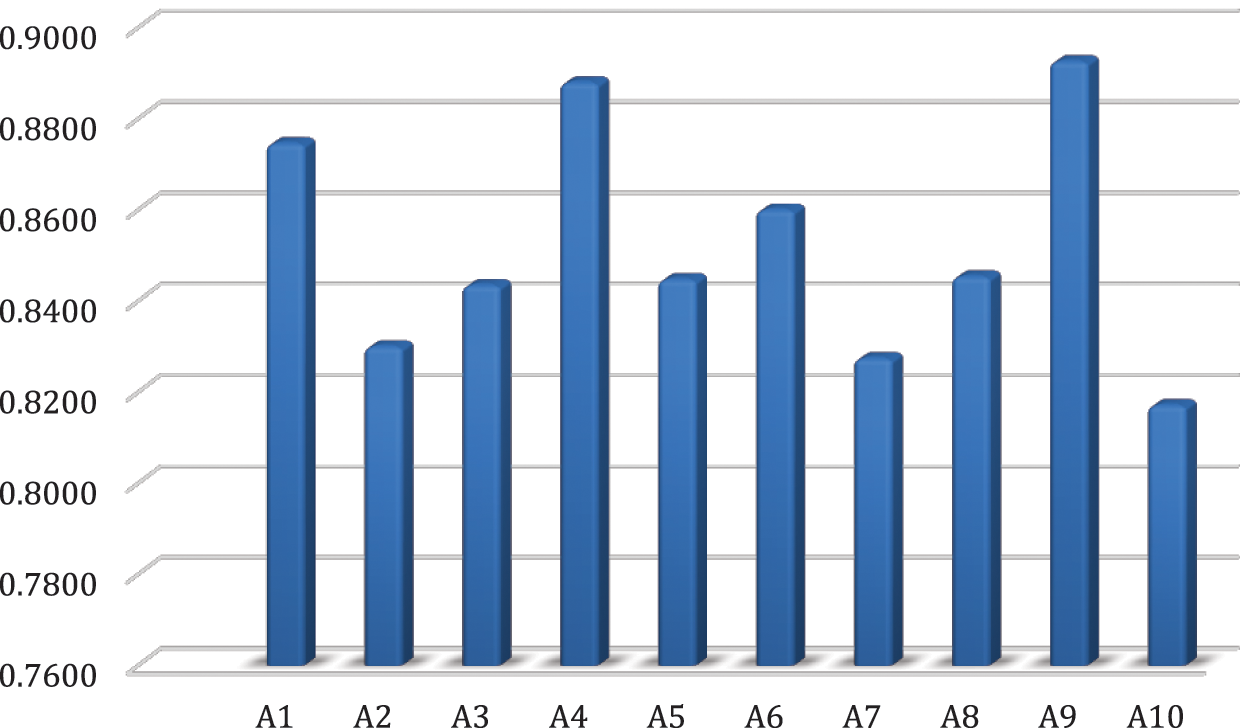
Figure 3: Final ranking
The multi-criteria decision-making (MCDM) model is applied in many fields to select the optimal solution, but there are very few studies using the MCDM model to support project managers in evaluating and selecting optimal solutions in port capacity up-grade project. Initially, a FANP model is employed to calculate the fuzzy weights of the criteria of the supplier selection processes. Then, a WASPAS process is applied to rank all Container gantry crane suppliers. The ranking performance of WASPAS method with respect to changing λ values is also studied. The robustness of FANP - WASPAS method is proved which will help in its widespread application as an efficient MCDM tool. Thus, the hybrid model FANP-WASPAS can be apply on other MCDM problem since it shows good result in the research.
The scientific and practical contribution of this study is to successfully propose a decision support model in a fuzzy environment. The results of the study will be a useful guideline to assist decision makers in port capacity upgrading projects in Taiwan as well as in other countries around the world.
For further studies regarding this topic, the study can be expanded to other MCDM approaches such as TOPSIS, DEA, CoCoSo, ELECTRE III, …or cost of materials when applied in the scheduling process which could be a further study in the future. This model can be applicable from management perspective. The result of extended model can provide for manager the optimal completion time and have the new schedule for the orders next period.
Funding Statement: The authors received no specific funding for this study.
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
1. C. V. Chuyen, “Danh sách cảng biển tại Đài loan,” Cuocvanchuyen.vn. [Online]. Available: https://cuocvanchuyen.vn/tin-tuc/danh-sach-cang-bien-tai-taiwan-dai-loan--185.html. [Google Scholar]
2. F. Bahtić, “Taiwan to invest $1.37 billion to upgrade seven commercial ports,” Offshore-energy.biz, 2021. [Online]. Available: https://www.offshore-energy.biz/taiwan-invests-1-37-billion-to-upgrade-7-commercial-ports/. [Google Scholar]
3. H. Machine, “Cần Cẩu giàn container,” https://hndfcrane.com, 2021. [Online]. Available: https://www.hndfcrane.com/vi/container-gantry-cranes/. [Google Scholar]
4. R. Lin, “An integrated model for supplier selection under a fuzzy situation,” International Journal of Production Economics, vol. 138, no. 1, pp. 55–61, 2012. [Google Scholar]
5. S. Vinodh, R. Ramiya and S. Gautham, “Application of fuzzy analytic network process for supplier selection in a manufacturing organization,” Expert Systems with Applications, vol. 38, no. 1, pp. 272–280, 2011. [Google Scholar]
6. J. Huang and M. Hu, “Two-stage solution approach for supplier selection: A case study in a Taiwan automotive industry,” International Journal of Computer Integrated Manufacturing, vol. 26, no. 3, pp. 237–251, 2013. [Google Scholar]
7. A. Fallahpour, S. Nayeri, M. Sheikhalishahi, K. Wong, G. Tian et al., “A Hyper-hybrid fuzzy decision-making framework for the sustainable-resilient supplier selection problem: A case study of Malaysian palm oil industry,” Environmental Science and Pollution Research, vol. 28, pp. 1–21, 2021. [Google Scholar]
8. A. Dargi, A. Anjomshoae, M. Galankashi, A. Memari and M. Tap, “Supplier selection: A fuzzy-ANP approach,” Procedia Computer Science, vol. 31, pp. 691–700, 2014. [Google Scholar]
9. P. Samut, “Integrated FAANP-f-MIGP model for supplier selection in the renewable energy sector,” Journal of Business Economics and Management, vol. 18, no. 3, pp. 427–450, 2017. [Google Scholar]
10. H. Kang, A. Lee and C. Y. Yang. “A fuzzy ANP model for supplier selection as applied to IC packaging,” Journal of Intelligent Manufacturing, vol. 23, no. 5, pp. 1477–1488, 2012. [Google Scholar]
11. B. Pang and S. Bai. “An integrated fuzzy synthetic evaluation approach for supplier selection based on analytic network process,” Journal of Intelligent Manufacturing, vol. 24, no. 1, pp. 163–174, 2013. [Google Scholar]
12. M. Galankashi, “Prioritizing green supplier selection criteria using fuzzy analytical network process,” Procedia CIRP, vol. 26, pp. 689–694, 2015. [Google Scholar]
13. E. Tirkolaee, A. Mardani, Z. Dashtian, M. Soltani and G. Weber, “A novel hybrid method using fuzzy decision making and multi-objective programming for sustainable-reliable supplier selection in two-echelon supply chain design,” Journal of Cleaner Production, vol. 250, pp. 119517, 2020. [Google Scholar]
14. C. Wang, V. Nguyen, H. Thai, N. Tran and T. Tran, “Sustainable supplier selection process in edible oil production by a hybrid fuzzy analytical hierarchy process and green data envelopment analysis for the SMES food processing industry,” Mathematics, vol. 6, no. 12, pp. 302, 2018. [Google Scholar]
15. C. Wang, V. Nguyen, D. Duong and H. Do, “A hybrid fuzzy analytic network process (FANP) and data envelopment analysis (DEA) approach for supplier evaluation and selection in the rice supply chain,” Symmetry, vol. 10, no. 6, pp. 221, 2018. [Google Scholar]
16. A. Guneri, M. Cengiz and S. Seker, “A fuzzy ANP approach to shipyard location selection,” Expert Systems with Applications, vol. 36, no. 4, pp. 7992–7999, 2009. [Google Scholar]
17. D. Chang, “Applications of the extent analysis method on fuzzy AHP,” European Journal of Operational Research, vol. 95, no. 3, pp. 649–655, 1996. [Google Scholar]
18. L. Hwang and K. Yoon, “Multiple attribute decision making: Methods and applications,” in A State-of-the-Art Survey, 1, Ed., New York: Springer-Verlag Berlin Heidelberg, 1981. [Google Scholar]
19. S. Kusumadewi and I. Guswaludin, “Fuzzy multi-criteria decision making,” Media Informatika, vol. 3, no. 1, pp. 25–38, 2005. [Google Scholar]
20. R. Singh and S. Modgil, “Supplier selection using SWARA and WASPAS – a case study of Indian cement industry,” Measuring Business Excellence, vol. 24, no. 2, pp. 243–265, 2020. [Google Scholar]
21. S. H. Nasab and A. S. Anvari, “An extension of best-worst method with D numbers: Application in evaluation of renewable energy resources,” Sustainable Energy Technologies and Assessments, vol. 40, pp. 100771, 2020. [Google Scholar]
 | This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. |