DOI:10.32604/cmc.2021.015223

| Computers, Materials & Continua DOI:10.32604/cmc.2021.015223 |  |
| Article |
The Hyperbolic Two Temperature Semiconducting Thermoelastic Waves by Laser Pulses
1Department of Mathematics, College of Science Al-Zulfi, Majmaah University, 11952, Saudi Arabia
2Department of Mathematics, Faculty of Science, Zagazig University, Zagazig, Egypt
3Arab Academy for Science, Technology and Maritime Transport, Alexandria, Egypt
4Department of Mathematics, Faculty of Science, Taibah University, Madinah, Saudi Arabia
5National Committee for Mathematics, Academy of Scientific Research and Technology, Cairo, Egypt
*Corresponding Author: Kh. Lotfy. Email: khlotfy_1@yahoo.com
Received: 11 November 2020; Accepted: 05 January 2021
Abstract: A novel model of a hyperbolic two-temperature theory is investigated to study the propagation the thermoelastic waves on semiconductor materials. The governing equations are studied during the photo-excitation processes in the context of the photothermal theory. The outer surface of o semiconductor medium is illuminated by a laser pulse. The generalized photo-thermoelasticity theory in two dimensions (2D) deformation is used in many models (Lord–Shulman (LS), Green–Lindsay (GL) and the classical dynamical coupled theory (CD)). The combinations processes between the hyperbolic two-temperature theory and photo-thermoelasticity theory under the effect of laser pulses are obtained analytically. The harmonic wave technique is used to obtain the exact solutions of the main physical fields under investigation. The mechanical, thermal and recombination plasma loads are applied at the free surface of the medium to obtain the complete solutions of the basic physical fields. Some comparisons are made between the three thermoelastcity theories under the electrical effect of thermoelectric coupling parameter. The influence of hyperbolic two-temperature, two-temperature and one temperature parameters on the distributions of wave propagation of physical fields for semiconductor silicon (Si) medium is shown graphically and discussed.
Keywords: Photo-thermo-elasticity theory; semiconductors; laser pulses; hyperbolic two temperature; harmonic waves; thermo-elastic waves
In the first half of the last century, many authors used the generalized thermoelasticity theory to describe the elastic and thermal waves in elastic material such as semiconductors (semi-insulating). In this case, the semiconductor materials have been studied as an elastic media only. But at the end of the last century, various scientists studied the semiconductor materials especially the inner structures of them during the microelectronics processes. In this study, the photothermal (PT) technique is used to describe the wave propagation of the semiconductor material in the context of photo-acoustic (PA) modern technology. In modern studies the heat conduction effect for semiconductor solid materials is very important when the change of mass and heat transport (thermal diffusivity) occur. The diffusion of heat and mass clearly happens in semiconductor materials when the PT and PA in modern technology are taken into consideration in the context of sensitive to photo-excited transport processes [1,2]. The thermal excitation of the laser pulses causes the electrons to move rapidly and plasma waves (carrier density) are generated in semiconductor materials. This process is very important in microelectronic devices industry during the waves of plasma and elastic-thermal created. A non-Gaussian laser beam is used in this problem to heat the semiconductor plane surface.
Biot [3] developed the coupled thermoelasticity theory (CD theory) when motived the law of Fourier heat conduction that became appropriate for modern engineering applications spicily in high temperature case. But in low temperature case, the thermoelastic models are physically unacceptable and cannot obtain equilibrium state. Lord et al. [4] (LS) inserted one relaxation time and Green et al. [5] (GL) inserted two relaxation times in the heat conduction equation (Fourier’s law of heat conduction) to overcome this contradiction. Many scientists developed many works with some applications in the generalized thermoelasticity theory when used these theories (CD, LS and GL) [6–10].
When an intracavity sample of semiconductor is exposed to laser pulses or beams of laser light sources for photoacoustic spectroscopy analysis in this case, the photothermal theory is introduced [11–14]. Experimentally the electrical resistance of semiconductor materials depends on the temperature increasing. Due to the thermal wave an elastic vibration and thermoelatic deformation (TE) is occurred in the medium, in this case the thermoelastic mechanism during photothermal processes will generate [15]. In the context of photo-excited transport processes the free carriers (carrier density) appear this process is the electronic deformation (ED) [16]. During the two processes TE and Ed, the coupled between plasma and thermo-elastic waves are obtained in a semiconductor medium [17,18]. In modern technology (renewable energy), a semiconductor material industry is widely used specially in solar cells. When the inner structure of semiconductor material is taken into consideration a two different temperatures (the conductive temperature (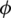 ) and the thermo-dynamical temperature (T)) should study. The system two temperature theory is investigated during structural stability and convergence by Quintanilla et al. [19]. Youssef et al. [20,21] developed a novel technique when used two-temperature theory in the context of the linear generalized thermoelasticity. Lotfy et al. [22–30] used the photo-thermoelasticty theory during TE and ED deformation when used pulse heat flux and gravity field in the context of two-temperature theory with many external fields and various thermal memories. Mondal et al. [31,32] used the memory dependent and fractional derivative during piezo-thermoelastic medium to study Photo-thermo-elastic wave propagation in semiconductor medium.
) and the thermo-dynamical temperature (T)) should study. The system two temperature theory is investigated during structural stability and convergence by Quintanilla et al. [19]. Youssef et al. [20,21] developed a novel technique when used two-temperature theory in the context of the linear generalized thermoelasticity. Lotfy et al. [22–30] used the photo-thermoelasticty theory during TE and ED deformation when used pulse heat flux and gravity field in the context of two-temperature theory with many external fields and various thermal memories. Mondal et al. [31,32] used the memory dependent and fractional derivative during piezo-thermoelastic medium to study Photo-thermo-elastic wave propagation in semiconductor medium.
Recently, Youssef et al. [33] investigated a new model in generalized thermoelsticity theory when they introduced the theory of hyperbolic two-temperature. Ezzat [34] developed the hyperbolic two-temperature theory to study thermal-plasma-elastic wave propagation in organic semiconductor material. Hobiny [35] studied the influence of the hyperbolic two-temperature theory for Photo-thermal waves in a semiconducting medium without energy dissipation. Abbas et al. [36,37] used the hyperbolic two-temperature during the interaction between photo-thermal waves in a cylindrical cavity of semiconductor medium. Many authors developed many models in thermoelasticity theory [38,39].
The basic goal of the investigation is solving a new mathematical model in the context of the 2D deformation processes for hyperbolic two-temperature theory. The problem is studied in photo-thermoelasticity (coupled between plasma and thermal waves) theory of a thin film semiconductor material which exposed to laser pulses. The photo-excitation transport processes occur due to the thermal effect of laser pulses. The problem is studied when using three models of thermoelasticity theory. The harmonic waves (normal mode analysis) expressions are used to obtain the analytical solutions and exact expressions of the main physical quantities. The effects of the hyperbolic two-temperature parameters, thermoelectric (electrical) parameters and the relaxation times (thermal memories) on some physical quantities are obtained. The obtained results have been depicted by simulation (using Si material) graphically and theoretical discussed.
In the present work, a theoretical dissuasion during the heat transport process when the inner structure of semiconductor is taken into consideration. The interaction between plasma-thermal and elastic waves altogether are generated in the context of the own temperature (the hyperbolic two-temperature). The system of equations in this work depend on four main variable quantities, the carrier density 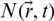 which describe the plasma wave, the thermal wave or thermodynamic temperature distribution
which describe the plasma wave, the thermal wave or thermodynamic temperature distribution 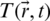 , the conductive temperature
, the conductive temperature 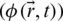 and the elastic waves (displacement distribution)
and the elastic waves (displacement distribution) 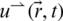 . In this problem, the vector
. In this problem, the vector 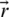 is the position vector and t is the time during the heat flux located, the two-temperature influence appear for a linear, homogeneous and isotropic semiconductor medium. In 2D deformation the variables x and in the xz-plane are taken into account. When the semiconductor surface is excited that it exposed to a laser pulses [40] with the heat input function Q as follows (see the schematic Fig. 1):
is the position vector and t is the time during the heat flux located, the two-temperature influence appear for a linear, homogeneous and isotropic semiconductor medium. In 2D deformation the variables x and in the xz-plane are taken into account. When the semiconductor surface is excited that it exposed to a laser pulses [40] with the heat input function Q as follows (see the schematic Fig. 1):


Figure 1: Schematic representation of the problem (a) spatial profile and temporal of the pulse (b) geometry of the problem
The function 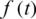 expresses the temporal profile which can be represented as,
expresses the temporal profile which can be represented as,

The pulse Gaussian spatial profile 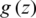 for the laser beam in z-direction is:
for the laser beam in z-direction is:

The laser pulse causes a heat deposition 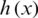 which it decays exponentially and propagates in the semiconductor medium as the follows:
which it decays exponentially and propagates in the semiconductor medium as the follows:

Using Eqs. (2)–(4) and substitution in Eq. (1), yields:

The laser constants I0, t0, a and 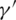 represent the absorbed energy, the pulse rise time, the radius of the laser beam and the absorption heating energy at the medium depth (z) respectively.
represent the absorbed energy, the pulse rise time, the radius of the laser beam and the absorption heating energy at the medium depth (z) respectively.
When the external surface of semiconductor is exposed to thermal effect due to the laser pulses the net charge carrier density is generated. During the excitation transport heat, the coupled plasma, thermal and elastic equations can be obtained in the context of the hyperbolic two temperature theory in the absence of heat source as the form [5,20,41,42]:
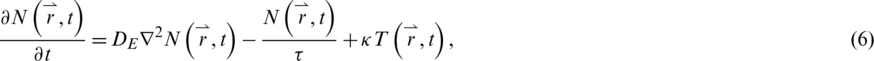
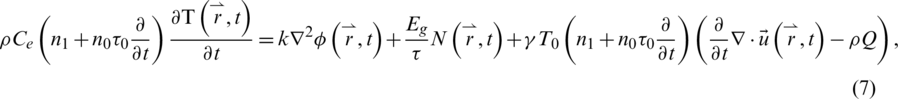
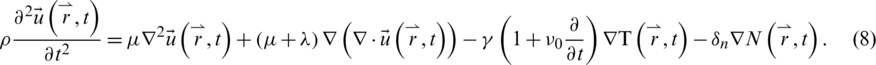
The hyperbolic two temperature theory which it describes the coupled between the heat conduction and the thermo-dynamical temperatures can be written in the following form [40]:

where 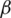 is arbitrary positive constant and it expresses the hyperbolic two-temperature parameter and
is arbitrary positive constant and it expresses the hyperbolic two-temperature parameter and 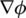 represents the gradient in heat conduction flux.
represents the gradient in heat conduction flux.
In the above equations, 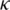 is a non-zero coupling parameter which expresses about the thermal activation
is a non-zero coupling parameter which expresses about the thermal activation 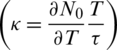 in equilibrium carrier concentration N0 at the temperature T (high temperature) [43–45]. The elastic Lame’s constants are
in equilibrium carrier concentration N0 at the temperature T (high temperature) [43–45]. The elastic Lame’s constants are 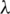 and
and 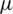 , but
, but 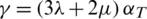 and
and 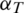 represents the linear thermal expansion coefficient. The other constants, DE,
represents the linear thermal expansion coefficient. The other constants, DE, 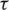 , Eg,
, Eg, 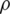 , k, Ce,
, k, Ce, 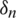 and T0 express about the carrier diffusion coefficient, the lifetime during the photo-generated carrier processes, the energy gap, the density of the material, the heating conductivity, the specific heat coefficient, the deformation potential difference with valence band and the absolute temperature respectively. The thermal memories constants (the thermal relaxation time) are
and T0 express about the carrier diffusion coefficient, the lifetime during the photo-generated carrier processes, the energy gap, the density of the material, the heating conductivity, the specific heat coefficient, the deformation potential difference with valence band and the absolute temperature respectively. The thermal memories constants (the thermal relaxation time) are 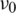 and
and 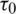 . The parameters n0, n1 are chosen in dimensionless according to the thermoelasticity theories [43,44]. In this investigation all main variables are analyzed the xz -plane, in this case the displacement vector can be taken the components form as
. The parameters n0, n1 are chosen in dimensionless according to the thermoelasticity theories [43,44]. In this investigation all main variables are analyzed the xz -plane, in this case the displacement vector can be taken the components form as 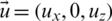 , where the two components of displacement are
, where the two components of displacement are 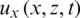 and
and 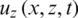 .
.
The constitutive (stress–strain) relation during the coupled processes can be represented in 2D as:
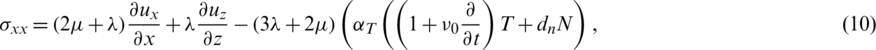
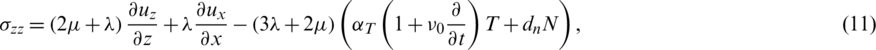

The dimensionless displacement (ux and uz) can be represented in terms of the scalar and vector potentials 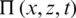 and
and 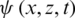 functions as follows:
functions as follows:
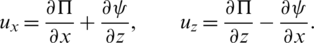
In the other hand, the all quantities can be introduced in non-dimensional quantities for simplicity as follows:
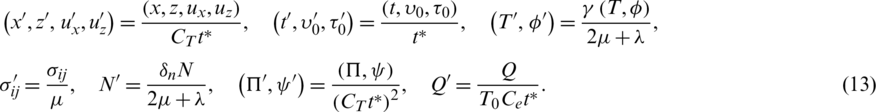
Therefore, the dimensionless Eq. (13) and the scalar and vector potential function (9) can be used in the governing Eqs. (6)–(12), yields (in this case, the primes are dropped for convenient):

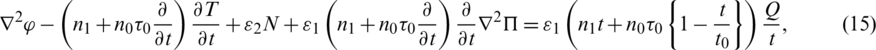



where,
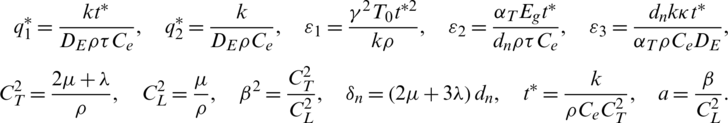
Where,  ,
, 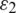 and
and 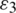 are parameters which describe the thermal-elastic, thermal-energy and thermal-electric coupling parameters respectively, dn is the coefficient of electronic deformation.
are parameters which describe the thermal-elastic, thermal-energy and thermal-electric coupling parameters respectively, dn is the coefficient of electronic deformation.
The constitutive (stress–strain) relations can be obtained in dimensionless form as:
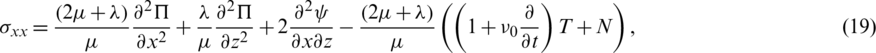
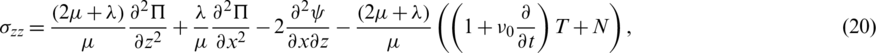

Using the harmonic wave technique (normal mode method) to discuss the wave propagation of the main quantities, which it can be expressed in the xz-plane as follows:
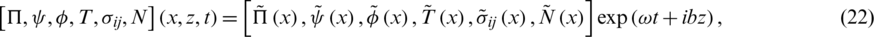
where 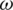 , i, b and
, i, b and 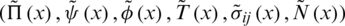 represent the angular frequency (constant of a complex time), the imaginary unit, the number of wave in z-direction and the amplitudes of the main quantities (
represent the angular frequency (constant of a complex time), the imaginary unit, the number of wave in z-direction and the amplitudes of the main quantities (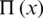 ,
, 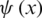 ,
, 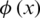 ,
, 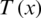 ,
, 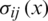 ,
, 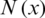 ) respectively.
) respectively.
Applying the normal mode (harmonic wave technique) which defined in Eq. (22) on the main Eqs. (14)–(18), the system of non-homogeneous ordinary deferential equations (ODE):

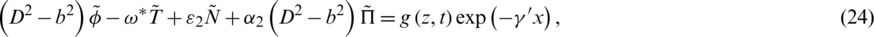



In the other hand, the constitutive (stress–strain) relations (19)–(21) when the normal mode analysis is applied yields:



where,
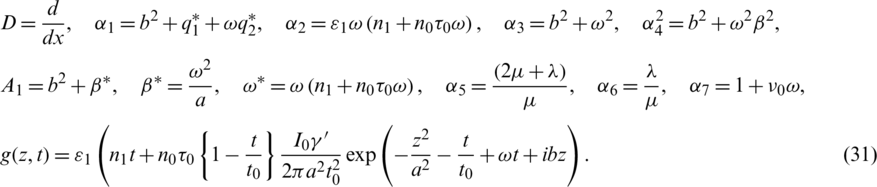
Solving the system of Eqs. (23)–(25) and (27) by eliminate 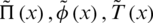 , and
, and 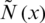 , the non-homogeneous six-order ODE in
, the non-homogeneous six-order ODE in 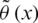 can be obtained as:
can be obtained as:
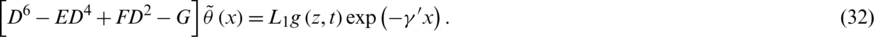
where the coefficients of Eq. (32) are:
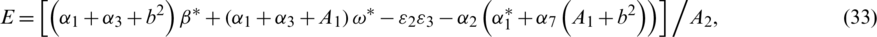

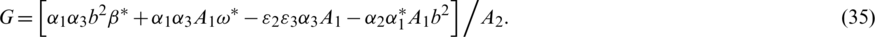
The other constants are:
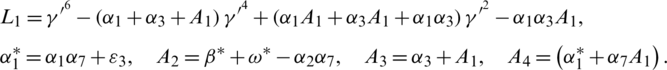
The factorization of six-order homogenous ODE (32) can be expressed as:

But the characteristic equation six-order homogenous ODE (36) which it has the roots 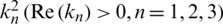 can be taken the following form:
can be taken the following form:

In the other hand, the characteristic equation of the six-order non-homogenous ODE (32) and homogenous ODE (26) can be rewritten the same equation as the follows:
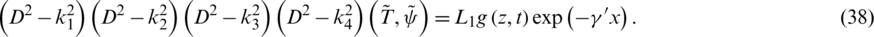
where 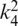 represents the real root of Eq. (26).
represents the real root of Eq. (26).
The solution of the eighth-order non-homogenous ODE Eq. (38) takes the following form:
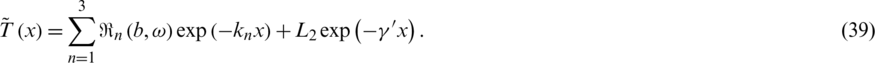
where, 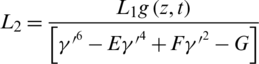 .
.
By the same way,
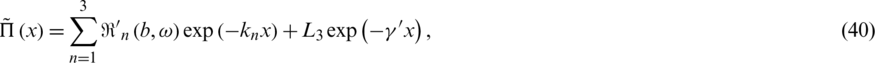
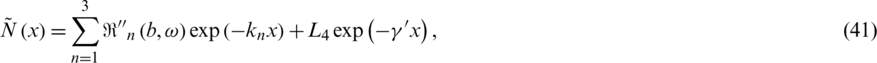
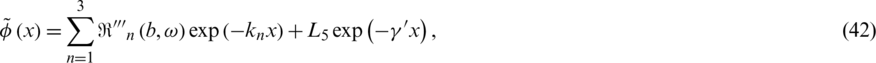

where, 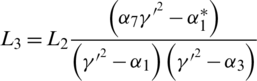 ,
, 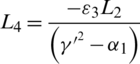 ,
, 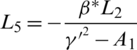 and the parameters
and the parameters 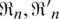 ,
, 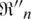 ,
, 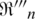 and
and  are unknown which they depend on b and
are unknown which they depend on b and 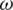 .
.
The displacement components in terms of the amplitude of the scalar and vector potential functions can be rewritten as:

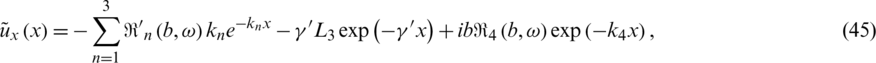

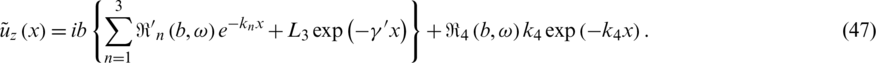
Using Eqs. (39)–(42) into Eqs. (23)–(25) and (27) to obtain the main relations between the unknown parameters 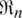 ,
, 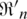 ,
, 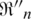 and
and 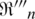 which they can written as follows:
which they can written as follows:
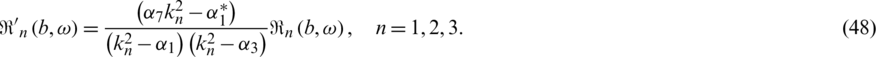


Therefore, the other physical quantities can be represented in terms of the unknown parameters 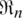 as follows:
as follows:
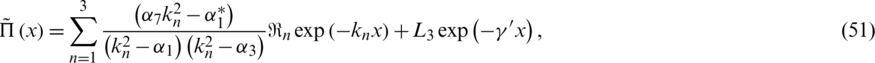
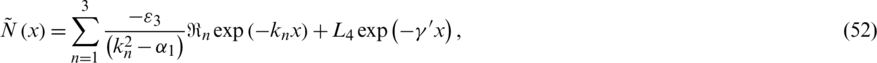
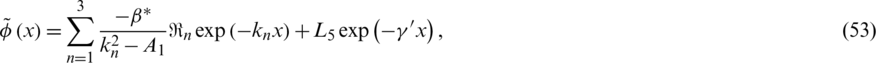
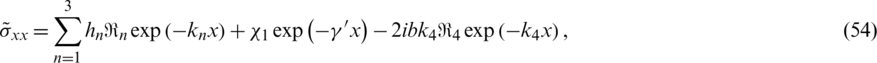
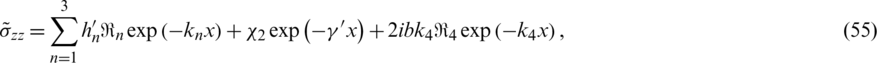
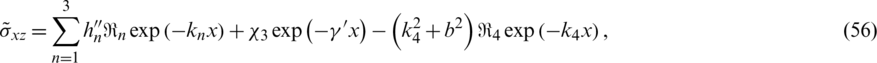
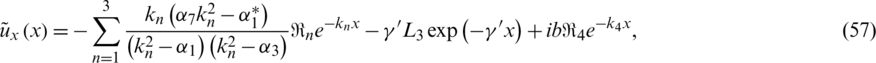
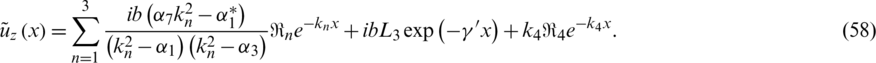
Where, 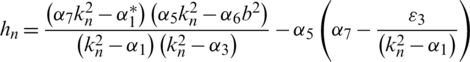 ,
, 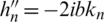 ,
, 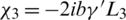 ,
, 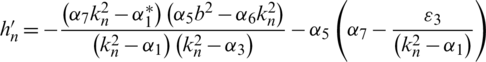 ,
, 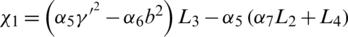 ,
, 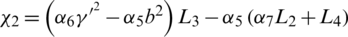 .
.
In this section, some boundary conditions are applied at the free surface of the semi-infinites semiconducting 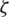 medium to obtain the unknown parameters
medium to obtain the unknown parameters  when the positive exponentials unlimited when x tends to infinity. The problem is studied in the hyperbolic two temperature theory with laser pulses that generate thermal, elastic, plasma effects in the context of the photothermal transport during recombination processes.
when the positive exponentials unlimited when x tends to infinity. The problem is studied in the hyperbolic two temperature theory with laser pulses that generate thermal, elastic, plasma effects in the context of the photothermal transport during recombination processes.
i) Mechanical loads at the surface:
ii) At surface x = 0, the traction can be chosen as loaded with force p1 in the normal direction as:

But, the traction is free for half-space surface as:

Isolated thermal boundary: The thermal boundary at the free surface x = 0 can be chosen as thermally insulated:

Diffusion recombination boundary: During the photothermal transport processes, the carrier’s diffusion occur in the context of the limited recombination processes (possibility), in this case the condition can be chosen as:

With applying the above boundary conditions in harmonic wave technique, the following expressions in terms of the parameters 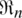 can be obtained as:
can be obtained as:




Using Cramer’s rule for the above Eqs. (63)–(66), the four unknown parameters  can be determinate at x = 0. In this case, the complete solutions of physical quantities can be obtained in time-space domain.
can be determinate at x = 0. In this case, the complete solutions of physical quantities can be obtained in time-space domain.
To obtain the two-temperature thermoelasticity theory in classical model, Eq. (9) can be rewritten in the following form [46]:

where, 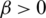 is the two temperature parameter and the problem can be studied in photo-thermoelasticity theory with laser pulses in two temperature field.
is the two temperature parameter and the problem can be studied in photo-thermoelasticity theory with laser pulses in two temperature field.
To obtain the one-temperature theory when the two temperatures are identical (conductive temperature and the thermodynamic temperature) when 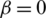 , in this case the heat conduction Eq. (7) can be rewritten in the following form [22]:
, in this case the heat conduction Eq. (7) can be rewritten in the following form [22]:
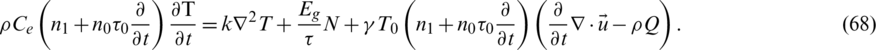
When the heat input function Q is neglected, then the problem is studied in photo-thermoelasticty theory with hyperbolic two temperature theory, in this case the heat Eq. (7) can be written as [47]:
6.4 The Thermoelasticity Theory without Photothermal Excitation
When the carrier density effects 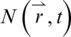 (free electrons) which describe the plasma wave is neglected (i.e., N = 0), in this case the main governing equation can be expressed in the generalized thermoelasticity theory in hyperbolic two temperature theory with laser pulses [37].
(free electrons) which describe the plasma wave is neglected (i.e., N = 0), in this case the main governing equation can be expressed in the generalized thermoelasticity theory in hyperbolic two temperature theory with laser pulses [37].
6.5 The Generalized Photo-Thermoelasticity Theory
In generalized photo-thermoelasticity theory in this problem three theories can be obtained as follows:
i) When n1 = 1, n0 = 0, 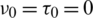 , the CD theory can be discussed [3].
, the CD theory can be discussed [3].
ii) When n1 = n0 = 1, 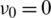 ,
, 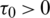 , the LS theory can be discussed [4].
, the LS theory can be discussed [4].
iii) When n1 = 1, n0 = 0, 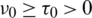 , the GL theory can be discussed [5].
, the GL theory can be discussed [5].
7 Numerical Results and Discussions
To investigate the obtained results theoretically, the physical properties and physical constants in SI unit of silicon (Si) as a semiconductor elastic material is used. Silicon (Si) constants are used to make the numerical simulation and discussed the computational results. The MATLAB (2018) is used to complete the numerical simulation; the constants of Si are shown in Tab. 1 as [46,47]:
In the above computations, 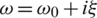 (
(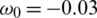 ) for small time t = 0.0005 s and
) for small time t = 0.0005 s and 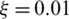 , the exponential function can be expressed as
, the exponential function can be expressed as 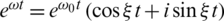 where
where 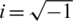 represents the imaginary unit. The obtained results are calculated when the wave number is b = 1.0 and load force is p1 = 1, the distributions of physical quantities are taken for the real part in this problem.
represents the imaginary unit. The obtained results are calculated when the wave number is b = 1.0 and load force is p1 = 1, the distributions of physical quantities are taken for the real part in this problem.
Table 1: The physical constants of Si
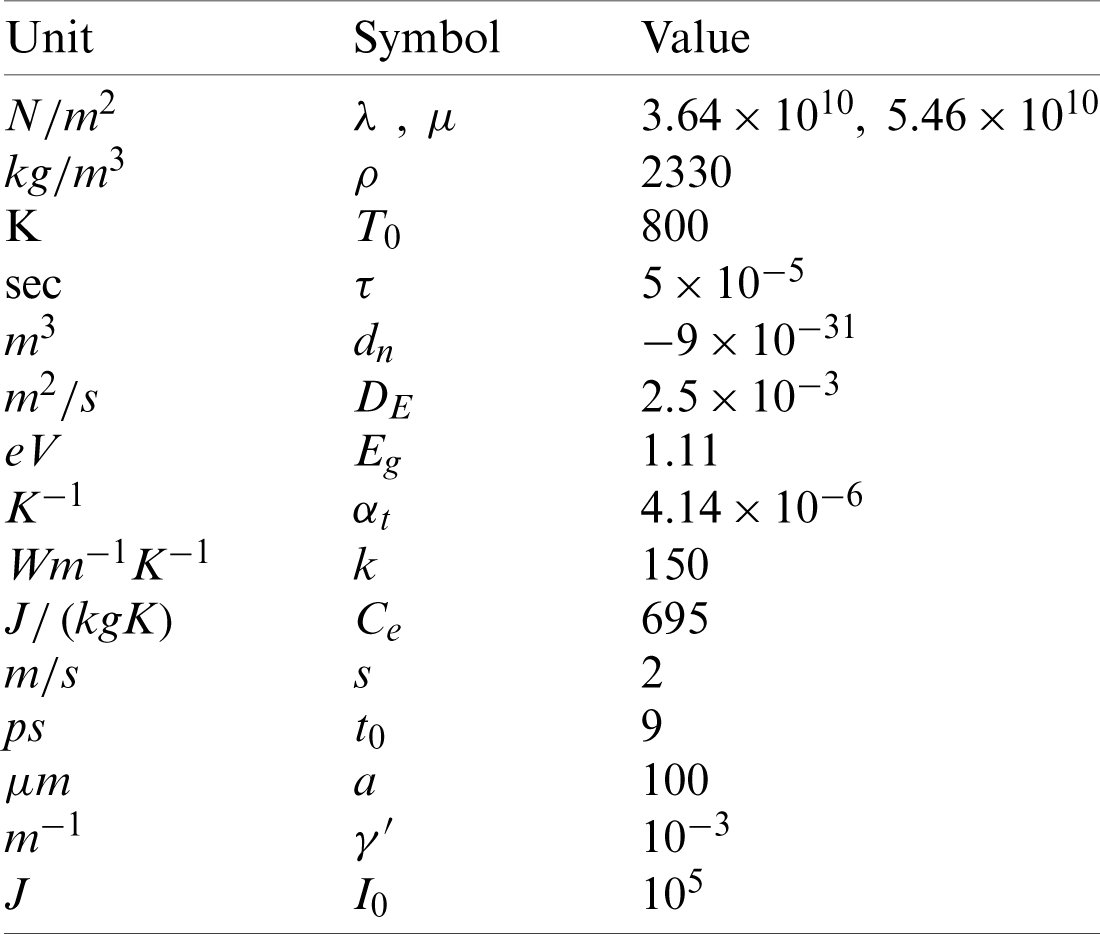
Fig. 2 shows the wave propagation of the main physical fields (thermo-dynamical temperature T, the horizontal displacement ux, heat conduction temperature 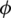 , the normal and tangent stresses (
, the normal and tangent stresses (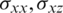 ) and carrier density N) against the horizontal distance x at the plane
) and carrier density N) against the horizontal distance x at the plane 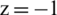 . A three theories of generalized photo-thermoelasticity are applied, the coupled model (CD theory (solid lines)), the Lord-Şhulman model (LS theory (dashed-dotted lines)) with one thermal memory (relaxation time) when
. A three theories of generalized photo-thermoelasticity are applied, the coupled model (CD theory (solid lines)), the Lord-Şhulman model (LS theory (dashed-dotted lines)) with one thermal memory (relaxation time) when 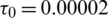 s, and generalized theory Green-Lindsay model (GL theory (dashed lines)) with two thermal memories when
s, and generalized theory Green-Lindsay model (GL theory (dashed lines)) with two thermal memories when 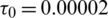 s,
s, 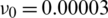 s. In these figures, the real dimensionless forms are applied for main fields in hyperbolic two temperature field under the influence of laser pulse at
s. In these figures, the real dimensionless forms are applied for main fields in hyperbolic two temperature field under the influence of laser pulse at 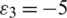 . From the subfigures in Fig. 2, all physical fields satisfy the thermal, mechanical and plasma conditions at the boundary surface. All waves’ distributions start from minimum values and increases in the first range near the boundary surface due to the effect of laser pulses. The distributions of waves are damped which have an exponential wave form with a finite speed with the increasing in the horizontal distance due to the effect of hyperbolic two temperature parameter.
. From the subfigures in Fig. 2, all physical fields satisfy the thermal, mechanical and plasma conditions at the boundary surface. All waves’ distributions start from minimum values and increases in the first range near the boundary surface due to the effect of laser pulses. The distributions of waves are damped which have an exponential wave form with a finite speed with the increasing in the horizontal distance due to the effect of hyperbolic two temperature parameter.

Figure 2: The variation of main physical fields against the horizontal distance under generalized three theories when 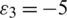 with laser pulses in hyperbolic two temperature field
with laser pulses in hyperbolic two temperature field
The second category (Fig. 3) displays the variation of main physical fields with the horizontal distance under the effect of different three negative values of thermoelectric coupling parameter in hyperbolic two temperature field with laser pulse under GL theory. From the second figure, the wave propagation distributions coincide with the increasing of distance x at infinity due to a finite speed of waves. The thermoelectric coupling parameters have a great significant effect of all physical field distributions. The amplitude of most wave propagations increases when the value of the thermoelectric coupling parameters decreases.

Figure 3: The variation of main physical fields against the horizontal distance under generalized Gl theory with laser pulses in hyperbolic two temperature field and different thermoelectric coupling parameter
Fig. 4 represents the comparison between the main physical fields under investigation three cases, the first case when the thermodynamic temperature T is equal to the conductive temperature 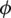 (one-temperature (OT)) in the absence of heat supply which it expresses by the solid lines. The second case represents the classical two temperature parameter (CTT) in absence of heat supply which it represents by dashed-dotted lines. But the third case represents hyperbolic two temperature (HTT) case which it expresses by dashed lines. All obtained results are made when
(one-temperature (OT)) in the absence of heat supply which it expresses by the solid lines. The second case represents the classical two temperature parameter (CTT) in absence of heat supply which it represents by dashed-dotted lines. But the third case represents hyperbolic two temperature (HTT) case which it expresses by dashed lines. All obtained results are made when 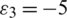 under the influence of laser pulses under in GL model. A clear significant difference is obtained in three different cases.
under the influence of laser pulses under in GL model. A clear significant difference is obtained in three different cases.

Figure 4: The variation of main physical fields against the horizontal distance under generalized Gl theory with laser pulses in one temperature, two temperature and hyperbolic two temperature when 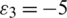
The forth category (Fig. 5) shows that the variations of the basic physical fields against the horizontal distance in two cases, the first when the problem is studied without laser pulse (WOLP) which it represents by solid lines. The second case when the problem is investigated under the effect of laser pulse (WLP), all computationals are carried out in GL photo-thermoelasticity theory in hyperbolic two temperature field when 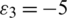 . The influence of laser pulses clearly shows in the amplitude of the wave propagation. The values of the amplitude of physical quantity are greater under the influence of laser pulse (WLP) than without the effect of laser pulse (WOLP) due to the physical properties of semiconductor materials (laser with thermal effect causes photo-excited electrons).
. The influence of laser pulses clearly shows in the amplitude of the wave propagation. The values of the amplitude of physical quantity are greater under the influence of laser pulse (WLP) than without the effect of laser pulse (WOLP) due to the physical properties of semiconductor materials (laser with thermal effect causes photo-excited electrons).
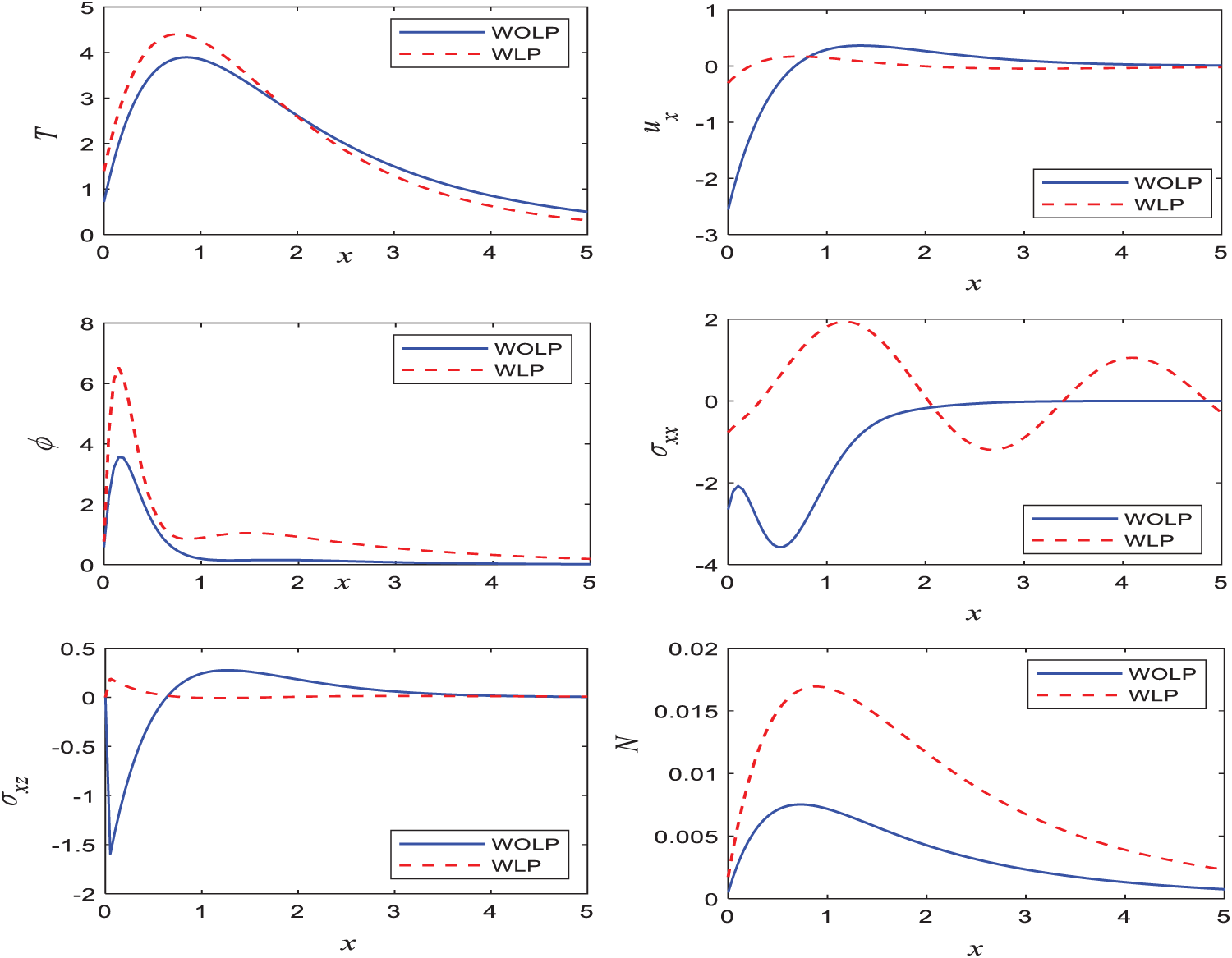
Figure 5: The variation of main physical fields against the horizontal distance under generalized Gl theory with and without laser pulses in hyperbolic two temperature field when 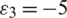
In the present problem, the coupled between the plasma, elastic and thermal waves is investigated in generalized photo-thermoelasticity theory in the context of the hyperbolic two temperature theory with the effect of laser pulses of a 2D deformation semiconductor wafer. The harmonic wave analysis is used to obtain the physical fields analytically, therefore they are illustrated graphically and discussed. The influences of the thermal memories time, the thermoelectric coupling parameters, three theories of thermodynamic-conduction temperatures (OT, CTT and HTT) and laser pulses effect are discussed. From the above investigations, some important results are obtained as follows:
1. The wave propagation of all physical fields decreases exponentially when the horizontal distance x increases.
2. The thermal memories according to the thermoelasticity theories have a great influence of all the physical field distributions.
3. The thermoelectric coupling parameters have a significant effect in all physical fields under the hyperbolic two temperatures theory due to the effect of laser pulse.
4. The hyperbolic two-temperature in generalize photo-thermoelasticity theory is more effective for discus the wave propagation on the distribution of field quantities when study the behavior of the inner structure of the semiconductor elastic solid body more real than the two temperature theory and one-temperature with laser pulses.
5. The laser pulses in the hyperbolic two temperature theory is important phenomena which it has a great influence on the distribution of field quantities.
Acknowledgement: This paper was funded by the deputyship for Research & Innovation Ministry of Education in Saudi Arabia through the project number (IFP-2020-08).
Funding Statement: The authors extend their appreciation to the deputyship for Research & Innovation Ministry of Education in Saudi Arabia for funding this research work through the project number (IFP-2020-08).
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
1. A. Mandelis. (1987). Photoacoustic and Thermal Wave Phenomena in Semiconductors. United States: Elsevier. [Google Scholar]
2. D. Almond and P. Patel. (1996). Photothermal Science and Techniques. Berlin, Germany: Springer Science & Business Media. [Google Scholar]
3. M. A. Biot. (1956). “Thermoelasticity and irreversible thermodynamics,” Journal of Applied Physics, vol. 27, no. 3, pp. 240–253. [Google Scholar]
4. H. Lord and Y. Shulman. (1967). “A generalized dynamical theory of thermoelasticity,” Journal of the Mechanics and Physics of Solids, vol. 15, no. 5, pp. 299–309. [Google Scholar]
5. A. E. Green and K. A. Lindsay. (1972). “Thermoelasticity,” Journal of Elasticity, vol. 2, no. 1, pp. 1–7. [Google Scholar]
6. D. S. Chandrasekharaiah. (1986). “Thermoelasticity with second sound: A review,” Applied Mechanics Reviews, vol. 39, no. 3, pp. 355–376. [Google Scholar]
7. D. S. Chandrasekharaiah. (1998). “Hyperbolic thermoelasticity: A review of recent literature,” Applied Mechanics Reviews, vol. 51, no. 12, pp. 705–729. [Google Scholar]
8. K. Lotfy and S. Abo-Dahab. (2015). “Two-dimensional problem of two temperature generalized thermoelasticity with normal mode analysis under thermal shock problem,” Journal of Computational and Theoretical Nanoscience, vol. 12, no. 8, pp. 1709–1719. [Google Scholar]
9. M. Othman and K. Lotfy. (2015). “The influence of gravity on 2-D problem of two temperature generalized thermoelastic medium with thermal relaxation,” Journal of Computational and Theoretical Nanoscience, vol. 12, no. 9, pp. 2587–2600. [Google Scholar]
10. K. Lotfy and M. Othman. (2011). “The effect of rotation on plane waves in generalized thermo-microstretch elastic solid with one relaxation time for a mode-I crack problem,” Chinese Physics B, vol. 20, no. 7, pp. 74601. [Google Scholar]
11. J. P. Gordon, R. C. C. Leite, R. S. Moore, S. P. S. Porto and J. R. Whinnery. (1965). “Long-transient effects in lasers with inserted liquid samples,” Journal of Applied Physics, vol. 36, no. 1, pp. 3–8. [Google Scholar]
12. L. B. Kreuzer. (1971). “Ultralow gas concentration infrared absorption spectroscopy,” Journal of Applied Physics, vol. 42, no. 42, pp. 2934–2943. [Google Scholar]
13. A. C. Tam. (1983). Ultrasensitive Laser Spectroscopy. New York (NYAcademic Press, pp. 1–108. [Google Scholar]
14. A. C. Tam. (1986). “Applications of photoacoustic sensing techniques,” Reviews of Modern Physics, vol. 58, no. 2, pp. 381–431. [Google Scholar]
15. A. C. Tam. (1989). Photothermal Investigations in Solids and Fluids. Boston (MAAcademic Press, pp. 1–33. [Google Scholar]
16. D. M. Todorović, P. M. Nikolić and A. I. Bojičić. (1999). “Photoacoustic frequency transmission technique: Electronic deformation mechanism in semiconductors,” Journal of Applied Physics, vol. 85, no. 11, pp. 7716–7726. [Google Scholar]
17. Y. Song, D. M. Todorovic, B. Cretin and P. Vairac. (2010). “Study on the generalized thermoelastic vibration of the optically excited semiconducting microcantilevers,” International Journal of Solids and Structures, vol. 47, no. 14–15, pp. 1871–1875. [Google Scholar]
18. J. K. Chen, J. E. Beraun and C. L. Tham. (2004). “Ultrafast thermoelasticity for short-pulse laser heating,” International Journal of Engineering Science, vol. 42, no. 8–9, pp. 793–807. [Google Scholar]
19. T. Q. Quintanilla and C. L. Tien. (1993). “Heat transfer mechanism during short-pulse laser heating of metals,” ASME Journal of Heat Transfer, vol. 115, no. 4, pp. 835–841. [Google Scholar]
20. H. M. Youssef. (2006). “Theory of two-temperature-generalized thermoelasticity,” IMA Journal of Applied Mathematics, vol. 71, no. 3, pp. 383–390. [Google Scholar]
21. H. M. Youssef and E. A. Al-Lehaibi. (2007). “State–space approach of two-temperature generalized thermoelasticity of one-dimensional problem,” International Journal of Solids Structure, vol. 44, pp. 1550–1562. [Google Scholar]
22. Kh. Lotfy and A. A. El-Bary. (2019). “A photothermal excitation for a semiconductor medium due to pulse heat flux and volumetric source of heat with thermal memory,” Waves in Random and Complex Media, . https://doi.org/10.1080/17455030.2019.1662511. [Google Scholar]
23. K. Lotfy, M. Gabr and A. El-Bary. (2020). “Surface wave propagation in two-dimensional models of generalized two temperature thermoelasticity with gravity effect,” Archives of Thermodynamics, vol. 41, no. 1, pp. 219–243. [Google Scholar]
24. K. Lotfy, W. Hassan, A. A. El-Bary and Mona A. Kadry. (2020). “Response of electromagnetic and Thomson effect of semiconductor mediu due to laser pulses and thermal memories during photothermal excitation,” Results in Physics, vol. 16, pp. 102877. [Google Scholar]
25. A. K. Khamis, A. A. El-Bary, K. Lotfy and A. Bakali. (2020). “Photothermal excitation processes with refined multi dual phase-lags theory for semiconductor elastic medium,” Alexandria Engineering Journal, vol. 59, no. 1, pp. 1–9. [Google Scholar]
26. M. Yasein, K. Lotfy, N. Mabrouk, A. A. El-Bary and W. Hassan. (2020). “Response of thermo-electro-magneto semiconductor elastic medium to photothermal excitation process with Thomson influence,” Silicon, vol. 12, pp. 2789–2798. [Google Scholar]
27. K. Lotfy, A. A. El-Bary, W. Hassan, A. R. Alharbi and M. B. Almatrafi. (2020). “Electromagnetic and Thomson effects during photothermal transport process of a rotator semiconductor medium under hydrostatic initial stress,” Results in Physics, vol. 16, pp. 102983. [Google Scholar]
28. M. I. A. Othman and K. Lotfy. (2010). “On the plane waves of generalized thermo-microstretch elastic half-space under three theories,” International Communications in Heat and Mass Transfer, vol. 37, no. 2, pp. 192–200. [Google Scholar]
29. Othman M. I. A., Abo-Dahab S. M. and Lotfy K. (2014). “Gravitational effect and initial stress on generalized magneto-thermo-microstretch elastic solid for different theories,” Applied Mathematics and Computation, vol. 230, pp. 597–608. [Google Scholar]
30. Lotfy Kh. (2014). “Two temperature generalized magneto-thermoelastic interactions In an elastic medium under three theories,” Applied Mathematics and Computation, vol. 227, pp. 871–888. [Google Scholar]
31. S. Mondal and M. Othman. (2020). “Memory dependent derivative effect on generalized piezo-thermoelastic medium under three theories,” Waves Random Complex Medium, vol. 3, pp. 1–18. [Google Scholar]
32. S. Mondal and A. Sur. (2020). “Photo-thermo-elastic wave propagation in an orthotropic semiconductor with a spherical cavity and memory responses,” Waves Random Complex Medium, vol. 10, no. 1, pp. 1–24. [Google Scholar]
33. H. Youssef and A. El-Bary. (2018). “Theory of hyperbolic two-temperature generalized thermoelasticity,” Materials Physics and Mechanics, vol. 40, pp. 158–171. [Google Scholar]
34. M. A. Ezzat. (2020). “Hyperbolic thermal-plasma wave propagation in semiconductor of organic material,” Waves in Random and Complex Media, . https://doi.org/10.1080/17455030.2020.1772524. [Google Scholar]
35. A. Hobiny. (2020). “Effect of the hyperbolic two-temperature model without energy dissipation on Photo-thermal interaction in a semi-conducting medium,” Results in Physics, vol. 18, pp. 103167. [Google Scholar]
36. A. Hobiny and I. Abbas. (2018). “Analytical solutions of photo-thermo-elastic waves in a non-homogenous semiconducting material,” Results in Physics, vol. 10, pp. 385–390. [Google Scholar]
37. I. Abbas, T. Saeed and M. Alhothuali. (2020). “Hyperbolic two-temperature photo-thermal interaction in a semiconductor medium with a cylindrical cavity,” Silicon, . https://doi.org/10.1007/s12633-020-00570-7. [Google Scholar]
38. E. Abd-Elaziz, M. Marin and M. Othman. (2019). “On the effect of Thomson and initial stress in a thermo-porous elastic solid under GN electromagnetic theory,” Symmetry Basel, vol. 11, no. 3, pp. 413. [Google Scholar]
39. C. Itu, A. Öchsner, S. Vlase and M. Marin. (2019). “Improved rigidity of composite circular plates through radial ribs,” Proc. of the Institution of Mechanical Engineers Part L-Journal of Materials-Design and Applications, vol. 233, no. 8, pp. 1585–1593. [Google Scholar]
40. A. Mandelis, M. Nestoros and C. Christofides. (1997). “Thermoelectronic-wave coupling in laser photothermal theory of semiconductors at elevated temperatures,” Optical Engineering, vol. 36, pp. 459–468. [Google Scholar]
41. D. M. Todorović. (2003). “Plasma, thermal, and elastic waves in semiconductors,” Review of Scientific Instruments, vol. 74, no. 1, pp. 582–585. [Google Scholar]
42. A. N. Vasil’ev and V. B. Sandomirskii. (1984). “Photoacoustic effects in finite semiconductors,” Sovient Physics Semiconductors, vol. 18, pp. 1095–1099. [Google Scholar]
43. C. Christofides, A. Othonos and E. Loizidou. (2002). “Influence of temperature and modulation frequency on the thermal activation coupling term in laser photothermal theory,” Journal of Applied Physics, vol. 92, no. 3, pp. 1280–1285. [Google Scholar]
44. Y. Q. Song, J. T. Bai and Z. Y. Ren. (2012). “Study on the reflection of photothermal waves in a semiconducting medium under generalized thermoelastic theory,” Acta Mechanica, vol. 223, no. 7, pp. 1545–1557. [Google Scholar]
45. A. Hobiny and I. A. Abbas. (2016). “A study on photothermal waves in an unboundedsemiconductor medium with cylindrical cavity,” Mech Time-Depend Mater, vol. 6, pp. 1–12. [Google Scholar]
46. K. Lotfy and M. Gabr. (2017). “Response of a semiconducting infinite medium under two temperature theory with photothermal excitation due to laser pulses,” Optics and Laser Technology, vol. 97, pp. 198–208. [Google Scholar]
47. K. Lotfy. (2016). “The elastic wave motions for a photothermal medium of a dual-phase-lag model with an internal heat source and gravitational field,” Canadian Journal of Physics, vol. 94, no. 4, pp. 400–409. [Google Scholar]
 | This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. |