Open Access

SHORT COMMUNICATION

New Definitions of the Isometric Latitude and the Mercator Projection

Miljenko Lapaine^*

Faculty of Geodesy, University of Zagreb, Zagreb, HR-10000, Croatia

* Corresponding Author: Miljenko Lapaine. Email:

Revue Internationale de Géomatique 2024, 33, 155-165. https://doi.org/10.32604/rig.2024.052258

Received 27 March 2024; Accepted 20 May 2024; Issue published 10 June 2024

Abstract

The short communication discusses the interrelationships of loxodromes, isometric latitudes and the normal aspect of Mercator projection. It is shown that by applying the isometric latitude, a very simple equation of the loxodrome on the sphere is reached. The consequence of this is that the isometric latitude can be defined using the generalized longitude, and not only using the latitude, as was common until now. Generalized longitude is the longitude defined for every real number; modulo 2π of generalized and usual longitude are congruent. Since the image of the loxodrome in the plane of the Mercator projection is a straight line, the isometric latitude can also be defined using this projection. Finally, a new definition of the Mercator projection is given, according to which it is a normal aspect cylindrical projection in which the images of the loxodromes on the sphere are straight lines in the plane of the projection that, together with the images of the meridians in the projection, form equal angles, as do the loxodromes with the meridians on the sphere. The short communication provides additional knowledge to all those who are interested in the theory of maps in navigation and have a piece of requisite mathematical knowledge, as well as an interest in map projections. It will be useful for teachers and students studying cartography and GIS, navigation or applied mathematics.

---

Graphic Abstract

---

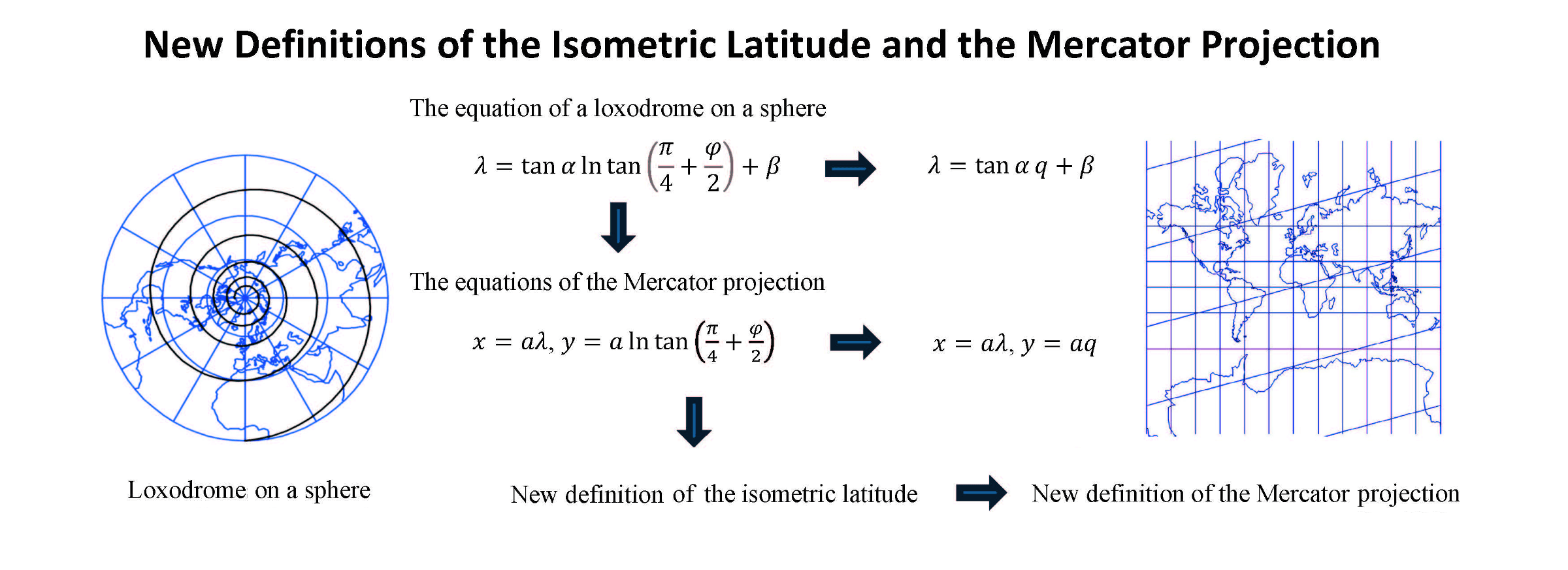
Keywords

---