Open Access

ARTICLE

An Efficient Local Radial Basis Function Method for Image Segmentation Based on the Chan–Vese Model

Shupeng Qiu, Chujin Lin, Wei Zhao^*

School of Mathematics and Statistics, Guangdong University of Technology, Guangzhou, 510520, China

* Corresponding Author: Wei Zhao. Email:

(This article belongs to the Special Issue: New Trends on Meshless Method and Numerical Analysis)

Computer Modeling in Engineering & Sciences 2024, 139(1), 1119-1134. https://doi.org/10.32604/cmes.2023.030915

Received 02 May 2023; Accepted 28 July 2023; Issue published 30 December 2023

Abstract

In this paper, we consider the Chan–Vese (C-V) model for image segmentation and obtain its numerical solution accurately and efficiently. For this purpose, we present a local radial basis function method based on a Gaussian kernel (GA-LRBF) for spatial discretization. Compared to the standard radial basis function method, this approach consumes less CPU time and maintains good stability because it uses only a small subset of points in the whole computational domain. Additionally, since the Gaussian function has the property of dimensional separation, the GA-LRBF method is suitable for dealing with isotropic images. Finally, a numerical scheme that couples GA-LRBF with the fourth-order Runge–Kutta method is applied to the C-V model, and a comparison of some numerical results demonstrates that this scheme achieves much more reliable image segmentation.

---

Graphic Abstract

---

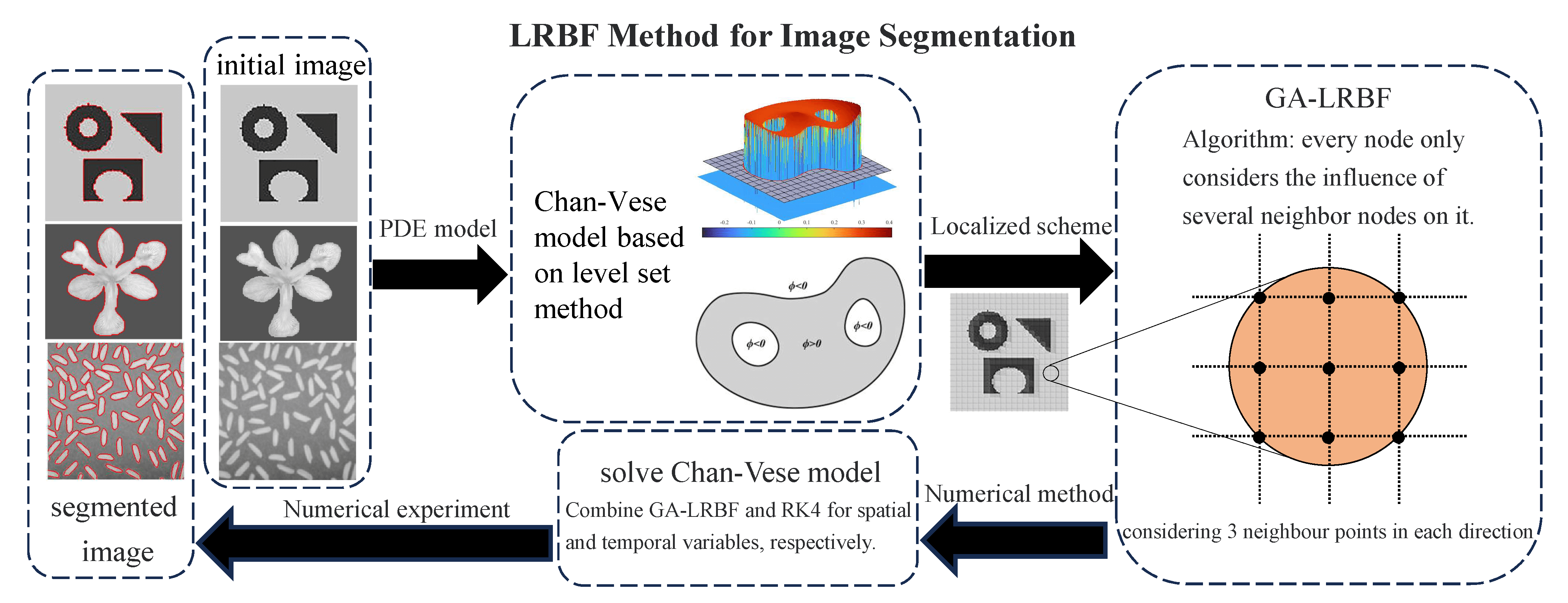
Keywords

---