Covert Communication in Integrated High Altitude Platform Station Terrestrial Networks

1  Introduction

The Internet of Things (IoT) is an information carrier based on the Internet and traditional telecommunication networks, which allows all ordinary physical objects that can be independently addressed to form an interconnected network. Nowadays, because the demand for large-scale IoT can not be satisfied by the fifth generation (5G) terrestrial mobile communication, the sixth generation (6G) terrestrial mobile communication development is becoming the general trend. Moreover, over recent years, high altitude platform station (HAPS) has been considered a critical part of the next-generation wireless communication networks for its high altitude, large capacity, flexible communication services, lower communication delay, and smaller terrestrial receiving antenna [1]. Without loss of generality, HAPS can be regarded as a “pseudo satellite,” which is viewed as a supplement or substitute for satellites in many scenes [2]. In addition, as an exemplary network architecture for future communications, the integrated HAPS terrestrial network has momentous practical significance, which can overcome obstacles and geographical constraints flexibly, compared with the integrated satellite-terrestrial network (ISTN) [3,4]. Karabulut Kurt et al. [5] provided a vision and framework for the HAPS networks of the future, including highlighting the unrealized potential of HAPS systems and elaborating on their unique ability to serve metropolitan areas. Liu et al. [6] studied the performance of non-orthogonal multiple access (NOMA)-based integrated satellite-terrestrial relay network (ISTRN) in the presence of multiple primary users (PUs) under a spectrum sharing environment. Shuai et al. [7] investigated the performance of NOMA-based integrated satellite-HAPS-terrestrial networks with transmit antenna selection (TAS) in the presence of imperfect channel state information (CSI) and successive interference cancelation (SIC). Furthermore, the performance of the NOMA-based overlay cognitive integrated satellite-terrestrial relay network with secondary network selection was investigated by Liu et al. [8]. The combined effects of channel estimation errors and hardware impairments on the secrecy performance of cognitive integrated satellite-terrestrial relay networks were researched by Guo et al. [9]. Moreover, Guo et al. [10] studied the performance of the reconfigurable intelligent surface-assisted integrated satellite-unmanned aerial vehicle (UAV)-terrestrial networks with hardware impairments and interference in the presence of an unavailable direct link. Gao et al. [11] optimized the deployment of an aerial reconfigurable intelligent surface (ARIS) to assist the HAPS down-link transmission when the direct link was blocked. Pace et al. [12] investigated optimal routing issues in a multilayered terrestrial-HAPS-satellite network. The massive access for a satellite-aerial-terrestrial network (SATN) was investigated by Huang et al. [13], where a HAPS was deployed as a relay to assist the uplink transmission from terrestrial user equipment (UE) to satellite.

However, the openness of wireless communication and the broad coverage of the HAPS beam will lead to a series of security problems, such as channel monitoring, information eavesdropping, and malicious interruption. Generally, many traditional security policies focus on preventing the extraction of information, such as coding encryption or physical layer security technology using information theory. Popovic et al. [14] analyzed and compared the security features of network architectures based on HAPS and satellites by proposing a security comparison method for network architectures that were based on airborne infrastructure. A new selective encryption and decryption framework based on the start code for high-efficiency video coding was proposed by Lee et al. [15]. On the other hand, Guo et al. [16] investigated the impacts of joint relay selection and user scheduling scheme on the physical layer security for a hybrid satellite-terrestrial relay system. Yerrapragada et al. [17] analyzed new schemes for securing applications at low latency by extending physical layer security (PLS) algorithms to beyond-5G/6G systems and designed protocols that advanced a specific form of PLS. The PLS performance of a wireless communication link through a large reflecting surface (LRS) with phase errors was analyzed by Vega Sanchez et al. [18]. Zhang et al. [19] developed a novel layered physical layer security model to secure multiple messages simultaneously.

Nevertheless, preventing transmission from being perceived is considered a more direct and effective measure. Compared with the above means, covert communication has more advantages than other security policies, which can prevent transmission content and behavior from being detected by non-cooperative eavesdroppers to solve the problem of information security fundamentally. Based on this, many scientists and engineers have focused on the research of covert communication. Covert communication was first cited in military communications by Prescott et al. [20]. Recently, the restriction theory of information transmission was being studied deeply by Bash et al. [21,22]. It was proved that to meet the demands of covert communication in the AWGN channel, the transmission power of the transmitter decreased with the increase of codeword length, resulting in a near-zero effective covert communication rate on both sides of legal communication which is meaningless [23]. Nevertheless, in the actual wireless communication environment, there are many interference signals which can be used to interfere with the eavesdropper’s communication signal [24]. Namely, widespread interference can be used to hide the transmission of information. The interference was firstly proposed to be utilized to realize a positive covert communication by Li et al. [25]. Topal et al. [26] provided a physical layer supported defense mechanism against traffic analysis attacks by exploring the covert communication capability of digital modulation schemes. Covert communication between a pair of legitimate transmitter-receiver against a watchful warden over slow fading channels was studied by Zheng et al. [27]. Yang et al. [28] considered covert communications having uncertainty about the noise variance in fading channels where an eavesdropper used a radio-meter detector to detect the legitimate signal. Furthermore, Gao et al. [29] studied the performance of covert communication under a scenario consisting of a source-destination pair, a passive warden, and multiple relays. The requirement of information freshness in covert communications was considered for the first time by Wang et al. [30]. Su et al. [31] investigated the covert communication performance in relay networks with relay selection. Zhang et al. [32] considered a covert mmWave communication system, where legitimate parties Alice and Bob adopted a beam training approach for directional link establishment. A new framework for jointly characterizing the covertness and timeliness of short-packet communications was developed by Yang et al. [33], in which a new metric named covert age of information (CAoI) was first proposed and derived. Channel uncertainty was exploited to achieve covert communication in relay networks by Wang et al. [34], in which a transmitter sent messages to the legitimate receiver with the help of a relay, and the eavesdroppers wanted to detect the existence of the transmission.

Inspired by the works mentioned above, this article considers an integrated HAPS terrestrial network with one constant power auxiliary node, a terrestrial eavesdropper, a HAPS as the transmitter, and a terrestrial user as the receiver. Moreover, all nodes are equipped with a single omnidirectional antenna and operate in half-duplex mode. Specifically, the significant contributions of this paper are summarized below:

1.    Firstly, considering the single-user scenario, a novel integrated HAPS terrestrial covert communication network structure is established, and a constant power auxiliary node assists the covert communication between HAPS and the user.

2.    Secondly, considering the actual situation of signal transmission, such as rainfall attenuation and free path loss, the statistical characteristics of the channel are given. In addition, the covert constraints on universal significance are derived.

3.    Thirdly, based on the above, the closed expression of outage probability (OP) under this covert communication network is deduced to obtain more in-depth insights and laws. Furthermore, the index to measure the covert performance named effective covert communication rate is given.

4.    Finally, the numerical simulation results are given further to analyze key parameters’ impact on system covert performance. Moreover, the observation results are analyzed in detail.

2  System Model and Problem Formulation

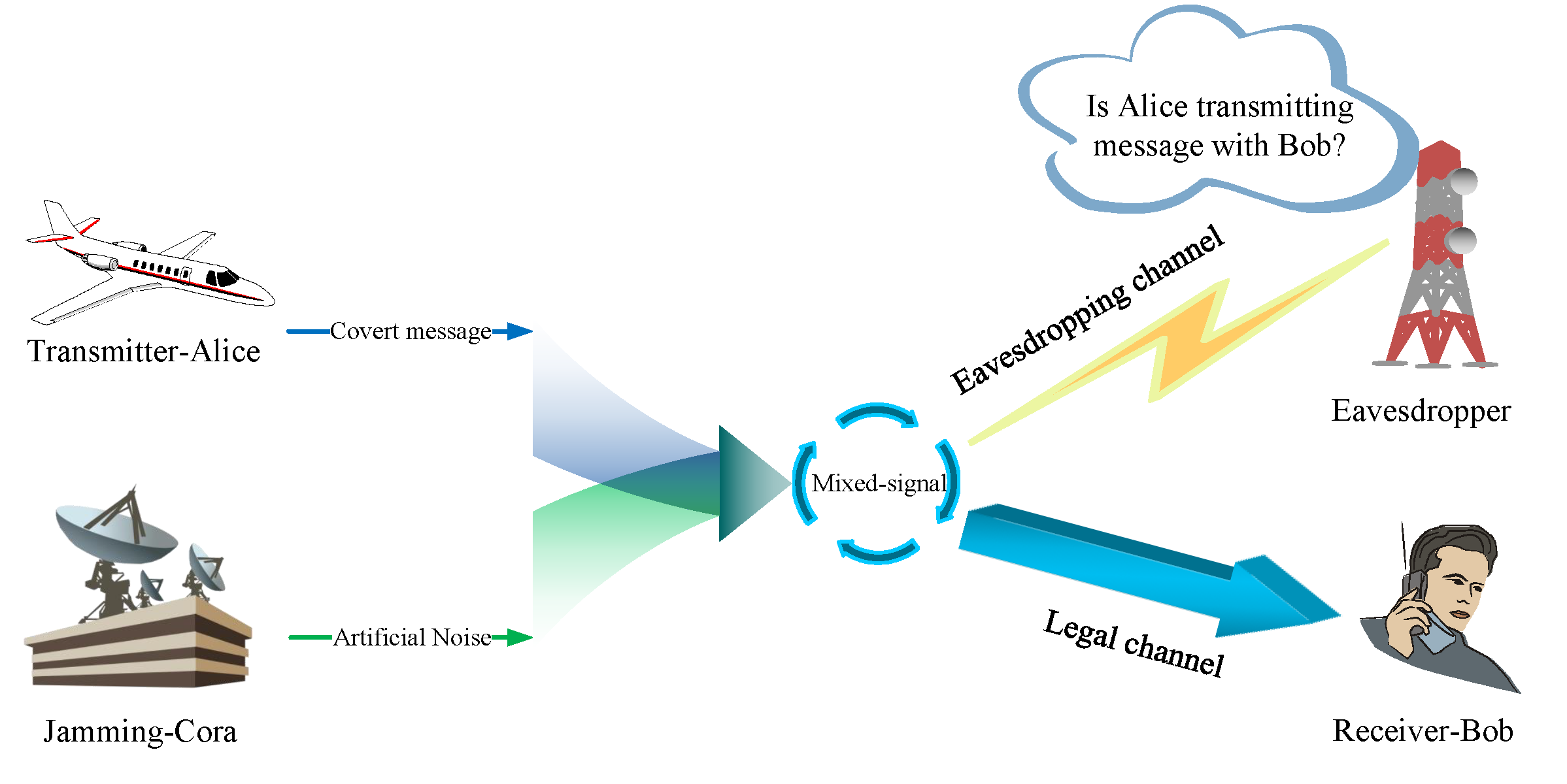
As shown in Fig. 1, an integrated HAPS terrestrial network is established, which consists of a HAPS A(Alice), a terrestrial user B(Bob), an auxiliary node C(Cora) near the A, and a terrestrial eavesdropper E(Eavesdropper). Alice and Bob are legal communication parties, and Alice is a transmitter that wants to transmit information to the receiver Bob and must be unknown to the Eavesdropper. In general, Eavesdropper knows the location of Alice and tends to detect its transmission behavior. Besides, Cora is the jammer deployed by Alice and Bob, which constantly emits a constant power of artificial noise (AN). Due to the persistent interference signal in the environment, it is assumed that Eavesdropper knows the existence of Cora. Moreover, all nodes are equipped with a single omnidirectional antenna and work in half-duplex mode.

Figure 1: System model

2.1 Channel Model

In order to get close to realistic transmission scenarios, the impacts of rain attenuation, free space loss, and antenna gain are considered in this system. Thus, the channel coefficient between i−th node and j−th node is given by

hij=Vijgij,(1)

where gij is the random channel coefficient of Shadowed-Rician (SR) fading, Vij=ωijξijψij/ζij, ζij is the rain attenuation coefficient which undergoes lognormal random distribution, ωij represents the free space loss coefficient which is decided by carrier frequency and the distance, ξij denotes the antenna gain of i−th node while ψij is the antenna gain at j−th node, (ij∈{ae,ce,ab,cb}).

In Fig. 1, all nodes are equipped with a single omnidirectional antenna and work in half-duplex mode. For specific analysis, it is assumed that there are discrete-time channels with N time slots between each node, and each time slot has n transmission symbol period or channel uses, which is expressed as the Fig. 2.

Figure 2: N time slot discrete channel

Moreover, we assume that the channels within the system experience standard SR fading, which means that the channel gain of each time slot remains unchanged but varies independently from one-time slot to another. Based on the above, it can be considered that the hij is modeled by quasi-stationary SR fading, which is a complex Gaussian random variable with E[|hij|2]=1. Meanwhile, only a downlink time slot is considered in the subsequent analysis of covert communication performance. Finally, it is assumed that the time slots between the nodes are strictly synchronized, and the prior probability of Alice performing covert information transmission in each time slot is equal, which is 0.5.

Besides, following the general assumptions in covert communication theory [22], we assume that Alice and Bob share a long enough key in advance so that Bob knows the transmission strategy of Alice, but Eavesdropper knows nothing about it.

2.2 Signal Model

Regarding a given maximum correct detection probability η of Eavesdropper, there is a constraint on the average signal-to-noise ratio (SNR) of Alice and Cora. The information transmission process needs to be analyzed first to investigate the constraint relationship between them. And the received signal at user Bob can be given by

yb(k)={PchcbDcb−α/2xc(k)+nb(k)H0PahabDab−α/2xa(k)+PchcbDcb−α/2xc(k)+nb(k)H1,(2)

where k=1,2,…,n is the symbol subscript in one time slot, α denotes the path loss coefficient, hij(ij∈{ae,ce,ab,cb}) represents the channel fading coefficient, Dij is the node spacing, xa and xc denotes the complex transmission signal of Alice and Cora, nb is the complex Gaussian white noise received by Bob with nb(k)∼CN(0,σb2). Pa and Pc denote the transmission power of Alice and Cora, respectively. H1 represents Alice sends valid signals to Bob and H0 is the opposite.

Similarly, the received signal of Eavesdropper can be given by

ye(k)={PchceDce−α/2xc(k)+ne(k)H0PahaeDae−α/2xa(k)+PchceDce−α/2xc(k)+ne(k)H1.(3)

where ne is the complex Gaussian white noise received by Eavesdropper, and ne(k)∼CN(0,σe2).

2.3 Problem Formulation

In our model, only a single auxiliary node is considered, and the energy detection method is adopted by Eavesdropper, which has been proved as the best method in quasi-stationary fading channel [25].

The Eavesdropper adopts the energy detection method to probe and judge whether Alice is transmitting the information. Namely, when the average power of the Eavesdropper’s received signal is larger than the preset energy detection threshold, Eavesdropper considers Alice, and Bob are transmitting signals to each other, and when the average power of the Eavesdropper’s received signal is less than the preset energy detection threshold, Eavesdropper considers that there is no information transmission between Alice and Bob, which is given by

Pe>D1<D0λ,(4)

where Pe=1/n∑k=1n|ye(k)|2 represents the average power of the Eavesdropper’s received signal in a time slot, λ is the preset energy detection threshold, and D0 and D1 respectively express that the judgment results of Eavesdropper are H0 and H1.

Therefore, the error detection of Eavesdropper can be divided into two categories as

Pfa=P(Pe≥λ|H0)Pmd=P(Pe≤λ|H1),(5)

where Pfa is the false alarm probability and Pmd is the missed detection probability.

On the above foundation, the requirements of covert communication can be expressed as

for∀min⁡η>0,Pfa+Pmd>1−η.(6)

Furthermore, since the probability density functions of |hae|2 and |hce|2 are continuous and convergent, when η is given, the following formulas can be satisfied, which is given by

{P(σc2>σ^c2)<η4P(σa2>σ^a2)<η8,(7)

where σa2=|hae|2Dae−αPa and σc2=|hce|2Dce−αPc. σ^a and σ^c are corresponding constants. Under the condition of H0, we can get

Pe=σc21χn2+σe22χn2n,(8)

where 1χn2 stands for the noncentral chi−square distribution with degrees of freedom n, while 2χn2 stands for the chi−square distribution with degrees of freedom n.

According to the weak law of large numbers and some mathematical steps, when σc2≤σ^c2 and n is large enough, we can obtain

Pfa(σc2)=P(Pe≥λ1|H0)>1−η2,(9)

where λ1<σc2+σe2−θ and θ is a constant greater than zero.

Proof. See Appendix A.

Similarly, when σc2≤σ^c2, σa2≤σ^a2 and n is large enough, we can get

Pmd(σc2,σa2)=P(Pe≤λ2|H1)>1−η2,(10)

where λ2>σa2+σc2+σe2+θ.

By means of (9) and (10), when n is large enough, we can derive

Pfa(σc2)+Pmd(σc2,σa2)>1−η2,(11)

Moreover, the sufficient conditions of (11) can be expressed as

{σc2≤σ^c2σa2≤σ^a2(σc2,σa2)∈BBC={σc2+σe2−θ<λ<σc2+σa2+σe2+θ},(12)

where BC represents the complement of set B.

With the help of (12) and in the light of the different fading conditions involved, we can obtain

P(BC)=P(λ−σe2−σa2−θ<σc2<λ−σe2+θ),(13)

P(BC|σa2<σ^a2)≤8(σ^a2+2θ)Dce−αPc,(14)

Proof. See Appendix B.

Then, the following formula can be obtained as

P(Z)=({σc2≤σ^c2}∩{σa2≤σ^a2}∩B)≥[1−P(BC|σa2≤σ^a2)]P(σa2≤σ^a2)−P(σc2≥σ^c2)>1−η2,(15)

where Z is the set represents all the conditions in (12) hold together, σ^a2=Dce−αPcη128 and θ=Dce−αPcη256 according to formula (14).

Hence, the average error detection of Eavesdropper can be expressed as

Pfa+Pmd=E(σc2,σa2)[Pfa(σc2)+Pmd(σc2,σa2)]>[Pfa(σc2)+Pmd(σc2,σa2)|Z]P(Z)>(1−η2)2>1−η,(16)

In conclusion, Alice is able to meet the requirements of covert communication in (6) by controlling own transmit power, and due to the above formula (7), the covert constraint relationship between γ¯ce and γ¯ae is given by

P(hae2>η128γ¯ceγ¯aeDce−αDae−α)<η8.(17)

where γ¯ae=Pa/σe2 and γ¯ce=Pc/σe2.

3  Performance Analysis

In this section, the statistical properties of SR fading are provided. On this basis, the exact expression of OP for the covert communication in this integrated HAPS terrestrial network is obtained. Besides, we derive the closed-form expression of the effective covert communication rate to measure the system’s covert communication performance.

3.1 Statistical Properties of Channels

Firstly, the probability distribution function (PDF) and cumulative distribution function (CDF) of SR fading are obtained. From [35], the PDF of gij is given by

f|gij|2(x)=αije−βijx1F1(mij;1;δijx),(18)

where αij=Δ12bij(2bijmij2bijmij+Ωij)mij, βij=Δ12bij, δij=ΔΩij2bij(2bijmij+Ωij), mij represents the Nakagami-m parameter which is always greater than 0, and 2bij is the average power of the multi-path part, while Ωij is that of line of sight (LOS) part.

Under the situation that mij is an integer, with the utilizing of [36], 1F1(mij;1;δijx) is represented as

1F1(mij;1;δijx)=e−δijx∑n=0mij−1(−δij)n(1−mij)n(n!)2xn.(19)

According to (18) and (19), the PDF of gab can be re-written as

f|gij|2(x)=αij∑n=0mij−1(−δij)n(1−mij)n(n!)2xne−(βij−δij)x,(20)

From (1) and γij=γ¯ij|hij|2 [37], we can get the PDF and CDF of γij as

fγij(x)=αij∑n=0mij−1μxne−Δijx,(21)

Fγij(x)=1−αij∑n=0mij−1∑t=0nn!μ(t!)(Δij)n−t+1xte−Δijx.(22)

where Δij=(βij−δij)γ¯ij and μ=(−δij)n(1−mij)n(n!)2(γ¯ij)n+1.

3.2 OP

OP can well evaluate the performance of the system, and in this paper we define the OP as the probability of the instantaneous capacity for any node lower than its excepted capacity. When Alice communicates with Bob legally, the SNR of the received signal at Bob can be given by

γb=Dab−αγabDcb−αγcb+1,(23)

where γab=(hab2Pa)/σb2 and γcb=(hcb2Pc)/σb2.

Assuming that the default coding rate of Alice is R^ab, the OP of transmission between Alice and Bob which is given by

Pout=P(Cab<R^ab),(24)

where Cab=log2(1+γb).

By substituting (21) into (22), we can obtain the OP as

Pout=P(Dab−αγabDcb−αγcb+1<γth)=∫0∞∫0γth(Dcb−αγcb+1)Dab−αfγab(x)fγcb(y)dxdy,(25)

where γth=2R^ab−1.

Furthermore, the final expression of OP can be derived as

Pout=1−αabαcbe−Δab(γthDab−α)∑n1=0mab−1∑n2=0mcb−1∑t=0n1∑p=0t(tp)ω(n1)ω(n2)ϕ(n1,t)φ(n2,p).(26)

where

ω(n1)=(−δab)n1(1−mab)n1(n1!)2(γ¯ab)n1+1,(27)

ω(n2)=(−δcb)n2(1−mcb)n2(n2!)2(γ¯cb)n2+1,(28)

ϕ(n1,t)=(γthDab−α)t(n1!)(Δab)n1−t+1(t!),(29)

φ(n2,p)=(Dcb−α)p(n2+p)!(ΔabγthDcb−αDab−α+Δcb)n2+p+1.(30)

3.3 Effective Covert Communication Rate

Effective covert communication rate is also a momentous and more intuitive index in the covert communication system, defined as the average ratio of practical transmission information to the transmission time from transmitter to the receiver under all fading conditions. Finally, the effective covert communication rate Rab can be expressed as

Rab=(1−Pout)R^ab,(31)

Furthermore, the final expression of the effective covert communication rate is given by

Rab=R^abαabαcbe−Δab(γthDab−α)∑n1=0mab−1∑n2=0mcb−1∑t=0n1∑p=0t(tp)ω(n1)ω(n2)ϕ(n1,t)φ(n2,p).(32)

4  Numerical Results

In this section, the critical constraint relationship of average SNR between the transmitting node and the auxiliary node, and the effective covert communication rate of the considered system are proved through MC simulations. In general, we set Dab−α=Dcb−α=1 and it is assumed that hab and hcb own the same fading condition. In addition, we assume that a certain covert constraint is maintained in different fading channels. The SR fading channel parameters [38] are given by

•   Frequent heavy shadowing (FHS): mij=1,bij=0.063,Ωij=0.0007;

•   Average shadowing (AS): mij=5, bij=0.251, Ωij=0.279;

•   Infrequent light shadowing (ILS): mij=10, bij=0.158, Ωij=1.29.

Fig. 3 shows that γ¯ce increases with γ¯ae in a linear proportional relationship with a given maximum allowable correct detection probability η under the same fading conditions, which can be explained that the Eavesdropper can receives successfully only when receiving SNR (γ¯aeγ¯ce) is greater than its detection sensitivity. Moreover, this proportion (γ¯ceγ¯ae) decreases with the increase of η, which indicates that the higher covert demand leads to the stricter constraint relationship between γ¯ae and γ¯ce. Hence, γ¯ae and γ¯ce should be chosen appropriately according to η. Finally, we can see that when the fading is more serious, the required average SNR of the auxiliary node is lower because it is difficult for the Eavesdropper to acquire correct judgment under serious fading conditions, which is equivalent to natural interference. At this moment, covert communication can be realized with less interference from the auxiliary node.

Figure 3: Covert constraint of γ^

Fig. 4 depicts the relationship between R^ab and Rab with setting γ¯ab=20dB. It can be observed that Rab first increases and then degrades with the rise of R^ab. It is because there is an optimal R^ab, which can be adjusted to obtain the maximum effective covert communication rate. Moreover, a larger η or the more serious fading conditions, which means more loose covert constraints or natural interference, can enhance the effective covert communication rate.

Figure 4: Rab vs. R^ab

Figs. 5 and 6 plot that Rab vs. different γ¯ab, including linear and logarithmic forms, which can show different conclusions, respectively. We set the coding rate R^ab=0.1bit/symbol. Under the given η, it can be found that Rab reaches a stable limit value in high average SNR regimes from Fig. 5, where γb is approximately the proportional coefficient of the average SNR. In addition, it can be observed that the rise of the maximum allowable correct detection probability η loses the covert constraints, and a higher effective covert communication rate can be obtained. From Fig. 6, we can realize that the worse fading condition in high average SNR regimes will lead to better performance, while the rate under FHS is lower than that under AS or ILS in low average SNR regimes. Due to that, much more serious fading conditions will prominently influence the quality of legitimate transmission service in a low SNR regime. In contrast, the worse channel condition will help implement covert communication easier.

Figure 5: Rab vs. γ^ (linear)

Figure 6: Rab vs. γ^ (logarithm)

5  Conclusions

This article systematically investigated the covert constraint and effective performance of covert communication in the integrated HAPS terrestrial networks, which utilized an auxiliary node with constant power. In particular, the covert constraint relationship between the transmitting node and the auxiliary node was discussed. Furthermore, the exact expressions of OP and effective covert communication rate were derived. According to numerical results, we found that the power of the transmitting node and auxiliary node under the covert constraint had a linear relationship. Secondly, the tightening of the covert constraint caused the deterioration of the achievable effective covert communication rate. Thirdly, there was an optimal preset coding rate to obtain the maximum effective covert communication rate. In addition, we knew that it was difficult for the Eavesdropper to acquire correct judgment under severe fading conditions, which means natural interference, namely, covert communication, can be realized with less interference of the auxiliary node. Similarly, it was worth noting that the worse fading condition would enhance effective performance in the high average SNR regimes.

Acknowledgement: Thanks to all the authors for their contributions to this paper. The authors would like to thank the anonymous reviewers for their insightful suggestions that helped us improve this paper’s quality.

Funding Statement: This work was supported by the National Science Foundation of China under Grant 62001517, in part by the Research Project of Space Engineering University under Grants 2020XXAQ01 and 2019XXAQ05, and in part by the Science and Technology Innovation Cultivation Fund of Space Engineering University.

Conflicts of Interest: The authors declare that they have no conflicts of interest regarding the publication of this article.

References

 1.  Mohammed, A., Mehmood, A., Pavlidou, F. N., Mohorcic, M. (2011). The role of high-altitude platforms in the global wireless connectivity. Proceedings of the IEEE, 99(11), 1939–1953. [Google Scholar]

 2.  An, K., Li, Y., Yan, X., Liang, T. (2019). On the performance of cache-enabled hybrid satellite-terrestrial relay networks. IEEE Wireless Communications Letters, 8(5), 1506–1509. DOI 10.1109/LWC.5962382. [Google Scholar] [CrossRef]

 3.  Zhang, X., Guo, D., An, K., Zheng, G., Chatzinotas, S. et al. (2021). Auction-based multichannel cooperative spectrum sharing in hybrid satellite-terrestrial IoT networks. IEEE Internet of Things Journal, 8(8), 7009–7023. DOI 10.1109/JIOT.2020.3037408. [Google Scholar] [CrossRef]

 4.  Lin, Z., Lin, M., Zhu, W. P., Wang, J. B., Cheng, J. (2021). Robust secure beamforming for wireless powered cognitive satellite-terrestrial networks. IEEE Transactions on Cognitive Communications and Networking, 7(2), 567–580. DOI 10.1109/TCCN.2020.3016096. [Google Scholar] [CrossRef]

 5.  Karabulut Kurt, G., Khoshkholgh, M. G., Alfattani, S., Ibrahim, A., Darwish, T. S. J. et al. (2021). A vision and framework for the high altitude platform station (HAPS) networks of the future. IEEE Transactions on Cognitive Communications and Networking, 23(2), 729–779. [Google Scholar]

 6.  Liu, R., Guo, K., An, K., Zhu, S., Shuai, H. (2021). Performance analysis of noma-based overlay cognitive integrated satellite-aerial-terrestrial networks. IEEE Wireless Communications Letters, 10(6), 1266–1270. DOI 10.1109/LWC.2021.3063759. [Google Scholar] [CrossRef]

 7.  Shuai, H., Guo, K., An, K., Huang, Y., Zhu, S. (2022). Transmit antenna selection in noma-based integrated satellite-HAP-terrestrial networks with imperfect CSI and SIC. IEEE Wireless Communications Letters, 11(8), 1565–1569. DOI 10.1109/LWC.2022.3165710. [Google Scholar] [CrossRef]

 8.  Liu, R., Guo, K., An, K., Zhu, S. (2022). Noma-based overlay cognitive integrated satellite-terrestrial relay networks with secondary network selection. IEEE Transactions on Vehicular Technology, 71(2), 2187–2192. DOI 10.1109/TVT.2021.3122029. [Google Scholar] [CrossRef]

 9.  Guo, K., Dong, C., An, K. (2022). Noma-based cognitive satellite terrestrial relay network: Secrecy performance under channel estimation errors and hardware impairments. IEEE Internet of Things Journal. DOI 10.1109/JIOT.2022.3157673. [Google Scholar] [CrossRef]

10. Guo, K., An, K. (2018). On the performance of RIS-assisted integrated satellite-UAV-terrestrial networks with hardware impairments and interference. IEEE Wireless Communications Letters, 22(6), 1240–1243. [Google Scholar]

11. Gao, N., Jin, S., Li, X., Matthaiou, M. (2022). Aerial RIS-assisted high altitude platform communications. IEEE Wireless Communications Letters, 11(1), 131–135. [Google Scholar]

12. Pace, P., Aloi, G. (2007). Effective routing algorithm for multilayered terrestrial-HAP-satellite networks. IEEE Communications Letters, 11(6), 510–512. DOI 10.1109/LCOMM.2007.070238. [Google Scholar] [CrossRef]

13. Huang, Q., Lin, M., Zhu, W. P., Cheng, J., Alouini, M. S. (2021). Uplink massive access in mixed RF/FSO satellite-aerial-terrestrial networks. IEEE Transactions on Communications, 69(4), 2413–2426. DOI 10.1109/TCOMM.2021.3049364. [Google Scholar] [CrossRef]

14. Popovic, M., Basicevic, I. (2010). On security advantages of HAPS over satellites. URSI Radio Science Bulletin, 2010(334), 19–24. [Google Scholar]

15. Lee, M. K., Jang, E. S. (2020). Start code-based encryption and decryption framework for HEVC. IEEE Access, 8, 202910–202918. DOI 10.1109/Access.6287639. [Google Scholar] [CrossRef]

16. Guo, K., An, K., Zhang, B., Huang, Y., Guo, D. (2018). Physical layer security for hybrid satellite terrestrial relay networks with joint relay selection and user scheduling. IEEE Access, 6, 55815–55827. DOI 10.1109/ACCESS.2018.2872718. [Google Scholar] [CrossRef]

17. Yerrapragada, A. K., Eisman, T., Kelley, B. (2021). Physical layer security for beyond 5G: Ultra secure low latency communications. IEEE Open Journal of the Communications Society, 2, 2232–2242. DOI 10.1109/OJCOMS.2021.3105185. [Google Scholar] [CrossRef]

18. Sanchez, V., D, J., Espinosa, R. R., Lopez-Martinez, P., J, F. (2021). Physical layer security of large reflecting surface aided communications with phase errors. IEEE Wireless Communications Letters, 10(2), 325–329. DOI 10.1109/LWC.5962382. [Google Scholar] [CrossRef]

19. Zhang, W., Chen, J., Kuo, Y., Zhou, Y. (2019). Artificial-noise-aided optimal beamforming in layered physical layer security. IEEE Communications Letters, 23(1), 72–75. DOI 10.1109/LCOMM.2018.2881182. [Google Scholar] [CrossRef]

20. Prescott, G., Gutman, L., Connolly, D., Holtzman, J. (1991). A methodology for employing modulation quality factors in the analysis of lPI waveforms. MILCOM 91-Conference Record, 2, 532–536. DOI 10.1109/MILCOM.1991.258311. [Google Scholar] [CrossRef]

21. Wang, L., Wornell, G. W., Zheng, L. (2016). Fundamental limits of communication with low probability of detection. IEEE Transactions on Information Theory, 62(6), 3493–3503. DOI 10.1109/TIT.2016.2548471. [Google Scholar] [CrossRef]

22. Bash, B. A., Goeckel, D., Towsley, D. (2013). Limits of reliable communication with low probability of detection on AWGN channels. IEEE Journal on Selected Areas in Communications, 31(9), 1921–1930. DOI 10.1109/JSAC.2013.130923. [Google Scholar] [CrossRef]

23. Bloch, M. R. (2016). Covert communication over noisy channels: A resolvability perspective. IEEE Transactions on Information Theory, 62(5), 2334–2354. DOI 10.1109/TIT.2016.2530089. [Google Scholar] [CrossRef]

24. Tse, D., Viswanath, P. (2005). Fundamentals of wireless communication. London, UK: Cambridge University Press. [Google Scholar]

25. Li, K., Sobers, T., Towsley, D., Goeckel, D. (2017). Covert communication in the presence of an uninformed jammer. IEEE Transactions on Wireless Communications, 16(9), 6193–6206. DOI 10.1109/TWC.2017.2720736. [Google Scholar] [CrossRef]

26. Topal, O. A., Kurt, G. K. (2021). A countermeasure for traffic analysis attacks: Covert communications with digital modulation. IEEE Wireless Communications Letters, 10(2), 441–445. DOI 10.1109/LWC.5962382. [Google Scholar] [CrossRef]

27. Zheng, T. X., Yang, Z., Wang, C., Li, Z., Yuan, J. et al. (2021). Wireless covert communications aided by distributed cooperative jamming over slow fading channels. IEEE Transactions on Wireless Communications, 20(11), 7026–7039. DOI 10.1109/TWC.2021.3080382. [Google Scholar] [CrossRef]

28. Yang, H., Sun, L. (2020). Power allocation for covert wireless communications in fading channels. 2020 IEEE 6th International Conference on Computer and Communications (ICCC), pp. 20–25. Chengdu, China. [Google Scholar]

29. Gao, C., Yang, B., Jiang, X., Inamura, H., Fukushi, M. (2021). Covert communication in relay-assisted IOT systems. IEEE Internet of Things Journal, 8(8), 6313–6323. DOI 10.1109/JIOT.2021.3051694. [Google Scholar] [CrossRef]

30. Wang, Y., Yan, S., Yang, W., Cai, Y. (2021). Covert communications with constrained age of information. IEEE Wireless Communications Letters, 10(2), 368–372. DOI 10.1109/LWC.5962382. [Google Scholar] [CrossRef]

31. Su, Y., Sun, H., Zhang, Z., Lian, Z., Xie, Z. et al. (2021). Covert communication with relay selection. IEEE Wireless Communications Letters, 10(2), 421–425. DOI 10.1109/LWC.5962382. [Google Scholar] [CrossRef]

32. Zhang, J., Li, M., Yan, S., Liu, C., Chen, X. et al. (2021). Joint beam training and data transmission design for covert millimeter-wave communication. IEEE Transactions on Information Forensics and Security, 16, 2232–2245. DOI 10.1109/TIFS.10206. [Google Scholar] [CrossRef]

33. Yang, W., Lu, X., Yan, S., Shu, F., Li, Z. (2021). Age of information for short-packet covert communication. IEEE Wireless Communications Letters, 10(9), 1890–1894. DOI 10.1109/LWC.2021.3085025. [Google Scholar] [CrossRef]

34. Wang, J., Tang, W., Zhu, Q., Li, X., Rao, H. et al. (2019). Covert communication with the help of relay and channel uncertainty. IEEE Wireless Communications Letters, 8(1), 317–320. DOI 10.1109/LWC.2018.2872058. [Google Scholar] [CrossRef]

35. Miridakis, N. I., Vergados, D. D., Michalas, A. (2015). Dual-hop communication over a satellite relay and shadowed rician channels. IEEE Transactions on Vehicular Technology, 64(9), 4031–4040. DOI 10.1109/TVT.2014.2361832. [Google Scholar] [CrossRef]

36. The wolfram function site. http://functions.wolfram.com. [Google Scholar]

37. Brychkov, Y. (2008). Handbook of special functions: Derivatives, integrals, series and other formulas. Florida, USA: CRC Press. [Google Scholar]

38. Abdi, A., Lau, W., Alouini, M. S., Kaveh, M. (2003). A new simple model for land mobile satellite channels: First- and second-order statistics. IEEE Transactions on Wireless Communications, 2(3), 519–528. DOI 10.1109/TWC.2003.811182. [Google Scholar] [CrossRef]

Appendix A

Recalling the transmission model, under the condition of H0, we can obtain

Pe=σc21χn2+σe22χn2n,(33)

where 1χn2 stands for the noncentral chi−square distribution with degrees of freedom n, while 2χn2 stands for the chi−square distribution with degrees of freedom n.

Due to the weak law of large number and E[|hij|2]=1, we can get

1χn2n→probability⁡1,(34)

2χn2n→probability⁡1,(35)

Further, for ∀θ>0, there is a constant N0 which is large enough that for ∀n>N0, we can derive

P(Pe(σc2+σe2)∈(1−θσ^c2+σe2,1+θσ^c2+σe2))>1−η2,(36)

Moreover, for ∀n>N0, we can get

P(Pe∈((σc2+σe2)(1−θσ^c2+σe2),(σc2+σe2)(1+θσ^c2+σe2)))>1−η2,(37)

When σc2≤σ^c2, there is

σc2+σe2≤σ^c2+σe2,(38)

Therefore, at this time, for ∀n>N0, we can obtain

P(Pe∈(σe2+σc2−θ,σe2+σc2+θ))>1−η2,(39)

Hence, when σc2≤σ^c2 and n is large enough, (8) is proved which is given by

Pfa(σc2)=P(Pe≥λ1|H0)>1−η2,(40)

where λ1<σc2+σe2−θ and θ is a constant greater than zero.

Similarly, under the condition of H1, (10) can be proved by us.

Appendix B

Based on the previous Eq. (12) in this article, we have obtained

P(BC)=P(λ−σe2−σa2−θ<σc2<λ−σe2+θ),(41)

where σc2=|hce|2Dce−αPc.

Moreover, due to

P(XY)≤P(X)P(Y),(42)

we can get

P(BC|σa2<σ^a2)≤P(BC),(43)

Therefore, according to the scenario all we consider and the probability density distribution curve of |hce|2, We can deduce

P(BC)=P(λ−σe2−σa2−θDce−αPc<|hce|2<λ−σe2+θDce−αPc)≤8(σ^a2+2θ)Dce−αPc,(44)

Namely,

P(BC|σa2<σ^a2)≤8(σ^a2+2θ)Dce−αPc.(45)