Computer Modeling in Engineering & Sciences

DOI: 10.32604/cmes.2022.017368

ARTICLE

Design of Multi-Coupled Laminates with Extension-Twisting Coupling for Application in Adaptive Structures

Da Cui1,2, Daokui Li1,2,* and Shiming Zhou1,2

1College of Aerospace Science and Engineering, National University of Defense Technology, Changsha, 410073, China
2Hunan Key Laboratory of Intelligent Planning and Simulation for Aerospace Missions, Changsha, 410073, China
*Corresponding Author: Daokui Li. Email: lidaokui@nudt.edu.cn
Received: 07 May 2021; Accepted: 16 July 2021

1  Introduction

Passive adaptive structure has become the forefront and research hotspot in the present era with its unique advantages in the field of aerospace and wind power. For instance, the bending-twisting coupled principle bearing structure can control the aeroelastic deformation of the forward-swept wing aircraft, thereby inhibiting the rapid decline of the critical velocity caused by aeroelastic divergence and improving design performance and aeroelastic properties [1,2]. The adaptive bending-twisting coupled wind turbine blades structure enables the blades to change their aerodynamic torsion angles in real time according to wind speed, thus effectively avoiding blade damage and fracture under the action of strong wind [3,4].

The stiffness characteristics of the passive adaptive structure can be realized by the paving design of composite laminate [5]. The reasonable paving angle of each lamina enables the laminates to have special couplings, including extension-shearing [6], extension-twisting [7] and bending-twisting couplings [8], etc. Then, the structures composed of these laminates demonstrate the required coupling stiffness. It is worth noting that hygro-thermal deformation should be firstly considered in the paving design process of laminates. Under this premise, many scholars have studied various types of laminates to varying degrees. On the basis of the concept of hygro-thermal warpage stability proposed by Winckler [9], Cross et al. [10] designed asymmetrical composite materials without hygro-thermal warpage deformation. Then, Haynes et al. [7] optimized the stacking sequence of hygro-thermally warpage stable composite materials with extension-twisting coupling and bending-twisting coupling [11]. Subsequently, York successively studied the hygro-thermally warpage stable laminates of standard laminates [6] and other special angle laminates [12], and then designed extension-shearing and bending-twisting coupled laminated plates [13] to maximize the shear buckling strength of the adaptive flexible wing box structure. On this basis, a large number of researches on laminate layup design have been carried out [14,15]. Furthermore, the buckling load and coupling of hygro-thermally stable laminates were simultaneously considered by Li in the process of optimization design, including extension-shearing coupled laminates [16] and extension-twisting coupled laminates [17]. Cui designed and optimized the single fiber [18] and interlayer hybrid [19] extension-shearing coupled laminates with hygro-thermal stability.

The above-mentioned research is mainly aimed at the single coupling of laminate, and strives to achieve the best mechanical properties of laminates or composite structures formed by laminates. The single coupling of laminate limits the range of the paving angle to a large extent despite the fact that it is easy to design, resulting in the inability of the laminate to effectively give play to its advantages in improving mechanical properties in the form of free layup. Moreover, the single coupling of laminate is likely to cause limitations, such as bending-twisting coupling that may reduce buckling load [20]. Therefore, this article focuses on multi-coupled laminates, expecting to achieve a significant improvement in the mechanical properties.

The multi-coupled laminates refer to laminates that contain two or more couplings that can exist independently at the same time. There are few studies on multi-coupled laminates in existing research. Moore et~al. [5] realized the paving design of the hygro-thermally isotropic laminate with the maximum extension-twisting coupling, and the optimized laminate had both extension-twisting coupling and extension-bending couplings. Subsequently, York et al. [13] designed a laminate with both extension-shearing and bending-twisting couplings to maximize the shear buckling strength of the adaptive flexible wing box structure. In view of the advantages of single-coupled laminates in improving mechanical properties and overcoming the limitations, the paving design of multi-coupled laminates was studied.

The innovation of this article lies in improving the comprehensive mechanical properties of the composite structure from the perspective of the multi-couplings of the laminate. The universal analytical conditions for hygro-thermally stable multi-coupled laminates with extension-twisting coupling were established. Then, the multi-coupled laminates were used to design hygro-thermally stable adaptive structures, in which the configuration of laminate and structure could be extended to variable cross-section configuration. The optimal design was realized for the multi-coupled laminates and adaptive structures. Meanwhile, the effect of multi-coupling on laminates and adaptive structures was explored in terms of coupling effect, failure strength, bucking load and robustness.

2  Multi-Coupled Laminates

There are many kinds of multi-coupled laminates. Considering the extension-twisting coupling (a kind of extensively studied coupling) of laminate plays a notable role in the design of adaptive structures, the multi-coupled laminates with such coupling are studied in the following research. In order to maximize the advantages of laminates, the paving form of laminates should be no longer limited to special forms such as standard layups and symmetrical layups. The possible coupling, except extension-twisting coupling, may possess include one or more of the following couplings: extension-shearing, extension-bending, bending-twisting and shearing-twisting couplings. It is worth noting that the coexistence of extension-shearing coupling and extension-twisting coupling will not be conducive to the improvement of the adaptive ability of the structure. It mainly reflects that the paving orders of the upper and lower flanges of adaptive box structure are completely the opposite. Thus, other couplings here do not include extension-shearing coupling.

2.1 Double-Coupled Laminates

In addition to extension-twisting coupling, other coupling of double-coupled laminates may include one of the following couplings: extension-bending coupling, shearing-twisting coupling and bending-twisting coupling. The ASBltDS laminate (extension-bending and extension-twisting coupled) was firstly studied. Based on the classical laminates theory (CLT) [21], extension-twisting coupling coefficients B16,B26 and extension-bending coupling coefficients B11,B22 should not be zero. Meanwhile, remaining stiffness coefficients should be zero. The stiffness equation is expressed as

{NxNyNxyMxMyMxy}=[A11A120B110B16A12A2200B22B2600A66B16B260B110B16D11D1200B22B26D12D220B16B26000D66]{εx0εy0γxy0κxκyκxy}(1)

The stiffness coefficients Aij,Bij,Dij(i,j=1,2,6) of laminate can be calculated by the material constants Ui(i=1,2,…,5) of each lamina and the geometric factors ξi(i=1,2,…,12) of laminate [16], namely

{A11A12A22A66A16A26}=H[1ξ1ξ20000−ξ2101−ξ1ξ20000−ξ2010ξ3/2ξ4000ξ3/2−ξ400]{U1U2U3U4U5},{B11B12B22B66B16B26}=H24[0ξ5ξ60000−ξ6000−ξ5ξ60000−ξ6000ξ7/2ξ8000ξ7/2−ξ800]{U1U2U3U4U5},{D11D12D22D66D16D26}=H312[1ξ9ξ100000−ξ10101−ξ9ξ100000−ξ10010ξ11/2ξ12000ξ11/2−ξ1200]{U1U2U3U4U5}(2)

where, the expressions of Ui and ξi are shown in Eq. (3). The purpose of using geometric factors is applicable to all elastic composite materials.

{U1=18(3Q11+3Q22+2Q12+4Q66),U2=12(Q11−Q22),U3=18(Q11+Q22−2Q12−4Q66),U4=18(Q11+Q22+6Q12−4Q66),U5=18(Q11+Q22−2Q12+4Q66),(ξ1ξ2ξ3ξ4)=12∑k=1n(cos⁡2θkcos⁡4θksin⁡2θksin⁡4θk)(2zkH−2zk−1H),(ξ5ξ6ξ7ξ8)=12∑k=1n(cos⁡2θkcos⁡4θksin⁡2θksin⁡4θk)[(2zkH)2−(2zk−1H)2],(ξ9ξ10ξ11ξ12)=12∑k=1n(cos⁡2θkcos⁡4θksin⁡2θksin⁡4θk)[(2zkH)3−(2zk−1H)3](3)

where, H is the thickness of the laminate; θk(k=1,2,…,n) and zk(k=1,2,…,n) are the layer angle and position of k-ply lamina. Substituting Eq. (2) into Eq. (1) gives

ξ3=ξ4=ξ6=ξ11=ξ12=0,|ξ5|+|ξ6|≠0,|ξ7|+|ξ8|≠0(4)

The relationships of hygro-thermal stability for laminates are [18]

ξ1=ξ3=ξ5=ξ7=0(5)

However, ξ5 cannot satisfy the relationships between Eqs. (4) and (5), indicating the non-existence of hygro-thermally stable ASBltDS laminate.

Then, considering ASBtDF laminate (bending-twisting and extension-twisting coupled), whose stiffness equation is

{NxNyNxyMxMyMxy}=[A11A12000B16A12A22000B2600A66B16B26000B16D11D12D1600B26D12D22D26B16B260D16D26D66]{εx0εy0γxy0κxκyκxy}(6)

Inserting Eq. (2) into Eq. (6), it yields

ξ3=ξ4=ξ5=ξ6=0,|ξ7|+|ξ8|≠0,|ξ11|+|ξ12|≠0(7)

Substituting Eq. (7) into Eq. (5), we obtain hygro-thermally stable ASBtDF laminate

ξ1=ξ3=ξ4=ξ5=ξ6=ξ7=0,ξ8≠0,|ξ11|+|ξ12|≠0(8)

Furthermore, the extension-bending coupling must co-exist with shearing-twisting coupling (B12=B66≠0), indicating that there is no laminate with only shearing-twisting coupling or extension-twisting coupling. In brief, when the double-coupled laminate with extension-twisting coupling is used to construct hygro-thermally stable composite structures, only ASBtDF laminate is feasible.

2.2 Triple- and Quadruple-Coupled Laminates

The shearing-twisting coupling will produce the extension-bending coupling (B12=B66≠0); however, the reverse is not necessarily true (B12=B66=0,B11≠0,B22≠0). Thus, there are two kinds of triple-coupled laminates: ASBFDS laminate (shearing-twisting, extension-bending and extension-twisting coupled) and ASBltDF laminate (bending-twisting, extension-bending and extension-twisting coupled). In terms of quadruple-coupled laminates, only ASBFDF laminate (bending-twisting, extension-bending, shearing-twisting and extension-twisting coupled) is feasible.

The stiffness equation of ASBFDS laminate is

{NxNyNxyMxMyMxy}=[A11A120B11B12B16A12A220B12B22B2600A66B16B26B66B11B12B16D11D120B12B22B26D12D220B16B26B6600D66]{εx0εy0γxy0κxκyκxy}(9)

Substituting Eq. (2) into Eq. (9), it gives

ξ3=ξ4=ξ11=ξ12=0,ξ6≠0,|ξ7|+|ξ8|≠0(10)

Substituting Eq. (10) into Eq. (5), we obtain the hygro-thermally stable ASBFDS laminate, namely

ξ1=ξ3=ξ4=ξ5=ξ7=ξ11=ξ12=0,ξ6≠0,ξ8≠0(11)

Furthermore, the stiffness equation of ASBltDF laminate is expressed as

{NxNyNxyMxMyMxy}=[A11A120B110B16A12A2200B22B2600A66B16B260B110B16D11D12D160B22B26D12D22D26B16B260D16D26D66]{εx0εy0γxy0κxκyκxy}(12)

Substituting Eq. (2) into Eq. (12), it gives

ξ3=ξ4=ξ6=0,ξ5≠0,|ξ7|+|ξ8|≠0,|ξ11|+|ξ12|≠0(13)

However, ξ5 cannot satisfy the relationships between Eqs. (13) and (5), indicating the non-existence of hygro-thermally stable ASBltDF laminate.

Similarly, for composite ASBFDF laminates, the stiffness equation is

{NxNyNxyMxMyMxy}=[A11A120B11B12B16A12A220B12B22B2600A66B16B26B66B11B12B16D11D12D16B12B22B26D12D22D26B16B26B66D16D26D66]{εx0εy0γxy0κxκyκxy}(14)

Substituting Eq. (2) into Eq. (14), it yields

ξ3=ξ4=0,ξ6≠0,|ξ7|+|ξ8|≠0,|ξ11|+|ξ12|≠0(15)

Substituting Eq. (15) into Eq. (5), we obtain hygro-thermally stable ASBFDF laminate, namely

ξ1=ξ3=ξ4=ξ5=ξ7=0,ξ6≠0,ξ8≠0,|ξ11|+|ξ12|≠0(16)

In brief, when the extension-twisting coupled laminates with three or more couplings are used to construct hygro-thermally stable composite structures, only ASBFDS laminate (triple-coupled) and ASBFDF laminate (quadruple-coupled) are presented, as shown in Table 1. As can be seen from the table, ASBtDS laminate is the single coupled laminate with extension-twisting coupling and shearing-bending coupling. Since these two couplings cannot exist separately, ASBtDS laminate is recorded as the single-coupled laminate. The necessary and sufficient conditions of the hygro-thermally stable ASBtDS laminate are given in [22].

3  Bending-Twisting Coupled Structures

There are two types of typical composite adaptive structures, namely bending-twisting coupled structure and extension-twisting coupled structure [23]. Both of these adaptive structures can be designed by laminates with extension-twisting coupling. The method of studying the extension-twisting coupled adaptive structure is similar to that of the bending-twisting coupled adaptive structure, so this article only focuses on only one adaptive structure. The bending-twisting coupled structure, which has broad application prospects in the field of aerospace and wind power, is studied here.

3.1 Mathematical Model

The geometric shape of bending-twisting coupled variable cross-section box structure is shown in Fig. 1, where the bending-twisting coupled structure is composed of an upper flange, a lower flange and two web laminates. Meanwhile, the size of laminate can be not limited to rectangular shape. The cross-sectional area from the fixed end to the free end gradually decreases. The reference frame indicates the frame of box structure with equal section, which is to help describe the size of the variable cross-section box structure. The angles between the upper and lower flanges with the vertical surfaces of the fixed end (surface C1C2C3C4 and surface C5C6C7C8) are β1 and β2 (as shown in vertical view), respectively. The angles between the two web laminates with the vertical surfaces of the fixed end (surface C1C5C8C4 and surface C2C6C7C3) are γ1 and γ2 (as shown in end view), respectively. The box structure can be applied to the analysis of the box section structure, such as the main load-bearing box segment wing structure.

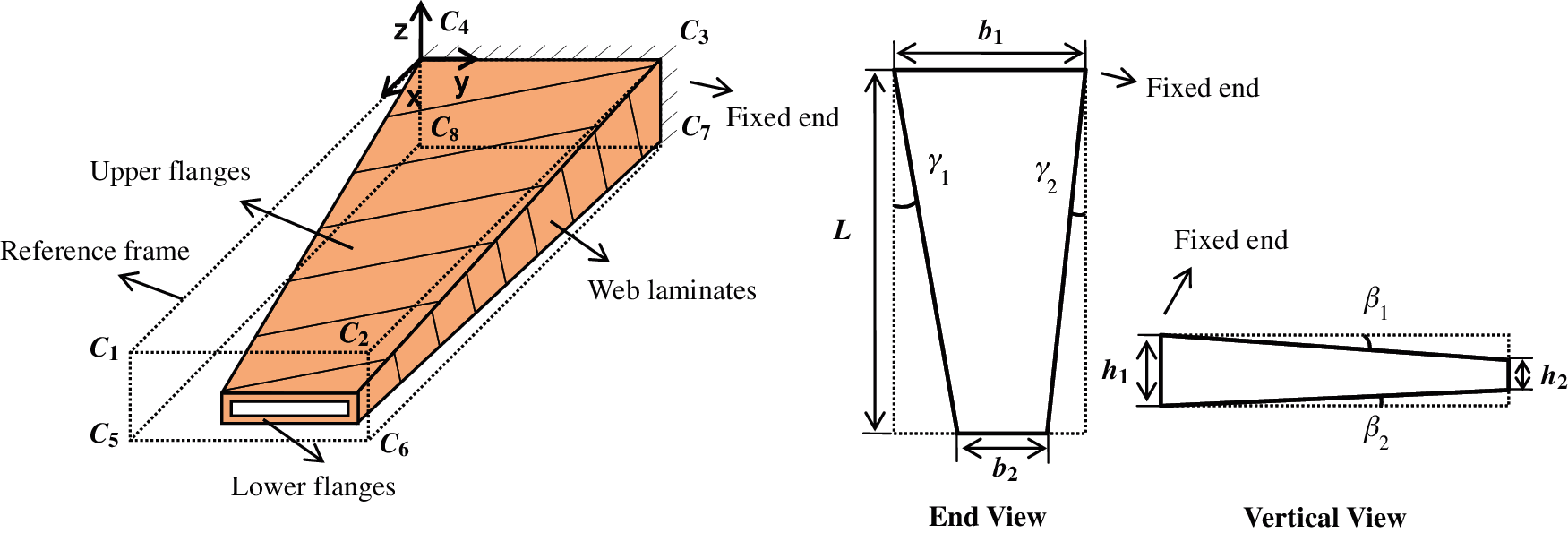
Figure 1: Geometric diagram of bending-twisting coupled variable cross-section box structure

Considering the outstanding performance of extension-twisting coupling of laminate on constituting the bending-twisting coupling of box structure, the laminates with extension-twisting coupling in Table 1 are taken to design the variable cross-section bending-twisting coupled box structure, hoping to maximize torsion deformation under the same bending moment. Thus, the optimization goal is to maximize the bending-twisting coupling of the box structure. Furthermore, merits and demerits of multi-couplings in the application of adaptive structures should be explored. The extension-twisting coupling of multi-coupled laminate mainly determines the bending-twisting coupling of box structure, so the optimization goal should also be to maximize extension-twisting coupling, which can be achieved by the extension-twisting coupled flexibility coefficient b16.

The torsion deformation of the box structure can be measured by the vertical (z-direction) displacement difference c of the two corner points of the free end of upper or lower flanges, as shown in Fig. 2. The design of the bending-twisting coupled structure with multi-coupling laminate makes the coupling performance of the structure more complicated, which leads to the difficulty in the analytical expression of the static deformation of the structure. Therefore, the parametric modeling method is used to achieve high-precision solution of the displacement of each point of bending-twisting coupled structure with variable size and stacking sequence, and the corresponding deformation results can be extracted with the help of the finite element software.

The wing of an executive jet transport (EJT) was used as a research object. In the light of the physical parameters of EJT, the wing size can be set as the lengths of the wing root, wing tip and wingspan are 4.57 m, 1.52 m and 8.26 m, respectively [24]. Considering the complex combination forms of actual wing structure and tiny ratio of skin thickness to wing span, it is pointless to apply the load directly to the skin structure. Therefore, a scaled model of wing structure was taken for the design optimization, with b1 = 100 mm, b2 = 33 mm and L = 180 mm. The height difference between the upper and lower flanges at the fixed end h1 is 10 mm, and the height difference at the free end h2 is 4 mm, β1=β2 and γ1=γ2. In view of the skin thickness is generally 2 mm, the number of layers n can be set to 12–16 if the thickness of lamina is 0.14 mm. The surface C4C8C7C3 (as shown in Fig. 1) of box structure is fixed, and other surfaces are no longer constrained by displacement. The material parameters of lamina are presented in Table 2 [18].

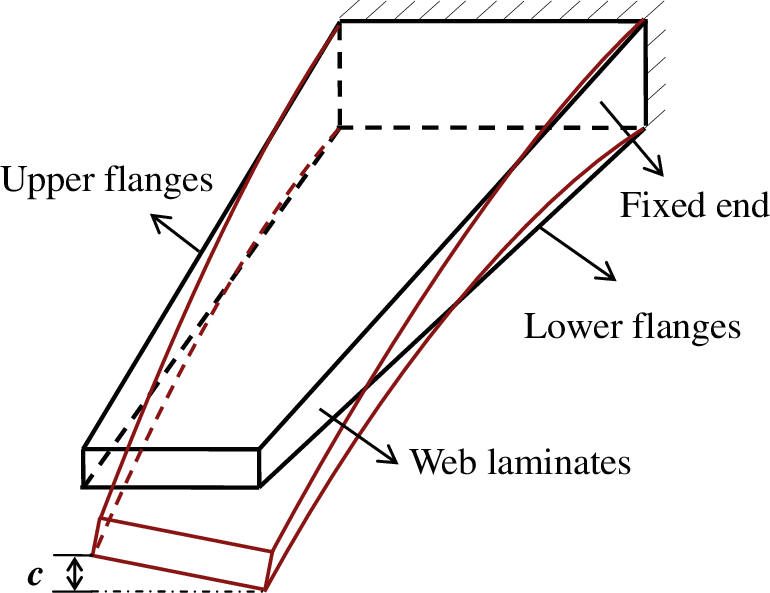
Figure 2: Schematic diagram of torsion deformation of the bending-twisting coupled box structure

The upper and lower flanges of the wing should produce torsion deformation in the same direction. The bending moment of box structure can be equivalent to the force of the same magnitude and opposite direction. Therefore, the paving angle of the upper and lower flanges should be opposite to each other. Compared with the upper and lower flanges, the web laminate is very small in size, and the laminate [60/−60/−60/0/0/0/60/60/0/−60/60/60/−60/−60/−60/0/0/60]T without any couplings is taken. The external bending moment M is 0.12 N⋅m. The constraint conditions are the relationships in Table 1. The optimal mathematical models for four extension-twisting coupled laminates in Table 1 and their bending-twisting coupled box structures are shown in Eqs. (17)–(20), respectively.

minF(θ1,θ2,…,θn)=−|b16|orminF(θ1,θ2,…,θn)=−cs.t.{−90∘≤θi≤90∘(i=1,2,…,n)ξ1=ξ3=ξ4=ξ5=ξ6=ξ7=ξ11=ξ12=0,ξ8≠0(17)

minF(θ1,θ2,…,θn)=−|b16|orminF(θ1,θ2,…,θn)=−cs.t.{−90∘≤θi≤90∘(i=1,2,..,n)ξ1=ξ3=ξ4=ξ5=ξ6=ξ7=0,ξ8≠0,|ξ11|+|ξ12|≠0(18)

minF(θ1,θ2,…,θn)=−|b16|orminF(θ1,θ2,…,θn)=−cs.t.{−90∘≤θi≤90∘(i=1,2,..,n)ξ1=ξ3=ξ4=ξ5=ξ7=ξ11=ξ12=0,ξ6≠0,ξ8≠0(19)

minF(θ1,θ2,…,θn)=−|b16|orminF(θ1,θ2,…,θn)=−cs.t.{−90∘≤θi≤90∘(i=1,2,..,n)ξ1=ξ3=ξ4=ξ5=ξ7=0,ξ6≠0,ξ8≠0,|ξ11|+|ξ12|≠0(20)

3.2 Optimization

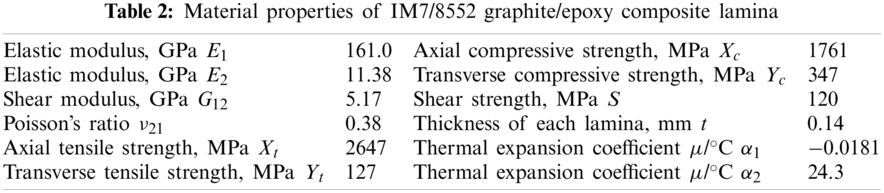
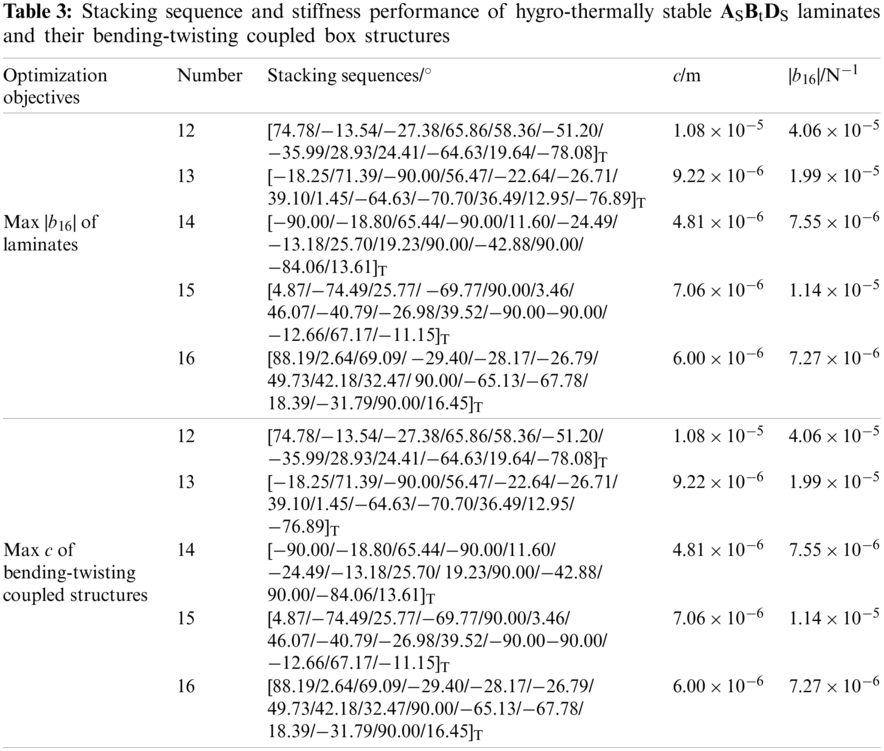
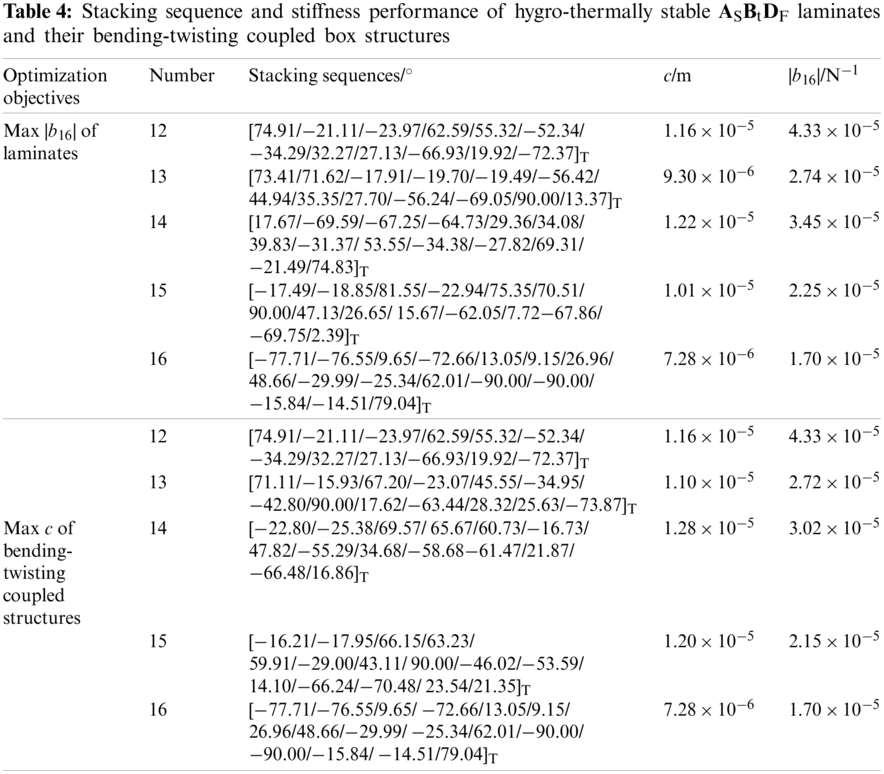
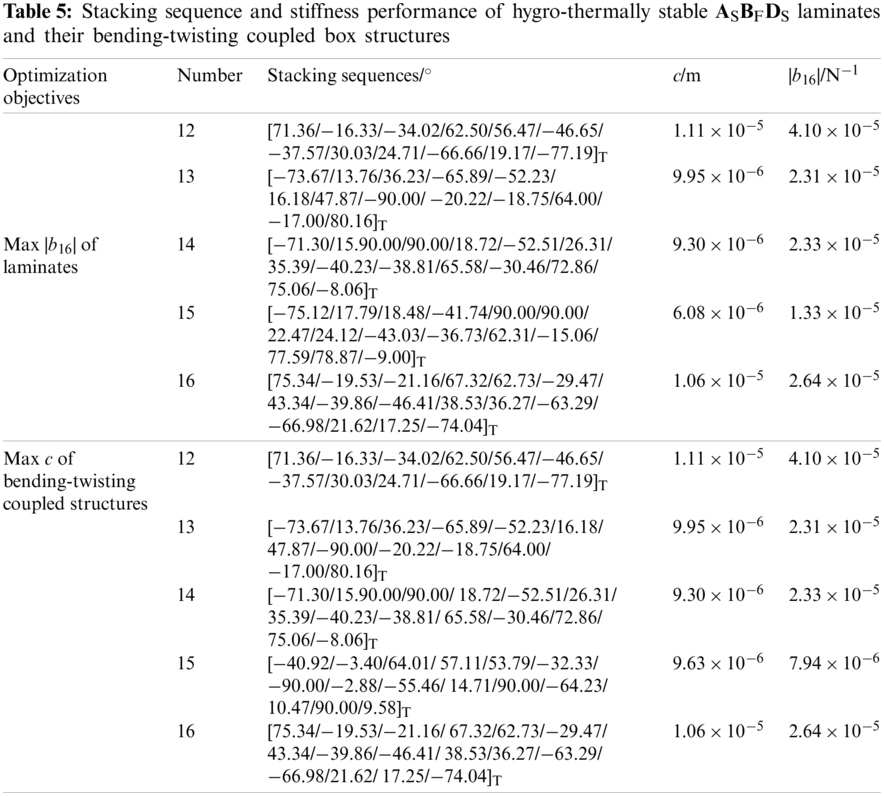
Owing to the strong nonlinear equality constraints, the DE_CMSBHS algorithm was used for optimization combined with the penalty function [18]. The initial population was about 15 times of n (number of plies). The evolutionary algebra was set as 3000 (large enough to ensure convergence). According to the optimized mathematical model shown in Eqs. (17)–(20), the optimal design of laminate and box structure was realized. Tables 3–6 show the stacking sequences, analytical solutions of extension-twisting coupling and torsion deformation of bending-twisting coupled structure, respectively.

3.3 Robustness

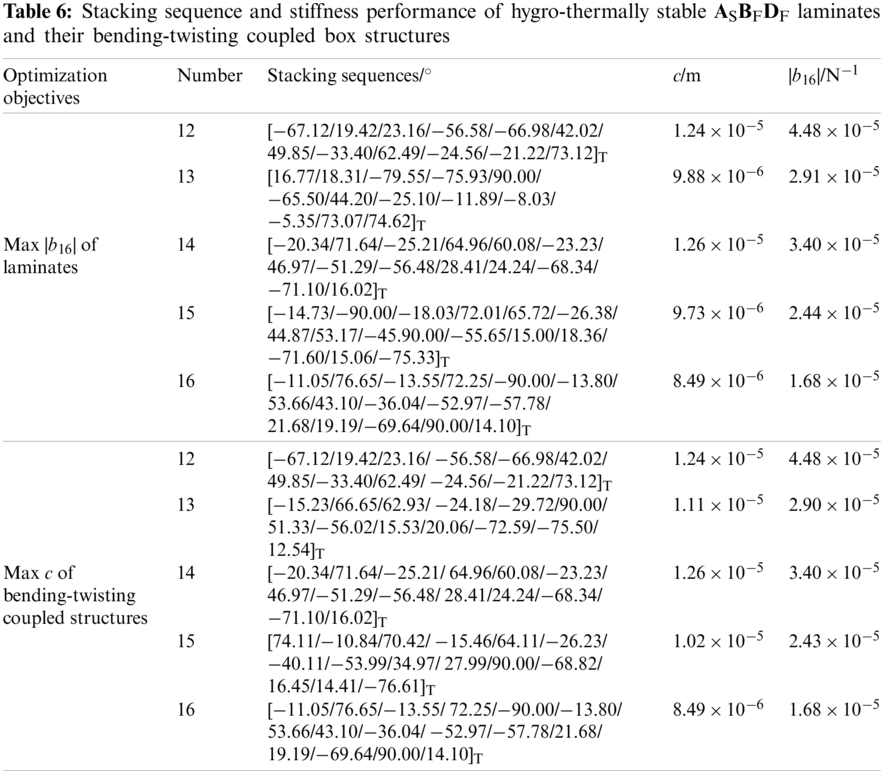
Considering that equipment and human errors are often unavoidable when laminates are laid by existing processing technologies, it is meaningful to test the deformation deviation caused by the paving angle deviation for free-layer laminate. Taking the 14-ply laminates with maximum |b16| as examples, the robustness of extension-twisting coupling was verified by using the Monte Carlo method.

Assume that the angle deviation of the k-ply lamina is 2∘ (Δθk=2∘) [10] and the number of random sampling is 20,000, the distribution of coupling deviations and its confidence intervals are illustrated in Fig. 3. The results indicated that the coupling deviations could be controlled within 4% at the confidence level of 95%. Furthermore, the deviations of ASBtDF and ASBFDF laminates were smaller than that of ASBtDS and ASBFDS laminates, implying that robustness could be improved by the bending-twisting coupling.

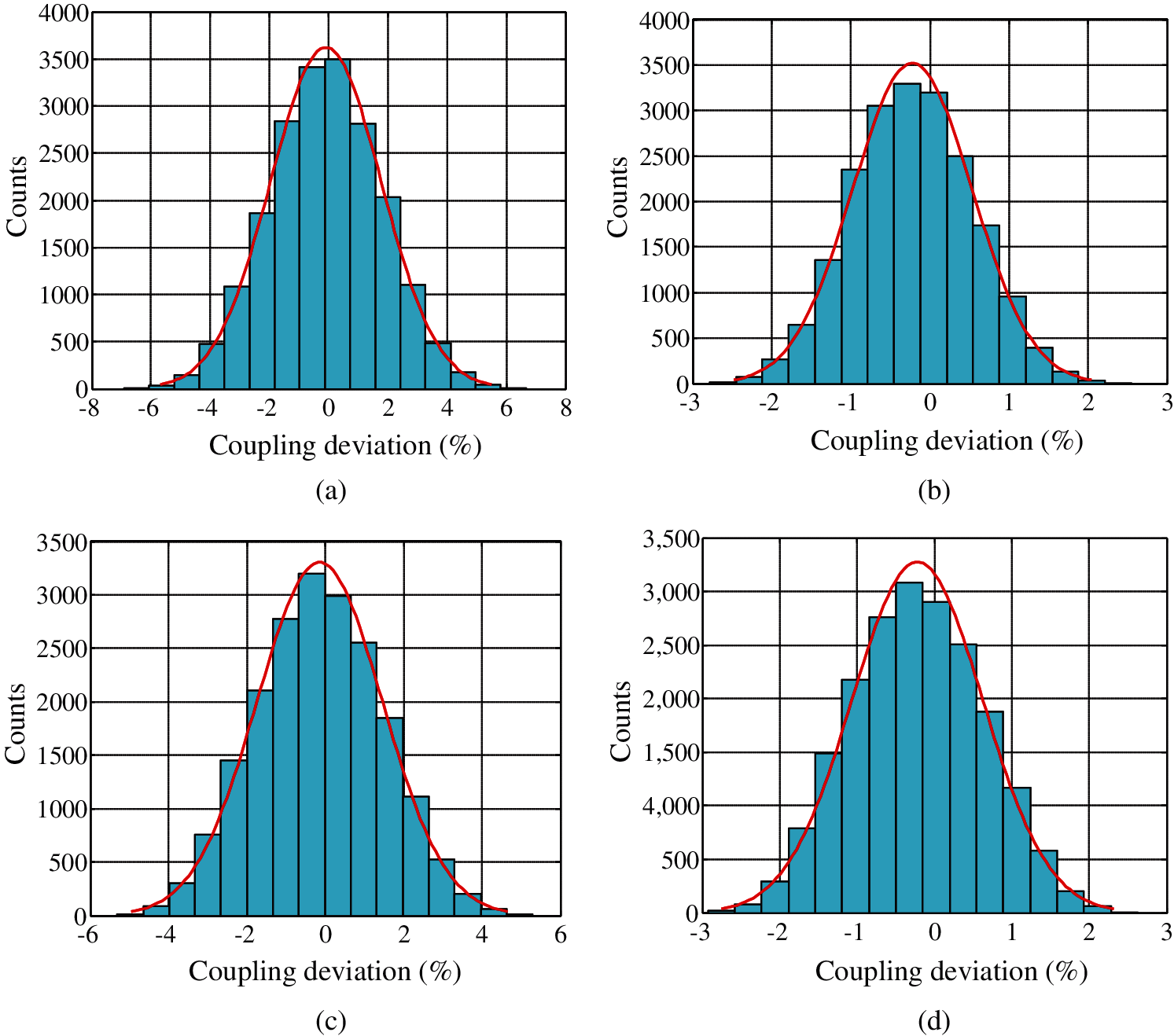
Figure 3: Deviation distribution of extension-twisting coupling of laminates based on 20,000 samples (a) ASBtDS laminate [−3.75%, 3.57%] (b) ASBtDF laminate [−1.70%, 1.28%] (c) ASBFDS laminate [−3.27%, 3.00%] (d) ASBFDF laminate [−1.88%, 1.44%]

4  Numerical Verification

4.1 Hygro-Thermal Stability

The hygro-thermal performance of laminates and box structures was tested by using the FEM, as shown in Tables 3–6. The laminate size was set as a parallel base length of 100 mm and 33 mm, and a height of 180 mm. The size of the box structure was the same as in the optimization process. The boundary condition was a fixed support at the bottom midpoint for laminate and box structure. In addition, the free end of box structure was constrained by the rigid surface RBE2. The laminate was divided into 300 shell elements, and the bending-twisting coupled structure was divided into 840 shell elements in total. The temperature difference was set as 180°C to simulate the curing process.

The free shrinkage deformation results under cooling of laminates and the bending-twisting coupled structure were solved by the software MSC.Nastran, as shown in Fig. 4. In order to analyze the effect of humidity changes on the deformation of laminates, the Abaqus software was used for numerical simulation. The laminate was divided into a total of 2,800 solid elements (Pore Fluid/Stress), and the bending-twisting coupled structure was divided into 27,200 solid elements in total. The relative humidity changed from 50% to 40%. The free contraction displacement cloud of laminates and box structures caused the humidity changes was solved by the “soils” function of the software Abaqus, as shown in Fig. 5. Only the results of 14-ply ASBtDS, ASBtDF, ASBFDS and ASBFDF laminate are presented here, and the results of other laminates are the same. The zero shear strain, bending and twisting curvatures results in both Figs. 4 and 5 illustrate the hygro-thermal stability.

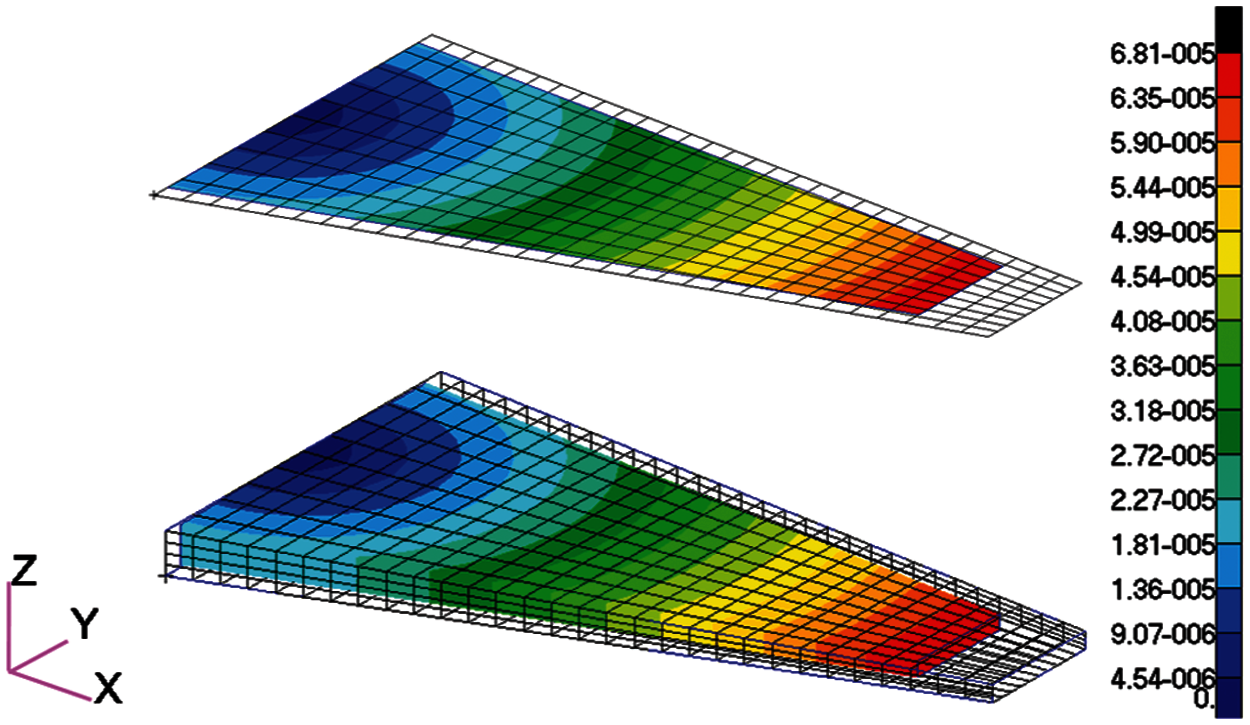
Figure 4: Cooling shrinkage displacement diagrams of 14-ply laminates with maximum extension-twisting coupling in Tables 3–6 (including ASBtDS laminate, ASBtDF laminate, ASBFDS laminate and ASBFDF laminate) and box structures due to the temperature changes, in meters

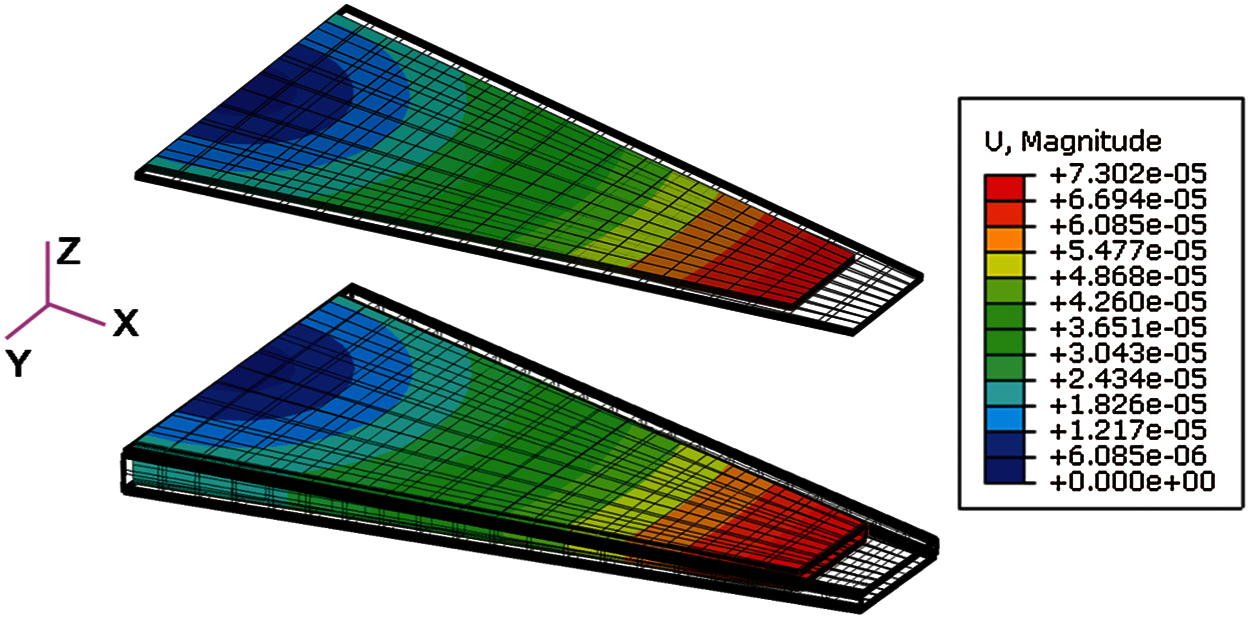
Figure 5: Free contraction displacement diagrams of 14-ply laminates with maximum extension-twisting coupling in Tables 3–6 (including ASBtDS laminate, ASBtDF laminate, ASBFDS laminate and ASBFDF laminate) and box structures due to the humidity changes, in meters

In addition, laminates with different paving angles produce the same thermal linear strains, which suggests that the hygro-thermal linear strain of laminate is independent of the stacking sequence. In view of the fact that the effect of humidity changes on laminates is similar to that of temperature changes, only the thermal coefficient needs to be replaced with the humidity coefficient. Thus, the reasons for this phenomenon were analyzed only from the perspective of temperature changes. The thermal linear strain of the laminate with extension-twisting coupling is expressed as

{εxTεyTγxyTκxTκyTκxyT}=[a11a120b11b12b16a12a220b12b22b2600a66b16b26b66b11b12b16d11d12d16b12b22b26d12d22d26b16b26b66d16d26d66]{NxTNyTNxyTMxTMyTMxyT}(21)

where the upper corner “T” represents variables related to thermal effects. Since the hygro-effect is similar to the thermal-effect, only the thermal-effect is presented. Then the thermal internal force and thermal moment of the composite laminate can be expressed by two independent parameters: the thermal constant of the material UiT only related to the material properties of each lamina in the laminate, and the geometric factor only related to the stacking sequence, namely

{NxTNyTNxyT}=HΔT2[1ξ11−ξ10ξ3][U1TU2T],{MxTMyTMxyT}=H2ΔT8[0ξ50−ξ50ξ7][U1TU2T](22)

The material thermal constant UiT(i=1,2) can be calculated by Ui and the thermal expansion coefficients αi(i=1,2).

U1T=(α1+α2)(U1+U4)+(α1−α2)U2U2T=(α1+α2)U2+(α1−α2)(U1+2U3−U4)(23)

Substituting Eq. (5) into Eq. (22), it gives

{NxTNyTNxyT}=HΔTU1T2{110},{MxTMyTMxyT}={000}(24)

Substituting Eq. (24) into Eq. (21), we can further get

{εxTεyTγxyT}=HΔTU1T2{a11+a12a12+a220}(25)

Inserting the common analytical conditions ξ1=ξ3=ξ4=ξ5=ξ7=0 of the four types of laminates in Table 1 into Eq. (2), it gives

{A11A12A22A66A16A26}=H{U1+ξ2U3U4−ξ2U3U1+ξ2U3U5−ξ2U300},{B11B12B22B66B16B26}=H24U3{ξ6−ξ6ξ6−ξ6ξ8−ξ8}(26)

Then Substituting Eq. (25) into Eq. (26), we can further get

{εxTεyTγxyT}=HΔTU1T2(A11+A12){110}=ΔTU1T2(U1+U4){110}(27)

From Eq. (27), we can see that γxyT is zero, and that the thermal strain in the two main axis directions is the same, which is only related to the material constant U1 and U4, thermal material constant U1T and temperature difference ΔT. Indeed, it has nothing to do with the number of layers and the stacking sequence of the laminate.

4.2 Mechanical Properties

In order to verify the stiffness performance of the laminates, the finite element model of a rectangular laminate with the size of 0.18 by 0.1 m was established. The purpose of using rectangular laminates for verification was to facilitate the extraction of the distortion of laminates. The external load was the axial uniform tension (400 N/m). 800 shell elements were divided and the geometric center of laminate was fixed. The size of the box structure was the same as in the optimal design process, and the bending moment of the free end of the structure M was 0.12 N⋅m. Displacement cloud diagrams of the laminates and the box structures were calculated. The results of 14-ply laminates are shown in Fig. 6 as examples. The results illustrate the deformation of laminate is consistent with the expected design, with the extension-twisting and corresponding couplings. Box-shaped structure produced not only bending deformation but also torsion deformation under the action of bending moment.

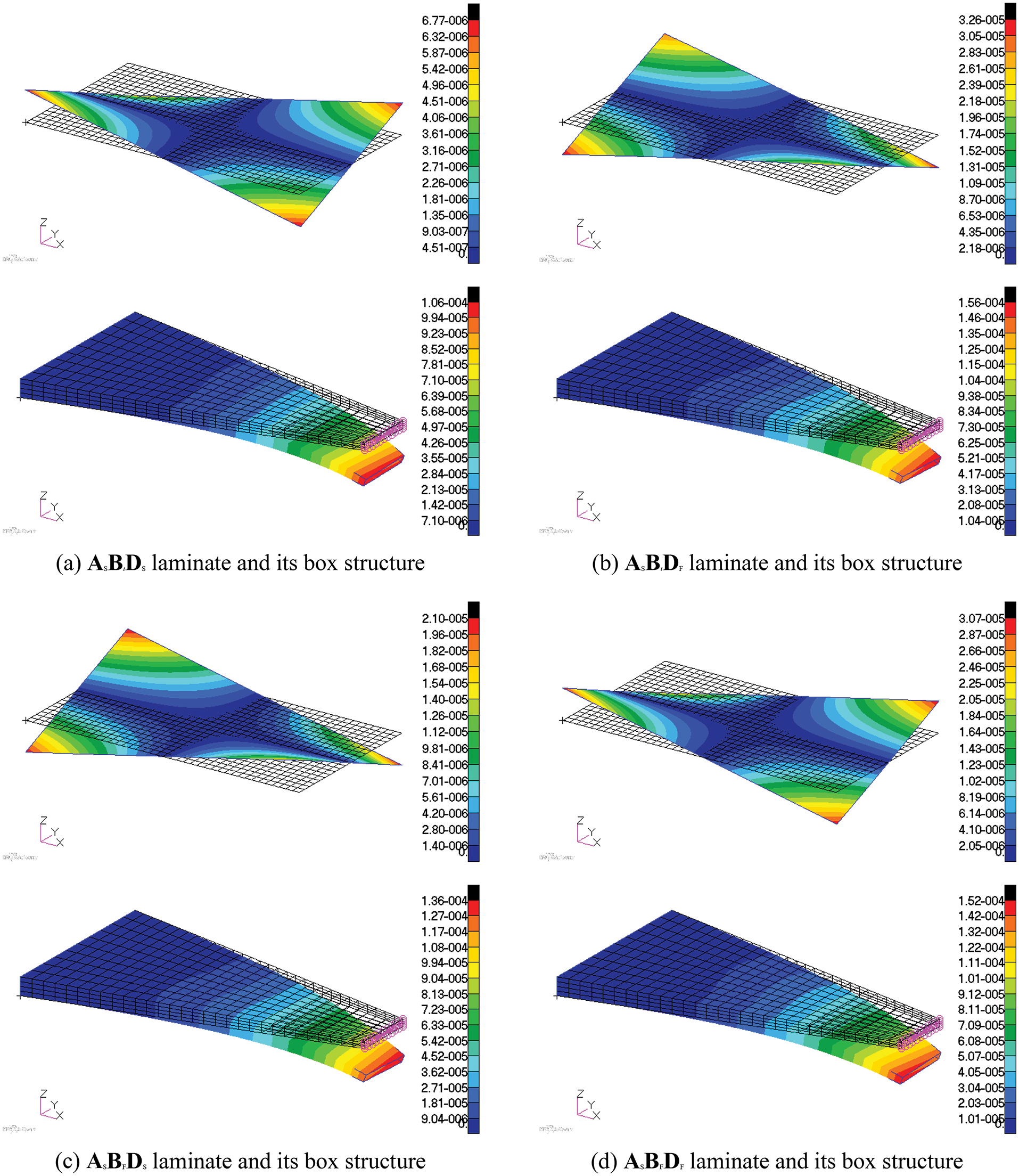
Figure 6: Displacement diagrams of laminates and bending-twisting coupled structures formed by respective laminates, in meters (a) ASBtDS laminate and its box structure (b) ASBtDF laminate and its box structure (c) ASBFDS laminate and its box structure (d) ASBFDF laminate and its box structure

The torsion angle φ (along the x axis) at the midpoint of the free end of rectangular laminate was used to express the torsion angle of the laminate. Assuming that the x-axis length of the laminate is L, the torsion curvature κxy can be expressed as [18]

κxy=2φL(28)

Table 7 shows the analytical and numerical solutions of torsion deformation of each laminate, as well as the percentage difference and improvement. The results illustrate that the simulation results of torsion deformation of laminate are consistent with the analytical results (difference within 1%), which verifies the accuracy of the extension-twisting coupling.

5  Impact Analysis

5.1 Coupling Effect

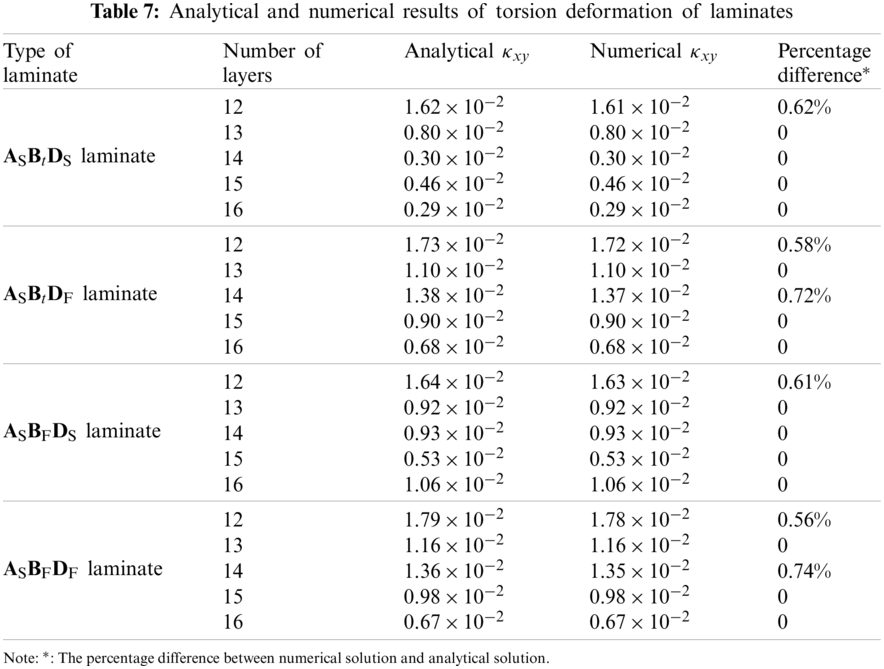
According to the optimization results in Tables 3–6, the comparison results of the extension-twisting coupling of laminates and the bending-twisting coupling of box structures were summarized, as shown in Tables 8 and 9.

The results show that: (1) Compared with the single-coupled ASBtDS laminate, the multi-coupled laminates (ASBtDF laminate, ASBFDS laminate and ASBFDF laminate) have a higher extension-twisting coupling, in which the extension-twisting coupling of 14-ply ASBtDF laminate is the maximum, reaching 456.95%; (2) For ASBtDS laminate, the same optimization results were obtained when optimizing with the maximum |b16| and c as objectives, which verified that the extension-twisting coupling of single coupled laminate was the decisive factor that constituted the bending-twisting coupling of box structure; (3) Compared with the box structures based on ASBtDS laminates, the torsion deformations of box structures based on multi-coupled laminates were significantly improved, wherein, the box structure composed of 14-ply ASBtDF laminate is the most obvious (increased to 266.11%), indicating that multi-couplings will obviously improve the bending-twisting coupling of box structure; (4) Comparing the box structures composed of multi-coupled laminates with the same number of layers and the type of coupling in Tables 8 and 9, it can be found that the extension-twisting coupling of laminates becomes smaller but the torsion deformation of the box structure still increases. Taking the box structure composed of 15-ply ASBFDS laminate as an example, when |b16| of laminate changed from 1.33 × 10−5 N−1 to 7.94 × 10−6 N−1, the c of box structure changed from 6.08 × 10−6 m to 9.63 × 10−6 m, indicating that other couplings except for the extension-twisting coupling (i.e., shearing-twisting and extension-bending) have the potential to enhance the bending-twisting coupling of box structure.

5.2 Failure Strength

When the axial external load of laminate increases gradually, the destruction will occur first on one lamina. This will affect the rigidity of the entire laminate, and may cause further damage to other laminae. Thus, the failure load should be considered during the design process. The tension and compression external loads of first ply failure Nt and Nc are selected to judge the failure strength. Under the axial load N1, the middle surface strains and curvatures of laminate can be calculated by the flexibility equation, namely

{εx0εy0γxy0κxκyκxy}=[a11a12a16b11b12b16a12a22a26b12b22b26a16a26a66b16b26b66b11b12b16d11d12d16b12b22b26d12d22d26b16b26b66d16d26d66]{N100000}(29)

Then, the strain of each lamina is expressed as

[εxεyγxy]k=[εx0εy0γxy0]+zk[κxκyκxy](30)

and the principal stresses of each lamina is expressed as

[σ1σ2τ12]k=Qk(Tk−1)T[εxεyγxy]k(31)

where, Tk and Qk are the conversion matrix of stress direction and positive axis stiffness coefficient matrix of k-ply lamina, namely

Tk=[cos2θksin2θk2sin⁡θkcos⁡θksin2θkcos2θk−2sin⁡θkcos⁡θk−sin⁡θkcos⁡θksin⁡θkcos⁡θkcos2θk−sin2θk],Qk=[Q11Q120Q12Q11000Q66]k(32)

The choice of strength theory depends on the actual laminate composite material. Studies have shown that for glass fiber/epoxy composite materials and composite materials with the same tensile strength and compressive strength, the Hill-Tsai strength theory is close to the experimental value in both qualitative and quantitative aspects, while the Tsai-Wu criterion is suitable for composite materials with different tensile and compressive strengths [25]. Because the tensile and compressive strength of lamina made of carbon fiber/epoxy prepreg are different, the Tsai-Wu strength criterion should be more applicable for the strength prediction. The principal stress of each lamina satisfies the relationships of

F1σ1+F2σ2+F6τ12+F11σ12+F22σ22+F66τ122+2F12σ1σ2=1{F1=1Xt−1Xc,F11=1XtXc,F2=1Yt−1Yc,F22=1YtYc,F6=0,F66=1S2,F12=−12(XtXcYtYc)−1/2(33)

where, Xt(c) and Yt(c) represent the axial and transverse tensile (compressive) strength of lamina, S represents the shear strength. Because the value of F12 in the Tsai-Wu strength criterion is not easy to be determined, the Tsai-Wu quadratic failure criterion based upon the generalized von Mises isotropic criterion is used here to take the value of F12 [26]. The advantage of this widely used value method is its accuracy and relative simplicity [27,28].

Substituting the paving angle of each lamina into the stiffness equation, we can obtain the flexibility matrix coefficient of laminates. Then, combining Eqs. (29)–(31) with Eq. (33) gives the value of the external load Nmax(k) at which each lamina fails. At this time, the external load Nmax(k) corresponding to the k-ply lamina has two solutions: the positive solution Nmax1(k) is the maximum tensile load, and the negative solution Nmax2(k) is the maximum compressive load. Then, Nt,Nc can be obtained through the smallest |Nmax1(k)|(min) and |Nmax2(k)|(min)(k=1,2,…,n), namely

Nt=|Nmax1(k)|(min),Nc=|Nmax2(k)|(min)(34)

where NT and NC represent the tension and compression failure strengths of first ply failure, namely

NT=NtH,NC=NcH(35)

Taking the laminates with maximum |b16| as examples in Tables 3–6, the NT and NC of various types of laminates were calculated respectively to explore the influence of the multi-couplings on failure strengths. The analysis results of failure strengths for laminates with extension-twisting coupling are shown in Table 10. The dimensions, forces and boundary conditions of the laminates are consistent with those in Section 4.2. Data shows that: (1) The tensile strengths are generally less than the compressive strengths, except for the 13-ply ASBFDF laminate, indicating that in the actual engineering application of laminates with extension-twisting coupling, the tensile strength performance should be considered firstly in terms of strength performance. (2) Compared with the single extension-twisting coupled laminate, the failure strengths of multi-coupled laminates are reduced to varying degrees, illustrating the negative impact of multi-couplings on failure strength.

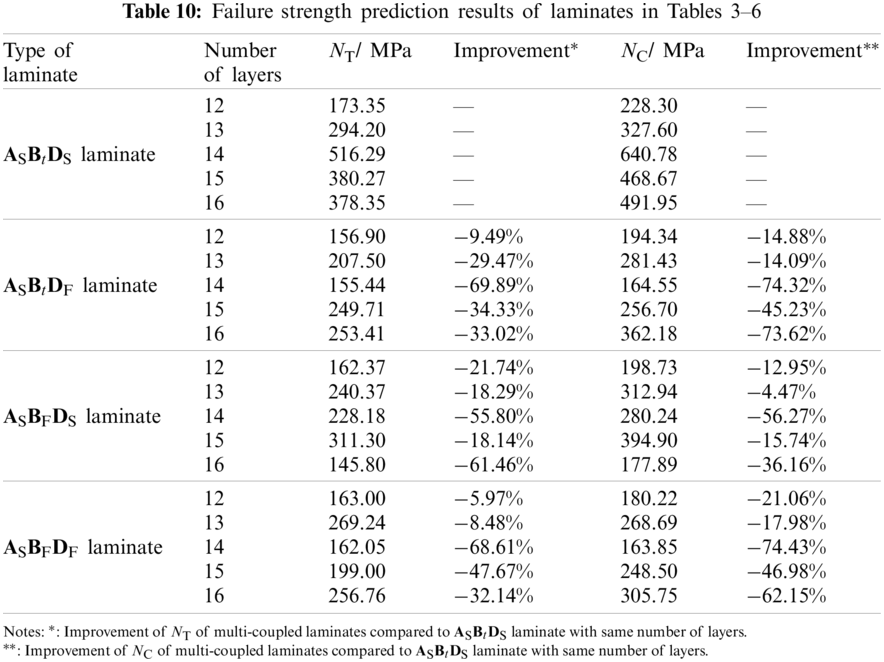
However, the negative impact of strength performance can be minimized through reasonable paving methods. Taking 14-ply ASBtDF laminate as an example, whose failure strengths NT and NC are greatly reduced (−69.89% and −74.32%, respectively), let us assume that the lower limits of the failure strengths NT0 and NC0 are 60%, 70%, 80%, 90% and 100% of those of the 14-ply ASBtDS laminate in Table 9, respectively. Then, the corresponding optimization mathematical model is expressed as

minF(θ1,θ2,…,θ14)=−|b16|s.t.{−90∘≤θi≤90∘(i=1,2,…,14)ξ1=ξ3=ξ4=ξ5=ξ6=ξ7=0,ξ8≠0,|ξ11|+|ξ12|≠0NT≥NT0,NC≥NC0(36)

Table 11 shows the stacking sequence and mechanical properties of 14-ply ASBtDF laminates, mainly including extension-twisting couplings and failure strength. The hygro-thermal stability and extension-twisting coupling were verified by numerical simulation. The results demonstrate that: (1) The failure strength of the optimized laminate meets the requirement of lower limit; (2) As NT0 and NC0 increase, the maximum extension-twisting coupling of laminate gradually decreases. (3) The negative impact of strength performance of multi-coupled laminates can be minimized by reasonable paving design and optimization under different suitable constraint conditions.

5.3 Bucking Load

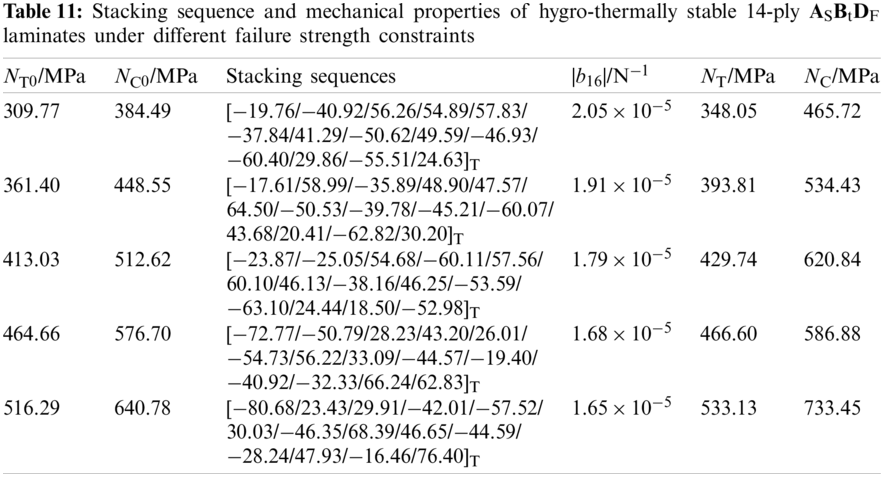
With the increase of the in-plane compression load, the multi-coupled laminate entered an unstable equilibrium. Once the load continued to increase, buckling occurred, which further affected the mechanical properties. Multi-couplings may increase the out-of-plane deformation under the in-plane load, which will lead to earlier buckling. Thus, it is very important to determine the influence of multiple coupling on the buckling load.

The analytical solution of buckling load N¯x can be solved based on the buckling differential equation. For example, reference [17] gives the analytical solution of buckling load of single extension-twisting coupled ASBtDS laminates under the condition of four-sided simply supported boundary displacement. Although the analytical method is highly accurate and convenient for subsequent optimization design, it has poor applicability under different boundary conditions, mainly manifested as follows: (1) The buckling load of laminates under different boundary conditions is difficult to express analytically; (2) Except the extension-twisting coupling, the multi-coupled laminates also have extension-bending coupling (ASBFDS laminate and ASBFDF laminate) and bending-twisting coupling (ASBtDF laminate and ASBFDF laminate). As a result, the commonly used series method, variation method and other methods are unable to get a solution form satisfying the constraint conditions and the analytical solution is difficult to obtain. Therefore, the FEM and parametric modeling were used to obtain the buckling loads by MSC.Patran and Matlab software under various boundary conditions. A rectangular laminated plate was taken as an example for analysis. It was assumed that the size was 0.18 meters long and 0.1 meters wide, and that the in-plane lengthwise external load was 400 N/m.

Taking the laminates with maximum |b16| as examples in Tables 3–6, the size, force and boundary conditions of laminate are consistent with laminates in Section 4.2. Table 12 shows the buckling performance of laminates, mainly including the buckling factors and corresponding buckling loads. The results demonstrate that: compared with the single extension-twisting coupled laminate, the buckling loads of multi-coupled laminates were reduced to varying degrees, illustrating the negative impact of multi-couplings on buckling loads.

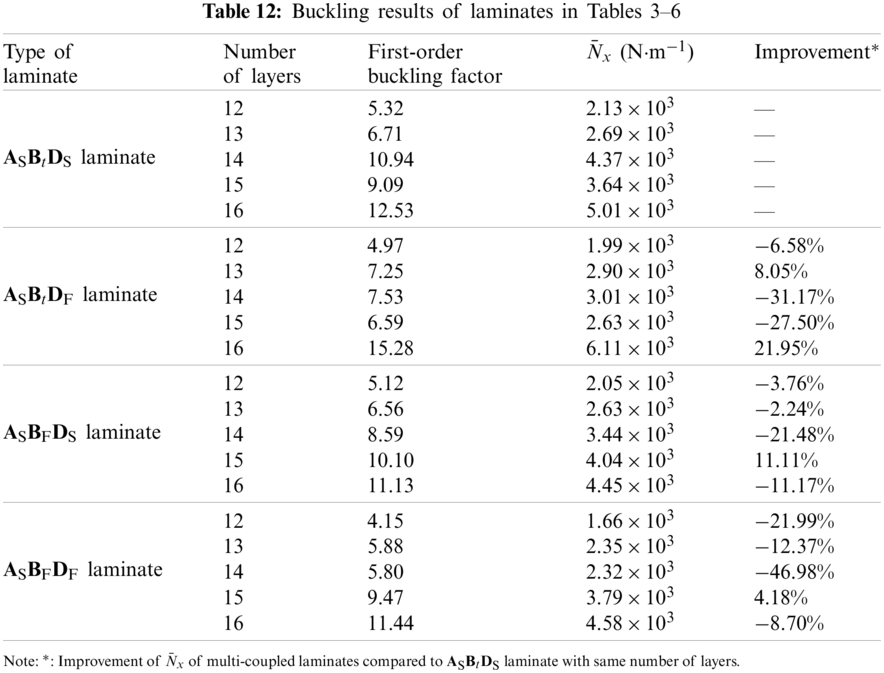
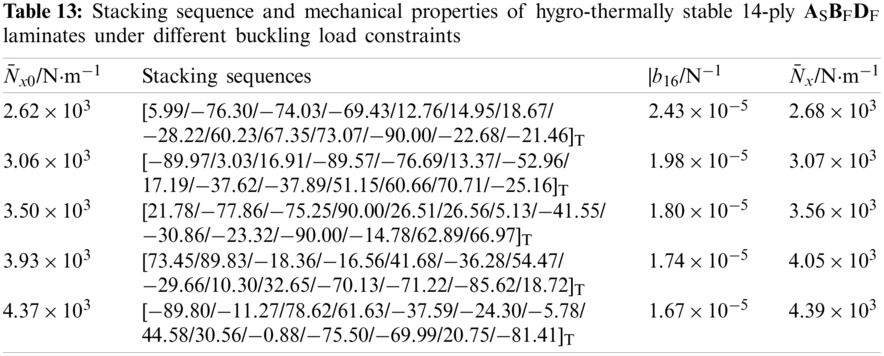
However, the negative impact of buckling can be minimized through reasonable paving design [13,17,20]. Actually, this problem can be equivalent to an optimization design problem with objective constraints. In view of the fact that the buckling load of laminate in this article was difficult to directly analytically express, the method of parametric modeling was also used to achieve this problem. Taking 14-ply ASBFDF laminate as an example, whose buckling load reduced the most (−46.98%), let us assume that the lower limits of the buckling load (N¯x0) are 60%, 70%, 80%, 90% and 100% of buckling load of the 14-ply ASBtDS laminate in Table 9, then the corresponding optimization mathematical model is expressed as

minF(θ1,θ2,…,θ14)=−|b16|s.t.{−90∘≤θi≤90∘(i=1,2,..,14)ξ1=ξ3=ξ4=ξ5=ξ7=0,ξ6≠0,ξ8≠0,|ξ11|+|ξ12|≠0N¯x≥N¯x0(37)